Exercise 2.2: Multi-Level Signals
From LNTwww
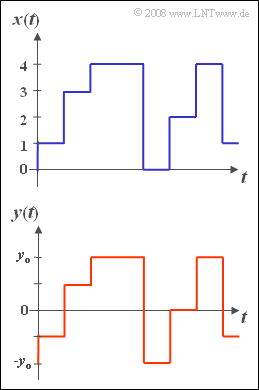
Let the square wave signal x(t) be dimensionless and can only have the current values 0, 1, 2, ... , M−2, M−1 with equal probability. The upper graph shows this signal for the special case M=5.
The square wave signal y(t) is also M–stepped, but zero mean and restricted to the range from y>−y0 to y<+y0 .
In the graph below you can see the signal y(t), again for the number of steps M=5.
Hints:
- The exercise belongs to the chapter Moments of a Discrete Random Variable.
- The topic of this chapter is illustrated with examples in the (German language) learning video
Calculating Moments for Discrete-Valued Random Variables ⇒
- The topic of this chapter is illustrated with examples in the (German language) learning video
- Fur numerical calculations, use y0=2V.
Questions
Musterlösung
(1) Man erhält durch Mittelung über alle möglichen Signalwerte für den linearen Mittelwert:
- mx=M−1∑μ=0pμ⋅xμ=1M⋅M−1∑μ=0μ=1M⋅(M−1)⋅M2=M−12.
- Im Sonderfall M=5 ergibt sich der lineare Mittelwert zu mx=2_.
(2) Analog gilt für den quadratischen Mittelwert:
- m2x=M−1∑μ=0pμ⋅x2μ=1M⋅M−1∑μ=0μ2=1M⋅(M−1)⋅M⋅(2M−1)6=(M−1)⋅(2M−1)6.
- Im Sonderfall M=5 ergibt sich der quadratische Mittelwert zu m2x=6.
- Daraus kann die Varianz mit dem Satz von Steiner berechnet werden:
- σ2x=m2x−m2x=(M−1)⋅(2M−1)6−(M−1)24=M2−112.
- Im Sonderfall M=5 ergibt sich für die Varianz σ2x=2_.
(3) Aufgrund der Symmetrie von y gilt unabhängig von M:
- mx=2_.
(4) Zwischen x(t) und y(t) gilt folgender Zusammenhang:
- y(t)=2⋅y0M−1⋅[x(t)−mx].
- Daraus folgt für die Varianzen:
- σ2y=4⋅y20(M−1)2⋅σ2x=y20⋅(M2−1)3⋅(M−1)2=y20⋅(M+1)3⋅(M−1).
- Im Sonderfall M=5 ergibt sich hierfür:
- σ2y=y20⋅63⋅4=2V2_.