Exercise 1.08Z: Equivalent Codes

• Return to book

Vier verschiedene (6, 3)–Blockcodes

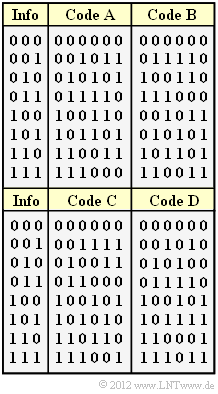
In der Grafik sind die Zuordnungen $\underline{u} → \underline{x}$ für verschiedene Codes angegeben, die im Folgenden jeweils durch die Generatormatrix G und die Prüfmatrix H charakterisiert werden:

• $\color{red}{\boldsymbol{\rm Code \ A}}$:

$${ \boldsymbol{\rm G}}_{\rm A} = \begin{pmatrix} 1 &0 &0 &1 &1 &0\\ 0 &1 &0 &1 &0 &1\\ 0 &0 &1 &0 &1 &1 \end{pmatrix} \hspace{0.05cm},$$

$${ \boldsymbol{\rm H}}_{\rm A} = \begin{pmatrix} 1 &1 &0 &1 &0 &0\\ 1 &0 &1 &0 &1 &0\\ 0 &1 &1 &0 &0 &1 \end{pmatrix} \hspace{0.05cm}.$$

• $\color{red}{\boldsymbol{\rm Code \ B}}$:

$${ \boldsymbol{\rm G}}_{\rm B} = \begin{pmatrix} 0 &0 &1 &0 &1 &1\\ 1 &0 &0 &1 &1 &0\\ 0 &1 &1 &1 &1 &0 \end{pmatrix} \hspace{0.05cm},$$

$$ { \boldsymbol{\rm H}}_{\rm B} = \begin{pmatrix} 1 &0 &1 &0 &1 &0\\ 1 &1 &0 &1 &0 &0\\ 0 &1 &1 &0 &0 &1 \end{pmatrix} \hspace{0.05cm}.$$

• $\color{red}{\boldsymbol{\rm Code \ C}}$:

$${ \boldsymbol{\rm G}}_{\rm C} = \begin{pmatrix} 1 &0 &0 &1 &0 &1\\ 0 &1 &0 &0 &1 &1\\ 0 &0 &1 &1 &1 &1 \end{pmatrix} \hspace{0.05cm},{ \boldsymbol{\rm H}}_{\rm C} = \begin{pmatrix} 1 &0 &1 &1 &0 &0\\ 0 &1 &1 &0 &1 &0\\ 1 &1 &1 &0 &0 &1 \end{pmatrix} \hspace{0.05cm},$$

• $\color{red}{\boldsymbol{\rm Code \ D}}$:

$${ \boldsymbol{\rm G}}_{\rm D} = \begin{pmatrix} 1 &0 &0 &1 &0 &1\\ 0 &1 &0 &1 &0 &0\\ 0 &0 &1 &0 &1 &0 \end{pmatrix} \hspace{0.05cm},{ \boldsymbol{\rm H}}_{\rm D} = \begin{pmatrix} 1 &1 &0 &1 &0 &0\\ 0 &0 &1 &0 &1 &0\\ 1 &0 &0 &0 &0 &1 \end{pmatrix} \hspace{0.05cm}.$$

In dieser Aufgabe soll untersucht werden, welche dieser Codes bzw. Codepaare

• systematisch sind,
• identisch sind (das heißt: Verschiedene Codes haben gleiche Codeworte),
• äquivalent sind (das heißt: Verschiedene Codes haben gleiche Codeparameter).

Hinweis :

Die Aufgabe gehört zum Themengebiet von Kapitel Allgemeine Beschreibung linearer Blockcodes Anzumerken ist, dass die Angabe einer Prüfmatrix H nicht eindeutig ist. Verändert man die Reihenfolge der Prüfgleichungen, so entspricht dies einer Vertauschung von Zeilen.

Fragebogen

1

Welche der nachfolgend aufgeführten Codes sind systematisch?

	Code A,
	Code B,
	Code C,
	Code D.

2

Welche der vorgegebenen Codepaare sind identisch?

	Code A und Code B,
	Code B und Code C,
	Code C und Code D.

3

Welche der gegebenen Codepaare sind äquivalent, aber nicht identisch?

	Code A und Code B,
	Code B und Code C,
	Code C und Code D.

4

Wie unterscheiden sich die Generatormatrizen $G_{\rm B}$ und $G_{\ rm C}$?

	Durch verschiedene Linearkombinationen verschiedener Zeilen.
	Durch zyklische Vertauschung der Zeilen um 1 nach unten.
	Durch zyklische Vertauschung der Spalten um 1 nach rechts.

5

Bei welchen Codes gilt ${ \boldsymbol{\rm H}} · { \boldsymbol{\rm G}}^{\rm T} = \boldsymbol{0}$?

	Code A,
	Code B,
	Code C,
	Code D.

Musterlösung

Solution

1. 2. 3. 4. 5. 6. 7.