Exercise 1.4: Rayleigh PDF and Jakes PDS

• Return to book

PDF and  $|z(t)|$  for Rayleigh Fading with Doppler effect

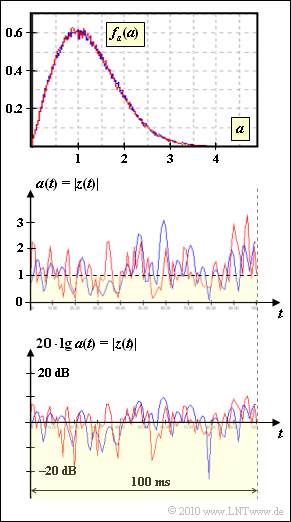
We consider two different mobile radio channels with  Rayleigh fading. In both cases the PDF of the magnitude  $a(t) = |z(t)| ≥ 0$  is

$$f_a(a) = \frac{a}{\sigma^2} \cdot {\rm e}^{ -{a^2}/(2\sigma^2)} \hspace{0.05cm}.$$

The probability that this amount is not greater than a given value  $A$  is

$${\rm Pr}(|z(t)| \le A) = 1 - {\rm e}^{ -{A^2}/(2\sigma^2)} \hspace{0.05cm}.$$

The two channels, which are designated according to the colors „Red” and „Blue” in the graphs with  $\rm R$  and  $\rm B$  respectively, differ in the speed  $v$  and thus in the form of the power spectral density (PSD)   ${\it \Phi}_z(f_{\rm D})$.

• In both cases, however, the PSD is a Jakes spectrum.

• For a Doppler frequency  $f_{\rm D}$  with  $|f_{\rm D}| <f_{\rm D,\hspace{0.05cm}max}$  the Jakes spectrum is given by

$${\it \pi}_z(f_{\rm D}) = \frac{1}{\pi \hspace{-0.05cm}\cdot \hspace{-0.05cm}f_{\rm D, \hspace{0.05cm} max} \hspace{-0.05cm}\cdot \hspace{-0.05cm}\sqrt{ 1 \hspace{-0.05cm}- \hspace{-0.05cm}(f_{\rm D}/f_{\rm D, \hspace{0.05cm} max})^2} } \hspace{0.05cm}.$$

• For Doppler frequencies outside this interval from  $-f_{\rm D,\hspace{0.05cm}max}$  to  $+f_{\rm D,\hspace{0.05cm}max}$,   we have ${\it \pi}_z(f_{\rm D})=0$.

The corresponding descriptor in the time domain is the autocorrelation function (ACF):

$$\varphi_z ({\rm \delta}t) = 2 \sigma^2 \cdot {\rm J_0}(2\pi \cdot f_{\rm D, \hspace{0.05cm} max} \cdot {\rm \delta}t)\hspace{0.05cm}.$$

• Here,  ${\rm J_0}(.)$  is the Bessel function of the first kind and zeroth order. We have   ${\rm J_0}(0) = 1$.
• The maximum Doppler frequency of the channel model  $\rm R$ : is known to be   $f_{\rm D,\hspace{0.05cm}max} = 200 \ \rm Hz$.
• It is also known that the speeds  $v_{\rm R}$  and  $v_{\rm B}$  differ by the factor  $2$ .
• Whether  $v_{\rm R}$  is twice as large as  $v_{\rm B}$  or vice versa, you should decide based on the above graphs.

Notes:

• This task belongs to the topic of  Statistical bindings within the Rayleigh process.
• To check your results you can use the interactive applet  PDF, CDF and Moments of Special Distributions.

Questionns

1

Determine the Rayleigh parameter  $\sigma$  for the channels  $\rm R$  and  $\rm B$.

$\sigma_{\rm R} \ = \ $ $\ \ \rm$

$\sigma_{\rm B} \ = \ $ $\ \ \rm$

2

In each case, give the probability that  $20 \cdot {\rm lg} \ a ≤ –10 \ \ \ \rm dB$  which is also  $a ≤ 0.316$  at the same time.

Channel  ${\rm R}\text{:} \hspace{0.4cm} {\rm Pr}(a ≤ 0.316) \ = \ $ $\ \rm \%$

Channel  ${\rm B}\text{:} \hspace{0.4cm} {\rm Pr}(a ≤ 0.316) \ = \ $ $\ \rm \%$

3

Which statements are correct regarding the driving speeds  $v$ ?

	$v_{\rm B}$  is twice as big as  $v_{\rm R}$.
	$v_{\rm B}$  is half as big as  $v_{\rm R}$.
	With  $v = 0$,   $|z(t)|$  would be constant.
	With  $v = 0$   $|z(t)|$  would have a white spectrum.
	With  $v → ∞$   $|z(t)|$  would be constant.
	With  $v → ∞$   $|z(t)|$  would be white.

4

Which of the following statements are correct?

	The PSD value  ${\it \Phi_z}(f_{\rm D} = 0)$  is the same for both channels.
	The ACF value  $\varphi_z(\Delta t = 0)$  is the same for both channels.
	The area under  ${\it \Phi_z}(f_{\rm D})$  is the same for both channels.
	The area below  $\varphi_z(\Delta t)$  is the same for both channels.

Solutions

Solution

(1)  The maximum value of the PDF for both channels is $0.6$ and occurs at $a = 1$.

• The Rayleigh PDF and its derivative are

$$f_a(a) \hspace{-0.1cm} = \hspace{-0.1cm} \frac{a}{\sigma^2} \cdot {\rm e}^{ -a^2/(2\sigma^2)} \hspace{0.05cm},$$

$$\frac{{\rm d}f_a(a)}{{\rm d}a} \hspace{-0.1cm} = \hspace{-0.1cm} \frac{1}{\sigma^2} \cdot {\rm e}^{ -a^2/(2\sigma^2)}- \frac{a^2}{\sigma^4} \cdot {\rm e}^{ -a^2/(2\sigma^2)} \hspace{0.05cm}.$$

• By setting the derivative to $0$, you can show that the maximum of the PDF occurs at $a = \sigma$. Since the Rayleigh PDF applies to both channels, it follows that

$$\sigma_{\rm R} = \sigma_{\rm B} \hspace{0.15cm} \underline{ = 1} \hspace{0.05cm}.$$

(2)  As they fading coefficients have the same PDF, the desired probability is also the same for both channels.

• Using the given equation, we have

$${\rm Pr}(a \le 0.316) = {\rm Pr}(20 \cdot {\rm lg}\hspace{0.15cm} a \le -10\,\,{\rm dB}) = 1 - {\rm e}^{ -{0.316^2}/(2\sigma^2)} = 1- 0.951 \hspace{0.15cm} \underline{ \approx 4.9 \%} \hspace{0.05cm}.$$

(3)  The correct solutions are 2, 3 and 6:

• The smaller speed $v_{\rm B}$ can be recognized by the fact that the magnitude $|z(t)|$ changes more slowly with the blue curve.
• When the vehicle is stationary, the PSD degenerates to ${\it \Phi_z}(f_{\rm D}) = 2\sigma^2\cdot \delta(f_{\rm D})$, and we have $|z(t)| = A = \rm const.$, where the constant $A$ is drawn from the Rayleigh distribution.
• At extremely high speed, the Jakes spectrum becomes flat and has an increasingly smaller magnitude over an increasingly wide range. It then approaches the PSD of white noise. However, $v$ would have to be in the order of the speed of light.

(4)  Statements 2 and 3 are correct:

• The Rayleigh parameter $\sigma = 1$ also determines the „power” ${\rm E}[|z(t)|^2] = 2\sigma^2 = 2$ of the random process.
• This applies to both R and B:

$$\varphi_z ({\rm \delta}t = 0) = 2 \hspace{0.05cm}, \hspace{0.2cm} \int_{-\infty}^{+\infty}{\it \Phi}_z(f_{\rm D}) \hspace{0.15cm}{\rm d}f_{\rm D} = 2 \hspace{0.05cm}.$$