Exercise 3.6Z: Two Imaginary Poles

Two imaginary poles and one zero

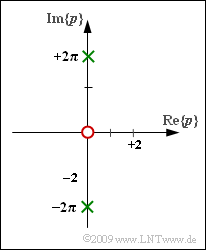
In this exercise, we consider a causal signal $x(t)$ with the Laplace transform

$X_{\rm L}(p) = \frac { p} { p^2 + 4 \pi^2}= \frac { p} { (p-{\rm j} \cdot 2\pi)(p+{\rm j} \cdot 2\pi)} \hspace{0.05cm}$

corresponding to the graph (one red zero and two green poles).

In contrast, the signal $y(t)$ has the Laplace spectral function

$Y_{\rm L}(p) = \frac { 1} { p^2 + 4 \pi^2} \hspace{0.05cm}.$

Thus, the red zero does not belong to $Y_{\rm L}(p)$ .

Abschließend wird noch das Signal $z(t)$ mit der Laplace–Transformierten

$Z_{\rm L}(p) = \frac { p} { (p-{\rm j} \cdot \beta)(p+{\rm j} \cdot \beta)} \hspace{0.05cm}$

betrachtet, insbesondere der Grenzfall für $\beta → 0$ .

Please note:

The exercise belongs to the chapter Inverse Laplace Transform.
Die Frequenzvariable $p$ ist so normiert, dass nach Anwendung des Residuensatzes die Zeit $t$ in Mikrosekunden angegeben ist.
Ein Ergebnis $t = 1$ ist somit als $t = T$ mit $T = 1 \ \rm µ s$ zu interpretieren.
Der residue theorem lautet am Beispiel der Funktion $X_{\rm L}(p)$ mit zwei einfachen Polstellen bei $\pm {\rm j} \cdot \beta$ :

$x(t) = X_{\rm L}(p) \cdot (p - {\rm j} \cdot \beta) \cdot {\rm e}^{\hspace{0.03cm}p\hspace{0.05cm} \cdot \hspace{0.05cm}t} \Bigg |_{\hspace{0.1cm} p\hspace{0.05cm}=\hspace{0.05cm}{\rm j \hspace{0.05cm} \cdot\hspace{0.05cm} \it \beta}}+X_{\rm L}(p) \cdot (p + {\rm j} \cdot \beta) \cdot {\rm e}^{\hspace{0.03cm}p \hspace{0.05cm} \cdot\hspace{0.05cm}t} \Bigg |_{\hspace{0.1cm} p\hspace{0.05cm}=\hspace{0.05cm}{-\rm j \hspace{0.05cm} \cdot\hspace{0.05cm} \it \beta}} \hspace{0.05cm}.$

Questions

Solution

(1) Richtig sind die Lösungsvorschläge 1, 3 und 4:

Durch Anwendung des Residuensatzes erhält man für das Signal $x(t)$ bei positiven Zeiten:

$x_1(t)\hspace{0.25cm} = \hspace{0.2cm} {\rm Res} \bigg |_{p \hspace{0.05cm}= \hspace{0.05cm}p_{{\rm x}1}} \hspace{0.7cm}\{X_{\rm L}(p)\cdot {\rm e}^{\hspace{0.05cm}p \hspace{0.05cm}\cdot \hspace{0.05cm}t}\}= \frac {p} { p+{\rm j} \cdot 2\pi}\cdot {\rm e}^{\hspace{0.05cm}p \hspace{0.05cm}\cdot \hspace{0.05cm}t} \bigg |_{p \hspace{0.05cm}= \hspace{0.05cm}{\rm j} \hspace{0.05cm}\cdot \hspace{0.05cm}2\pi}= \frac{1}{2} \cdot {\rm e}^{\hspace{0.05cm}{\rm j} \hspace{0.05cm} \cdot \hspace{0.05cm}2\pi t}\hspace{0.05cm} ,$

$x_2(t)\hspace{0.25cm} = \hspace{0.2cm} {\rm Res} \bigg |_{p \hspace{0.05cm}= \hspace{0.05cm}p_{{\rm x}2}} \hspace{0.7cm}\{X_{\rm L}(p)\cdot {\rm e}^{\hspace{0.05cm}p \hspace{0.05cm}\cdot \hspace{0.05cm} t}\}= \frac {p} { p-{\rm j} \cdot 2\pi}\cdot {\rm e}^{\hspace{0.05cm}p \hspace{0.05cm}\cdot \hspace{0.05cm}t} \bigg |_{p \hspace{0.05cm}= -{\rm j} \hspace{0.05cm}\cdot \hspace{0.05cm}2\pi}= \frac{1}{2} \cdot {\rm e}^{-{\rm j} \hspace{0.05cm} \cdot \hspace{0.05cm}2\pi t} \hspace{0.05cm} .$

$\Rightarrow \hspace{0.3cm} x(t) = x_1(t) + x_2(t) = {1}/{2} \cdot \left [ {\rm e}^{\hspace{0.05cm}{\rm j} \hspace{0.05cm} \cdot \hspace{0.05cm}2\pi t}+{\rm e}^{-{\rm j} \hspace{0.05cm} \cdot \hspace{0.05cm}2\pi t}\right ] = \cos(2\pi t) \hspace{0.05cm} .$

(2) Richtig sind die Lösungsvorschläge 2 und 4:

Prinzipiell könnte diese Teilaufgabe in gleicher Weise gelöst werden wie die Teilaufgabe (1).
Man kann aber auch den Integrationssatz heranziehen.
Dieser besagt unter anderem, dass die Multiplikation mit $1/p$ im Spektralbereich der Integration im Zeitbereich entspricht:

$Y_{\rm L}(p) = {1}/{p} \cdot X_{\rm L}(p) \hspace{0.3cm} \Rightarrow \hspace{0.3cm} t \ge 0:\quad y(t) = \int_{-\infty}^t \cos(2\pi \tau)\,\,{\rm d}\tau = {1}/({2\pi}) \cdot \sin(2\pi t) \hspace{0.05cm} .$

Hinweis: Das kausale Cosinussignal $x(t)$ sowie das kausale Sinussignal $y(t)$ sind auf dem Angabenblatt zu Aufgabe 3.6 als $c_{\rm K}(t)$ bzw. $s_{\rm K}(t)$ dargestellt.

(3) Richtig sind die Lösungsvorschläge 1 und 3:

Ein Vergleich mit der Berechnung von $x(t)$ zeigt, dass $z(t) = \cos (\beta \cdot t)$ für $t \ge 0$ und $z(t) = 0$ für $t < 0$ gilt.
Der Grenzübergang für $\beta → 0$ führt damit zur Sprungfunktion $\gamma(t)$ .
Zum gleichen Ergebnis kommt man durch die Betrachtung im Spektralbereich:

$Z_{\rm L}(p) = \lim_{\beta \hspace{0.05cm} \rightarrow \hspace{0.05cm} 0}\hspace{0.1cm}\frac{p}{p^2 + \beta^2} = {1}/{p} \hspace{0.3cm} \Rightarrow \hspace{0.3cm} z(t) = \gamma(t) \hspace{0.05cm} .$

	$x(t)$ ist ein kausales Cosinussignal.
	$x(t)$ ist ein kausales Sinussignal.
	Die Amplitude von $x(t)$ ist $1$ .
	Die Periodendauer von $x(t)$ ist $T = 1 \ \rm µ s$ .

	$y(t)$ ist ein kausales Cosinussignal.
	$y(t)$ ist ein kausales Sinussignal.
	Die Amplitude von $y(t)$ ist $1$ .
	Die Periodendauer von $y(t)$ ist $T = 1 \ \rm µ s$ .

	Für $\beta > 0$ verläuft $z(t)$ cosinusförmig.
	Für $\beta > 0$ verläuft $z(t)$ sinusförmig.
	Der Grenzfall $\beta → 0$ führt zur Sprungfunktion $\gamma(t)$ .