Exercise 3.11Z: Extremely Asymmetrical Channel
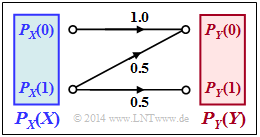
Betrachtet wird der nebenstehend gezeichnete Kanal mit den folgenden Eigenschaften:
- Das Symbol $X = 0$ wird immer richtig übertragen und führt stets zum Ergebnis $Y = 0$.
- Das Symbol $X = 1$ wird maximal verfälscht.
Aus Sicht der Informationstheorie bedeutet dies:
- $${\rm Pr}(Y \hspace{-0.05cm} = 0\hspace{-0.05cm}\mid \hspace{-0.05cm} X \hspace{-0.05cm}= 1) ={\rm Pr}(Y \hspace{-0.05cm} = 1\hspace{-0.05cm}\mid \hspace{-0.05cm} X \hspace{-0.05cm}= 1) = 0.5 \hspace{0.05cm}.$$
Zu bestimmen sind in dieser Aufgabe:
- die Transinformation $I(X; Y)$ für $P_X(0) = p_0 = 0.4$ und $P_X(1) = p_1 = 0.6$.
Es gilt allgemein:
- $$ I(X;Y) = H(X) - H(X \hspace{-0.1cm}\mid \hspace{-0.1cm} Y)\hspace{0.05cm}=H(Y) - H(Y \hspace{-0.1cm}\mid \hspace{-0.1cm} X)\hspace{0.05cm} =\hspace{-0.15cm} H(X) + H(Y)- H(XY)\hspace{0.05cm},$$
- die Kanalkapazität:
- $$ C = \max_{P_X(X)} \hspace{0.15cm} I(X;Y) \hspace{0.05cm}.$$
Hinweise:
- Die Aufgabe gehört zum Kapitel Anwendung auf die Digitalsignalübertragung.
- Bezug genommen wird insbesondere auf die Seite Kanalkapazität eines Binärkanals.
- In der Aufgabe 3.14 sollen die hier gefundenen Ergebnisse im Vergleich zum BSC–Kanal interpretiert werden.
Fragebogen
Musterlösung
- $$H(X) = H_{\rm bin}(p_0)= H_{\rm bin}(0.4) \hspace{0.15cm} \underline {=0.971\,{\rm bit}} \hspace{0.05cm}.$$
(2) Die Wahrscheinlichkeiten der Sinkensymbole sind:
- $$P_Y(1) = p_1/2 = (1 - p_0)/2 = 0.3\hspace{0.05cm},\hspace{0.2cm} P_Y(0) = 1-P_Y(1) = p_1/2 = (1 - p_0)/2 = 0.7$$
- $$\Rightarrow \hspace{0.3cm} H(Y) = H_{\rm bin}(\frac{1+p_0}{2})= H_{\rm bin}(0.7) \hspace{0.15cm} \underline {=0.881\,{\rm bit}} \hspace{0.05cm}.$$
(3) Die Verbundwahrscheinlichkeiten $p_{μκ} = {\rm Pr}\big[(X = μ) ∩ (Y = κ)\big] $ ergeben sich zu:
- $$ p_{00} = p_0 \hspace{0.05cm},\hspace{0.3cm} p_{01} = 0 \hspace{0.05cm},\hspace{0.3cm} p_{10} = (1 - p_0)/2 \hspace{0.05cm},\hspace{0.3cm} p_{11} = (1 - p_0)/2$$
- $$\Rightarrow \hspace{0.3cm} H(XY) =p_0 \cdot {\rm log}_2 \hspace{0.1cm} \frac{1}{ p_0} + 2 \cdot \frac{1-p_0}{2} \cdot {\rm log}_2 \hspace{0.1cm} \frac{2}{ 1- p_0} = p_0 \cdot {\rm log}_2 \hspace{0.1cm} \frac{1}{ p_0} + (1-p_0) \cdot {\rm log}_2 \hspace{0.1cm} \frac{1}{ 1- p_0} + (1-p_0) \cdot {\rm log}_2 \hspace{0.1cm} (2)$$
- $$\Rightarrow \hspace{0.3cm}H(XY) =H_{\rm bin}(p_0) + 1 - p_0 \hspace{0.05cm}.$$
Das numerische Ergebnis für $p_0 = 0.4$ lautet somit:
- $$H(XY) = H_{\rm bin}(0.4) + 0.6 = 0.971 + 0.6 \hspace{0.15cm} \underline {=1.571\,{\rm bit}} \hspace{0.05cm}.$$
(4) Eine (mögliche) Gleichung zur Berechnung der Transinformation lautet:
- $$ I(X;Y) = H(X) + H(Y)- H(XY)\hspace{0.05cm}$$
Daraus erhält man mit den Ergebnissen der ersten drei Teilaufgaben:
- $$I(X;Y) = H_{\rm bin}(p_0) + H_{\rm bin}(\frac{1+p_0}{2}) - H_{\rm bin}(p_0) -1 + p_0 = H_{\rm bin}(\frac{1+p_0}{2}) -1 + p_0.$$
- $$ \Rightarrow \hspace{0.3cm} p_0 = 0.4 {\rm :}\hspace{0.5cm} I(X;Y) = H_{\rm bin}(0.7) - 0.6 = 0.881 - 0.6 \hspace{0.15cm} \underline {=0.281\,{\rm bit}}\hspace{0.05cm}.$$
(5) Die Kanalkapazität $C$ ist die Transinformation $I(X; Y)$ bei bestmöglichen Wahrscheinlichkeiten $p_0$ und $p_1$ der Quellensymbole.
- Nach Differentiation erhält man die Bestimmungsgleichung:
- $$\frac{\rm d}{{\rm d}p_0} \hspace{0.1cm} I(X;Y) = \frac{\rm d}{{\rm d}p_0} \hspace{0.1cm} H_{\rm bin}(\frac{1+p_0}{2}) +1 \stackrel{!}{=} 0 \hspace{0.05cm}.$$
- Mit dem Differentialquotienten der binären Entropiefunktion
- $$ \frac{\rm d}{{\rm d}p} \hspace{0.1cm} H_{\rm bin}(p) = {\rm log}_2 \hspace{0.1cm} \frac{1-p}{ p} \hspace{0.05cm},$$
und entsprechendes Nachdifferenzieren erhält man:
- $${1}/{2} \cdot {\rm log}_2 \hspace{0.1cm} \frac{(1-p_0)/2}{1- (1-p_0)/2} +1 \stackrel{!}{=} 0 \hspace{0.3cm} \Rightarrow \hspace{0.3cm} {1}/{2} \cdot {\rm log}_2 \hspace{0.1cm} \frac{(1-p_0)/2}{(1+p_0)/2} +1 \stackrel{!}{=} 0$$
- $$ \Rightarrow \hspace{0.3cm} {\rm log}_2 \hspace{0.1cm} \frac{1+p_0}{1-p_0} \stackrel{!}{=} 2 \hspace{0.3cm} \Rightarrow \hspace{0.3cm} \frac{1+p_0}{1-p_0} \stackrel{!}{=} 4 \hspace{0.3cm}\Rightarrow \hspace{0.3cm} p_0 \hspace{0.15cm} \underline {=0.6}\hspace{0.05cm}.$$
(6) Für die Kanalkapazität gilt dementsprechend:
- $$C = I(X;Y) \big |_{p_0 \hspace{0.05cm}=\hspace{0.05cm} 0.6} = H_{\rm bin}(0.8) - 0.4 = 0.722 -0.4 \hspace{0.15cm} \underline {=0.322\,{\rm bit}}\hspace{0.05cm}.$$
In der Aufgabe A3.14 wird dieses Ergebnis im Vergleich zum BSC–Kanalmodell interpretiert.
(7) Für die Äquivokation gilt:
- $$ H(X \hspace{-0.1cm}\mid \hspace{-0.1cm}Y) = H(X) - I(X;Y) = 0.971 -0.322 \hspace{0.15cm} \underline {=0.649\,{\rm bit}}\hspace{0.05cm}.$$
- Wegen $H_{\rm bin}(0.4) = H_{\rm bin}(0.6)$ ergibt sich die gleiche Quellenentropie $H(X)$ wie in Teilaufgabe (1).
- Die Sinkenentropie muss neu berechnet werden.
- Mit $p_0 = 0.6$ erhält man $H(Y) = H_{\rm bin}(0.8) = 0.722\ \rm bit$, und damit ergibt sich für die Irrelevanz:
- $$H(Y \hspace{-0.1cm}\mid \hspace{-0.1cm} X) = H(Y) - I(X;Y) = 0.722 -0.322 \hspace{0.15cm} \underline {=0.400\,{\rm bit}}\hspace{0.05cm}.$$