Exercise 1.1: Music Signals

• Return to book

Music signals, original and
noisy and/or distorted?

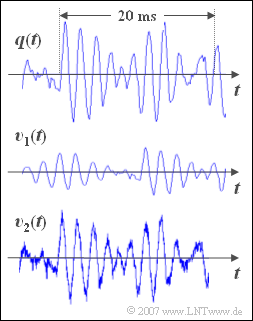
On the right you see a ca.  $\text{30 ms}$  long section of a music signal  \(q(t)\). It is the piece „For Elise” by Ludwig van Beethoven.

• Underneath are drawn two sink signals  \(v_1(t)\)  and  \(v_2(t)\), which were recorded after the transmission of the music signal  \(q(t)\)  over two different channels.

• The following controls allow you to listen to the first fourteen seconds of each of the three audio signals  \(q(t)\),  \(v_1(t)\)  and  \(v_2(t)\).

Originalsignal  \(q(t)\)

Sinkensignal  \(v_1(t)\)

Sinkensignal  \(v_2(t)\)

Notes:

• The task belongs to chapter  Prinzip der Nachrichtenübertragung.

Questions

1

Estimate the signal frequency of  \(q(t)\)  in the displayed section.

	The signal frequency is approximately  \(f = 250\,\text{Hz}\).
	The signal frequency is approximately  \(f = 500\,\text{Hz}\).
	The signal frequency is about  \(f = 1\,\text{kHz}\).

2

Which statements are true for the signal  \(v_1(t)\) ?

	The signal  \(v_1(t)\)  is undistorted compared to \(q(t)\).
	The signal  \(v_1(t)\)  shows distortions compared to  \(q(t)\) .
	The signal  \(v_1(t)\)  is noisy compared to  \(q(t)\) .

3

Which statements are true for the signal  \(v_2(t)\) ?

	The signal  \(v_2(t)\)  is undistorted compared to  \(q(t)\) .
	The signal  \(v_2(t)\)  shows distortions compared to  \(q(t)\) .
	The signal  \(v_2(t)\)  is noisy compared to  \(q(t)\) .

4

One of the signals is opposite the original  \(q(t)\)  undistorted and not noisy.
Estimate the attenuation factor and the running time for this.

\( \alpha \ = \ \)

\( \tau \ = \ \) $\ \text{ms}$

Solutions

Solution

(1)  Correct is the solution 2:

• In the marked range of $20$ milliseconds approx.   $10$  oscillations can be detected.
• From this the result  follows approximately for the signal frequency; $f = {10}/(20 \,\text{ms}) = 500 \,\text{Hz}$.

(2)  Correct is the solution 1:

• The signal  \(v_1(t)\)  is undistorted compared to the original signal \(q(t)\). The following applies:   $v_1(t)=\alpha \cdot q(t-\tau) .$

• Eine Dämpfung  \(\alpha\)  und eine Laufzeit  \(\tau\)  führen nicht zu Verzerrungen, sondern das Signal ist dann nur leiser und es kommt später als das Original.

(3)  Richtig sind die Lösungsvorschläge 1 und 3:

• Man erkennt sowohl im dargestellten Signalverlauf  \(v_2(t)\)  als auch im Audiosignal  additives Rauschen   ⇒   Lösungsvorschlag 3.
• Der Signalrauschabstand beträgt dabei ca.  $\text{30 dB}$; dies ist aber aus dieser Darstellung nicht erkennbar.
• Richtig ist aber auch der Lösungsvorschlag 1:   Ohne diesen Rauschanteil wäre  \(v_2(t)\)  identisch mit  \(q(t)\).

(4)  Das Signal  \(v_1(t)\)  ist formgleich mit dem Originalsignal  \(q(t)\)  und unterscheidet sich von diesem lediglich

• durch den Amplitudenfaktor  $\alpha = \underline{\text{0.3}}$  (dies entspricht etwa  $\text{–10 dB)}$
• und die Laufzeit  $\tau = \underline{10\,\text{ms}}$.