Exercise 2.6: Two-Way Channel
From LNTwww
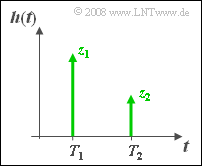
The so-called "two-way channel" is characterised by the following impulse response $($with $T_1 < T_2)$:
- $$h(t) = z_1 \cdot \delta ( t - T_1) + z_2 \cdot \delta ( t - T_2).$$
- Except for a few combinations of the system parameters $z_1$, $T_1$, $z_2$ and $T_2$, this channel will result in linear distortions.
- There is a distortion-free channel at hand only if not a single input signal is distorted by it.
- This means: Even if the two-way channel is per se distorting, there may be special cases where indeed $y(t) = \alpha \cdot x(t - \tau)$.
The test signals applied to the system input are:
- a Dirac comb $x_1(t)$ at a time interval of $T_0 = 1 \ \rm ms$, whose spectral function $X_1(f)$ is also a Dirac comb with an interval of $f_0 = 1/T_0 = 1 \ \rm kHz$:
- $$x_1(t) = \sum_{n = - \infty}^{+\infty} \delta ( t - n \cdot T_0) ,\hspace{0.5cm} X_1(f) = T_0 \cdot \sum_{k = - \infty}^{+\infty} \delta ( f - k \cdot f_0) ,$$
- a cosine signal with frequency $f_2 = 250 \ \rm Hz$:
- $$x_2(t) = \cos(2 \pi \cdot f_2 \cdot t) ,$$
- the sum of two cosine signals with frequencies $f_2 = 250 \ \rm Hz$ and $f_3 = 1250 \ \rm Hz$:
- $$x_3(t) = \cos(2 \pi \cdot f_2 \cdot t) + \cos(2 \pi \cdot f_3 \cdot t) .$$
Please note:
- The task belongs to the chapter Linear Distortions.
- To spare you calculations the result for the parameter set $\big [z_1 = 1$, $T_1 = 0$, $z_2 =0.5$, $T_2 = 1 \ \rm ms\big ]$ is given:
- $$|H(f = f_2)| = |H(f = f_3)| = \sqrt{1.25} \approx 1.118, \; \; \; \; b(f = f_2) = b(f = f_3) = \arctan (0.5) \approx 0.464.$$
Questions
Solution
(1) Statements 1 and 2 are correct:
- $h(t) = \delta(t)$ is true with $z_1 = 1$, $T_1 = 0$ and $z_2 =0$ and correspondingly $H(f) = 1$ so that $y(t) = x(t)$ will always hold.
- Each distortion-free channel impulse response $h(t)$ consists of a single Dirac function, for example at $t = T_1$.
- This case is accounted for in the model by $z_2 =0$ . Thus, the frequency response is:
- $$H(f)= z_1\cdot {\rm e}^{-{\rm j}\cdot \hspace{0.05cm}2 \pi f T_1} \ \Rightarrow \ y(t) = z_1 \cdot x(t- T_1).$$
- In contrast, the channel will lead to linear distortions whenever $z_1$ and $z_2$ are simultaneously non-zero.
(2) The Fourier transformation of the impulse response $h(t)$ results in the equation:
- $$H(f) = z_1\cdot {\rm e}^{-{\rm j}\hspace{0.05cm} \cdot \hspace{0.05cm}2 \pi f T_1}+ z_2\cdot {\rm e}^{-{\rm j}\hspace{0.05cm} \cdot \hspace{0.05cm}2 \pi f T_2} .$$
- The following is obtained with $z_1 = 1$, $T_1 = 0$, $z_2 =0.5$ and $T_2 = 1 \ \rm ms$ :
- $$H(f) =1 + 0.5 \cdot {\rm e}^{-{\rm j}\hspace{0.05cm} \cdot \hspace{0.05cm}2 \pi f T_2}.$$
- Broken down by real and imaginary part, this yields:
- $${\rm Re}\big[H(f)\big] = 1 + 0.5 \cdot \cos(2 \pi f \cdot 1\,{\rm ms}) \ \Rightarrow \ \underline{{\rm Re}[H(f = f_1 =1 \ \rm kHz)] = 1.5}, $$
- $${\rm Im}\big[H(f)\big] = -0.5 \cdot \sin(2 \pi f \cdot 1\,{\rm ms}) \ \Rightarrow \ \underline{{\rm Im}\big[H(f = f_1 =1 \ \rm kHz)\big] = 0}, $$
(3) The first answer is the only correct one:
- From (2) it follows that the absolute value function is $|H(f)| = 1.5$ and the phase function $b(f) \equiv 0$ for all multiples of $f_1 =1 \ \rm kHz$ .
- Thus, the phase delay time is also zero in each case for these discrete frequency values.
- But since the spectrum $X_1(f)$ of the Dirac comb has spectral lines exactly at these frequencies, $y_1(t) = 1.5 \cdot x_1(t)$ holds.
(4) The absolute value function is:
- $$|H(f)| = \sqrt{{\rm Re}[H(f)]^2 + {\rm Im}[H(f)]^2} $$
- $$\Rightarrow \; |H(f)| = \sqrt{1 + 0.25 \cdot \cos^2(2 \pi f \cdot T_2)+ \cos(2 \pi f \cdot T_2) + 0.25 \cdot \sin^2(2 \pi f \cdot T_2)} = \sqrt{1.25 + \cos(2 \pi f \cdot T_2) }.$$
- Thus, the following is obtained for the frequency $f_2 =0.25 \ \rm kHz$ :
- $$|H(f)| = \sqrt{1.25 + \cos(\frac{\pi}{2} ) }= \sqrt{1.25} = 1.118.$$
- The phase function is generally or at the frequency $f_2 =0.25 \ \rm kHz$:
- $$b(f) = - {\rm arctan}\hspace{0.1cm}\frac{{\rm Im}[H(f)]}{{\rm Re}[H(f)]} = - {\rm arctan}\hspace{0.1cm}\frac{-0.5 \cdot \sin(2 \pi f T_2)}{1+0.5 \cdot \cos(2 \pi f T_2)},$$
- $$b(f = f_2) = - {\rm arctan}\hspace{0.1cm}\frac{-0.5 \cdot \sin( \pi/2)}{1+0.5 \cdot \cos(\pi/2)}={\rm arctan}\hspace{0.1cm}\frac{0.5}{1} = 0.464.$$
- Thus, the phase delay time for this frequency is:
- $$\tau_2 = \frac {b(f_2)}{2 \pi f_2} = \frac {0.464}{2 \pi \cdot 0.25\,{\rm kHz}} \approx 0.3\,{\rm ms},$$
- Hence, the following holds for the output signal:
- $$y_2(t) = 1.118 \cdot \cos(2 \pi \cdot 0.25\,{\rm kHz}\cdot (t - 0.3\,{\rm ms})).$$
- The signal value at zero-time is therefore:
- $$y_2(t=0) = 1.118 \cdot \cos(-2 \pi \cdot 0.25\,{\rm kHz} \cdot 0.3\,{\rm ms}) \approx 1.118 \cdot 0.891 \hspace{0.15cm}\underline{= 0.996}.$$
(5) Both frequencies have the same attenuation factor $\alpha = 1.118$ . Therefore, no attenuation distortions are observed.
- The following is obtained for the phase function with $f_3 = 1.25 \ \rm kHz$ and $T_2 = 1 \ \rm ms$ :
- $$b(f = f_3) = - {\rm arctan}\hspace{0.1cm}\frac{-0.5 \cdot \sin( 2.5 \pi)}{1+0.5 \cdot \cos(2.5 \pi)}= 0.464 = b(f = f_2),$$
- so exactly the same value as for the frequency $f_2 = 0.25 \ \rm kHz$.
- Despite this, however, phase distortions now occur since for $f_3$ the phase delay time is only $\tau = 60 \ µ \rm s$ anymore.
- So, the following can be formulated for the output signal:
- $$y_3(t) = 1.118 \cdot \cos(2 \pi f_2 \cdot (t - 0.3\,{\rm ms}) + 1.118 \cdot \cos(2 \pi f_3 \cdot (t - 0.06\,{\rm ms})$$
- $$\Rightarrow \; \; y_3(t) = 1.118 \cdot \cos(2 \pi f_2 \cdot t - 27^\circ) + 1.118 \cdot \cos(2 \pi f_3 \cdot t - 27^\circ).$$
Accordingly, the correct answer is 3:
- So, there are phase distortions although for both oscillations, $\varphi_2 = \varphi_3= 27^\circ$ holds.
- To avoid phase distortions
- the phase delay times $\tau_2$ and $\tau_3$ would have to be equal and
- the phase values $\varphi_2$ and $\varphi_3$ would have to increase linearly with the corresponding frequencies.