Loading [MathJax]/jax/output/HTML-CSS/fonts/TeX/fontdata.js

Exercise 4.6: k-parameters and alpha-parameters

From LNTwww
Revision as of 17:05, 29 October 2021 by Guenter (talk | contribs)

Dämpfungsmaß  (0.5 mm  Doppelader) mit  k– und  α-Parameter

Für symmetrische Kupfer–Doppeladern findet man in  [PW95]  die folgende empirische Formel, gültig für den Frequenzbereich  0≤f≤30 MHz:

αI(f)=k1+k2⋅(f/f0)k3,f0=1MHz.

Dagegen ist das Dämpfungsmaß eines Koaxialkabels meist in der folgenden Form angegeben:

αII(f)=α0+α1⋅f+α2⋅√f.

Insbesondere zur Berechnung von Impulsantwort und Rechteckantwort ist es von Vorteil, auch für die Kupfer–Doppeladern die zweite Darstellungsform mit den Kabelparametern  α0,  α1  und  α2  anstelle der Beschreibung durch  k1,  k2  und  k3 zu wählen.

Für die Umrechnung geht man dabei wie folgt vor:

  • Aus obigen Gleichungen ist offensichtlich, dass der die Gleichsignaldämpfung charakterisierende Koeffizient  α0=k1  ist.
  • Zur Bestimmung von  α1  und  α2  wird davon ausgegangen, dass der mittlere quadratische Fehler im Bereich einer vorgegebenen Bandbreite  B  minimal sein soll:
E[ε2(f)]=∫B0[αII(f)−αI(f)]2df⇒Minimum.
  • Die Differenz  ε2(f)  und der mittlere quadratische Fehler  E[ε2(f)]  ergeben sich dabei wie folgt:
ε2(f)=[α1⋅f+α2⋅√f−k2⋅(f/f0)k3]2=α21⋅f2+2α1α2⋅f1.5+α21⋅f+k22⋅f2k3f2k30−2k2α1⋅fk3+1fk30−2k2α2⋅fk3+0.5fk30
⇒E[ε2(f)]=α21⋅B33+45⋅α1α2⋅B2.5+α21⋅B22+k222k3+1⋅B2k3+1f2k30−2k2α1k3+2⋅
Diese Gleichung beinhaltet die zu verrechnenden Kabelparameter  α1,  α2,  k2  und  k3  sowie die Bandbreite  B,  innerhalb derer die Approximation gültig sein soll.
  • Durch Nullsetzen der Ableitungen von  E[ε2(f)]  nach  α1  bzw.  α2  erhält man zwei Gleichungen für die bestmöglichen Koeffizienten  α1  und  α2, die den mittleren quadratischen Fehler minimieren. Diese lassen sich in folgender Form darstellen:
dE[ε2(f)]dα1=0⇒α1+C1⋅α2+C2=0,
dE[ε2(f)]dα2=0⇒α1+D1⋅α2+D2=0.
  • Aus der Gleichung  C1⋅α2+C2=D1⋅α2+D2  lässt sich daraus der Koeffizient  α2  berechnen und anschließend aus jeder der beiden oberen Gleichungen der Koeffizient  α1.


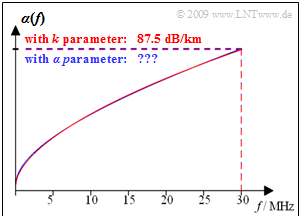
Die Grafik zeigt das Dämpfungsmaß für eine Kupferdoppelader mit  0.5 mm  Durchmesser, deren  k–Parameter lauten:

k1=4.4dB/km,k2=10.8dB/km,k3=0.60.
  • Die rote Kurve zeigt die damit berechnete Funktion  α(f).  Für  f=30 MHz  ergibt sich das Dämpfungsmaß  α(f)=87.5 dB/km.
  • Die blaue Kurve gibt die Approximation mit den  α–Koeffizienten an.  Diese ist von der roten Kurve innerhalb der Zeichengenauigkeit fast nicht zu unterscheiden.





Hinweise:

  • Sie können zur Überprüfung Ihrer Ergebnisse das interaktive Applet  Dämpfung von Kupferkabeln  benutzen.
  • [PW95]  kennzeichnet folgenden Literaturhinweis:   Pollakowski, P.; Wellhausen, H.-W.: Eigenschaften symmetrischer Ortsanschlusskabel im Frequenzbereich bis 30 MHz. Deutsche Telekom AG, Forschungs- und Technologiezentrum Darmstadt, 1995.


Fragebogen

1

Berechnen Sie die Parameter  C1  und  C2  der Gleichung  α1+C1⋅α2+C2=0, die sich aus der Ableitung  dE[...]/dα1  ergeben.
Welche Ergebnisse sind zutreffend?

C1=6/5⋅B−0.5,
C1=5/4⋅B−0.5,
C1=4/3⋅B2,
C2=−4/3⋅B−2$,
C2=−5/2⋅k2/(k3+1.5)⋅Bk3−1⋅f−k30,
C2=−3⋅k2/(k3+2)⋅Bk3−1⋅f−k30.

2

Berechnen Sie die Parameter  D1  und  D2  der Gleichung  α1+D1⋅α2+D2=0, die sich aus der Ableitung  dE[...]/dα2  ergeben.
Welche Ergebnisse sind zutreffend?

D1=6/5⋅B−0.5,
D1=5/4⋅B−0.5,
D1=4/3⋅B2,
D2=−4/3⋅B−2,
D2=−5/2⋅k2/(k3+1.5)⋅Bk3−1⋅f−k30,
D2=−3⋅k2/(k3+2)⋅Bk3−1⋅f−k30.

3

Berechnen Sie die Koeffizienten  α1  und  α2  für die vorgegebenen  k2  und  k3.
Welche der folgenden Aussagen sind zutreffend?

Für  k3=1.0  gilt  α1=k2/f0  und  α2=0.
Für  k3=0.5  gilt  α1=0  und  α2=k2/f0.50.

4

Ermitteln Sie die Koeffizienten  α1  und  α2  zahlenmäßig für die Approximationsbandbreite  B=30 MHz.

α1 = 

 dB/(km ⋅ MHz)
α2 = 

 dB/(km ⋅ √MHz)

5

Berechnen Sie mit den  α–Parametern das Dämpfungsmaß für die Frequenz  f=30 MHz.

αII(f=30 MHz) = 

 dB/km


Musterlösung

Solution

(1)  Richtig sind die Lösungsvorschläge 1 und 6:

  • Die Ableitung des angegebenen Erwartungswertes nach  α1  ergibt:
dE[ε2(f)]dα1=23⋅B3⋅α1+45⋅B2.5⋅α2−2k2k3+2⋅Bk3+2fk30=0.
  • Durch Nullsetzen und Division durch  2B2/3  erhält man daraus:
α1+65⋅B−0.5⋅α2−3k2k3+2⋅Bk3−1fk30=0⇒C1=65⋅B−0.5,C2=−3k2k3+2⋅Bk3−1fk30.


(2)  Richtig sind die Lösungsvorschläge 2 und 5:

  • Bei gleicher Vorgehensweise wie in der Teilaufgabe  (1)  erhält man:
dE[ε2(f)]dα2=45⋅B2.5⋅α1+B2⋅α2−2k2k3+1.5⋅Bk3+1.5fk30=0
⇒α1+54⋅B−0.5⋅α2−2.5⋅k2k3+1.5⋅Bk3−1fk30=0⇒D1=54⋅B−0.5,D2=−2.5⋅k2k3+1.5⋅Bk3−1fk30.


(3)  Aus  C1⋅α2+C2=D1⋅α2+D2  ergibt sich eine lineare Gleichung für  α2. Mit dem Ergebnis aus  (2)  kann hierfür geschrieben werden:

α2=D2−C2C1−D1=−2.5⋅k2k3+1.5⋅Bk3−1fk30+3k2k3+2⋅Bk3−1fk306/5⋅B−0.5−5/4⋅B−0.5=−2.5⋅k2⋅(k3+2)+3k2⋅(k3+1.5)(6/5−5/4)(k3+1.5)(k3+2)⋅Bk3−0.5fk30
⇒α2=10⋅(B/f0)k3−0.5⋅1−k3(k3+1.5)(k3+2)⋅k2√f0.
  • Für den Parameter  α1  gilt dann:
α1=−C1⋅α2−C2=−65⋅B−0.5⋅10⋅(B/f0)k3−0.5⋅1−k3(k3+1.5)(k3+2)⋅k2√f0+3k2k3+2⋅Bk3−1fk30
⇒α1=(B/f0)k3−1⋅−12⋅(1−k3)+3⋅(k3+1.5)(k3+1.5)(k3+2)⋅k2f0⇒α1=15⋅(B/f0)k3−1⋅k3−0.5(k3+1.5)(k3+2)⋅k2f0.

Beide Lösungsvorschläge sind richtig.

  • Unabhängig von der Bandbreite erhält man für  k3=1:
α1=(B/f0)k3−1⋅15⋅(k3−0.5)(k3+1.5)(k3+2)⋅k2f0=15⋅0.52.5⋅3⋅k2f0=k2/f0_,
α2=(B/f0)k3−0.5⋅10⋅(1−k3)(k3+1.5)(k3+2)⋅k2√f0=0_.
  • Dagegen ergibt sich für  k3=0.5:
α1=(B/f0)k3−1⋅15⋅(k3−0.5)(k3+1.5)(k3+2)⋅k2f0=0_,
α2=(B/f0)k3−0.5⋅10⋅(1−k3)(k3+1.5)(k3+2)⋅k2√f0=10⋅0.52⋅2.5⋅k2√f0=k2/√f0_.


(4)  Für die beiden Koeffizienten gilt mit  k2=10.8 dB/km,  k3=0.6 dB/km  und  B/f0=30:

α1=(B/f0)k3−1⋅15⋅(k3−0.5)(k3+1.5)(k3+2)⋅k2f0=30−0.4⋅15⋅0.12.1⋅2.6⋅10.8dB/km1MHz≈0.761dB/(km⋅MHz)_,
α2=(B/f0)k3−0.5⋅10⋅(1−k3)(k3+1.5)(k3+2)⋅k2√f0=k2√f0=300.1⋅10⋅0.42.1⋅2.6⋅10.8dB/km1MHz0.5≈11.1dB/(km⋅√MHz)_.


(5)  Entsprechend der angegebenen Gleichung  αII(f)  gilt damit auch:

αII(f=30MHz)=α0+α1⋅f+α2⋅√f=[4.4+0.761⋅30+11.1⋅√30]dBkm≈88.1dB/km_.

Retrieved from "http://en.lntwww.de/index.php?title=Aufgaben:Exercise_4.6:_k-parameters_and_alpha-parameters&oldid=42619"
  • Powered by MediaWiki