Exercise 4.14: ACF and CCF for Square Wave Signals

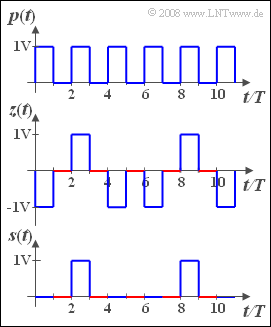
ACF and CCF for rectangular signals

We consider a periodic square wave signal $p(t)$ corresponding to the top sketch with the two possible amplitude values $0 \hspace{0.05cm} \rm V$ and $1 \hspace{0.05cm} \rm V$ and the rectangular duration $T$. Thus, the period duration is $T_0 = 2T$.

Below this is drawn the random signal $z(t)$ .

This is between $(2i-1)\cdot T$ and $2i \cdot T$ respectively $z(t)=0 \hspace{0.05cm} \rm V$ (highlighted in red in the figure).
In the intervals drawn in blue between $2i \cdot T$ and $(2i+1) \cdot T$ the signal value is two-point distributed $\pm 1 \hspace{0.05cm} \rm V$.

The probability that in the intervals shown in blue $z(t)=+1 \hspace{0.05cm} \rm V$ holds is generally equal $p$ and independent of the previously selected values.

The lowest signal in the adjacent graph can be constructed from the first two. It holds:

$$s(t) = {1}/{2} \cdot \big[p(t) + z(t)\big].$$

In the time intervals drawn in red between $(2i-1) \cdot T$ and $2i \cdot T$ $(i$ integer$)$ holds $s(t)=0 \hspace{0.05cm} \rm V$, since here both $p(t)$ and $z(t)$ are equal to zero.
In the intervening intervals, the amplitude value is two-point distributed between $0 \hspace{0.05cm} \rm V$ and $1 \hspace{0.05cm} \rm V$, where the value $1 \hspace{0.05cm} \rm V$ occurs again with probability $p$ .
Or in other words, The signals $z(t)$ and $s(t)$ are equivalent pattern signals of the identical random process with bipolar $(-1 \hspace{0.05cm} \rm V, \ +1 \hspace{0.05cm} \rm V)$ bzw. unipolarer $(0 \hspace{0.05cm} \rm V, \ 1 \hspace{0.05cm} \rm V)$ signal representation, respectively.

Hints:

Die Aufgabe gehört zum Kapitel Kreuzkorrelationsfunktion und Kreuzleistungsdichte.
Bezug genommen wird auch auf das Kapitel Autokorrelationsfunktion.

Skizzieren Sie die gesuchten Korrelationsfunktionen jeweils im Bereich von $-7T$ bis $+7T$.

Fragebogen

$\varphi_z(\tau= 0) \ = \ $

$\ \rm V^2$

$\varphi_z(\tau= 3T) \ = \ $

$\ \rm V^2$

$\varphi_z(\tau= 6T) \ = \ $

$\ \rm V^2$

$\varphi_p(\tau= 0) \ = \ $

$\ \rm V^2$

$\varphi_p(\tau= 3T) \ = \ $

$\ \rm V^2$

$\varphi_p(\tau= 6T) \ = \ $

$\ \rm V^2$

$\varphi_{pz}(\tau= 0) \ = \ $

$\ \rm V^2$

$\varphi_{pz}(\tau= 3T) \ = \ $

$\ \rm V^2$

$\varphi_{pz}(\tau= 6T) \ = \ $

$\ \rm V^2$

	$\varphi_c(\tau) = \varphi_a(\tau) + \varphi_b(\tau)$.
	$\varphi_c(\tau) = \varphi_a(\tau) + \varphi_{ab}(\tau) + \varphi_{ba}(\tau) + \varphi_b(\tau)$.
	$\varphi_c(\tau) = \varphi_a(\tau) \star \varphi_b(\tau)$.

$\varphi_s(\tau= 0) \ = \ $

$\ \rm V^2$

$\varphi_s(\tau= 3T) \ = \ $

$\ \rm V^2$

$\varphi_s(\tau= 6T) \ = \ $

$\ \rm V^2$

Musterlösung

Solution

(1) Der AKF-Wert bei $\tau = 0$ gibt die mittlere Leistung an:

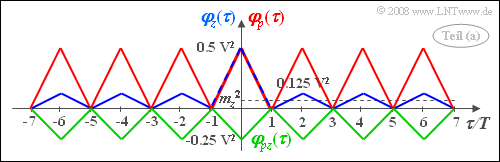
$$\varphi_z ( \tau = 0) = {1}/{2} \cdot (1 {\rm V})^2 \hspace{0.15cm}\underline{= 0.5 {\rm V}^2}.$$

Für $\tau = \pm T$, $\underline{\tau = \pm 3T}$, ... ergibt sich $\varphi_z ( \tau)\hspace{0.15cm}\underline{ = 0}$.

Für die Zwischenwerte $\tau = \pm 2T$, $\tau = \pm 4T$, $\underline{\tau = \pm 6T}$, ... gilt:

$$\varphi_z ( \tau) = \frac {1 {\rm V}^2}{2} \left(p \hspace{0.02cm} \cdot \hspace{0.02cm}p \hspace{0.2cm} + \hspace{0.2cm}p \hspace{0.02cm}\cdot \hspace{0.02cm}(p-1) \hspace{0.2cm}+\hspace{0.2cm} (p-1)\hspace{0.02cm} \cdot \hspace{0.02cm}p \hspace{0.2cm}+\hspace{0.2cm} (p-1)\hspace{0.02cm} \cdot \hspace{0.02cm}(p-1)\right) = \hspace{0.1cm}\text{...} \hspace{0.1cm}= 0.5\, {\rm V}^2 \cdot (1-2p)^2 .$$

Autokorrelationsfunktionen und Kreuzkorrelationsfunktion

Hierbei steht $p$ für $p \cdot (+1)$ und $(p-1)$ für $(1-p) \cdot (-1)$, also jeweils Wahrscheinlichkeit mal normierter Amplitudenwert.
Mit $p = 0.25$ erhält man $\varphi_z ( \tau = \pm 6 T) \hspace{0.15cm}\underline{=0.125 \rm V^2}$.

Die blaue Kurve zeigt $\varphi_z(\tau)$ für $p = 0.25$ im Bereich von $-7T \le \tau \le +7T$:

Aufgrund des rechteckförmigen Signalverlaufs ergibt sich eine Summe von Dreieckfunktionen.
Für $p = 0.5$ würden die äußeren (kleineren) Dreiecke verschwinden.

(2) Die AKF $\varphi_p(\tau)$ des unipolaren periodischen Signals $p(t)$ ist in der allgemeingültigen Darstellung von (1) ⇒ AKF $\varphi_z(\tau)$ als Sonderfall für $p = 1$ enthalten.

Man erhält nun eine periodische AKF (siehe roter Kurvenverlauf in obiger Skizze) mit

$$\varphi_p ( \tau = 0) = \varphi_p ( \tau = \pm 2 T) = \varphi_p ( \tau = \pm 4 T) = \hspace{0.1cm} \text{...} \hspace{0.1cm}\hspace{0.15cm}\underline{= 0.5 {\rm V}^2},$$

$$\varphi_p ( \tau = \pm T) = \varphi_p ( \tau = \pm 3T) = \hspace{0.1cm} ... \hspace{0.1cm}\hspace{0.15cm}\underline{= 0}.$$

(3) Auch für die Kreuzkorrelationsfunktion ergibt sich für $\tau = \pm T$, $\underline{\tau = \pm 3T}$, ... stets der Wert Null.

Dagegen sind die KKF-Werte für $\tau = \pm 2T$, $\tau = \pm 2T$, ... identisch mit denen bei $\tau = 0$:

$$\varphi_{pz} ( \tau = 0) = \varphi_{pz} ( \tau = \pm 2 T) = \varphi_{pz} ( \tau = \pm 4 T) = \hspace{0.1cm} \text{...} \hspace{0.1cm}= \frac {1 {\rm V}^2}{2} \left( p - (1-p)\right) = \frac {2p -1}{2}\, {\rm V}^2 .$$

Man erhält mit $p = 0.25$ folgende Ergebnisse (siehe grüne Kurve in obiger Skizze):

$$\varphi_{pz} ( \tau = 0)\hspace{0.15cm}\underline{= -0.25 {\rm V}^2},\hspace{0.5cm} \varphi_{pz} ( \tau = 3T)\hspace{0.15cm}\underline{= 0},\hspace{0.5cm} \varphi_{pz} ( \tau = 6T)\hspace{0.15cm}\underline{= -0.25 {\rm V}^2}.$$

Mit $p = 1$ würde dagegen $z(t) \equiv p(t)$ gelten und damit natürlich auch $\varphi_{pz}(\tau) \equiv \varphi_{p}(\tau) \equiv \varphi_{z}(\tau)$.
Für den Sonderfall $p = 0.5$ ergäbe sich keine Korrelation zwischen $p(t)$ und $z(t)$ und damit $\varphi_{pz}(\tau) \equiv 0$.

(4) Durch Einsetzen von $c(t) = a(t) + b(t)$ in die allgemeine AKF-Definition erhält man:

$$\varphi_c ( \tau ) = \overline{c(t)\hspace{0.02cm} \cdot \hspace{0.02cm} c(t + \tau)} = \overline{a(t)\hspace{0.02cm} \cdot \hspace{0.02cm} a(t + \tau)} \hspace{0.1cm}+\hspace{0.1cm}\overline{a(t)\hspace{0.02cm} \cdot \hspace{0.02cm} b(t + \tau)} +\overline{b(t)\hspace{0.02cm} \cdot \hspace{0.02cm} a(t + \tau)} \hspace{0.1cm}+\hspace{0.1cm}\overline{b(t)\hspace{0.02cm} \cdot \hspace{0.02cm} b(t + \tau)}. $$

$$\Rightarrow \hspace{0.5cm} \varphi_c ( \tau ) = \varphi_{a} ( \tau ) \hspace{0.1cm} + \hspace{0.1cm} \varphi_{ab} ( \tau ) \hspace{0.1cm} + \hspace{0.1cm} \varphi_{ba} ( \tau ) \hspace{0.1cm} + \hspace{0.1cm}\varphi_{a} ( \tau ). $$

Richtig ist somit der Lösungsvorschlag 2.
Der Lösungsvorschlag 1 trifft nur zu, wenn $a(t)$ und $b(t)$ unkorreliert sind.
Der letzte Vorschlag, die Faltungsoperation, ist immer falsch.
Eine ähnliche Gleichung würde sich nur dann ergeben, wenn wir die WDF $f_c(c)$ der Summe $c(t) = a(t) + b(t)$ betrachten und $a(t)$ und $b(t)$ statistisch unabhängig sind: $f_c (c) = f_a (a) \star f_b (b) .$

(5) Mit dem Ergebnis aus (4) und unter Berücksichtigung des Faktors $1/2$ erhält man:

$$\varphi_s ( \tau ) = {1}/{4} \cdot \big[ \varphi_{p} ( \tau ) \hspace{0.1cm} + \hspace{0.1cm} \varphi_{z} ( \tau ) \hspace{0.1cm} + \hspace{0.1cm} 2 \cdot \varphi_{pz} ( \tau ) \big] . $$

Hierbei ist bereits berücksichtigt, dass die KKF zwischen $p(t)$ und $z(t)$ eine gerade Funktion ist, so dass auch $\varphi_{pz}(\tau) = \varphi_{zp}(\tau)$ gilt.
Für $\tau = 0$ erhält man deshalb mit den obigen Ergebnissen allgemein:

$$\varphi_s( \tau = 0) = {1}/{4} \cdot \left( 0.5 {\rm V}^2 +0.5 {\rm V}^2 + 2 \cdot \frac{2p-1}{2} {\rm V}^2\right) .$$

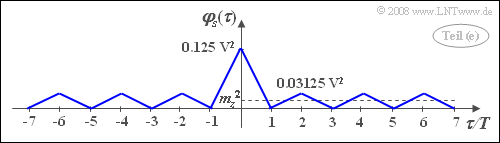
Mit $p = 0.25$ ergibt sich $\varphi_{pz} ( \tau = 0 ) = 0.125\rm V^2$. Dieses Ergebnis ist plausibel. Im Mittel ist nur in jedem achten Intervall $s(t)=1 \hspace{0.05cm} \rm V$; ansonsten ist $s(t)=0 \hspace{0.05cm} \rm V$.

Für geradzahlige Vielfache von $T$ gilt:

$$ \varphi_s ( \tau = \pm 2 T) = \varphi_s ( \tau = \pm 4 T) = \hspace{0.1cm} \text{ ...} \hspace{0.1cm} = \frac {0.5 {\rm V}^2}{4} \left( (1-2p)^2 +1 + 2 \cdot (2p -1)\right) = 0.5 \, {\rm V}^2 \hspace{0.02cm} \cdot \hspace{0.02cm} p^2.$$

Mit $p = 0.5$ erhält man hierfür den Wert $0.03125 \hspace{0.1cm}{\rm V}^2$. Alle AKF-Werte bei ungeradzahligen Vielfachen von $T$ sind wieder Null.
Damit ergibt sich der skizzierte AKF–Verlauf.

AKF eines unipolaren Rechtecksignals

Die gesuchten Zahlenwerte sind somit:

$$\varphi_{s} ( \tau = 0)\hspace{0.15cm}\underline{= 0.125 {\rm V}^2},$$

$$\varphi_{s} ( \tau = 3T)\hspace{0.15cm}\underline{= 0},$$

$$\varphi_{s} ( \tau = 6T)\hspace{0.15cm}\underline{= -0.03125 {\rm V}^2}.$$

Ein Vergleich mit der Skizze zur Teilaufgabe (1) zeigt, dass das binäre Signal $s(t)$ bis auf den Faktor $1/4$ die gleiche AKF aufweist wie das Ternärsignal $z(t)$.