Exercise 3.2Z: Bessel Spectrum

Progression of Bessel functions

Consider the complex signal

$$x(t) = {\rm e}^{\hspace{0.05cm}{\rm j} \hspace{0.05cm}\cdot \hspace{0.05cm}\eta \hspace{0.05cm}\cdot \hspace{0.05cm}\sin (\omega_{\rm 0} \hspace{0.05cm}\cdot \hspace{0.05cm} t) }\hspace{0.05cm}.$$

For example, the equivalent low-pass signal at the output of an angle modulator (PM, FM) can be represented in this form if appropriate normalizations are made.

When $T_0 = 2π/ω_0$, the Fourier series representation is:

$$x(t) = \sum_{n = - \infty}^{+\infty}D_n \cdot{\rm e}^{\hspace{0.05cm}{\rm j} \hspace{0.05cm}\cdot \hspace{0.05cm}n \hspace{0.05cm}\cdot \hspace{0.05cm}\omega_{\rm 0} \hspace{0.05cm}\cdot \hspace{0.05cm} t }\hspace{0.05cm},$$

$$ D_n = \frac{1}{T_0}\cdot \int_{- T_0/2}^{+T_0/2}x(t) \cdot{\rm e}^{\hspace{0.05cm}{-\rm j} \hspace{0.05cm}\cdot \hspace{0.05cm}n \hspace{0.05cm}\cdot \hspace{0.05cm}\omega_{\rm 0} \hspace{0.05cm} \cdot \hspace{0.05cm} t }\hspace{0.1cm}{\rm d}t \hspace{0.05cm}.$$

These complex Fourier coefficients can be expressed using $n$–th order Bessel functions of the first kind:

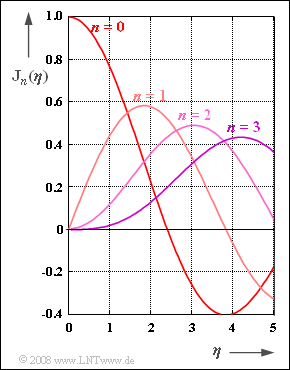
$${\rm J}_n (\eta) = \frac{1}{2\pi}\cdot \int_{-\pi}^{+\pi} {{\rm e}^{\hspace{0.05cm}{\rm j}\hspace{0.05cm}\cdot \hspace{0.05cm}(\eta \hspace{0.05cm}\cdot \hspace{0.05cm}\sin(\alpha) -\hspace{0.05cm} n \hspace{0.05cm}\cdot \hspace{0.05cm}\alpha)}}\hspace{0.1cm}{\rm d}\alpha \hspace{0.05cm}.$$

These are shown on the graph in the range $0 ≤ η ≤ 5$ . For negative values of $n$ one obtains:

$${\rm J}_{-n} (\eta) = (-1)^n \cdot {\rm J}_{n} (\eta)\hspace{0.05cm}.$$

The series representation of the Bessel functions is:

$${\rm J}_n (\eta) = \sum\limits_{k=0}^{\infty}\frac{(-1)^k \cdot (\eta/2)^{n \hspace{0.05cm} + \hspace{0.05cm} 2 \hspace{0.02cm}\cdot \hspace{0.05cm}k}}{k! \cdot (n+k)!} \hspace{0.05cm}.$$

If the function values for $n = 0$ and $n = 1$ are known, the Bessel functions for $n ≥ 2$ can be determined from them by iteration:

$${\rm J}_n (\eta) = \frac{2 \cdot (n-1)}{\eta} \cdot {\rm J}_{n-1} (\eta) - {\rm J}_{n-2} (\eta) \hspace{0.05cm}.$$

Hints:

This exercise belongs to the chapter Phase Modulation.
Particular reference is made to the page Equivalent low-pass signal in phase modulation.
The values of the Bessel functions can be found in collections of formulae in table form.
You can also use the interactive applet Bessel functions of the first kind to solve this task.

Questions

	$x(t)$ is imaginary for all times $t$ .
	$x(t)$ is periodic.
	The spectral function $X(f)$ is obtained via the Fourier integral.

	All $D_n$ are equal to ${\rm J}_η(0)$.
	$D_n = {\rm J}_n(η)$ holds.
	$D_n = -{\rm J}_η(n)$ holds.

	All $D_n$ are purely real.
	Alle $D_n$ are purely imaginary.

$D_2 \ = \ $

$D_3 \ = \ $

$D_{-2} \ = \ $

$D_{-3} \ = \ $

Musterlösung

Solution

(1) Richtig ist nur der zweite Lösungsvorschlag:

$x(t)$ ist ein komplexes Signal, das nur in Ausnahmefällen reell wird, zum Beispiel zur Zeit $t = 0$.
Ein rein imaginärer Wert (zu gewissen Zeiten) kann sich nur dann ergeben, wenn $η ≥ π/2$ ist ⇒ Antwort 1 ist falsch.
Mit $T_0 = 2π/ω_0$ gilt beispielsweise:

$$ x(t + k \cdot T_0) = {\rm e}^{\hspace{0.05cm}{\rm j} \hspace{0.05cm}\cdot \hspace{0.05cm}\eta \hspace{0.05cm}\cdot \hspace{0.05cm}\sin (\omega_{\rm 0} \hspace{0.05cm}\cdot \hspace{0.05cm} (t \hspace{0.05cm}+ \hspace{0.05cm} k \hspace{0.05cm}\cdot \hspace{0.05cm}T_0)) } = {\rm e}^{\hspace{0.05cm}{\rm j} \hspace{0.05cm}\cdot \hspace{0.05cm}\eta \hspace{0.05cm}\cdot \hspace{0.05cm}\sin (\omega_{\rm 0} \hspace{0.05cm}\cdot \hspace{0.05cm} t \hspace{0.05cm} + \hspace{0.05cm} k \hspace{0.05cm}\cdot \hspace{0.05cm} 2 \pi) } ={\rm e}^{\hspace{0.05cm}{\rm j} \hspace{0.05cm}\cdot \hspace{0.05cm}\eta \hspace{0.05cm}\cdot \hspace{0.05cm}\sin (\omega_{\rm 0} \hspace{0.05cm}\cdot \hspace{0.05cm} t \hspace{0.05cm} ) } = x(t)\hspace{0.05cm}.$$

Dieses Signal ist periodisch. Zur Berechnung der Spektralfunktion muss die Fourierreihe und nicht das Fourierintegral herangezogen werden.

(2) Die Fourierkoeffizienten lauten:

$$ D_n = \frac{1}{T_0}\cdot \int_{- T_0/2}^{+T_0/2}{\rm e}^{\hspace{0.05cm}{\rm j} \hspace{0.05cm}\cdot \hspace{0.05cm}\eta \hspace{0.05cm}\cdot \hspace{0.05cm}\sin (\omega_{\rm 0} \hspace{0.05cm} \cdot \hspace{0.05cm} t) }\cdot{\rm e}^{\hspace{0.05cm}{-\rm j} \hspace{0.05cm}\cdot \hspace{0.05cm}n \hspace{0.05cm}\cdot \hspace{0.05cm}\omega_{\rm 0} \hspace{0.05cm} \cdot \hspace{0.05cm} t }\hspace{0.1cm}{\rm d}t \hspace{0.05cm}.$$

Durch Zusammenfassen der beiden Terme und nach der Substitution $α = ω_0 · t$ erhält man:

$$D_n = \frac{1}{2\pi}\cdot \int_{-\pi}^{+\pi} {{\rm e}^{\hspace{0.05cm}{\rm j}\hspace{0.05cm}\cdot \hspace{0.05cm}(\eta \hspace{0.05cm}\cdot \hspace{0.05cm}\sin(\alpha) -\hspace{0.05cm} n \hspace{0.05cm}\cdot \hspace{0.05cm}\alpha)}}\hspace{0.1cm}{\rm d}\alpha \hspace{0.05cm} = {\rm J}_n (\eta) .$$

Richtig ist also der zweite Lösungsvorschlag.

(3) Mit dem Satz von Euler können die Fourierkoeffizienten wie folgt dargestellt werden:

$$D_n = \frac{1}{2\pi}\cdot \int_{-\pi}^{+\pi} {\cos( \eta \cdot \sin(\alpha) - n \cdot \alpha)}\hspace{0.1cm}{\rm d}\alpha + \frac{\rm j}{2\pi}\cdot \int_{-\pi}^{+\pi} {\sin( \eta \cdot \sin(\alpha) - n \cdot \alpha)}\hspace{0.1cm}{\rm d}\alpha \hspace{0.05cm}.$$

Der Integrand des ersten Integrals ist eine gerade Funktion von $\alpha$:

$$I_1 (-\alpha) = {\cos( \eta \cdot \sin(-\alpha) + n \cdot \alpha)} = {\cos( -\eta \cdot \sin(\alpha) + n \cdot \alpha)}= {\cos( \eta \cdot \sin(\alpha) - n \cdot \alpha)} = I_1 (\alpha) \hspace{0.05cm}.$$

Dagegen ist der zweite Integrand eine ungerade Funktion:

$$I_2 (-\alpha) = {\sin( \eta \cdot \sin(-\alpha) + n \cdot \alpha)} = {\sin( -\eta \cdot \sin(\alpha) + n \cdot \alpha)}= -{\sin( \eta \cdot \sin(\alpha) - n \cdot \alpha)} = -I_2 (\alpha) \hspace{0.05cm}.$$

Somit verschwindet das zweite Integral und man erhält unter Berücksichtigung der Symmetrie:

$$D_n = \frac{1}{\pi}\cdot \int_{0}^{\pi} {\cos( \eta \cdot \sin(\alpha) - n \cdot \alpha)}\hspace{0.1cm}{\rm d}\alpha \hspace{0.05cm}.$$

Richtig ist somit der Lösungsvorschlag 1.

(4) Entsprechend der iterativen Berechnungsformel gilt für $η = 2$:

$$ D_2 = D_1 - D_0 = 0.577 - 0.224 \hspace{0.15cm}\underline {= 0.353} \hspace{0.05cm},$$

$$D_3 = 2 \cdot D_2 - D_1 = 2 \cdot 0.353 - 0.577 \hspace{0.15cm}\underline {= 0.129} \hspace{0.05cm}.$$

(5) Aufgrund der angegebenen Symmetriebeziehung gilt weiter:

$$ D_{–2} = D_2\hspace{0.15cm}\underline {= 0.353} \hspace{0.05cm},$$

$$D_{–3} = -D_3 \hspace{0.15cm}\underline {= -0.129} \hspace{0.05cm}.$$