Exercise 3.5: PM and FM for Rectangular Signals

• Return to book

Zwei Signalverläufe bei Winkelmodulation

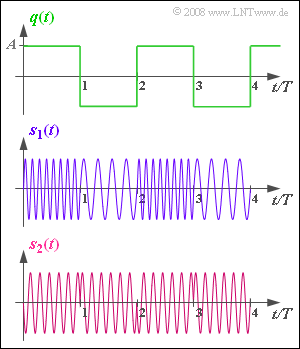
Assume a bipolar and rectangular source signal $q(t)$ , as shown in the upper diagram.  This signal can only take on the two signal values  $±A = ±2 \ \rm V$  and the duration of the positive and negative rectangles are each $T = 1 \ \rm ms$.  The period of  $q(t)$  is therefore  $T_0 = 2 \ \rm ms$.

The signals $s_1(t)$  and  $s_2(t)$  display two transmit signals with angle modulation  $\rm (WM)$, each of which can be represented as

$$s(t) = A_{\rm T} \cdot \cos \hspace{-0.05cm}\big [\psi (t) \big ]$$

Here, we distinguish between phase modulation  $\rm (PM)$  with the angular function

$$\psi(t) = \omega_{\rm T} \cdot t + \phi(t) = \omega_{\rm T} \cdot t + K_{\rm PM} \cdot q(t)$$

and frequency modulation  $\rm (FM)$, where the instantaneous freqiency is linearly related to $q(t)$:

$$f_{\rm A}(t) = \frac{\omega_{\rm A}(t)}{2\pi}, \hspace{0.3cm} \omega_{\rm A}(t) = \frac{{\rm d}\hspace{0.03cm}\psi(t)}{{\rm d}t}= \omega_{\rm T} + K_{\rm FM} \cdot q(t)\hspace{0.05cm}.$$

$K_{\rm PM}$  and  $K_{\rm FM}$  denote the dimensionally constrained constants given by the realizations of the PM and FM modulators, respectively.  The frequency deviation  $Δf_{\rm A}$  indicates the maximum deviation of the instantaneous frequency from the carrier frequency.

Hints:

• This exercise belongs to the chapter  Frequency Modulation.
• Reference is also made to the chapter  Phase Modulation.

• In anticipation of the fourth chapter, it should be mentioned that phase modulation with a digital input signal is also called Phase Shift Keying  $\rm (PSK)$  and frequency modulation is analogously called Frequency Shift Keying  $\rm (FSK)$ .

Questions

1

Which of the signals is due to phase modulation and which is due to frquency modulation?

	$s_1(t)$  represents a phase modulation.
	$s_1(t)$  represents a frequency modulation.

2

What is the carrier phase  $ϕ_{\rm T}$ that could be measured without a message signal   ⇒   $q(t) \equiv 0$ ?

$ϕ_{\rm T} \ = \ $ $\ \rm Grad$

3

What carrier frequency  $($with respect to  $1/T)$  was used in the graphs?

$f_{\rm T} · T \ = \ $

4

The phase of the PM signal is  $±90^\circ$.  What is the modulator constant?

$K_{\rm PM} \ = \ $ $\ \rm V^{-1}$

5

What is the frequency deviation  $Δf_{\rm A}$  of the FM signal with respect to  $1/T$?

$Δf_{\rm A} · T \ = \ $

6

What is the FM modulator constant?

$K_{\rm FM} \ = \ $ $\ \rm (Vs)^{-1}$

Solution

Solution

(1)  Answer 2 is correct:

• For a rectangular (digital) source signal, phase modulation (PM) can be recognised by the typical phase jumps – see the signal waveform  $s_2(t)$.
• Frequency modulation (FM), on the other hand, has diverse instantaneous frequencies at different times, as in  $s_1(t)$.

(2)  When  $q(t) = 0$ , the equations provided for both PM and FM give

$$s(t) = A_{\rm T} \cdot \cos (\omega_{\rm T} \cdot t ) \hspace{0.3cm}\Rightarrow\hspace{0.3cm} \phi_{\rm T} \hspace{0.15cm}\underline {= 0}\hspace{0.05cm}.$$

(3)  The carrier frequency  $f_{\rm T}$  can be directly determined only from the PM signal   $s_2(t)$ .

• By counting the oscillations of  $s_2(t)$  in the time interval  $T$ , it can be seen that  $f_{\rm T} · T\hspace{0.15cm}\underline{ = 6}$  was used.
• When frequency modulating a bipolar source signal,   $f_{\rm T}$  does not occur directly.
• However, the graphs do indicate that   $f_{\rm T} · T = 6$  is also used here.

(4)  The amplitude value  $A = 2 \ \rm V$  results in the phase  $90^\circ$  or  $π/2$  (minus sine wave).  This gives:

$$K_{\rm PM} = \frac {\pi /2}{2\,{\rm V}} \hspace{0.15cm}\underline {= 0.785\,{\rm V}^{-1}} \hspace{0.05cm}.$$

(5)  The graph for  $s_1(t)$  shows that either four or eight oscillations arise within a time interval  $T$ :   $4 \le f_{\rm A}(t) \cdot T \le 8\hspace{0.05cm}.$

• Considering the (normalized) carrier frequency  $f_{\rm T} · T = 6$ , the (normalized) frequency deviation is:

$$\Delta f_{\rm A} \cdot T \hspace{0.15cm}\underline {=2}\hspace{0.05cm}.$$

(6)  The frequency deviation can also be represented as follows:

$$\Delta f_{\rm A} = \frac {K_{\rm FM}}{2\pi}\cdot A \hspace{0.05cm}.$$

• With   $Δf_{\rm A} · {\rm A} = 2$  we thus get:

$$K_{\rm FM} = \frac {2 \cdot 2\pi}{A \cdot T}= \frac {4\pi}{2\,{\rm V} \cdot 1\,{\rm ms}}\hspace{0.15cm}\underline {= 6283 \,{\rm V}^{-1}{\rm s}^{-1}} \hspace{0.05cm}.$$