Exercise 1.17Z: BPSK Channel Capacity
Binary signals can be transmitted error-free over the "AWGN channel" according to the "channel coding theorem" if and only if
- one uses a channel code of rate $R = k/n$,
- the block length $n$ of this code is chosen very large ⇒ $n → ∞$,
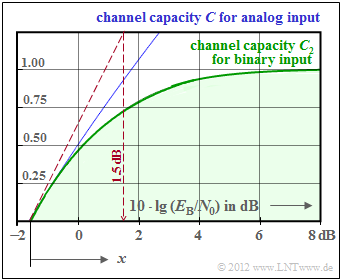
- the rate $R$ is smaller than the channel capacity valid for binary input $C_{2}$,
- where the BPSK channel capacity $C_{2}$ depends on the AWGN quotient $E_{\rm B}/N_{0}$ .
Note:
- The permissible range for the code rate $R$ is highlighted in green in the graph.
- The limit curve $C_{2}$, valid for binary input signals $($therefore the index $2)$ and sometimes also referred to as BPSK channel capacity (stands for "Binary Phase Shift Keying").
- However, this curve cannot be specified in mathematically closed form, but is the result of an integral that can only be evaluated numerically.
- As blue curve the channel capacity $C$ is entered, if arbitrary real input signals are allowed.
- For multilevel signals the rate may well assume values $R > 1$.
- For a Gaussian distribution the rate $R$ is the smallest possible $(E_{\rm B}/N_{0})_{\rm min}$ according to the equation
- $$\left (E_{\rm B}/N_0 \right)_{\rm min} = \frac{2^{2R}-1}{2R}\hspace{0.05cm}.$$
Conversely, the rate $R$ for the given AWGN quotient $E_{\rm B}/N_{0}$ is upper bounded:
- The just allowable code rate $R_{\rm max}$ for a given channel $(E_{\rm B}/N_{0} = \rm const.)$ we refer to as the channel capacity $C$.
- For $E_{\rm B}/N_{0} = 1 ⇒ 10 \ \lg {E_{\rm B}/N_0} = 0\ {\rm dB}$ we obtain, for example $C = 0.5$.
- This means: Even with the best possible amplitude distribution of the real input signal, the code rate must not exceed $R = 0.5$.
- For binary input, a slightly smaller value results according to $C_{2}.$
In this exercise, try to approximate the graphically given channel capacitance $C_{2}$ by an exponential function:
- For the abscissa, use the auxiliary variable (see graph)
- $$x = \frac {x_0 + 10 \cdot {\rm lg} \hspace{0.1cm} E_{\rm B}/N_0 }{1\,{\rm dB}}\hspace{0.05cm}.$$
- That is, $x$ is without unit; the pseudo unit "$\rm dB$" is omitted.
- Consider that for a small $E_{\rm B}/N_{0}$ the approximation $C_{2} \approx C$ is valid (see graph), from which the parameter $x_{0}$ can be determined.
- Set for $C_{2}\hspace{0.01cm}' = 1 - {\rm e}^{-a\hspace{0.05cm} · \hspace{0.05cm}x}$ and determine the parameter $a$ from the red dashed tangent such that $C_{2}\hspace{0.01cm} ' \approx C$ holds.
Hints:
- The exercise belongs to the chapter "Information Theoretical Limits of Channel Coding". It complements the "Exercise 1.17".
- The "bit/channel use" pseudo-unit of channel capacity is omitted in these exercises.
Questions
Solution
(1) In the lower $E_{\rm B} /N_{0}$ range, the capacity curves
- $C_{2}$ (valid for binary input, for example BPSK) and
- $C$ (valid for analog real-valued input).
converge. For a given rate $R$ $E_{\rm B}/N_{0}$ must be greater than $(2^{2R} - 1)/2R.$
The limit transition for $R → 0$ provides the absolute Shannon limit above which error-free transmission is no longer possible:
- $${\rm Min}\hspace{0.1cm}\left [E_{\rm B}/N_0 \right] = \lim_{R \rightarrow 0}\hspace{0.1cm} \frac{2^{2R}-1}{2R} = {\rm ln}\hspace{0.1cm}2 \approx 0.693\hspace{0.3cm}\Rightarrow \hspace{0.3cm} 10 \cdot {\rm lg} \hspace{0.1cm} {\rm Min}\hspace{0.1cm}\left [E_{\rm B}/N_0 \right] \approx -1.6 \,{\rm dB} \hspace{0.3cm} \Rightarrow \hspace{0.3cm} x_0 \hspace{0.15cm} \underline{= 1.6 \,{\rm dB}}\hspace{0.05cm}.$$
(2) The tangent increase at the zero point can be estimated from the graph on the data page:
- $$\frac{{\rm d}C_2}{{\rm d}x} (x=0) = \frac{1.6 + 1.5}{1.25} =2.48 \hspace{0.3cm} \Rightarrow \hspace{0.3cm} a = \frac{1}{2.48} \hspace{0.15cm} \underline{\approx 0.4}\hspace{0.05cm}.$$
Thus, the approximation for the BPSK channel capacity as a function of the abscissa value $x$ is:
- $$C_2' = \hspace{0.15cm} \left\{ \begin{array}{c} 1 - {\rm e}^{- 0.4 \hspace{0.05cm}\cdot \hspace{0.05cm}x} \\ 0 \end{array} \right.\quad \begin{array}{*{1}c} {\rm for\hspace{0.15cm}} x > 0, \\{\rm for\hspace{0.15cm}} x < 0. \end{array}$$
(3) From $E_{\rm B} = N_{0}$ follows $\ 10 · \lg {(E_{\rm B} = N_0)} = 0 \ {\rm dB}$ and $x = 1.6$:
- $$C_2\hspace{0.01cm}' = 1 - {\rm e}^{- 0.4 \hspace{0.05cm}\cdot \hspace{0.05cm}1.6}\hspace{0.15cm}\underline{\approx 0.47}\hspace{0.05cm}.$$
(4) The corresponding numerical values are:
- $$10 \cdot {\rm lg} \hspace{0.1cm} E_{\rm B}/N_0 = \text{2 dB:} \hspace{0.3cm} C_2\hspace{0.01cm}' \hspace{-0.15cm}\ = \ \hspace{-0.15cm} 1 - {\rm e}^{- 0.4 \hspace{0.05cm}\cdot \hspace{0.05cm}3.6}\hspace{0.15cm}\underline{\approx 0.76}$$
- $$10 \cdot {\rm lg} \hspace{0.1cm} E_{\rm B}/N_0 = \text{4 dB:} \hspace{0.3cm} C_2\hspace{0.01cm}' \hspace{-0.15cm}\ = \ \hspace{-0.15cm} 1 - {\rm e}^{- 0.4 \hspace{0.05cm}\cdot \hspace{0.05cm}5.6}\hspace{0.15cm}\underline{\approx 0.89}$$
- $$10 \cdot {\rm lg} \hspace{0.1cm} E_{\rm B}/N_0 = \text{6 dB:} \hspace{0.3cm} C_2\hspace{0.01cm}' \hspace{-0.15cm}\ = \ \hspace{-0.15cm} 1 - {\rm e}^{- 0.4 \hspace{0.05cm}\cdot \hspace{0.05cm}7.6}\hspace{0.15cm}\underline{\approx 0.95}.$$
The values $C_{2}\hspace{0.01cm}'$ of the channel capacity for binary input approximated in this way are somewhat too small.
From the graph on the information page the exact values $C_{2}$ can be estimated:
- $$10 \cdot {\rm lg} \hspace{0.1cm} E_{\rm B}/N_0 =\text{2 dB:} \hspace{0.3cm} C_2 \hspace{-0.15cm}\ \approx \ \hspace{-0.15cm} {0.78}\hspace{0.05cm},$$
- $$10 \cdot {\rm lg} \hspace{0.1cm} E_{\rm B}/N_0 = \text{4 dB:} \hspace{0.3cm} C_2 \hspace{-0.15cm}\ \approx \ \hspace{-0.15cm} {0.94}\hspace{0.05cm},$$
- $$10 \cdot {\rm lg} \hspace{0.1cm} E_{\rm B}/N_0 = \text{6 dB:} \hspace{0.3cm} C_2 \hspace{-0.15cm}\ \approx \ \hspace{-0.15cm} {0.99}\hspace{0.05cm}.$$
From about $\ 10 \cdot \lg {(E_{\rm B} / N_0)} = 8 \ {\rm dB}$ applies within character accuracy: $C_{2}\hspace{0.01cm}'= C_{2} = 1$ $($bit/channel use$)$.