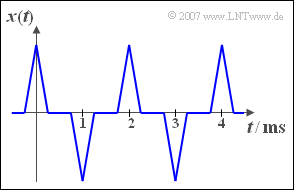
The graph shows the periodic signal x(t). If x(t) is applied to the input of a non-linearity with the characteristic curve
- y=g(x)={xforx≥0,0else,
the signal y(t) is obtained at the output. A second non-linear characteristic
- z=h(x)=|x|
delivers the signal z(t).
Note:
- This exercise belongs to the chapter General description of periodic signals.
Questions
Solution
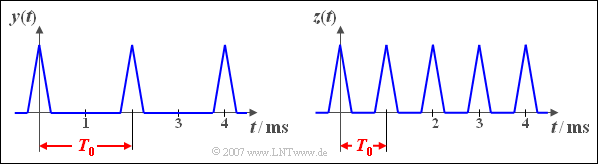
(1) Correct are the solutions 1 and 4:
- The non-linear characteristic y=g(x) describes a half-wave rectifier.
- z=h(x)=|x| describes a full-wave rectifier.
(2) The period duration x(t) is T0=2ms. The inverse magnitudes to the base frequency f0=500Hz_.
(3) The half-wave rectification does not change the duration of the period, see the left graph: T0=2ms_.
(4) After full-wave rectification, the signal z(t) has double the frequency (see right graph). The following values apply here:
- T0=1ms,f0=1kHz,ω0=62831/s_.