Exercise 3.6Z: Examination Correction
In an exam at the TU Munich 1000 students participated. From the grade "4.0" upwards up to "1.0" the exam is considered to be passed. The exam regulations provide for the following grades:
- 1.0, 1.3, 1.7, 2.0, 2.3, 2.7, 3.0, 3.3, 3.7, 4.0, 4.3, 4.7, 5.0.
Further, the exercise must take into account:
- The maximum achievable score is 100. The best student achieved 88 points.
- Due to the relatively large number of participants, the achieved score – this is the random variable z – has with good approximation a Gaussian distribution with mean mz=60 and standard deviation σz=10.
- In the correction,&nbs
p; not only whole scores were assigned, but also (arbitrary) intermediate values, so that the random variable z can be taken as "continuous valued" with good approximation.
For scoring, the guidelines given are:
- Even with six points less than the best (so from 82 points) one shall get "1.0".
- If one reaches 46% of the total score, one has passed the exam.
- The points/grades assignment shall be linear.
Hints:
- The exercise belongs to the chapter Gaussian distributed random variables.
- The "Gaussian distribution" is often called "normal distribution". This is not quite correct:
- A normally distributed random variable z does have a Gaussian PDF and CDF, but always with mean mz=0 and standard deviation σz=1.
Questions
Solution
- According to the central limit theorem, a Gaussian distribution is obtained for the sum of many independent quantities.
- Conversely, if there are only a few dependent tasks, there is no Gaussian distribution.
- A single yes/no question leads to a two-point distribution (0 points or maximum number of points).
- Even if these imperatives are followed, a Gaussian distribution will not be expected for very few participants.
(2) One gets a "1.0" with 82 points or more.
- Therefore, with the mean mz=60 and the standard deviation σz=10:
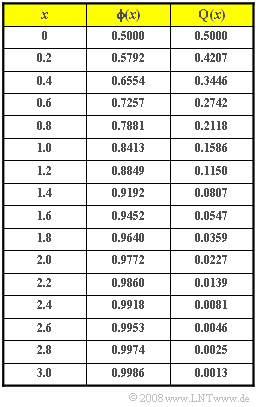
- Pr(z≥82)=Q(82−6010)=Q(2.2)=0.0139.
- For a thousand participants, it follows N1.0=14_.
(3) With less than 46 points, one has failed the exam:
- Pr(z<46)=Pr(z≤46)=ϕ(46−6010)=ϕ(−1.4)=Q(1.4)=0.0807.
- So 81 students have to compete again.
(4) The difference in points 82−46=36 must be divided among nine grade intervals (1.3, ... , 4.0).
- Each interval thus comprises 4 points.
- For example, one receives a grade of "3.0" if one has 58 to 62 points.
- The probability that the grade is in this range is given by
- Pr(58<z<62)=ϕ(62−6010)−ϕ(58−6010).
- Taking advantage of the symmetry, one obtains:
- Pr(58<z<62)=ϕ(0.2)−ϕ(−0.2)=0.5792−0.4207=0.1587(159participants)_.
Notes:
- z is to be taken as a continuous valued random variable.
- Therefore, the score 62 is simultaneously the upper bound for the grade "3.0" as well as the lower bound for the grade "2.7."
- If z were only an integer, then 62 would have to be assigned to either the "2.7" grade or the "3.0" grade, depending on the mood of the corrector.
- Of course, this would have to be done in the same way for all examinees.
(5) Analogous to the solution of the subtask (4) applies to the grade "2.7":
- Pr(62<z<66)=ϕ(0.6)−ϕ(0.2)=0.7257−0.5792=0.1465.
- For reasons of symmetry, the same value is obtained for the grade "3.3":
- Pr(54<z<58)=ϕ(−0.2)−ϕ(−0.6)=Q(0.2)−Q(0.6)=0.1465.
- So each 146 participants receive a grade of "2.7" or "3.3".
(6) With the points/grade assignment made here, not only the points are distributed around mz=60 symmetrically, but also the scores around "3.0". There are
- exactly as many "2.7"s as "3.3"s (around ±0.3 away from 3.0 ),
- exactly as many "2.3 "s as "3.7"s (3.0±0.7), and
- exactly as many "1.0 "s as "5.0 "s.
Therefore, the meangrade3.0_ results.