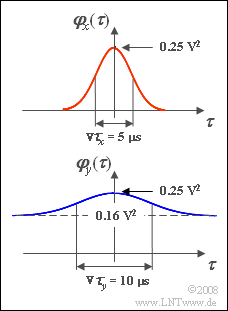
Let the random process considered here $\{x_i(t)\}$ be characterized by the auto-correlation function $\rm (ACF)$ outlined above This random process is mean-free and the equivalent ACF duration is ${ {\rm \nabla} }\tau_x = 5 \hspace{0.08cm} \rm µ s$:
- $$\varphi_x(\tau)=\rm 0.25 V^2\cdot \rm e^{-\pi \hspace{0.03cm}\cdot \hspace{0.03cm} ({\tau}{/ 5 \hspace{0.08cm}{\rm µ}s })^2} .$$
The bottom graph shows the ACF of the process $\{y_i(t)\}$. This reads with the equivalent ACF duration ${ {\rm \nabla} }\tau_y = 10 \hspace{0.08cm} \rm µ s$:
- $$ \varphi_y(\tau)=\rm 0.16 V^2 + \rm 0.09 V^2\cdot\rm e^{-\pi \hspace{0.03cm}\cdot \hspace{0.03cm} ({\tau}/{\nabla \it \tau_y})^2} .$$
In this exercise, the power-spectral densities of the two processes are sought.
Hints:
- This exercise belongs to the chapter Power-spectral density $\rm (PSD)$.
- Reference is also made to the chapter Auto-correlation function $\rm (ACF)$.
- To solve this exercise you can use the following Fourier correspondence:
- $$\rm e^{-\pi \hspace{0.03cm}\cdot \hspace{0.03cm} ({\it f}/{\rm \Delta\it f})^2}\ \bullet\!\!-\!\!\!-\!\!\!-\!\!\circ\,\ {\rm \Delta \it f} \cdot \rm e^{-\pi \hspace{0.05cm}\cdot \hspace{0.05cm} ({\rm \Delta\it f} \hspace{0.05cm}\cdot \hspace{0.05cm}\it t {\rm )}^{\rm 2}}.$$
Questions
Solution
- $$\nabla f_x = 1 / \nabla \tau_x \hspace{0.15cm}\underline{= {\rm 200\hspace{0.1cm}kHz}}.$$
(2) One can adapt the given Fourier correspondence to the task as follows:
- $$K\cdot{\rm e}^{-\pi({\tau}/{\nabla\tau_x})^2}\ \circ\!\!-\!\!\!-\!\!\!-\!\!\bullet\,\ \frac{\it K}{\nabla \it f_x}\cdot{\rm e}^{-\pi({f}/{\nabla f_x})^2}.$$
- With $K = 0.25 \hspace{0.05cm}\rm V^2$ and $ {\rm \nabla} \hspace{-0.05cm} f_x = 200\hspace{0.05cm} \rm kHz$ one obtains:
- $${\it \Phi_x}(f)=1.25\cdot\rm 10^{-\rm 6}\hspace{0.1cm}\frac{V^2}{Hz}\cdot\rm e^{-\pi({\it f}/{\nabla\it f_x})^2}$$
- $$\Rightarrow \hspace{0.3cm}{\it \Phi_x}(f = 0)=\hspace{0.15cm}\underline{\rm 1.25 \cdot 10^{-6} \hspace{0.1cm} V^2\hspace{-0.1cm}/Hz}, \hspace{0.5cm}{\it \Phi_x}(f = 200 \hspace{0.05cm} \rm kHz)=\hspace{0.15cm}\underline{\rm 0.054 \cdot 10^{-6} \hspace{0.1cm} V^2\hspace{-0.1cm}/Hz}.$$
(3) Correct are the solutions 1, 2, and 4:
- A mean-free process always results in a continuous PSD. This is narrower the wider the ACF is ("Reciprocity Law").
- The process power is equal to the integral of the PSD.
- Therefore, at constant power, a wider ACF (narrower PSD) must be compensated by higher PSD values.
- A DC component or periodic components always result in Dirac delta functions in the PSD; otherwise, the PSD is always continuous in value.
(4) Analogous to subtask (2) holds with $ {\rm \nabla} \hspace{-0.05cm} f_y = 100\hspace{0.05cm} \rm kHz$:
- $${\it \Phi_y}(f)=\frac{\rm 0.09 V^2}{\nabla\it f_y}\cdot\rm e^{-\pi({\it f}/{\nabla\it f_y})^2}+\it m_y^{\rm 2}\cdot\delta(f).$$
- Because of the DC component, there is a Dirac delta function at frequency $f = 0$ in addition to the continuous PSD component.
- The continuous PSD–part at $f= 0$ is ${\it \Phi_y}(f = 0)=\hspace{0.15cm}\underline{\rm 0.9 \cdot 10^{-6} \hspace{0.1cm} V^2\hspace{-0.1cm}/Hz}.$
- The continuous PSD–part at $f = 2 \cdot {\rm \nabla} \hspace{-0.05cm} f_y = 200 \hspace{0.05cm}\rm kHz$ is lower by a factor ${\rm e}^{-4} \approx 0.0183$ ⇒ ${\it \Phi_y}(f )=\hspace{0.15cm}\underline{\rm 0.0165 \cdot 10^{-6} \hspace{0.1cm} V^2\hspace{-0.1cm}/Hz}.$
(5) Correct is only the second proposed solution:
- The PSD of a mean-valued process generally involves a Dirac delta function at $f=0$ with weight $m_y^2$.
- In the present case, this value is equal to $0.16 \ \rm V^2$.
- Since $\delta(f)$ has unit $\rm 1/Hz = s$, the units of the continuous and discrete PSD components differ.