Difference between revisions of "Applets:Pulses and Spectra"
| Line 4: | Line 4: | ||
<br> | <br> | ||
Dargestellt werden impulsförmige symmetrische Zeitsignale ⇒ „Impulse” $x(t)$ und die dazugehörigen Spektralfunktionen $X(f)$, nämlich | Dargestellt werden impulsförmige symmetrische Zeitsignale ⇒ „Impulse” $x(t)$ und die dazugehörigen Spektralfunktionen $X(f)$, nämlich | ||
| − | * | + | *Gaussian impulse, |
| − | * | + | *rectangular impulse, |
| − | * | + | *triangular impulse, |
| − | * | + | *trapezoidal impulse, |
| − | * | + | *cosine-rolloff impulse. |
| Line 136: | Line 136: | ||
{{BlaueBox|TEXT= | {{BlaueBox|TEXT= | ||
| − | '''(1)''' Compare the <b>red Gaussian | + | '''(1)''' Compare the <b>red Gaussian impulse</b> $(A_1 = 1, \Delta t_1 = 1)$ with the <b>blue rectangular impulse</b> $(A_2 = 1, \Delta t_2 = 1)$ ⇒ default setting. |
<br> What are the differences in the time and frequency domain?}} | <br> What are the differences in the time and frequency domain?}} | ||
| Line 142: | Line 142: | ||
* Practically $x_1(t)$ for $|t| > 1.5$ and $X_1(f)$ for $|f| > 1.5$ are almost zero.<br> | * Practically $x_1(t)$ for $|t| > 1.5$ and $X_1(f)$ for $|f| > 1.5$ are almost zero.<br> | ||
* The rectangle is strictly limited in time: $x_2(|t| > 0.5) \equiv 0$. $X_2(f)$ has shares in a much larger range than $X_1(f)$. <br> | * The rectangle is strictly limited in time: $x_2(|t| > 0.5) \equiv 0$. $X_2(f)$ has shares in a much larger range than $X_1(f)$. <br> | ||
| − | * It holds $X_1(f = 0) = X_2(f = 0)$ since the integral over the Gaussian | + | * It holds $X_1(f = 0) = X_2(f = 0)$ since the integral over the Gaussian impulse $x_1(t)$ is equal to the integral over the rectangular impulse $x_2(t)$. |
{{BlaueBox|TEXT= | {{BlaueBox|TEXT= | ||
| − | '''(2)''' Compare the <b>red Gaussian | + | '''(2)''' Compare the <b>red Gaussian impulse</b> $(A_1 = 1, \Delta t_1 = 1)$ with the <b>blue rectangular impulse</b> $(A_2 = 1, \Delta t_2)$.<br> Vary the equivalent impulse duration $\Delta t_2$ between $0.5$ and $2$. Interpret the displayed graphs.}} |
| − | * One can recognize the reciprocity law of bandwidth and | + | * One can recognize the reciprocity law of bandwidth and impulse duration. The greater $\Delta t_2$, the higher and narrower the spectral function $X_2(f)$.<br> |
* For each setting of $\Delta t_2$, $x_1(t=0)$ and $x_2(t=0)$ are equal ⇒ Also, the integrals over $X_1(f)$ and $X_2(f)$ are identical. | * For each setting of $\Delta t_2$, $x_1(t=0)$ and $x_2(t=0)$ are equal ⇒ Also, the integrals over $X_1(f)$ and $X_2(f)$ are identical. | ||
{{BlaueBox|TEXT= | {{BlaueBox|TEXT= | ||
| − | '''(3)''' Compare the <b>red Gaussian | + | '''(3)''' Compare the <b>red Gaussian impulse</b> $(A_1 = 1, \Delta t_1 = 1)$ with the <b>blue rectangular impulse</b> $(A_2 = 1, \Delta t_2 = 0.5)$.<br> Vary $\Delta t_2$ between $0.05$ and $2$. Interpret the displayed graphs and extrapolate the result.}} |
* The blue spectrum is now twice as wide as the red one, but only half as high. First zero of $X_1(f)$ at $f = $1, of $X_2(f)$ at $f = $2.<br> | * The blue spectrum is now twice as wide as the red one, but only half as high. First zero of $X_1(f)$ at $f = $1, of $X_2(f)$ at $f = $2.<br> | ||
| Line 163: | Line 163: | ||
{{BlaueBox|TEXT= | {{BlaueBox|TEXT= | ||
| − | '''(4)''' Compare the <b> rectangular | + | '''(4)''' Compare the <b> rectangular impulse</b> $(A_1 = 1, \Delta t_1 = 1)$ with the <b>triangular impulse</b> $(A_2 = 1, \Delta t_2 = 1)$. Interpret the spectral functions.}} |
* The (normalized) spectrum of the rectangle $x_1(t)$ with the (normalized) parameters $A_1 = 1, \ \ \Delta t_1 = 1$ is: $X_1(f)= {\rm si}(\pi\cdot f)$.<br> | * The (normalized) spectrum of the rectangle $x_1(t)$ with the (normalized) parameters $A_1 = 1, \ \ \Delta t_1 = 1$ is: $X_1(f)= {\rm si}(\pi\cdot f)$.<br> | ||
| Line 171: | Line 171: | ||
{{BlaueBox|TEXT= | {{BlaueBox|TEXT= | ||
| − | '''(5)''' | + | '''(5)''' Compare the <b>trapezoidal impulse</b> $(A_1 = 1, \Delta t_1 = 1, r_1 = 0.5)$ with the |
| + | <b>triangular impulse</b> $(A_2 = 1, \Delta t_2 = 1)$.<br> Vary $r_1$ between $0$ and $1$. Interpret the spectral function $X_1(f)$.}} | ||
| − | * | + | * The trapezoidal impulse with roll–off factor $r_1= 0$ is identical to the rectangular impulse. The „normalized spectrum” is $X_1(f)= {\rm si}(\pi\cdot f)$.<br> |
| − | * | + | * The trapezoidal impulse with roll–off factor $r_1= 1$ is identical to the triangular impulse. The „normalized spectrum” is $X_1(f)= {\rm si}^2(\pi\cdot f)$. <br> |
| − | *In | + | * In both cases $X_1(f)$ has equidistant zeros at $\pm 1$, $\pm 2$, ... (none else); $0 < r_1 < 1$: depending on $r_1$ further zeros. |
{{BlaueBox|TEXT= | {{BlaueBox|TEXT= | ||
| − | '''(6)''' | + | '''(6)''' Compare this <b>trapezoidal impulse</b> with the <b>cosine-rolloff impulse</b> |
| + | $(A_2 = 1,\ \Delta t_2 = 1.0,\ r_2 = 0.5)$.<br> Vary $r_2$ between $0$ and $1$. Interpret the spectral function $X_2(f)$ for $r_2 = 0.7$.}} | ||
| − | * | + | * With the same $r= 0.5$ the cosine-rolloff impulse $X_2(f)$ is for $f > 1$ greater in amount than the trapezoidal impulse.<br> |
| − | * | + | * With the same rolloff factor $(r_1 = r_2= 0.5)$ the drop of $X_2(f)$ around the frequency $f = 0.5$ is steeper than the drop of $X_1(f)$. <br> |
| − | * | + | * With $r_1 = 0.5$ and $r_2 = 0.7$ $x_1(t) \approx x_2(t)$ is valid and therefore also $X_1(f) \approx X_2(f)$. Comparable edge steepness. |
{{BlaueBox|TEXT= | {{BlaueBox|TEXT= | ||
| − | '''(7)''' | + | '''(7)''' Compare the <b>red trapezoidal impulse</b> $(A_1 = 1, \Delta t_1 = 1, \ r_1 = 1)$ with the <b>blue cosine-rolloff impulse</b> $(A_2 = 1,\ \Delta t_2 = 1.0, \ r_2 = 1)$.<br> Interpret the time function $x_2(t)$ and the spectral function $X_2(f)$ system theoretically.}} |
| + | |||
| + | * $x_2(t) = \cos^2(|t|\cdot \pi/2) \ \ \ \text{for} \ |t| \le 1$ is the cosine-square impulse. Zeros at $f = \pm 1$, $\pm 2$, ... <br> | ||
| + | * For the frequency $f=\pm 0.5$ one obtains the spectral values $X_2(f)=0.5$. The asymptotic decline is shown here with $1/f^3$. | ||
| − | |||
| − | |||
| Line 197: | Line 200: | ||
| − | [[ | + | [[File:Spektrum_version1.png |left]] |
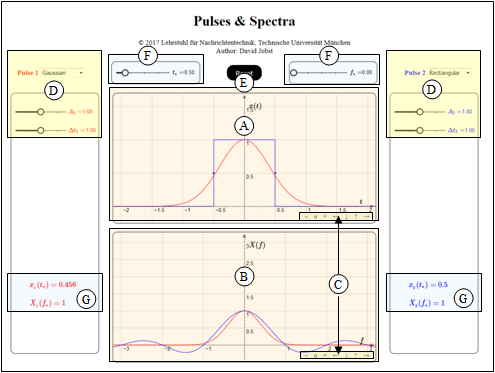
'''(A)''' Bereich der graphischen Darstellung für $x(t)$ | '''(A)''' Bereich der graphischen Darstellung für $x(t)$ | ||
Revision as of 15:13, 1 August 2020
Contents
Applet Description
Dargestellt werden impulsförmige symmetrische Zeitsignale ⇒ „Impulse” $x(t)$ und die dazugehörigen Spektralfunktionen $X(f)$, nämlich
- Gaussian impulse,
- rectangular impulse,
- triangular impulse,
- trapezoidal impulse,
- cosine-rolloff impulse.
Weiter ist zu beachten:
- Die Funktionen $x(t)$ bzw. $X(f)$ werden für bis zu zwei Parametersätzen in jeweils einem Diagramm dargestellt.
- Die roten Kurven und Zahlenangaben gelten für den linken Parametersatz, die blauen für den rechten Parametersatz.
- Die Abszissen $t$ (Zeit) und $f$ (Frequenz) sowie die Ordinaten $x(t)$ (Signalwerte) bzw. $X(f)$ (Spektralwerte) sind jeweils normiert.
Theoretical background
Zusammenhang $x(t)\Leftrightarrow X(f)$
- Der Zusammenhang zwischen der Zeitfunktion $x(t)$ und dem Spektrum $X(f)$ ist durch das erste Fourierintegral gegeben:
- $$X(f)={\rm FT} [x(t)] = \int_{-\infty}^{+\infty}x(t)\cdot {\rm e}^{-{\rm j}2\pi f t}\hspace{0.15cm} {\rm d}t\hspace{1cm} \rm FT\hspace{-0.1cm}: \ Fouriertransformation.$$
- Um aus der Spektralfunktion $X(f)$ die Zeitfunktion $x(t)$ berechnen zu können, benötigt man das zweite Fourierintegral:
- $$x(t)={\rm IFT} [X(f)] = \int_{-\infty}^{+\infty}X(f)\cdot {\rm e}^{+{\rm j}2\pi f t}\hspace{0.15cm} {\rm d}f\hspace{1cm} {\rm IFT}\hspace{-0.1cm}: \rm Inverse \ Fouriertransformation.$$
- In allen Beispielen verwenden wir reelle und gerade Funktionen. Somit gilt:
- $$x(t)=\int_{-\infty}^{+\infty}X(f)\cdot \cos(2\pi ft) \hspace{0.15cm} {\rm d}f \ \ \circ\!\!-\!\!\!-\!\!\!-\!\!\bullet\ \ \ X(f)=\int_{-\infty}^{+\infty}x(t)\cdot \cos(2\pi ft) \hspace{0.15cm} {\rm d}t .$$
- $x(t)$ und $X(f)$ haben unterschiedliche Einheiten, beispielsweise $x(t)$ in $\rm V$, $X(f)$ in $\rm V/Hz$.
- Der Zusammenhang zwischen diesem Modul und dem ähnlich aufgebauten Applet Frequenzgang & Impulsantwort basiert auf dem Vertauschungssatz.
- Alle Zeiten sind auf eine Zeit $T$ normiert und alle Frequenzen auf $1/T$ ⇒ die Spektralwerte $X(f)$ müssen noch mit der Normierungszeit $T$ multipliziert werden.
$\text{Beispiel:}$ Stellt man einen Rechteckimpuls mit Amplitude $A_1 = 1$ und äquivalenter Impulsdauer $\Delta t_1 = 1$ ein, so ist $x_1(t)$ im Bereich $-0.5 < t < +0.5$ gleich Eins und außerhalb dieses Bereichs gleich Null. Die Spektralfunktion $X_1(f)$ verläuft $\rm si$–förmig mit $X_1(f= 0) = 1$ und der ersten Nullstelle bei $f=1$.
- Soll mit dieser Einstellung ein Rechteckimpuls mit $A = K = 3 \ \rm V$ und $\Delta t = T = 2 \ \rm ms$ nachgebildet werden, dann sind alle Signalwerte mit $K = 3 \ \rm V$ und alle Spektralwerte mit $K \cdot T = 0.006 \ \rm V/Hz$ zu multiplizieren.
- Der maximale Spektralwert ist dann $X(f= 0) = 0.006 \ \rm V/Hz$ und die erste Nullstelle liegt bei $f=1/T = 0.5 \ \rm kHz$.
Gaussian Impulse
- Die Zeitfunktion des Gaußimpulses mit der Höhe $K$ und der (äquivalenten) Dauer $\Delta t$ lautet:
- $$x(t)=K\cdot {\rm e}^{-\pi\hspace{0.05cm}\cdot \hspace{0.05cm}(t/\Delta t)^2}.$$
- Die äquivalente Zeitdauer $\Delta t$ ergibt sich aus dem flächengleichen Rechteck.
- Der Wert bei $t = \Delta t/2$ ist um den Faktor $0.456$ kleiner als der Wert bei $t=0$.
- Für die Spektralfunktion erhält man gemäß der Fouriertransformation:
- $$X(f)=K\cdot \Delta t \cdot {\rm e}^{-\pi(f\hspace{0.05cm}\cdot \hspace{0.05cm} \Delta t)^2} .$$
- Je kleiner die äquivalente Zeitdauer $\Delta t$ ist, um so breiter und niedriger ist das Spektrum ⇒ Reziprozitätsgesetz von Bandbreite und Impulsdauer.
- Sowohl $x(t)$ als auch $X(f)$ sind zu keinem $f$– bzw. $t$–Wert exakt gleich Null.
- Für praktische Anwendungen kann der Gaußimpuls jedoch in Zeit und Frequenz als begrenzt angenommen werden. Zum Beispiel ist $x(t)$ bereits bei $t=1.5 \Delta t$ auf weniger als $0.1\% $ des Maximums abgefallen.
Rectangular Impulse
- Die Zeitfunktion des Rechteckimpulses mit der Höhe $K$ und der (äquivalenten) Dauer $\Delta t$ lautet:
- $$x(t) = \left\{ \begin{array}{l} \hspace{0.25cm}K \\ K /2 \\ \hspace{0.25cm} 0 \\ \end{array} \right.\quad \quad \begin{array}{*{20}c} {\rm{f\ddot{u}r}} \\ {\rm{f\ddot{u}r}} \\ {\rm{f\ddot{u}r}} \\ \end{array}\begin{array}{*{20}c} {\left| \hspace{0.05cm} t\hspace{0.05cm} \right| < T/2,} \\ {\left| \hspace{0.05cm}t\hspace{0.05cm} \right| = T/2,} \\ {\left|\hspace{0.05cm} t \hspace{0.05cm} \right| > T/2.} \\ \end{array}$$
- Der $\pm \Delta t/2$–Wert liegt mittig zwischen links- und rechtsseitigem Grenzwert.
- Für die Spektralfunktion erhält man entsprechend den Gesetzmäßigkeiten der Fouriertransformation (1. Fourierintegral):
- $$X(f)=K\cdot \Delta t \cdot {\rm si}(\pi\cdot \Delta t \cdot f) \quad \text{mit} \ {\rm si}(x)=\frac{\sin(x)}{x}.$$
- Der Spektralwert bei $f=0$ ist gleich der Rechteckfläche der Zeitfunktion.
- Die Spektralfunktion besitzt Nullstellen in äquidistanten Abständen $1/\Delta t$.
- Das Integral über der Spektralfunktion $X(f)$ ist gleich dem Signalwert zum Zeitpunkt $t=0$, also der Impulshöhe $K$.
Triangular Impulse
- Die Zeitfunktion des Dreieckimpulses mit der Höhe $K$ und der (äquivalenten) Dauer $\Delta t$ lautet:
- $$x(t) = \left\{ \begin{array}{l} \hspace{0.25cm}K\cdot (1-|t|/{\Delta t}) \\ \hspace{0.25cm} 0 \\ \end{array} \right.\quad \quad \begin{array}{*{20}c} {\rm{f\ddot{u}r}} \\ {\rm{f\ddot{u}r}} \\ \end{array}\begin{array}{*{20}c} {\left| \hspace{0.05cm} t\hspace{0.05cm} \right| < \Delta t,} \\ {\left| \hspace{0.05cm}t\hspace{0.05cm} \right| \ge \Delta t.} \\ \end{array}$$
- Die absolute Zeitdauer ist $2 \cdot \Delta t$; diese ist doppelt so groß als die des Rechtecks.
- Für die Spektralfunktion erhält man gemäß der Fouriertransformation:
- $$X(f)=K\cdot \Delta f \cdot {\rm si}^2(\pi\cdot \Delta t \cdot f) \quad \text{mit} \quad {\rm si}(x)=\frac{\sin(x)}{x}.$$
- Obige Zeitfunktion ist gleich der Faltung zweier Rechteckimpulse, jeweils mit Breite $\Delta t$.
- Daraus folgt: $X(f)$ beinhaltet anstelle der ${\rm si}$-Funktion die ${\rm si}^2$-Funktion.
- $X(f)$ weist somit ebenfalls Nullstellen im äquidistanten Abständen $1/\Delta f$ auf.
- Der asymptotische Abfall von $X(f)$ erfolgt hier mit $1/f^2$, während zum Vergleich der Rechteckimpuls mit $1/f$ abfällt.
Trapezoidal Impulse
Die Zeitfunktion des Trapezimpulses mit der Höhe $K$ und den Zeitparametern $t_1$ und $t_2$ lautet:
- $$x(t) = \left\{ \begin{array}{l} \hspace{0.25cm}K \\ K\cdot \frac{t_2-|t|}{t_2-t_1} \\ \hspace{0.25cm} 0 \\ \end{array} \right.\quad \quad \begin{array}{*{20}c} {\rm{f\ddot{u}r}}\quad \\ {\rm{f\ddot{u}r}}\quad \\ {\rm{f\ddot{u}r}} \quad \\ \end{array}\begin{array}{*{20}c} {\left| \hspace{0.05cm} t\hspace{0.05cm} \right| \le t_1,} \\ {t_1\le \left| \hspace{0.05cm}t\hspace{0.05cm} \right| \le t_2,} \\ {\left|\hspace{0.05cm} t \hspace{0.05cm} \right| \ge t_2.} \\ \end{array}$$
- Für die äquivalente Impulsdauer (flächengleiches Rechteck) gilt: $\Delta t = t_1+t_2$.
- Der Rolloff-Faktor (im Zeitbereich) kennzeichnet die Flankensteilheit:
- $$r=\frac{t_2-t_1}{t_2+t_1}.$$
- Der Sonderfall $r=0$ entspricht dem Rechteckimpuls und der Sonderfall $r=1$ dem Dreieckimpuls.
- Für die Spektralfunktion erhält man gemäß der Fouriertransformation:
- $$X(f)=K\cdot \Delta t \cdot {\rm si}(\pi\cdot \Delta t \cdot f)\cdot {\rm si}(\pi \cdot r \cdot \Delta t \cdot f) \quad \text{mit} \quad {\rm si}(x)=\frac{\sin(x)}{x}.$$
- Der asymptotische Abfall von $X(f)$ liegt zwischen $1/f$ $($für Rechteck, $r=0)$ und $1/f^2$ $($für Dreieck, $r=1)$.
Cosine-rolloff Impulse
Die Zeitfunktion des Cosinus-Rolloff-Impulses mit der Höhe $K$ und den Zeitparametern $t_1$ und $t_2$ lautet:
- $$x(t) = \left\{ \begin{array}{l} \hspace{0.25cm}K \\ K\cdot \cos^2\Big(\frac{|t|-t_1}{t_2-t_1}\cdot {\pi}/{2}\Big) \\ \hspace{0.25cm} 0 \\ \end{array} \right.\quad \quad \begin{array}{*{20}c} {\rm{f\ddot{u}r}}\quad \\ {\rm{f\ddot{u}r}}\quad \\ {\rm{f\ddot{u}r}}\quad \\ \end{array}\begin{array}{*{20}c} {\left| \hspace{0.05cm} t\hspace{0.05cm} \right| \le t_1,} \\ {t_1\le \left| \hspace{0.05cm}t\hspace{0.05cm} \right| \le t_2,} \\ {\left|\hspace{0.05cm} t \hspace{0.05cm} \right| \ge t_2.} \\ \end{array}$$
- Für die äquivalente Impulsdauer (flächengleiches Rechteck) gilt: $\Delta t = t_1+t_2$.
- Der Rolloff-Faktor (im Zeitbereich) kennzeichnet die Flankensteilheit:
- $$r=\frac{t_2-t_1}{t_2+t_1}.$$
- Der Sonderfall $r=0$ entspricht dem Rechteckimpuls und der Sonderfall $r=1$ dem Cosinus-Quadrat-Impuls.
- Für die Spektralfunktion erhält man gemäß der Fouriertransformation:
- $$X(f)=K\cdot \Delta t \cdot \frac{\cos(\pi \cdot r\cdot \Delta t \cdot f)}{1-(2\cdot r\cdot \Delta t \cdot f)^2} \cdot {\rm si}(\pi \cdot \Delta t \cdot f).$$
- Je größer der Rolloff-Faktor $r$ ist, desto schneller nimmt $X(f)$ asymptotisch mit $f$ ab.
Cosinus-square Impulse
- Dies ist ein Sonderfall des Cosinus-Rolloff-Impulses und ergibt sich für $r=1 \hspace{0.3cm} \Rightarrow \hspace{0.3cm}t_1=0, \ t_2= \Delta t$:
- $$x(t) = \left\{ \begin{array}{l} \hspace{0.25cm}K\cdot \cos^2\Big(\frac{|t|\hspace{0.05cm}\cdot \hspace{0.05cm} \pi}{2\hspace{0.05cm}\cdot \hspace{0.05cm} \Delta t}\Big) \\ \hspace{0.25cm} 0 \\ \end{array} \right.\quad \quad \begin{array}{*{20}c} {\rm{f\ddot{u}r}} \\ {\rm{f\ddot{u}r}} \\ \end{array}\begin{array}{*{20}c} {\left| \hspace{0.05cm} t\hspace{0.05cm} \right| < \Delta t,} \\ {\left| \hspace{0.05cm}t\hspace{0.05cm} \right| \ge \Delta t.} \\ \end{array}$$
- Für die Spektralfunktion erhält man gemäß der Fouriertransformation:
- $$X(f)=K\cdot \Delta f \cdot \frac{\pi}{4}\cdot \big [{\rm si}(\pi(\Delta t\cdot f +0.5))+{\rm si}(\pi(\Delta t\cdot f -0.5))\big ]\cdot {\rm si}(\pi \cdot \Delta t \cdot f).$$
- Wegen der letzten ${\rm si}$-Funktion ist $X(f)=0$ für alle Vielfachen von $F=1/\Delta t$. Die äquidistanten Nulldurchgänge des Cos-Rolloff-Impulses bleiben erhalten.
- Aufgrund des Klammerausdrucks weist $X(f)$ nun weitere Nulldurchgänge bei $f=\pm1.5 F$, $\pm2.5 F$, $\pm3.5 F$, ... auf.
- Für die Frequenz $f=\pm F/2$ erhält man die Spektralwerte $K\cdot \Delta t/2$.
- Der asymptotische Abfall von $X(f)$ verläuft in diesem Sonderfall mit $1/f^3$.
Exercises
- First select the number $(1,\text{...}, 7)$ of the exercise.
- A task description is displayed. The parameter values are adjusted.
- Solution after pressing "Show solution".
- The number $0$ corresponds to a "Reset": Same setting as at program start.
- "Red" refers to the first parameter set ⇒ $x_1(t) \circ\!\!-\!\!\!-\!\!\!-\!\!\bullet\ X_1(f)$.
- "Blue" refers to the second parameter set ⇒ $x_2(t) \circ\!\!-\!\!\!-\!\!\!-\!\!\bullet\ X_2(f)$.
- Zahlenwerte betragsmäig kleiner als $0.0005$ werden im Programm als „Null” ausgegeben.
(1) Compare the red Gaussian impulse $(A_1 = 1, \Delta t_1 = 1)$ with the blue rectangular impulse $(A_2 = 1, \Delta t_2 = 1)$ ⇒ default setting.
What are the differences in the time and frequency domain?
- The Gaussian impulse theoretically reaches infinity in the time– as well as in the frequency domain.
- Practically $x_1(t)$ for $|t| > 1.5$ and $X_1(f)$ for $|f| > 1.5$ are almost zero.
- The rectangle is strictly limited in time: $x_2(|t| > 0.5) \equiv 0$. $X_2(f)$ has shares in a much larger range than $X_1(f)$.
- It holds $X_1(f = 0) = X_2(f = 0)$ since the integral over the Gaussian impulse $x_1(t)$ is equal to the integral over the rectangular impulse $x_2(t)$.
(2) Compare the red Gaussian impulse $(A_1 = 1, \Delta t_1 = 1)$ with the blue rectangular impulse $(A_2 = 1, \Delta t_2)$.
Vary the equivalent impulse duration $\Delta t_2$ between $0.5$ and $2$. Interpret the displayed graphs.
- One can recognize the reciprocity law of bandwidth and impulse duration. The greater $\Delta t_2$, the higher and narrower the spectral function $X_2(f)$.
- For each setting of $\Delta t_2$, $x_1(t=0)$ and $x_2(t=0)$ are equal ⇒ Also, the integrals over $X_1(f)$ and $X_2(f)$ are identical.
(3) Compare the red Gaussian impulse $(A_1 = 1, \Delta t_1 = 1)$ with the blue rectangular impulse $(A_2 = 1, \Delta t_2 = 0.5)$.
Vary $\Delta t_2$ between $0.05$ and $2$. Interpret the displayed graphs and extrapolate the result.
- The blue spectrum is now twice as wide as the red one, but only half as high. First zero of $X_1(f)$ at $f = $1, of $X_2(f)$ at $f = $2.
- Reduction of $\Delta t_2$: $X_2(f)$ lower and wider. Very flat course at $\Delta t_2 = 0.05$: $X_2(f = 0)= 0.05$, $X_2(f = \pm 3)= 0.048$.
- If one chose $\Delta t_2 = \varepsilon \to 0$ (not possible in the program), the result would be the almost constant, very small spectrum $X_2(f)=A \cdot \varepsilon \to 0$.
- Increasing the amplitude to $A=1/\varepsilon$ results in the constant spectral function $X_2(f) = 1$ of the Dirac function $\delta(t)$. That means:
- $\delta(t)$ is approximated by a rectangle $($width $\Delta t = \varepsilon \to 0$, height $A = 1/\varepsilon \to \infty)$. The weight of the Dirac function is one: $x(t) = 1 \cdot \delta (t)$.
(4) Compare the rectangular impulse $(A_1 = 1, \Delta t_1 = 1)$ with the triangular impulse $(A_2 = 1, \Delta t_2 = 1)$. Interpret the spectral functions.
- The (normalized) spectrum of the rectangle $x_1(t)$ with the (normalized) parameters $A_1 = 1, \ \ \Delta t_1 = 1$ is: $X_1(f)= {\rm si}(\pi\cdot f)$.
- The convolution of the rectangle $x_1(t)$ with itself gives the triangle $x_2(t) = x_1(t) \star x_1(t)$. By the convolution theorem: $X_2(f) = X_1(f)^2 $.
- By squaring the $\rm si$–shaped spectral function $X_1(f)$ the zeros of $X_2(f)$ remain unchanged. But now it holds that: $X_2(f) \ge 0$.
(5) Compare the trapezoidal impulse $(A_1 = 1, \Delta t_1 = 1, r_1 = 0.5)$ with the
triangular impulse $(A_2 = 1, \Delta t_2 = 1)$.
Vary $r_1$ between $0$ and $1$. Interpret the spectral function $X_1(f)$.
- The trapezoidal impulse with roll–off factor $r_1= 0$ is identical to the rectangular impulse. The „normalized spectrum” is $X_1(f)= {\rm si}(\pi\cdot f)$.
- The trapezoidal impulse with roll–off factor $r_1= 1$ is identical to the triangular impulse. The „normalized spectrum” is $X_1(f)= {\rm si}^2(\pi\cdot f)$.
- In both cases $X_1(f)$ has equidistant zeros at $\pm 1$, $\pm 2$, ... (none else); $0 < r_1 < 1$: depending on $r_1$ further zeros.
(6) Compare this trapezoidal impulse with the cosine-rolloff impulse
$(A_2 = 1,\ \Delta t_2 = 1.0,\ r_2 = 0.5)$.
Vary $r_2$ between $0$ and $1$. Interpret the spectral function $X_2(f)$ for $r_2 = 0.7$.
- With the same $r= 0.5$ the cosine-rolloff impulse $X_2(f)$ is for $f > 1$ greater in amount than the trapezoidal impulse.
- With the same rolloff factor $(r_1 = r_2= 0.5)$ the drop of $X_2(f)$ around the frequency $f = 0.5$ is steeper than the drop of $X_1(f)$.
- With $r_1 = 0.5$ and $r_2 = 0.7$ $x_1(t) \approx x_2(t)$ is valid and therefore also $X_1(f) \approx X_2(f)$. Comparable edge steepness.
(7) Compare the red trapezoidal impulse $(A_1 = 1, \Delta t_1 = 1, \ r_1 = 1)$ with the blue cosine-rolloff impulse $(A_2 = 1,\ \Delta t_2 = 1.0, \ r_2 = 1)$.
Interpret the time function $x_2(t)$ and the spectral function $X_2(f)$ system theoretically.
- $x_2(t) = \cos^2(|t|\cdot \pi/2) \ \ \ \text{for} \ |t| \le 1$ is the cosine-square impulse. Zeros at $f = \pm 1$, $\pm 2$, ...
- For the frequency $f=\pm 0.5$ one obtains the spectral values $X_2(f)=0.5$. The asymptotic decline is shown here with $1/f^3$.
Applet Manual
(A) Bereich der graphischen Darstellung für $x(t)$
(B) Bereich der graphischen Darstellung für $X(f)$
(C) Variationsmöglichkeit für die graphischen Darstellungen
(D) Parametereingabe per Slider
links (rot): „Pulse 1”, rechts (blau): „Pulse 2”
(E) Parameter entsprechend der Voreinstellung ⇒ „Reset”
(F) Einstellung von $t_*$ und $f_*$ für Numerikausgabe
(G) Numerikausgabe von $x(t_*)$ und $X(f_*)$
links (rot): „Pulse 1”, rechts (blau): „Pulse 2”
Details zum obigen Punkt (C)
(*) Zoom–Funktionen „$+$” (Vergrößern), „$-$” (Verkleinern) und $\rm o$ (Zurücksetzen)
(*) Verschiebe–Funktionen „$\leftarrow$” (Bildausschnitt nach links, Ordinate nach rechts) sowie „$\uparrow$” „$\downarrow$” „$\rightarrow$”
Andere Möglichkeiten:
- Bei gedrückter Shifttaste und Scrollen kann im Koordinatensystem gezoomt werden.
- Bei gedrückter Shifttaste und gedrückter linker Maustaste kann das Koordinatensystem verschoben werden.
About the authors
Dieses interaktive Berechnungstool wurde am Lehrstuhl für Nachrichtentechnik der Technischen Universität München konzipiert und realisiert.
- Die erste Version wurde 2005 von Ji Li im Rahmen ihrer Diplomarbeit mit „FlashMX–Actionscript” erstellt (Betreuer: Günter Söder und Klaus Eichin).
- 2017 wurde „Impulse & Spektren” von David Jobst im Rahmen seiner Ingenieurspraxis (Betreuer: Tasnád Kernetzky) auf „HTML5” umgesetzt und neu gestaltet.
- Letztmalige Überarbeitung 2020 durch Carolin Mirschina im Rahmen einer Werkstudententätigkeit.