Difference between revisions of "Aufgaben:Exercise 1.4: Rayleigh PDF and Jakes PDS"
| Line 3: | Line 3: | ||
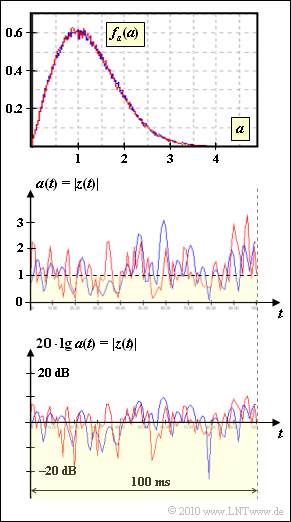
[[File:P_ID2119__Mob_A_1_4.png|right|frame| WDF und $|z(t)|$ bei Rayleigh-Fading mit Dopplereinfluss]] | [[File:P_ID2119__Mob_A_1_4.png|right|frame| WDF und $|z(t)|$ bei Rayleigh-Fading mit Dopplereinfluss]] | ||
| − | We consider two different mobile radio channels with [[Mobile_Kommunikation/Wahrscheinlichkeitsdichte_des_Rayleigh%E2%80%93Fadings#Beispielhafte_Signalverl.C3.A4ufe_bei_Rayleigh.E2.80.93Fading|Rayleigh fading]]. In both cases the PDF of the magnitude $a(t) = |z(t)| ≥ 0$ | + | We consider two different mobile radio channels with [[Mobile_Kommunikation/Wahrscheinlichkeitsdichte_des_Rayleigh%E2%80%93Fadings#Beispielhafte_Signalverl.C3.A4ufe_bei_Rayleigh.E2.80.93Fading|Rayleigh fading]]. In both cases the PDF of the magnitude $a(t) = |z(t)| ≥ 0$ is |
$$f_a(a) = \frac{a}{\sigma^2} \cdot {\rm e}^{ -{a^2}/(2\sigma^2)} | $$f_a(a) = \frac{a}{\sigma^2} \cdot {\rm e}^{ -{a^2}/(2\sigma^2)} | ||
\hspace{0.05cm}.$$ | \hspace{0.05cm}.$$ | ||
| − | The probability that this amount is not greater than a given value $A$ | + | The probability that this amount is not greater than a given value $A$ is |
$${\rm Pr}(|z(t)| \le A) = 1 - {\rm e}^{ -{A^2}/(2\sigma^2)} | $${\rm Pr}(|z(t)| \le A) = 1 - {\rm e}^{ -{A^2}/(2\sigma^2)} | ||
\hspace{0.05cm}.$$ | \hspace{0.05cm}.$$ | ||
| − | The two channels, which are designated according to the colors „Red” and „Blue” in the | + | The two channels, which are designated according to the colors „Red” and „Blue” in the graphs with $\rm R$ and $\rm B$ respectively, differ in the speed $v$ and thus in the form of the power density spectrum ${\it \Phi}_z(f_{\rm D})$. |
*In both cases, however, a so-called [[Mobile_Communication/Statistical_Bonds_within_Rayleigh%E2%80%93Process#AKF_and_LDS_at_Rayleigh.E2.80.93Fading|Jakes–Spectrum]] results. | *In both cases, however, a so-called [[Mobile_Communication/Statistical_Bonds_within_Rayleigh%E2%80%93Process#AKF_and_LDS_at_Rayleigh.E2.80.93Fading|Jakes–Spectrum]] results. | ||
Revision as of 19:26, 25 March 2020
We consider two different mobile radio channels with Rayleigh fading. In both cases the PDF of the magnitude $a(t) = |z(t)| ≥ 0$ is $$f_a(a) = \frac{a}{\sigma^2} \cdot {\rm e}^{ -{a^2}/(2\sigma^2)} \hspace{0.05cm}.$$
The probability that this amount is not greater than a given value $A$ is $${\rm Pr}(|z(t)| \le A) = 1 - {\rm e}^{ -{A^2}/(2\sigma^2)} \hspace{0.05cm}.$$
The two channels, which are designated according to the colors „Red” and „Blue” in the graphs with $\rm R$ and $\rm B$ respectively, differ in the speed $v$ and thus in the form of the power density spectrum ${\it \Phi}_z(f_{\rm D})$.
- In both cases, however, a so-called Jakes–Spectrum results.
- For a Doppler frequency $f_{\rm D}$ with $|f_{\rm D}| <f_{\rm D,\hspace{0.05cm}max}$ the equation is
$${\it \pi}_z(f_{\rm D}) = \frac{1}{\pi \hspace{-0.05cm}\cdot \hspace{-0.05cm}f_{\rm D, \hspace{0.05cm} max} \hspace{-0.05cm}\cdot \hspace{-0.05cm}\sqrt{ 1 \hspace{-0.05cm}- \hspace{-0.05cm}(f_{\rm D}/f_{\rm D, \hspace{0.05cm} max})^2} } \hspace{0.05cm}.$$
- Doppler frequencies outside this interval from $-f_{\rm D,\hspace{0.05cm}max}$ to $+f_{\rm D,\hspace{0.05cm}max}$ are excluded.
The corresponding descriptor in the time domain is the autocorrelation function (ACF):
$$\varphi_z ({\rm \delta}t) = 2 \sigma^2 \cdot {\rm J_0}(2\pi \cdot f_{\rm D, \hspace{0.05cm} max} \cdot {\rm \delta}t)\hspace{0.05cm}.$$
- Here denoted ${\rm J_0}(.)$ the first-order and zero-order Bessel function. It applies ${\rm J_0}(0) = 1$.
- The maximum Doppler frequency is known from the channel model $\rm R$ : $f_{\rm D,\hspace{0.05cm}max} = 200 \ \rm Hz$.
- It is also known that the speeds $v_{\rm R}$ and $v_{\rm B}$ differ by the factor $2$ .
- Ob $v_{\rm R}$ is twice as large as $v_{\rm B}$ or vice versa, you should decide based on the above graphics.
Notes:
- The task belongs to the topic of Statistical_Bondswithin_the_Rayleigh–Process.
- To check your results you can use the interactive applet WDF, VTF and Moments .
Questionnaire
Sample solution
- The Rayleigh–WDF and its derivation are general
- $$f_a(a) \hspace{-0.1cm} = \hspace{-0.1cm} \frac{a}{\sigma^2} \cdot {\rm e}^{ -a^2/(2\sigma^2)} \hspace{0.05cm},$$
$$\frac{\rm d}f_a(a)}{\rm d}a \hspace{-0.1cm} = \hspace{-0.1cm} \frac{1}{\sigma^2} \cdot {\rm e}^{ -a^2/(2\sigma^2)}- \frac{a^2}{\sigma^4} \cdot {\rm e}^{ -a^2/(2\sigma^2)} \hspace{0.05cm}.$$
- By zeroing the derivative, you can show that the WDF–maximum occurs at $a = \sigma$. Since the Rayleigh–WDF applies to both channels, it follows that
$$\sigma_{\rm R} = \sigma_{\rm B} \hspace{0.15cm} \underline{ = 1} \hspace{0.05cm}.$$
(2) Because of the same WDF, the searched probability is also the same for both channels.
- With the given equation you get for this
$${\rm Pr}(a \le 0.316) = {\rm Pr}(20 \cdot {\rm lg}\hspace{0.15cm} a \le -10\,\,{\rm dB}) = 1 - {\rm e}^{ -{0.316^2}/(2\sigma^2)} = 1- 0.951 \hspace{0.15cm} \underline{ \approx 4.9 \%} \hspace{0.05cm}.$$
(3) The correct solutions are 2, 3 and 6:
- The smaller speed $v_{\rm B}$ can be recognized by the fact that the amount $|z(t)|$ changes more slowly with the blue curve.
- With the vehicle stationary, the LDS degenerates to ${\it \Phi_z}(f_{\rm D}) = 2\sigma^2\cdot \delta(f_{\rm D})$, and it is $|z(t)| = A = \rm const.$, where the constant $A$ is diced according to the Rayleigh distribution.
- At extremely high speed, the Jakes–spectrum becomes flat and lower over an increasingly wide range. It then approaches the LDS of white noise. However, $v$ would have to be in the order of the speed of light.
(4) Correct are the statements 2 and 3:
- The Rayleigh–parameter $\sigma = 1$ also determines the „power” ${\rm E}[|z(t)|^2] = 2\sigma^2 = 2$ of the random process.
- This applies to both R and B:
$$\varphi_z ({\rm \delta}t = 0) = 2 \hspace{0.05cm}, \hspace{0.2cm} \int_{-\infty}^{+\infty}{\it \Phi}_z(f_{\rm D}) \hspace{0.15cm}{\rm d}f_{\rm D} = 2 \hspace{0.05cm}.$$