Difference between revisions of "Aufgaben:Exercise 1.6Z: Ergodic Probabilities"
From LNTwww
| Line 1: | Line 1: | ||
| − | {{quiz-Header|Buchseite= | + | {{quiz-Header|Buchseite=Theory_of_Stochastic_Signals/Markov_Chains}} |
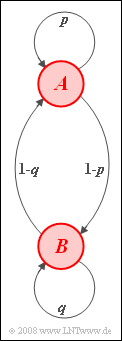
| − | [[File:P_ID452__Sto_Z_1_6.png|right|frame| | + | [[File:P_ID452__Sto_Z_1_6.png|right|frame|Binary Markov chain with $A$ and $B$]] |
| − | + | We consider a homogeneous stationary first-order Markov chain with events $A$ and $B$ and transition probabilities corresponding to the adjacent Markov diagram: | |
| − | + | For subtasks '''(1)''' to '''(4)''' , assume: | |
| − | * | + | *Event $A$ is followed by $A$ and $B$ with equal probability. |
| − | * | + | *After $B$ , event $A$ is twice as likely as $B$. |
| − | + | From subtask '''(5)''' on, $p$ and $q$ are free parameters, while the event probabilities ${\rm Pr}(A) = 2/3$ and ${\rm Pr}(B) = 1/3$ are fixed. | |
| Line 20: | Line 20: | ||
| − | + | Hints: | |
| − | + | *The exercise belongs to the chapter [[Theory_of_Stochastic_Signals/Markov_Chains|Markov Chains]]. | |
| − | * | ||
| − | * | + | *You can check your results with the interactive applet [[Applets:Markovketten|Event probabilities of a 1st order Markov chain]] . |
| − | === | + | ===Questions=== |
<quiz display=simple> | <quiz display=simple> | ||
| − | { | + | {What are the transition probabilities $p$ and $q$? |
|type="{}"} | |type="{}"} | ||
$p \ = \ $ { 0.5 3% } | $p \ = \ $ { 0.5 3% } | ||
$q \ = \ $ { 0.333 3% } | $q \ = \ $ { 0.333 3% } | ||
| − | { | + | {Calculate the ergodic probabilities. |
|type="{}"} | |type="{}"} | ||
${\rm Pr}(A) \ = \ $ { 0.571 3% } | ${\rm Pr}(A) \ = \ $ { 0.571 3% } | ||
${\rm Pr}(B) \ = \ $ { 0.429 3% } | ${\rm Pr}(B) \ = \ $ { 0.429 3% } | ||
| − | { | + | {What is the conditional probability that event $B$ occurs if event $A$ occurred two bars before? |
|type="{}"} | |type="{}"} | ||
${\rm Pr}(B_{\nu}\hspace{0.05cm}|\hspace{0.05cm}A_{\nu-2})\ = \ $ { 0.417 3% } | ${\rm Pr}(B_{\nu}\hspace{0.05cm}|\hspace{0.05cm}A_{\nu-2})\ = \ $ { 0.417 3% } | ||
| − | { | + | {What is the inferential probability that event $A$ occurred two bars before when event $B$ currently occurs? |
|type="{}"} | |type="{}"} | ||
${\rm Pr}(A_{\nu-2}\hspace{0.05cm}|\hspace{0.05cm}B_{\nu})\ = \ $ { 0.556 3% } | ${\rm Pr}(A_{\nu-2}\hspace{0.05cm}|\hspace{0.05cm}B_{\nu})\ = \ $ { 0.556 3% } | ||
| − | { | + | {Let now $p = 1/2$ and ${\rm Pr}(A) = 2/3$. Which value results for $q$? |
|type="{}"} | |type="{}"} | ||
$q\ = \ $ { 0. } | $q\ = \ $ { 0. } | ||
| − | { | + | {How must the parameters be chosen so that the sequence elements of the Markov chain are statistically independent and additionally ${\rm Pr}(A) = 2/3$ gilt? |
|type="{}"} | |type="{}"} | ||
$p \ = \ $ { 0.667 3% } | $p \ = \ $ { 0.667 3% } | ||
| Line 61: | Line 60: | ||
</quiz> | </quiz> | ||
| − | === | + | ===Solution=== |
{{ML-Kopf}} | {{ML-Kopf}} | ||
| − | '''(1)''' | + | '''(1)''' According to the instruction, $p = 1 - p$ ⇒ $\underline{p =0.500}$ and $q = (1 - q)/2$, ⇒ $\underline{q =0.333}$ holds. |
| − | '''(2)''' | + | '''(2)''' For the event probability of $A$ holds: |
:$${\rm Pr}(A) = \frac{{\rm Pr}(A \hspace{0.05cm} | \hspace{0.05cm}B)}{{\rm Pr}(A \hspace{0.05cm} | \hspace{0.05cm}B)+{\rm Pr}(B \hspace{0.05cm} | \hspace{0.05cm}A)} = \frac{1-q}{1-q+1-p} = \frac{2/3}{2/3 + 1/2}= \frac{4}{7} \hspace{0.15cm}\underline {\approx0.571}.$$ | :$${\rm Pr}(A) = \frac{{\rm Pr}(A \hspace{0.05cm} | \hspace{0.05cm}B)}{{\rm Pr}(A \hspace{0.05cm} | \hspace{0.05cm}B)+{\rm Pr}(B \hspace{0.05cm} | \hspace{0.05cm}A)} = \frac{1-q}{1-q+1-p} = \frac{2/3}{2/3 + 1/2}= \frac{4}{7} \hspace{0.15cm}\underline {\approx0.571}.$$ | ||
| − | * | + | *This gives ${\rm Pr}(B)= 1 - {\rm Pr}(A) = 3/7 \hspace{0.15cm}\underline {\approx 0.429}$. |
| − | '''(3)''' | + | '''(3)''' No statement is made about the time $\nu-1$ . |
| − | * | + | *At this time $A$ or $B$ may have occurred. Therefore holds: |
:$${\rm Pr}(B_{\nu} \hspace{0.05cm} | \hspace{0.05cm}A_{\nu -2}) = {\rm Pr}(A \hspace{0.05cm} | \hspace{0.05cm}A) \hspace{0.05cm} \cdot \hspace{0.05cm}{\rm Pr}(B \hspace{0.05cm} | \hspace{0.05cm}A) \hspace{0.15cm} +\hspace{0.15cm} {\rm Pr}(B \hspace{0.05cm} | \hspace{0.05cm}A) \hspace{0.05cm} \cdot \hspace{0.05cm}{\rm Pr}(B \hspace{0.05cm} | \hspace{0.05cm}B) = p \hspace{0.1cm} \cdot \hspace{0.1cm} (1-p) + q \hspace{0.1cm} \cdot \hspace{0.1cm} (1-p) | :$${\rm Pr}(B_{\nu} \hspace{0.05cm} | \hspace{0.05cm}A_{\nu -2}) = {\rm Pr}(A \hspace{0.05cm} | \hspace{0.05cm}A) \hspace{0.05cm} \cdot \hspace{0.05cm}{\rm Pr}(B \hspace{0.05cm} | \hspace{0.05cm}A) \hspace{0.15cm} +\hspace{0.15cm} {\rm Pr}(B \hspace{0.05cm} | \hspace{0.05cm}A) \hspace{0.05cm} \cdot \hspace{0.05cm}{\rm Pr}(B \hspace{0.05cm} | \hspace{0.05cm}B) = p \hspace{0.1cm} \cdot \hspace{0.1cm} (1-p) + q \hspace{0.1cm} \cdot \hspace{0.1cm} (1-p) | ||
= {5}/{12} \hspace{0.15cm}\underline {\approx 0.417}.$$ | = {5}/{12} \hspace{0.15cm}\underline {\approx 0.417}.$$ | ||
| Line 81: | Line 80: | ||
| − | '''(4)''' | + | '''(4)''' According to Bayes' theorem: |
:$${\rm Pr}(A_{\nu -2} \hspace{0.05cm} | \hspace{0.05cm}B_{\nu}) = \frac{{\rm Pr}(B_{\nu} \hspace{0.05cm} | \hspace{0.05cm}A_{\nu -2}) \cdot {\rm Pr}(A_{\nu -2} ) }{{\rm Pr}(B_{\nu}) } = \frac{5/12 \cdot 4/7 }{3/7 } | :$${\rm Pr}(A_{\nu -2} \hspace{0.05cm} | \hspace{0.05cm}B_{\nu}) = \frac{{\rm Pr}(B_{\nu} \hspace{0.05cm} | \hspace{0.05cm}A_{\nu -2}) \cdot {\rm Pr}(A_{\nu -2} ) }{{\rm Pr}(B_{\nu}) } = \frac{5/12 \cdot 4/7 }{3/7 } | ||
= {5}/{9} \hspace{0.15cm}\underline {\approx 0.556}.$$ | = {5}/{9} \hspace{0.15cm}\underline {\approx 0.556}.$$ | ||
| − | '' | + | ''Reasoning:'' |
| − | * | + | *The probability ${\rm Pr}(B_{\nu}\hspace{0.05cm}|\hspace{0.05cm}A_{\nu-2})= 5/12$ has already been calculated in subsection '''(3)''' . |
| − | * | + | *Due to stationarity, ${\rm Pr}(A_{\nu-2})= {\rm Pr}(A) = 4/7$ and ${\rm Pr}(B_{\nu})= {\rm Pr}(B) = 3/7$ holds. |
| − | * | + | *Thus, the value of $5/9$ is obtained for the sought inference probability according to the above equation.. |
| − | '''(5)''' | + | '''(5)''' According to subtask '''(2)''' , with ${p =1/2}$ for the probability of $A$ in general: |
:$${\rm Pr}(A) = \frac{1-q}{1.5 -q}.$$ | :$${\rm Pr}(A) = \frac{1-q}{1.5 -q}.$$ | ||
| − | * | + | *Thus from $ {\rm Pr}(A) = 2/3$ follows $\underline{q =0}$. |
| − | '''(6)''' | + | '''(6)''' In the case of statistical independence, for example, it must hold: |
:$${{\rm Pr}(A \hspace{0.05cm} | \hspace{0.05cm}A)} = {{\rm Pr}(A \hspace{0.05cm} | \hspace{0.05cm}B)} = {{\rm Pr}(A)}.$$ | :$${{\rm Pr}(A \hspace{0.05cm} | \hspace{0.05cm}A)} = {{\rm Pr}(A \hspace{0.05cm} | \hspace{0.05cm}B)} = {{\rm Pr}(A)}.$$ | ||
| − | * | + | *From this follows $p = {\rm Pr}(A) \hspace{0.15cm}\underline {= 2/3}$ and accordingly $q = 1-p \hspace{0.15cm}\underline {= 1/3}$. |
{{ML-Fuß}} | {{ML-Fuß}} | ||
Revision as of 15:08, 29 November 2021
We consider a homogeneous stationary first-order Markov chain with events $A$ and $B$ and transition probabilities corresponding to the adjacent Markov diagram:
For subtasks (1) to (4) , assume:
- Event $A$ is followed by $A$ and $B$ with equal probability.
- After $B$ , event $A$ is twice as likely as $B$.
From subtask (5) on, $p$ and $q$ are free parameters, while the event probabilities ${\rm Pr}(A) = 2/3$ and ${\rm Pr}(B) = 1/3$ are fixed.
Hints:
- The exercise belongs to the chapter Markov Chains.
- You can check your results with the interactive applet Event probabilities of a 1st order Markov chain .
Questions
Solution
(1) According to the instruction, $p = 1 - p$ ⇒ $\underline{p =0.500}$ and $q = (1 - q)/2$, ⇒ $\underline{q =0.333}$ holds.
(2) For the event probability of $A$ holds:
- $${\rm Pr}(A) = \frac{{\rm Pr}(A \hspace{0.05cm} | \hspace{0.05cm}B)}{{\rm Pr}(A \hspace{0.05cm} | \hspace{0.05cm}B)+{\rm Pr}(B \hspace{0.05cm} | \hspace{0.05cm}A)} = \frac{1-q}{1-q+1-p} = \frac{2/3}{2/3 + 1/2}= \frac{4}{7} \hspace{0.15cm}\underline {\approx0.571}.$$
- This gives ${\rm Pr}(B)= 1 - {\rm Pr}(A) = 3/7 \hspace{0.15cm}\underline {\approx 0.429}$.
(3) No statement is made about the time $\nu-1$ .
- At this time $A$ or $B$ may have occurred. Therefore holds:
- $${\rm Pr}(B_{\nu} \hspace{0.05cm} | \hspace{0.05cm}A_{\nu -2}) = {\rm Pr}(A \hspace{0.05cm} | \hspace{0.05cm}A) \hspace{0.05cm} \cdot \hspace{0.05cm}{\rm Pr}(B \hspace{0.05cm} | \hspace{0.05cm}A) \hspace{0.15cm} +\hspace{0.15cm} {\rm Pr}(B \hspace{0.05cm} | \hspace{0.05cm}A) \hspace{0.05cm} \cdot \hspace{0.05cm}{\rm Pr}(B \hspace{0.05cm} | \hspace{0.05cm}B) = p \hspace{0.1cm} \cdot \hspace{0.1cm} (1-p) + q \hspace{0.1cm} \cdot \hspace{0.1cm} (1-p) = {5}/{12} \hspace{0.15cm}\underline {\approx 0.417}.$$
(4) According to Bayes' theorem:
- $${\rm Pr}(A_{\nu -2} \hspace{0.05cm} | \hspace{0.05cm}B_{\nu}) = \frac{{\rm Pr}(B_{\nu} \hspace{0.05cm} | \hspace{0.05cm}A_{\nu -2}) \cdot {\rm Pr}(A_{\nu -2} ) }{{\rm Pr}(B_{\nu}) } = \frac{5/12 \cdot 4/7 }{3/7 } = {5}/{9} \hspace{0.15cm}\underline {\approx 0.556}.$$
Reasoning:
- The probability ${\rm Pr}(B_{\nu}\hspace{0.05cm}|\hspace{0.05cm}A_{\nu-2})= 5/12$ has already been calculated in subsection (3) .
- Due to stationarity, ${\rm Pr}(A_{\nu-2})= {\rm Pr}(A) = 4/7$ and ${\rm Pr}(B_{\nu})= {\rm Pr}(B) = 3/7$ holds.
- Thus, the value of $5/9$ is obtained for the sought inference probability according to the above equation..
(5) According to subtask (2) , with ${p =1/2}$ for the probability of $A$ in general:
- $${\rm Pr}(A) = \frac{1-q}{1.5 -q}.$$
- Thus from $ {\rm Pr}(A) = 2/3$ follows $\underline{q =0}$.
(6) In the case of statistical independence, for example, it must hold:
- $${{\rm Pr}(A \hspace{0.05cm} | \hspace{0.05cm}A)} = {{\rm Pr}(A \hspace{0.05cm} | \hspace{0.05cm}B)} = {{\rm Pr}(A)}.$$
- From this follows $p = {\rm Pr}(A) \hspace{0.15cm}\underline {= 2/3}$ and accordingly $q = 1-p \hspace{0.15cm}\underline {= 1/3}$.