Aufgaben:Exercise 2.1Z: 2D-Frequency and 2D-Time Representations

• Return to book

2D–Übertragungsfunktion, als Realteil und Imaginärteil

To describe a time-variant channel with several paths, the two-dimensional impulse response is used $$h(\tau,\hspace{0.05cm}t) = \sum_{m = 1}^{M} z_m(t) \cdot {\rm \delta} (\tau - \tau_m)\hspace{0.05cm}.$$

The first parameter  $(\tau)$  indicates the delay time, the second  $(t)$  makes statements about the time variance.

The Fourier transformation of  $h(\tau, t)$  finally leads to the time-variant transfer function

$$H(f,\hspace{0.05cm} t) \hspace{0.2cm} \stackrel {f,\hspace{0.05cm}{\a6}{\bullet}{\bullet\!-\!-\!-\!-\!-\!\circ} \hspace{0.2cm} h(\tau,\hspace{0.05cm}t) \hspace{0.05cm}.$$

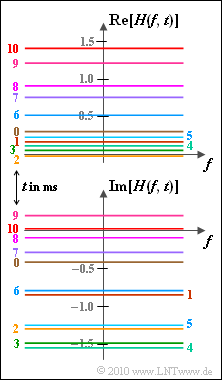
In the graph,  $H(f, t)$  is displayed as a function of frequency, for different values of absolute time  $t$  in the range of $0 \ \text{...} \ 10 \ \rm ms$.

In general,  $H(f, t)$  is complex. The real part (top) and the imaginary part (bottom) are drawn separately.

Notes:

• This task belongs to the topic of the chapter  Allgemeine Beschreibung zeitvarianter Systeme.
• In the above equation, an echo-free channel is represented with the parameter  $M = 1$ .
• Here are some numerical values of the specified time-variant transfer function:

$$H(f,\hspace{0.05cm} t \hspace{-0.1cm} \ = \ \hspace{-0.1cm} 0\, {\rm ms}) \approx 0.3 - {\rm j} \cdot 0.4 \hspace{0.05cm},\hspace{0.2cm} H(f,\hspace{0.05cm} t = 2\, {\rm ms}) \approx 0.0 - {\rm j} \cdot 1.3 \hspace{0.05cm},$$ $$H(f,\hspace{0.05cm} t \hspace{-0.1cm} \ = \ \hspace{-0.1cm} 4\, {\rm ms}) \approx 0.1 - {\rm j} \cdot 1.5 \hspace{0.05cm},\hspace{0.2cm} H(f,\hspace{0.05cm} t = 6\, {\rm ms}) \approx 0.5 - {\rm j} \cdot 0.8 \hspace{0.05cm},$$ $$H(f,\hspace{0.05cm} t \hspace{-0.1cm} \ = \ \hspace{-0.1cm} 8\, {\rm ms}) \approx 0.9 - {\rm j} \cdot 0.1 \hspace{0.05cm},\hspace{0.2cm} H(f,\hspace{0.05cm} t = 10\, {\rm ms}) \approx 1.4 \hspace{0.05cm}.$$

• As can already be guessed from the above graphic, neither the real nor the imaginary part of the 2D–transfer function  $H(f, t)$  are free of mean values.

=Questionnaire

1

Is there a time variant channel here?

	Yes.
	No.

2

Are there echoes on this channel?

	Yes.
	No.

3

How can the 2D–impulse response be described here?

	$h(\tau, t) = A \cdot \delta(\tau) + B \cdot \delta(\tau \, –5 \, \rm µ s)$.
	$h(\tau, t) = A \cdot \delta(\tau)$.
	$h(\tau, t) = z(t) \cdot \delta(\tau)$.

4

Estimate which channel the data was recorded for.

	AWGN channel,
	Two-way channel,
	Rayleigh channel,
	Rice channel.

Sample solution

{

Solution

(1)  As can be seen in the graph, the transfer function $H(f, t)$ is dependent on $t$. Thus $h(\tau, t)$ is also time-dependent. Correct is therefore YES.

(2)  If we look at a fixed point in time, for example $t = 2 \ \rm ms$, we obtain the following for the time-variant transfer function $$H(f,\hspace{0.05cm} t = 2\, {\rm ms}) = - {\rm j} \cdot 1.3 \hspace{0.05cm} = {\rm const.}$$

Thus the corresponding 2D–impulse response is $$h(\tau,\hspace{0.05cm} t = 2\, {\rm ms}) = - {\rm j} \cdot 1.3 \cdot \delta (\tau) \hspace{0.05cm} \hspace{0.3cm}\Rightarrow \hspace{0.3cm} M = 1 \hspace{0.05cm}.$$

With a path, however, multipath propagation cannot occur. This means that the correct solution is no.

(3)  The correct solution is solution 3:

• There is time variance but no frequency selectivity.
• Proposals 1 and 2, on the other hand, describe time-invariant systems.

(4)  Correct is the solution 4:

• For the AWGN–channel no transfer function can be specified.
• For a two-way channel, $H(f, t)$ is at no time $t$ constant.
• Since in the $H(f, t)$–graph in real– and imaginary part in each case an equal part not equal to zero can be recognized, the Rayleigh–channel can also be excluded.
• The data for the present task comes from a Rice–Channel with the following parameters:

$$\sigma = {1}/{\sqrt{2}} \hspace{0.05cm},\hspace{0.2cm} x_0 = {1}/{\sqrt{2}} \hspace{0.05cm},\hspace{0.2cm}y_0 = -{1}/{\sqrt{2}} \hspace{0.05cm},\hspace{0.2cm} f_{\rm D,\hspace{0.05cm} max} = 100\,\,{\rm Hz}\hspace{0.05cm}.$