Exercise 2.3Z: Polynomial Division

Multiplication and division of polynomials in $\rm GF(2)$

In this exercise we deal with the multiplication and especially the division of polynomials in the Galois field $\rm GF(2)$. In the figure the procedure is indicated by a simple and (hopefully) self-explanatory example:

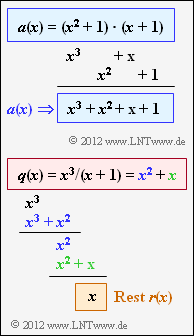
Multiplying the two polynomials $x^2 + 1$ and $x +1$ yields the result $a(x) = x^3 + x^2 + x + 1$.
Dividing the polynomial $b(x) = x^3$ by $p(x) = x + 1$ gives the quotient $q(x) = x^2 + x$ and the remainder $r(x) = x$.
One can check the latter result as follows:

$$b(x) \hspace{-0.15cm} \ = \ \hspace{-0.15cm} p(x) \cdot q(x) + r(x)\hspace{0.05cm}= \big[(x+1) \cdot (x^2+x)\big] +x =\big[x^3+ x^2+x^2+ x\big] +x = x^3\hspace{0.05cm}.$$

Hint:

This exercise belongs to the chapter "Extension field".

Fragebogen

Musterlösung

Solution

(1) Die Modulo–2–Multiplikation der beiden Polynome führt zum Ergebnis

$$a(x) \hspace{-0.15cm} \ = \ \hspace{-0.15cm} (x^3+ x+1) \cdot (x^2+1)= x^5+x^3+ x^2+ x^3+x+1 = x^5+ x^2+x+1\hspace{0.05cm}.$$

Richtig ist somit der Lösungsvorschlag 2.
Der letzte Lösungsvorschlag kann schon alleine deshalb nicht simmen, da der Grad des Produktpolynoms ungleich $5$ wäre.

Beispiel 1 zur Polynomdivision

(2) Mit den Abkürzungen

$$a(x) = x^5+ x^2+x+1\hspace{0.05cm},\hspace{0.4cm}p(x) = x^3+ x+1\hspace{0.05cm},\hspace{0.4cm}q(x) = x^2+ 1$$

und dem Ergebnis aus der Teilaufgabe (1) erhält man $a(x) = p(x) \cdot q(x)$.

Das heißt: Die Divisionen $a(x)/p(x)$ und $a(x)/q(x)$ sind restfrei möglich ⇒ Richtig sind die Lösungsvorschläge 1 und 2.

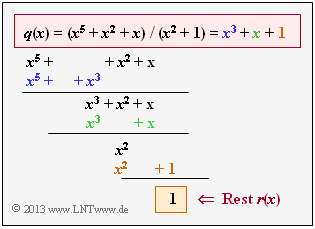
Auch ohne Rechnung erkennt man, dass $a(x)/x^2$ einen Rest ergeben muss. Nach Rechnung ergibt sich explizit:

$$(x^5 + x^2+x+1)/(x^2) = x^3 + 1 \hspace{0.05cm},\hspace{0.4cm}{\rm Rest}\hspace{0.15cm} r(x) = x+1\hspace{0.05cm}.$$

Zum letzten Lösungsvorschlag. Wir verwenden zur Abkürzung $b(x) = x^5 + x^2 + x = a(x) + 1$. Damit ist der vorgegebene Quotient:

$$b(x)/q(x) = a(x)/q(x) + 1/q(x) \hspace{0.05cm}.$$

Beispiel 2 zur Polynomdivision

Der erste Quotient $a(x)/q(x)$ ergibt entsprechend der Teilaufgabe (2) genau $p(x)$ ohne Rest, der zweite Quotient $0$ mit Rest $1$.
Somit ist hier der Rest des Quotienten $b(x)/q(x)$ gleich $r(x) = 1$, wie auch die Rechnung im Beispiel 1 zeigt.

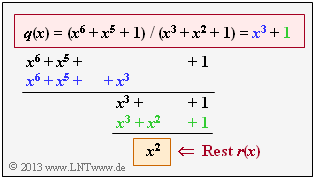
(3) Die Polynomdivision ist im Beispiel 2 ausführlich erläutert. Richtig ist der Lösungsvorschlag 3.

	$(x^5 + x^2 + x + 1)/(x^3 + x + 1)$.
	$(x^5 + x^2 + x + 1)/(x^2 + 1)$,
	$(x^5 + x^2 + x + 1)/(x^2)$,
	$(x^5 + x^2 + x)/(x^2 + 1)$.

	$a(x) = x^5 + x^3 + x^2 + 1$,
	$a(x) = x^5 + x^2 + x + 1$.
	$a(x) = x^6 + x^3 + x^2 + 1$-

	$q(x) = x^3 + x^2 + 1, \hspace{0.2cm} r(x) = 0$,
	$q(x) = x^3 + 1, \hspace{0.2cm} r(x) = 0$,
	$q(x) = x^3 + 1, \hspace{0.2cm} r(x) = x^2$.