Difference between revisions of "Aufgaben:Exercise 2.4: Number Lottery (6 from 49)"
| Line 6: | Line 6: | ||
For the number lotto the following conditions should apply: | For the number lotto the following conditions should apply: | ||
| − | Six winning numbers are drawn from the $49$ numbers (balls) in a drum ( | + | Six winning numbers are drawn from the $49$ numbers (balls) in a drum ("6 out of 49"), then as the seventh ball the so-called additional number $(Z)$. |
| − | + | Independently of this, a super number $S \in \{0, \ 1,\hspace{0.1cm} \text{...} \hspace{0.1cm}, \ 9\}$ is selected at random. If this number matches the final digit of the lottery ticket, the main prize is increased decisively. | |
| − | In | + | In this task, the following winning classes are considered: |
| − | :* 6 | + | :* 6 correct numbers with a super number |
| − | :* 6 | + | :* 6 correct numbers without a super number |
| − | :* 5 | + | :* 5 correct numbers with a super number |
| − | :* 5 | + | :* 5 correct numbers without a super number |
| − | :* 4 | + | :* 4 correct numbers |
| − | :* 3 | + | :* 3 correct numbers |
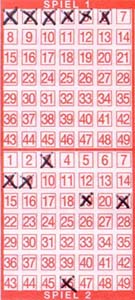
| − | + | For subtasks '''(1)''' to '''(6)''' , start from the upper lottery ticket: The player has ticked the numbers "1" to "6" here. | |
| Line 29: | Line 29: | ||
| + | Hints: | ||
| + | *The exercise belongs to the chapter [[Theory_of_Stochastic_Signals/Binomial_Distribution|Binomial Distribution]]. | ||
| + | |||
| + | *This exercise was designed around 2002. It is possible that the rules of the lottery have been changed in the meantime. | ||
| − | |||
| − | |||
| − | |||
| − | |||
| − | === | + | ===Questions=== |
<quiz display=simple> | <quiz display=simple> | ||
| − | { | + | {What is the probability for $\text{6 correct numbers}$ ? |
|type="{}"} | |type="{}"} | ||
| − | $ \rm Pr(6\ | + | $ \rm Pr(6\hspace{0.1cm}correct\hspace{0.1cm}numbers) \ = \ $ { 71.5 3% } $\ \cdot 10^{-9}$ |
| − | { | + | {What is the probability for $\text{6 correct numbers plus super number}$ ? |
|type="{}"} | |type="{}"} | ||
$ \rm Pr(6R + S) \ = \ $ { 7.15 3% } $\ \cdot 10^{-9}$ | $ \rm Pr(6R + S) \ = \ $ { 7.15 3% } $\ \cdot 10^{-9}$ | ||
| − | { | + | {What is the probability for $\text{5 correct numbers plus additional number}$ ? |
|type="{}"} | |type="{}"} | ||
$ \rm Pr(5R + Z) \ = \ $ { 0.429 3% } $\ \cdot 10^{-6}$ | $ \rm Pr(5R + Z) \ = \ $ { 0.429 3% } $\ \cdot 10^{-6}$ | ||
| − | { | + | {What is the probability for $\text{5 correct numbers without additional number}$ ? |
|type="{}"} | |type="{}"} | ||
| − | $\rm Pr(5\ | + | $\rm Pr(5\hspace{0.1cm}correct\hspace{0.1cm}numbers)\ = \ $ { 18.1 3% } $\ \cdot 10^{-6}$ |
| − | { | + | {What is the probability for $\text{4 correct numbers}$ ? What is the general result for $k$ correct numbers in the $m–\text{out of}–n$–lotto ? |
|type="{}"} | |type="{}"} | ||
| − | $\rm Pr(4\ | + | $\rm Pr(4\hspace{0.1cm}correct\hspace{0.1cm}numbers)\ = \ $ { 0.969 3% } $\ \cdot 10^{-3}$ |
| − | { | + | {What is the probability for $\text{3 correct numbers}$ ? |
|type="{}"} | |type="{}"} | ||
| − | $\rm Pr(3\ | + | $\rm Pr(3\hspace{0.1cm}correct\hspace{0.1cm}numbers)\ = \ $ { 18.1 3% } $\ \cdot 10^{-3}$ |
| − | { | + | {Consider the odds of winning the bottom lottery ticket with the numbers $3, \ 8, \ 9, \ 19, \ 21, \ 46$. Which of the statements are true? |
|type="[]"} | |type="[]"} | ||
| − | + | + | + The probabilities calculated so far still apply. |
| − | + | + | + He would very likely receive a larger winning sum with $\text{6 correct numbers}$ than with the guess $123456$. |
</quiz> | </quiz> | ||
| − | === | + | ===Solution=== |
{{ML-Kopf}} | {{ML-Kopf}} | ||
| − | '''(1)''' | + | '''(1)''' Assuming that the player has ticked the numbers „1“ to „6“ , the following applies: |
| − | :$${\rm \Pr}(6\hspace{0.1cm}{\rm | + | :$${\rm \Pr}(6\hspace{0.1cm}{\rm correct\hspace{0.1cm}numbers})={\rm \Pr}(123456\ \cup 123465\cup \ \text{...} \ \ \cup 654321).$$ |
| − | * | + | *This takes into account that the order does not matter in the drawing. |
| − | * | + | *In total, there are exactly $6! = 1 · 2 · 3 · 4 · 5 · 6$ gequally probable permutations for the set of numbers: |
| − | :$$\rm \Pr(6\hspace{0.1cm} | + | :$$\rm \Pr(6\hspace{0.1cm}correct\hspace{0.1cm}numbers)=6!\cdot Pr(123456).$$ |
| − | * | + | *The probability of the number „1“ being drawn as the first ball is $1/49$. The probability of „2“ being drawn as the second ball is correspondingly $1/48$ (since there is now one less ball in the drum). Thus one receives as final result: |
:$$\rm Pr(123456)=\frac{1}{49}\cdot\frac{1}{48}\cdot \frac{1}{47} \cdot \frac{1}{46}\cdot \frac{1}{45} \cdot \frac{1}{44},$$ | :$$\rm Pr(123456)=\frac{1}{49}\cdot\frac{1}{48}\cdot \frac{1}{47} \cdot \frac{1}{46}\cdot \frac{1}{45} \cdot \frac{1}{44},$$ | ||
| − | :$$\rm Pr(6\hspace{0.1cm} | + | :$$\rm Pr(6\hspace{0.1cm}correct\hspace{0.1cm}numbers)=\frac{1\cdot 2\cdot 3 \cdot 4 \cdot 5 \cdot 6}{49\cdot 48 \cdot 47 \cdot 46 \cdot 45 \cdot 44}.$$ |
| − | * | + | *This is exactly the inverse of „49 over 6“. It follows that: |
| − | :$$\rm Pr(6\hspace{0.15cm} | + | :$$\rm Pr(6\hspace{0.15cm}correct\hspace{0.1cm}numbers)=\left({49 \atop {6}}\right)^{-1}=13\hspace{0.08cm}983\hspace{0.08cm}816^{-1}\hspace{0.15cm} \underline{\approx71.5\cdot 10^{-9}}.$$ |
| − | '''(2)''' | + | '''(2)''' The probability that the final digit of a lottery ticket matches the super number is $10\%$. |
| − | * | + | *But since the drawing of the super number is independent of the normal drawing, we now get for the probability we are looking for |
:$$\text{ Pr(6R + S)} \hspace{0.15cm}\underline{\approx \ 7.15\cdot 10^{-9}}.$$ | :$$\text{ Pr(6R + S)} \hspace{0.15cm}\underline{\approx \ 7.15\cdot 10^{-9}}.$$ | ||
| − | '''(3)''' | + | '''(3)''' In what follows, $Z$ stands for "additional number". Then holds: |
| − | :$$\rm Pr(5\hspace{0.15cm} | + | :$$\rm Pr(5\hspace{0.15cm}correct\hspace{0.1cm}numbers\hspace{0.1cm}plus\hspace{0.1cm}additional\hspace{0.1cm}number)={\rm Pr(12345} Z \ \cup \ {\rm 1234} Z\rm 6\cup \ \text{...} \ \cup \ Z {\rm 23456)}.$$ |
| − | * | + | *There are six permutations here. The probability for $\rm 12345Z$ is the same as for $123456$. It follows with the result of subtask '''(1)''': |
| − | :$$\rm Pr(5\hspace{0.15cm} | + | :$$\rm Pr(5\hspace{0.15cm}correct\hspace{0.1cm}numbers\hspace{0.1cm}plus\hspace{0.1cm}additional\hspace{0.1cm}number)=6\cdot\left({49 \atop {6}}\right)^{-1}\hspace{0.15cm} \underline{=0.429\cdot10^{-6}}.$$ |
| − | '''(4)''' | + | '''(4)''' If we denote by $X$ a drawn number that is not one of those checked, we can write for the probability we are looking for: |
| − | :$${\rm \Pr(5\hspace{0.1cm} | + | :$${\rm \Pr(5\hspace{0.1cm}correct\hspace{0.1cm}numbers)=Pr(12345} X \ \cup \ {\rm 1234} X6\ \cup \ \text{...} \ \cup \ X23456).$$ |
| − | * | + | *There are six different possibilities for the location of $X$ , all of which are equally probable. It follows that: |
| − | :$$\rm Pr(5\hspace{0.15cm} | + | :$$\rm Pr(5\hspace{0.15cm}correct\hspace{0.1cm}numbers)=6\cdot Pr(12345\it X\rm ).$$ |
*$X$ kann hierbei für jede der Zahlen $7$ bis $49$ stehen, die wiederum alle gleichwahrscheinlich sind. | *$X$ kann hierbei für jede der Zahlen $7$ bis $49$ stehen, die wiederum alle gleichwahrscheinlich sind. | ||
*Mit $\rm Pr(123457) = Pr(123456)$, dem Ergebnis der Teilaufgabe '''(1)''', erhält man somit: | *Mit $\rm Pr(123457) = Pr(123456)$, dem Ergebnis der Teilaufgabe '''(1)''', erhält man somit: | ||
| − | :$$\rm Pr(5\hspace{0.1cm} | + | :$$\rm Pr(5\hspace{0.1cm}correct\hspace{0.1cm}numbers)=6\cdot 43\cdot\left({49 \atop {6}}\right)^{-1}=1.85\cdot10^{-5}.$$ |
*Zu subtrahieren ist noch der Fall, dass auch die Zusatzzahl richtig gezogen wurde. Deshalb gilt: | *Zu subtrahieren ist noch der Fall, dass auch die Zusatzzahl richtig gezogen wurde. Deshalb gilt: | ||
| − | :$$\rm \Pr(5\hspace{0.15cm} | + | :$$\rm \Pr(5\hspace{0.15cm}correct\hspace{0.1cm}numbers\hspace{0.1cm}without\hspace{0.1cm}additional\hspace{0.1cm}number)\approx 18.5\cdot 10^{-6}-0.429\cdot 10^{-6}\hspace{0.15cm} \underline{\approx 18.1\cdot 10^{-6}}.$$ |
'''(5)''' Nach einigen Überlegungen kommt man zum Ergebnis: | '''(5)''' Nach einigen Überlegungen kommt man zum Ergebnis: | ||
| − | :$$\rm Pr(\it k\hspace{0.15cm} \rm | + | :$$\rm Pr(\it k\hspace{0.15cm} \rm correct\hspace{0.1cm}numbers)=\left({\it m \atop {\it k}}\right)\cdot\left({\it n-m \atop {m-\it k}}\right)\cdot\left({\it n \atop {m}}\right)^{-1}.$$ |
*Der letzte Term in dieser Gleichung gibt die Wahrscheinlichkeit für eine ganz bestimmte "$m$ aus $n$"–Kombination an. | *Der letzte Term in dieser Gleichung gibt die Wahrscheinlichkeit für eine ganz bestimmte "$m$ aus $n$"–Kombination an. | ||
| Line 134: | Line 134: | ||
*Als Sonderfall erhalten wir beim „6 aus 49“ mit $k= 4$: | *Als Sonderfall erhalten wir beim „6 aus 49“ mit $k= 4$: | ||
| − | :$$\rm Pr(4\hspace{0.15cm} | + | :$$\rm Pr(4\hspace{0.15cm}correct\hspace{0.1cm}numbers)=\left({6 \atop {4}}\right)\cdot\left({43 \atop {2}}\right)\cdot\left({49 \atop {6}}\right)^{-1}\hspace{0.15cm} \underline{=0.969\cdot10^{-3}}.$$ |
'''(6)''' Entsprechend der unter '''(5)''' berechneten allgemeinen Formel gilt weiter: | '''(6)''' Entsprechend der unter '''(5)''' berechneten allgemeinen Formel gilt weiter: | ||
| − | :$$\rm Pr(3\hspace{0.15cm} | + | :$$\rm Pr(3\hspace{0.15cm}correct\hspace{0.1cm}numbers)=\left({6 \atop {3}}\right)\cdot\left({43 \atop {3}}\right)\cdot\left({49 \atop {6}}\right)^{-1}\hspace{0.15cm} \underline{=18.1\cdot10^{-3}}.$$ |
Revision as of 18:33, 12 December 2021
For the number lotto the following conditions should apply:
Six winning numbers are drawn from the $49$ numbers (balls) in a drum ("6 out of 49"), then as the seventh ball the so-called additional number $(Z)$.
Independently of this, a super number $S \in \{0, \ 1,\hspace{0.1cm} \text{...} \hspace{0.1cm}, \ 9\}$ is selected at random. If this number matches the final digit of the lottery ticket, the main prize is increased decisively.
In this task, the following winning classes are considered:
- 6 correct numbers with a super number
- 6 correct numbers without a super number
- 5 correct numbers with a super number
- 5 correct numbers without a super number
- 4 correct numbers
- 3 correct numbers
For subtasks (1) to (6) , start from the upper lottery ticket: The player has ticked the numbers "1" to "6" here.
Hints:
- The exercise belongs to the chapter Binomial Distribution.
- This exercise was designed around 2002. It is possible that the rules of the lottery have been changed in the meantime.
Questions
Solution
- $${\rm \Pr}(6\hspace{0.1cm}{\rm correct\hspace{0.1cm}numbers})={\rm \Pr}(123456\ \cup 123465\cup \ \text{...} \ \ \cup 654321).$$
- This takes into account that the order does not matter in the drawing.
- In total, there are exactly $6! = 1 · 2 · 3 · 4 · 5 · 6$ gequally probable permutations for the set of numbers:
- $$\rm \Pr(6\hspace{0.1cm}correct\hspace{0.1cm}numbers)=6!\cdot Pr(123456).$$
- The probability of the number „1“ being drawn as the first ball is $1/49$. The probability of „2“ being drawn as the second ball is correspondingly $1/48$ (since there is now one less ball in the drum). Thus one receives as final result:
- $$\rm Pr(123456)=\frac{1}{49}\cdot\frac{1}{48}\cdot \frac{1}{47} \cdot \frac{1}{46}\cdot \frac{1}{45} \cdot \frac{1}{44},$$
- $$\rm Pr(6\hspace{0.1cm}correct\hspace{0.1cm}numbers)=\frac{1\cdot 2\cdot 3 \cdot 4 \cdot 5 \cdot 6}{49\cdot 48 \cdot 47 \cdot 46 \cdot 45 \cdot 44}.$$
- This is exactly the inverse of „49 over 6“. It follows that:
- $$\rm Pr(6\hspace{0.15cm}correct\hspace{0.1cm}numbers)=\left({49 \atop {6}}\right)^{-1}=13\hspace{0.08cm}983\hspace{0.08cm}816^{-1}\hspace{0.15cm} \underline{\approx71.5\cdot 10^{-9}}.$$
(2) The probability that the final digit of a lottery ticket matches the super number is $10\%$.
- But since the drawing of the super number is independent of the normal drawing, we now get for the probability we are looking for
- $$\text{ Pr(6R + S)} \hspace{0.15cm}\underline{\approx \ 7.15\cdot 10^{-9}}.$$
(3) In what follows, $Z$ stands for "additional number". Then holds:
- $$\rm Pr(5\hspace{0.15cm}correct\hspace{0.1cm}numbers\hspace{0.1cm}plus\hspace{0.1cm}additional\hspace{0.1cm}number)={\rm Pr(12345} Z \ \cup \ {\rm 1234} Z\rm 6\cup \ \text{...} \ \cup \ Z {\rm 23456)}.$$
- There are six permutations here. The probability for $\rm 12345Z$ is the same as for $123456$. It follows with the result of subtask (1):
- $$\rm Pr(5\hspace{0.15cm}correct\hspace{0.1cm}numbers\hspace{0.1cm}plus\hspace{0.1cm}additional\hspace{0.1cm}number)=6\cdot\left({49 \atop {6}}\right)^{-1}\hspace{0.15cm} \underline{=0.429\cdot10^{-6}}.$$
(4) If we denote by $X$ a drawn number that is not one of those checked, we can write for the probability we are looking for:
- $${\rm \Pr(5\hspace{0.1cm}correct\hspace{0.1cm}numbers)=Pr(12345} X \ \cup \ {\rm 1234} X6\ \cup \ \text{...} \ \cup \ X23456).$$
- There are six different possibilities for the location of $X$ , all of which are equally probable. It follows that:
- $$\rm Pr(5\hspace{0.15cm}correct\hspace{0.1cm}numbers)=6\cdot Pr(12345\it X\rm ).$$
- $X$ kann hierbei für jede der Zahlen $7$ bis $49$ stehen, die wiederum alle gleichwahrscheinlich sind.
- Mit $\rm Pr(123457) = Pr(123456)$, dem Ergebnis der Teilaufgabe (1), erhält man somit:
- $$\rm Pr(5\hspace{0.1cm}correct\hspace{0.1cm}numbers)=6\cdot 43\cdot\left({49 \atop {6}}\right)^{-1}=1.85\cdot10^{-5}.$$
- Zu subtrahieren ist noch der Fall, dass auch die Zusatzzahl richtig gezogen wurde. Deshalb gilt:
- $$\rm \Pr(5\hspace{0.15cm}correct\hspace{0.1cm}numbers\hspace{0.1cm}without\hspace{0.1cm}additional\hspace{0.1cm}number)\approx 18.5\cdot 10^{-6}-0.429\cdot 10^{-6}\hspace{0.15cm} \underline{\approx 18.1\cdot 10^{-6}}.$$
(5) Nach einigen Überlegungen kommt man zum Ergebnis:
- $$\rm Pr(\it k\hspace{0.15cm} \rm correct\hspace{0.1cm}numbers)=\left({\it m \atop {\it k}}\right)\cdot\left({\it n-m \atop {m-\it k}}\right)\cdot\left({\it n \atop {m}}\right)^{-1}.$$
- Der letzte Term in dieser Gleichung gibt die Wahrscheinlichkeit für eine ganz bestimmte "$m$ aus $n$"–Kombination an.
- Der erste Term beschreibt die Anzahl der Permutationen, dass von $m$ gezogenen Zahlen genau $k$ richtig sind. Für $m = 6$ und $k= 5$ ergibt das den Faktor $6$.
- Der mittlere Faktor gibt die Anzahl der Möglichkeiten an, dass alle restlichen gezogenen Zahlen $($also $m-k)$ eine der ungünstigen Zahlen $($hierfür gibt es $n-m)$ ist. Für $m = 6$ und $k= 5$ ergibt dieser Term entsprechend Punkt (4) den Wert $43$.
- Als Sonderfall erhalten wir beim „6 aus 49“ mit $k= 4$:
- $$\rm Pr(4\hspace{0.15cm}correct\hspace{0.1cm}numbers)=\left({6 \atop {4}}\right)\cdot\left({43 \atop {2}}\right)\cdot\left({49 \atop {6}}\right)^{-1}\hspace{0.15cm} \underline{=0.969\cdot10^{-3}}.$$
(6) Entsprechend der unter (5) berechneten allgemeinen Formel gilt weiter:
- $$\rm Pr(3\hspace{0.15cm}correct\hspace{0.1cm}numbers)=\left({6 \atop {3}}\right)\cdot\left({43 \atop {3}}\right)\cdot\left({49 \atop {6}}\right)^{-1}\hspace{0.15cm} \underline{=18.1\cdot10^{-3}}.$$
(7) Beide Aussagen sind richtig:
- Die Wahrscheinlichkeiten sind natürlich gleich. Weil das viele wissen und diese ihr Wissen auch (zumindest sich selbst) demonstrieren wollen, spielen sehr viel mehr Menschen diese Kombination als eine eher unspektakuläre.
- Da aber der in einer Gewinnklasse auszuzahlende Betrag vorher festgelegt wird und dieser Gewinn dann durch eine größere Anzahl von Gewinnern zu teilen ist, bleibt für den Einzelnen natürlich weniger.
- Da andererseits viele Lottospieler auf ihr Geburtsdatum setzen, ist die hier gewählte Komination auch nicht so günstig. Die Zahlen „3“, „8“ und „9“ können sowohl den Tag als auch das Monat angeben und zudem beginnt fast bei allen Spielern das Geburtsjahr mit "19".