Difference between revisions of "Aufgaben:Exercise 2.6Z: Synchronous Demodulator"
(Die Seite wurde neu angelegt: „ {{quiz-Header|Buchseite=Lineare zeitinvariante Systeme/Lineare Verzerrungen }} right| :Das dargestellte Blockschaltbild…“) |
|||
| (53 intermediate revisions by 6 users not shown) | |||
| Line 1: | Line 1: | ||
| − | {{quiz-Header|Buchseite= | + | {{quiz-Header|Buchseite=Linear_and_Time_Invariant_Systems/Linear_Distortions |
}} | }} | ||
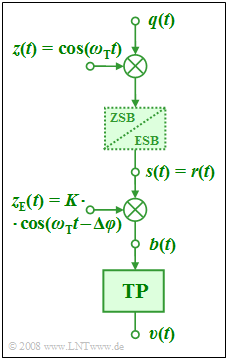
| − | [[File:P_ID913__LZI_Z_2_6_neu.png|right|]] | + | [[File:P_ID913__LZI_Z_2_6_neu.png|right|frame|Amplitude modulator (top), <br>synchronous demodulator]] |
| − | + | The depicted block diagram shows a transmission system | |
| + | *with [[Modulation_Methods/Double-Sideband_Amplitude_Modulation|Double-Sideband Amplitude Modulation]] $\rm(DSB\hspace{0.03cm}–\hspace{-0.1cm}AM)$ | ||
| + | *and [[Modulation_Methods/Synchronous_Demodulation|Synchronous Demodulation]] $\rm (SD)$. | ||
| + | |||
| + | |||
| + | Let the source signal consist of two harmonic oscillations with frequencies $f_2 = 2 \ \rm kHz$ and $f_5 = 5 \ \rm kHz$: | ||
:$$q(t) = {2 \, \rm V} \cdot {\rm cos}(\omega_2 t )+ {1 \, \rm V} | :$$q(t) = {2 \, \rm V} \cdot {\rm cos}(\omega_2 t )+ {1 \, \rm V} | ||
\cdot {\rm sin}(\omega_5 t ) .$$ | \cdot {\rm sin}(\omega_5 t ) .$$ | ||
| − | + | *This signal is multiplied by the dimensionless carrier signal $z(t) = \cos(\omega_{\rm T} \cdot T)$ of carrier frequency $f_{\rm T} = 50 \ \rm kHz$. <br>For DSB–AM, the dashed block is irrelevant so that the following holds for the transmission signal: | |
:$$s(t) = q(t) \cdot {\rm cos}(\omega_{\rm T} t ) .$$ | :$$s(t) = q(t) \cdot {\rm cos}(\omega_{\rm T} t ) .$$ | ||
| − | + | *In the synchronous demodulator, the received signal $r(t)$ – in an ideal channel identical to the signal $s(t)$ – is multiplied by the receive-site carrier signal $z_{\rm E}(t)$ where the following applies: | |
:$$z_{\rm E}(t) = K \cdot {\rm cos}(\omega_{\rm T} t - \Delta \varphi ) .$$ | :$$z_{\rm E}(t) = K \cdot {\rm cos}(\omega_{\rm T} t - \Delta \varphi ) .$$ | ||
| − | + | *This signal should not only be frequency-synchronous with $z(t)$ but also phase-synchronous ⇒ hence the name "synchronous demodulator". | |
| + | *The above approach takes into account a phase shift between $z(t)$ and $z_{\rm E}(t)$, which should ideally be $\Delta \varphi = 0$ but often cannot be avoided in real systems. | ||
| + | |||
| + | *The output signal $b(t)$ of the second multiplier includes components around twice the carrier frequency in addition to the desired low-frequency component. | ||
| + | *Using an ideal low-pass filter $\rm (LP)$ (e.g. with cut-off frequency $f_{\rm T}$) the sink signal $v(t)$, which ideally should be equal to the source signal $q(t)$, can be obtained. | ||
| − | |||
| − | + | For the transmitter, multiplication by the carrier signal $z(t)$ generally results in two sidebands. In [[Modulation_Methods/Single-Sideband_Modulation|Single-Sideband Modulation]] (ESB–AM), only one of the two bands is transmitted, for example the lower sideband (USB). Thus, the following is obtained with an ideal channel: | |
| − | :$$r(t) = s(t)= {1 \, \rm V} \cdot {\rm cos} | + | :$$r(t) = s(t)= {1 \, \rm V} \cdot {\rm cos}\big [(\omega_{\rm T} - |
| − | \omega_2 )t | + | \omega_2 )\cdot t \big ] - {0.5 \, \rm V} \cdot {\rm sin}\big [(\omega_{\rm T} - |
| − | \omega_5 )t | + | \omega_5 )\cdot t \big ] .$$ |
| − | + | *Here, synchronous demodulation results in the following distorted sink signal considering a phase shift $\Delta \varphi$, the constant $K = 4$ and the downstream low-pass filter: | |
| − | :$$v(t)= {1 \, \rm V} \cdot | + | :$$v(t)= {1 \, \rm V} \cdot {1}/{2}\cdot 4 \cdot{\rm cos}( |
\omega_2 t - \Delta \varphi)+ {0.5 \, \rm V} \cdot | \omega_2 t - \Delta \varphi)+ {0.5 \, \rm V} \cdot | ||
| − | + | {1}/{2}\cdot 4 \cdot{\rm sin}( \omega_5 t - \Delta \varphi)$$ | |
:$$\Rightarrow \hspace{0.5cm}v(t)= {2 \, \rm V} \cdot{\rm cos}( | :$$\Rightarrow \hspace{0.5cm}v(t)= {2 \, \rm V} \cdot{\rm cos}( | ||
\omega_2 t - \Delta \varphi)+ {1 \, \rm V} \cdot{\rm sin}( | \omega_2 t - \Delta \varphi)+ {1 \, \rm V} \cdot{\rm sin}( | ||
\omega_5 t - \Delta \varphi)$$ | \omega_5 t - \Delta \varphi)$$ | ||
| − | + | *In the ideal case of phase-synchronous demodulation $(\Delta \varphi = 0)$, $v(t) = q(t)$ holds again. | |
| − | + | ||
| − | |||
| − | : | + | |
| − | :$$\cos^2(\alpha) = | + | Please note: |
| − | + | *The exercise belongs to the chapter [[Linear_and_Time_Invariant_Systems/Linear_Distortions|Linear Distortions]]. | |
| − | + | *The topic "amplitude modulation/synchronous demodulator" is discussed in detail in the book [[Modulation_Methods]]. | |
| − | \beta)+ \cos(\alpha + \beta) \ | + | *The following trigonometric relationships are given: |
| − | + | :$$\cos^2(\alpha) = {1}/{2} \cdot \big [ 1 + | |
| + | \cos(2\alpha) \big ] \hspace{0.05cm}, $$ | ||
| + | :$$\cos(\alpha) \cdot \cos(\beta) = {1}/{2} \cdot \big[ \cos(\alpha - | ||
| + | \beta)+ \cos(\alpha + \beta) \big],$$ | ||
| + | :$$ \sin(\alpha) \cdot \cos(\beta) = {1}/{2} \cdot \big[ \sin(\alpha - | ||
\beta)+ \sin(\alpha + \beta) | \beta)+ \sin(\alpha + \beta) | ||
| − | \ | + | \big] \hspace{0.05cm}.$$ |
| − | + | *The signal designations result from the German original of this exercise. '''Here again as a listing''' $q(t)$ ⇒ source signal, $v(t)$ ⇒ sink signal, $z(t)$ ⇒ transmit-site carrier signal, $s(t)$ ⇒ transmission signal (BP), $r(t)$ ⇒ received signal (BP), $z_{\rm E}(t)$ ⇒ receive-site carrier signal, $b(t)$ ⇒ BP signal before low-pass. | |
| + | |||
| − | === | + | ===Questions=== |
<quiz display=simple> | <quiz display=simple> | ||
| − | { | + | {What is the sink signal $v(t)$ for DSB-AM and phase-synchronous modulation ⇒ $\Delta \varphi = 0$? <br>How should $K$ be chosen such that $v(t) = q(t)$ holds? |
| + | |type="{}"} | ||
| + | $K \ = \ $ { 2 3% } | ||
| + | |||
| + | |||
| + | {The following holds: $K = 2$. Specify the sink signal $v(t)$ considering a phase shift $\Delta \varphi$. Which of the following statements are true? | ||
|type="[]"} | |type="[]"} | ||
| − | - | + | - $v(t) = q(t)$ holds independently of $\Delta \varphi$ . |
| − | + | + | + $\Delta \varphi \ne 0$ results in frequency-independent attenuation. |
| + | - A phase shift $\Delta \varphi \ne 0$ results in attenuation distortions. | ||
| + | - A phase shift $\Delta \varphi \ne 0$ results in phase distortions. | ||
| + | + $v(t) = q(t)/2$ holds with $\Delta \varphi = \hspace{-0.05cm}-\hspace{0.05cm}60^\circ$. | ||
| + | |||
| + | {Which statements hold for synchronous demodulation of the SSB signal if a phase shift of $\Delta \varphi$ is considered? | ||
| + | |type="[]"} | ||
| + | - Regardless of $\Delta \varphi$, $v(t) = q(t)$ holds. | ||
| + | - $\Delta \varphi \ne 0$ results in frequency-independent attenuation. | ||
| + | - A phase shift $\Delta \varphi \ne 0$ results in attenuation distortions. | ||
| + | + A phase shift $\Delta \varphi \ne 0$ results in phase distortions. | ||
| + | - $v(t) = q(t)/2$ holds with $\Delta \varphi = \hspace{-0.05cm}-\hspace{0.05cm}60^\circ$ . | ||
| − | |||
| − | |||
| − | |||
| Line 64: | Line 89: | ||
</quiz> | </quiz> | ||
| − | === | + | ===Solution=== |
{{ML-Kopf}} | {{ML-Kopf}} | ||
| − | '''1 | + | '''(1)''' The following holds for the band-pass signal after the second multiplier: |
| − | '''2 | + | :$$b(t) = r(t) \cdot z_{\rm E}(t)= q(t) \cdot z(t) \cdot z_{\rm |
| − | '''3 | + | E}(t)= K \cdot q(t)\cdot |
| − | + | \cos^2(\omega_{\rm T} t).$$ | |
| − | + | ||
| − | + | *Using the trigonometric relation $\cos^2(\omega_{\rm T} t) = {1}/{2} \cdot\big[ 1 + | |
| − | + | \cos(2\omega_{\rm T} t)\big]$, one obtains: | |
| + | :$$b(t) = {K}/{2} \cdot q(t) + {K}/{2} \cdot q(t)\cdot | ||
| + | \cos(2\omega_{\rm T} t).$$ | ||
| + | |||
| + | *The second component is located at around twice the carrier frequency ⇒ $2 f_{\rm T}$. | ||
| + | *This is removed by the low-pass filter $($with the cut-off frequency $ f_{\rm G} = f_{\rm T})$ . | ||
| + | *Hence, the following is obtained: $v(t) = {K}/{2} \cdot q(t) .$ | ||
| + | *An ideal demodulation ⇒ $v(t) = q(t)$ is obtained with $\underline {K = 2}$ . | ||
| + | |||
| + | |||
| + | |||
| + | '''(2)''' Considering the relation | ||
| + | :$$\cos(\omega_{\rm T} t) \cdot \cos(\omega_{\rm T} t - \Delta \varphi) = {1}/{2} \cdot | ||
| + | \big[ \cos(\Delta \varphi)+ \cos(2\omega_{\rm T} t - \Delta \varphi) \big]$$ | ||
| + | |||
| + | and the downstream low-pass filter, which removes the component at around twice the carrier frequency, the following is obtained here with $ {K = 2}$: | ||
| + | :$$v(t) = q(t) \cdot \cos(\Delta \varphi).$$ | ||
| + | |||
| + | <u>Proposed solutions 2 and 5</u> are correct: | ||
| + | *A phase shift $\Delta \varphi$ only results in frequency-independent attenuation and not in attenuation distortions or phase distortions. | ||
| + | *A phase shift by $\varphi =\pm 60^\circ$ results in halving of the signal amplitude. | ||
| + | |||
| + | |||
| + | |||
| + | '''(3)''' Here, <u>proposed solution 4</u> is correct. | ||
| + | *Exactly the same phase shift $\Delta \varphi$ occurs for both summands, and phase distortions occur here: | ||
| + | :$$v(t)= {2 \, \rm V} \cdot{\rm cos}\big[ \omega_2 \cdot (t - \tau_2) \big]+ | ||
| + | {1 \, \rm V} \cdot{\rm sin}\big[ \omega_5 t \cdot (t - \tau_5)\big],$$ | ||
| + | :$${\rm where}\hspace{0.5cm}\tau_2 = \frac{\Delta \varphi}{\omega_2} | ||
| + | \hspace{0.5cm}\ne \hspace{0.5cm} \tau_5 = \frac{\Delta | ||
| + | \varphi}{\omega_5}.$$ | ||
| + | |||
| + | *A phase shift of $\varphi =60^\circ$ corresponding to $\pi/3$ leads to the following delay times here: | ||
| + | :$$\tau_2 = \frac{\pi/3}{2 \pi \cdot 2\,\,{\rm kHz }} \approx | ||
| + | 83.3\,{\rm µ s }, \hspace{0.5cm} | ||
| + | \tau_5 = \frac{\pi/3}{2 \pi \cdot 5\,\,{\rm kHz }} \approx | ||
| + | 33.3\,{\rm µ s }.$$ | ||
| + | |||
| + | *The lower-frequency signal is thus delayed more. | ||
{{ML-Fuß}} | {{ML-Fuß}} | ||
| − | [[Category: | + | [[Category:Linear and Time-Invariant Systems: Exercises|^2.3 Linear Distortions^]] |
Latest revision as of 15:29, 6 October 2021
The depicted block diagram shows a transmission system
- with Double-Sideband Amplitude Modulation $\rm(DSB\hspace{0.03cm}–\hspace{-0.1cm}AM)$
- and Synchronous Demodulation $\rm (SD)$.
Let the source signal consist of two harmonic oscillations with frequencies $f_2 = 2 \ \rm kHz$ and $f_5 = 5 \ \rm kHz$:
- $$q(t) = {2 \, \rm V} \cdot {\rm cos}(\omega_2 t )+ {1 \, \rm V} \cdot {\rm sin}(\omega_5 t ) .$$
- This signal is multiplied by the dimensionless carrier signal $z(t) = \cos(\omega_{\rm T} \cdot T)$ of carrier frequency $f_{\rm T} = 50 \ \rm kHz$.
For DSB–AM, the dashed block is irrelevant so that the following holds for the transmission signal:
- $$s(t) = q(t) \cdot {\rm cos}(\omega_{\rm T} t ) .$$
- In the synchronous demodulator, the received signal $r(t)$ – in an ideal channel identical to the signal $s(t)$ – is multiplied by the receive-site carrier signal $z_{\rm E}(t)$ where the following applies:
- $$z_{\rm E}(t) = K \cdot {\rm cos}(\omega_{\rm T} t - \Delta \varphi ) .$$
- This signal should not only be frequency-synchronous with $z(t)$ but also phase-synchronous ⇒ hence the name "synchronous demodulator".
- The above approach takes into account a phase shift between $z(t)$ and $z_{\rm E}(t)$, which should ideally be $\Delta \varphi = 0$ but often cannot be avoided in real systems.
- The output signal $b(t)$ of the second multiplier includes components around twice the carrier frequency in addition to the desired low-frequency component.
- Using an ideal low-pass filter $\rm (LP)$ (e.g. with cut-off frequency $f_{\rm T}$) the sink signal $v(t)$, which ideally should be equal to the source signal $q(t)$, can be obtained.
For the transmitter, multiplication by the carrier signal $z(t)$ generally results in two sidebands. In Single-Sideband Modulation (ESB–AM), only one of the two bands is transmitted, for example the lower sideband (USB). Thus, the following is obtained with an ideal channel:
- $$r(t) = s(t)= {1 \, \rm V} \cdot {\rm cos}\big [(\omega_{\rm T} - \omega_2 )\cdot t \big ] - {0.5 \, \rm V} \cdot {\rm sin}\big [(\omega_{\rm T} - \omega_5 )\cdot t \big ] .$$
- Here, synchronous demodulation results in the following distorted sink signal considering a phase shift $\Delta \varphi$, the constant $K = 4$ and the downstream low-pass filter:
- $$v(t)= {1 \, \rm V} \cdot {1}/{2}\cdot 4 \cdot{\rm cos}( \omega_2 t - \Delta \varphi)+ {0.5 \, \rm V} \cdot {1}/{2}\cdot 4 \cdot{\rm sin}( \omega_5 t - \Delta \varphi)$$
- $$\Rightarrow \hspace{0.5cm}v(t)= {2 \, \rm V} \cdot{\rm cos}( \omega_2 t - \Delta \varphi)+ {1 \, \rm V} \cdot{\rm sin}( \omega_5 t - \Delta \varphi)$$
- In the ideal case of phase-synchronous demodulation $(\Delta \varphi = 0)$, $v(t) = q(t)$ holds again.
Please note:
- The exercise belongs to the chapter Linear Distortions.
- The topic "amplitude modulation/synchronous demodulator" is discussed in detail in the book Modulation Methods.
- The following trigonometric relationships are given:
- $$\cos^2(\alpha) = {1}/{2} \cdot \big [ 1 + \cos(2\alpha) \big ] \hspace{0.05cm}, $$
- $$\cos(\alpha) \cdot \cos(\beta) = {1}/{2} \cdot \big[ \cos(\alpha - \beta)+ \cos(\alpha + \beta) \big],$$
- $$ \sin(\alpha) \cdot \cos(\beta) = {1}/{2} \cdot \big[ \sin(\alpha - \beta)+ \sin(\alpha + \beta) \big] \hspace{0.05cm}.$$
- The signal designations result from the German original of this exercise. Here again as a listing $q(t)$ ⇒ source signal, $v(t)$ ⇒ sink signal, $z(t)$ ⇒ transmit-site carrier signal, $s(t)$ ⇒ transmission signal (BP), $r(t)$ ⇒ received signal (BP), $z_{\rm E}(t)$ ⇒ receive-site carrier signal, $b(t)$ ⇒ BP signal before low-pass.
Questions
Solution
- $$b(t) = r(t) \cdot z_{\rm E}(t)= q(t) \cdot z(t) \cdot z_{\rm E}(t)= K \cdot q(t)\cdot \cos^2(\omega_{\rm T} t).$$
- Using the trigonometric relation $\cos^2(\omega_{\rm T} t) = {1}/{2} \cdot\big[ 1 + \cos(2\omega_{\rm T} t)\big]$, one obtains:
- $$b(t) = {K}/{2} \cdot q(t) + {K}/{2} \cdot q(t)\cdot \cos(2\omega_{\rm T} t).$$
- The second component is located at around twice the carrier frequency ⇒ $2 f_{\rm T}$.
- This is removed by the low-pass filter $($with the cut-off frequency $ f_{\rm G} = f_{\rm T})$ .
- Hence, the following is obtained: $v(t) = {K}/{2} \cdot q(t) .$
- An ideal demodulation ⇒ $v(t) = q(t)$ is obtained with $\underline {K = 2}$ .
(2) Considering the relation
- $$\cos(\omega_{\rm T} t) \cdot \cos(\omega_{\rm T} t - \Delta \varphi) = {1}/{2} \cdot \big[ \cos(\Delta \varphi)+ \cos(2\omega_{\rm T} t - \Delta \varphi) \big]$$
and the downstream low-pass filter, which removes the component at around twice the carrier frequency, the following is obtained here with $ {K = 2}$:
- $$v(t) = q(t) \cdot \cos(\Delta \varphi).$$
Proposed solutions 2 and 5 are correct:
- A phase shift $\Delta \varphi$ only results in frequency-independent attenuation and not in attenuation distortions or phase distortions.
- A phase shift by $\varphi =\pm 60^\circ$ results in halving of the signal amplitude.
(3) Here, proposed solution 4 is correct.
- Exactly the same phase shift $\Delta \varphi$ occurs for both summands, and phase distortions occur here:
- $$v(t)= {2 \, \rm V} \cdot{\rm cos}\big[ \omega_2 \cdot (t - \tau_2) \big]+ {1 \, \rm V} \cdot{\rm sin}\big[ \omega_5 t \cdot (t - \tau_5)\big],$$
- $${\rm where}\hspace{0.5cm}\tau_2 = \frac{\Delta \varphi}{\omega_2} \hspace{0.5cm}\ne \hspace{0.5cm} \tau_5 = \frac{\Delta \varphi}{\omega_5}.$$
- A phase shift of $\varphi =60^\circ$ corresponding to $\pi/3$ leads to the following delay times here:
- $$\tau_2 = \frac{\pi/3}{2 \pi \cdot 2\,\,{\rm kHz }} \approx 83.3\,{\rm µ s }, \hspace{0.5cm} \tau_5 = \frac{\pi/3}{2 \pi \cdot 5\,\,{\rm kHz }} \approx 33.3\,{\rm µ s }.$$
- The lower-frequency signal is thus delayed more.