Difference between revisions of "Aufgaben:Exercise 2.7Z: Coherence Bandwidth of the LTI Two-Path Channel"
m (Text replacement - "power spectral density" to "power-spectral density") |
|||
| (19 intermediate revisions by 3 users not shown) | |||
| Line 1: | Line 1: | ||
| − | {{quiz-Header|Buchseite= | + | {{quiz-Header|Buchseite=Mobile_Communications/The_GWSSUS_Channel_Model}} |
| − | [[File: | + | [[File:EN_Mob_A_2_7Z.png|right|frame|Two two-path channels]] |
| − | For the GWSSUS | + | For the GWSSUS model, two parameters are given, which both statistically capture the resulting delay $\tau$. More information on the topic "Multipath Propagation" can be found in the section [[Mobile_Communications/The_GWSSUS_Channel_Model#Parameters_of_the_GWSSUS_model| Parameters of the GWSSUS model]] of the theory part. |
| − | * The <b>delay spread</b> $T_{\rm V}$ is by definition equal to the standard deviation of the random variable $\tau$. <br>This can be determined from the probability density $f_{\rm V}(\tau)$ | + | * The <b>delay spread</b> $T_{\rm V}$ is by definition equal to the standard deviation of the random variable $\tau$. <br>This can be determined from the probability density function $f_{\rm V}(\tau)$. The PDF $f_{\rm V}(\tau)$ has the same shape as the delay power-spectral density ${\it \Phi}_{\rm V}(\tau)$. |
| − | * The <b>coherence bandwidth</b> $B_{\rm K}$ describes the same situation in the frequency domain. <br> It is defined as the value of $\ | + | * The <b>coherence bandwidth</b> $B_{\rm K}$ describes the same situation in the frequency domain. <br> It is defined as the value of $\Delta f$ at which the magnitude of the frequency correlation function $\varphi_{\rm F}(\Delta f)$ first drops to half its maximum value: |
:$$|\varphi_{\rm F}(\Delta f = B_{\rm K})| \stackrel {!}{=} {1}/{2} \cdot |\varphi_{\rm F}(\Delta f = 0)| \hspace{0.05cm}.$$ | :$$|\varphi_{\rm F}(\Delta f = B_{\rm K})| \stackrel {!}{=} {1}/{2} \cdot |\varphi_{\rm F}(\Delta f = 0)| \hspace{0.05cm}.$$ | ||
| Line 13: | Line 13: | ||
*Both definitions are only partially suitable for a time-invariant channel. | *Both definitions are only partially suitable for a time-invariant channel. | ||
| − | *For a time invariant two-path channel (i.e. one with constant path weights according to the above graph), the following approximation for the coherence bandwidth is often used: | + | *For a time-invariant two-path channel (i.e. one with constant path weights according to the above graph), the following approximation for the coherence bandwidth is often used: |
:$$B_{\rm K}\hspace{0.01cm}' = \frac{1}{\tau_{\rm max} - \tau_{\rm min}} \hspace{0.05cm}.$$ | :$$B_{\rm K}\hspace{0.01cm}' = \frac{1}{\tau_{\rm max} - \tau_{\rm min}} \hspace{0.05cm}.$$ | ||
| Line 19: | Line 19: | ||
In this task we want to clarify | In this task we want to clarify | ||
* why there are different definitions for the coherence bandwidth in the literature, | * why there are different definitions for the coherence bandwidth in the literature, | ||
| − | * which connection exists between $B_{\rm K}$ and $B_{\rm K}\hspace{0.01cm}'$ and | + | * which connection exists between $B_{\rm K}$ and $B_{\rm K}\hspace{0.01cm}'$, and |
* which definitions make sense for which boundary conditions. | * which definitions make sense for which boundary conditions. | ||
| Line 28: | Line 28: | ||
''Notes:'' | ''Notes:'' | ||
| − | *This | + | *This exercise belongs to the chapter [[Mobile_Communications/The_GWSSUS_Channel_Model|The GWSSUS Channel Model]]. |
| − | *This task also refers to some theory pages in chapter [[ | + | *This task also refers to some theory pages in chapter [[Mobile_Communications/Multi-Path_Reception_in_Mobile_Communications| Multi-Path Reception in Mobile Communications]]. |
| Line 36: | Line 36: | ||
===Questionnaire=== | ===Questionnaire=== | ||
<quiz display=simple> | <quiz display=simple> | ||
| − | {What is the approximate coherence bandwidth $B_{\rm K}\hspace{0.01cm}'$ for channels $\rm A$ and $\rm B$? | + | {What is the approximate coherence bandwidth $B_{\rm K}\hspace{0.01cm}'$ for channels $\rm A$ and $\rm B$? |
|type="{}"} | |type="{}"} | ||
Channel ${\rm A} \text{:} \hspace{0.4cm} B_{\rm K}\hspace{0.01cm}' \ = \ ${ 1000 3% } $\ \ \rm kHz$ | Channel ${\rm A} \text{:} \hspace{0.4cm} B_{\rm K}\hspace{0.01cm}' \ = \ ${ 1000 3% } $\ \ \rm kHz$ | ||
Channel ${\rm B} \text{:} \hspace{0.4cm} B_{\rm K}\hspace{0.01cm}' \ = \ ${ 1000 3% } $\ \ \rm kHz$ | Channel ${\rm B} \text{:} \hspace{0.4cm} B_{\rm K}\hspace{0.01cm}' \ = \ ${ 1000 3% } $\ \ \rm kHz$ | ||
| − | {Let $G$ be the weight of the second path. What is the PDF $f_{\rm V}(\tau)$? | + | {Let $G$ be the weight of the second path. What is the PDF $f_{\rm V}(\tau)$? |
|type="()"} | |type="()"} | ||
- $f_{\rm V}(\tau) = \delta(\tau) + G \cdot \delta(\tau \, –\tau_0)$, | - $f_{\rm V}(\tau) = \delta(\tau) + G \cdot \delta(\tau \, –\tau_0)$, | ||
| Line 53: | Line 53: | ||
{What is the coherence bandwidth $B_{\rm K}$ of channel ${\rm A}$ ? | {What is the coherence bandwidth $B_{\rm K}$ of channel ${\rm A}$ ? | ||
| − | |type=" | + | |type="()"} |
+ $B_{\rm K} = 333 \ \rm kHz$. | + $B_{\rm K} = 333 \ \rm kHz$. | ||
- $B_{\rm K} = 500 \ \rm kHz$. | - $B_{\rm K} = 500 \ \rm kHz$. | ||
| Line 60: | Line 60: | ||
{What is the coherence bandwidth $B_{\rm K}$ of channel ${\rm B}$ ? | {What is the coherence bandwidth $B_{\rm K}$ of channel ${\rm B}$ ? | ||
| − | |type=" | + | |type="()"} |
- $B_{\rm K} = 333 \ \rm kHz$. | - $B_{\rm K} = 333 \ \rm kHz$. | ||
- $B_{\rm K} = 500 \ \rm kHz$. | - $B_{\rm K} = 500 \ \rm kHz$. | ||
| Line 67: | Line 67: | ||
</quiz> | </quiz> | ||
| − | === | + | ===Solution=== |
| − | + | {{ML-Kopf}} | |
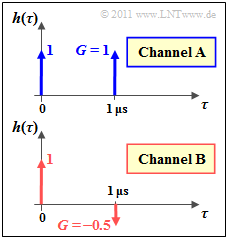
| − | '''(1)''' For both channels, the delay difference is $\Delta \tau = \tau_{\rm max} \, - \tau_{\rm min} = 1 \ \ \rm µ s$. | + | '''(1)''' For both channels, the delay difference is $\Delta \tau = \tau_{\rm max} \, - \tau_{\rm min} = 1 \ \ \rm µ s$. |
* That's why both channels have the same value: | * That's why both channels have the same value: | ||
| − | $$B_{\rm K}\hspace{0.01cm}' \ \ \underline {= 1000 \ \rm kHz}.$$ | + | :$$B_{\rm K}\hspace{0.01cm}' \ \ \underline {= 1000 \ \rm kHz}.$$ |
| + | |||
| − | '''(2)''' The graphs refer to the impulse response $h(\tau)$. | + | '''(2)''' The graphs refer to the impulse response $h(\tau)$. |
| − | *To obtain the delay–power density | + | *To obtain the delay–power-spectral density, the weights must be squared: |
:$${\it \Phi}_{\rm V}(\tau) = 1^2 \cdot \delta(\tau) + G^2 \cdot \delta(\tau - \tau_0) \hspace{0.05cm}.$$ | :$${\it \Phi}_{\rm V}(\tau) = 1^2 \cdot \delta(\tau) + G^2 \cdot \delta(\tau - \tau_0) \hspace{0.05cm}.$$ | ||
| − | *The integral of ${\it \Phi}_{\rm V}(\tau)$ is therefore $1 + G^2$. | + | *The integral of ${\it \Phi}_{\rm V}(\tau)$ is therefore $1 + G^2$. |
| − | *The probability density function (PDF), however, must have | + | *The probability density function $\rm (PDF)$, however, must have "area $1$" $($i.e., the sum of the two Dirac weights must be $1)$. From this follows: |
:$$f_{\rm V}(\tau) = \frac{1}{1 + G^2} \cdot \delta(\tau) + \frac{G^2}{1 + G^2} \cdot \delta(\tau - \tau_0) \hspace{0.05cm}.$$ | :$$f_{\rm V}(\tau) = \frac{1}{1 + G^2} \cdot \delta(\tau) + \frac{G^2}{1 + G^2} \cdot \delta(\tau - \tau_0) \hspace{0.05cm}.$$ | ||
| − | * | + | *Only <u>solution 3</u> is correct. |
| − | *The first option does not describe the PDF $f_{\rm V}(\tau)$, but the impulse response $h(\tau)$. | + | *The first option does not describe the PDF $f_{\rm V}(\tau)$, but the impulse response $h(\tau)$. |
| − | *The second equation specifies the delay | + | *The second equation specifies the delay power-spectral density ${\it \Phi}_{\rm V}(\tau)$. |
| − | '''(3)''' For channel $\rm A$ the two impulse weights are equal. | + | '''(3)''' For channel $\rm A$ the two impulse weights are equal. This means that the mean value $m_{\rm V}$ and the standard deviation $\sigma_{\rm V} = T_{\rm V}$ can be computed simply: |
| − | |||
:$$m_{\rm V} = \frac{\tau_0}{2} \hspace{0.15cm} {= 0.5\,{\rm µ s}}\hspace{0.05cm}, | :$$m_{\rm V} = \frac{\tau_0}{2} \hspace{0.15cm} {= 0.5\,{\rm µ s}}\hspace{0.05cm}, | ||
\hspace{0.2cm}T_{\rm V} = \sigma_{\rm V} =\frac{\tau_0}{2} \hspace{0.15cm}\underline {= 0.5\,{\rm µ s}} | \hspace{0.2cm}T_{\rm V} = \sigma_{\rm V} =\frac{\tau_0}{2} \hspace{0.15cm}\underline {= 0.5\,{\rm µ s}} | ||
\hspace{0.05cm}.$$ | \hspace{0.05cm}.$$ | ||
| − | For channel $\rm B$ the Dirac weights are $1/(1+0.5^2) = 0.8$ (for $\tau = 0$ | + | For channel $\rm B$ the Dirac weights are $1/(1+0.5^2) = 0.8$ $($for $\tau = 0)$ and $0.2$ $($for $\tau = 1 \ \rm µ s)$. |
| − | * According to the [[ | + | * According to the [[Theory_of_Stochastic_Signals/Expected_Values_and_Moments|basic laws]] of statistics, the non-central first and second order moments are: |
:$$m_{\rm 1} \hspace{-0.1cm} \ = \ \hspace{-0.1cm} 0.8 \cdot 0 + 0.2 \cdot 1\,{\rm µ s} = 0.2\,{\rm µ s} \hspace{0.05cm},\hspace{0.5cm} | :$$m_{\rm 1} \hspace{-0.1cm} \ = \ \hspace{-0.1cm} 0.8 \cdot 0 + 0.2 \cdot 1\,{\rm µ s} = 0.2\,{\rm µ s} \hspace{0.05cm},\hspace{0.5cm} | ||
m_{\rm 2} \hspace{-0.1cm} \ = \ \hspace{-0.1cm} 0.8 \cdot 0^2 + 0.2 \cdot (1\,{\rm µ s})^2 = 0.2\,({\rm µ s})^2 \hspace{0.05cm}.$$ | m_{\rm 2} \hspace{-0.1cm} \ = \ \hspace{-0.1cm} 0.8 \cdot 0^2 + 0.2 \cdot (1\,{\rm µ s})^2 = 0.2\,({\rm µ s})^2 \hspace{0.05cm}.$$ | ||
| − | *To get the result you are looking for you can use the [[ | + | *To get the result you are looking for you can use the [[Theory_of_Stochastic_Signals/Expected_Values_and_Moments#Some_common_central_moments| Steiner's Theorem]]: |
:$$\sigma_{\rm V}^2 = m_{\rm 2} - m_{\rm 1}^2 = 0.2\,({\rm µ s})^2 - (0.2\,{\rm µ s})^2 = 0.16\,({\rm µ s})^2 | :$$\sigma_{\rm V}^2 = m_{\rm 2} - m_{\rm 1}^2 = 0.2\,({\rm µ s})^2 - (0.2\,{\rm µ s})^2 = 0.16\,({\rm µ s})^2 | ||
\hspace{0.3cm}\Rightarrow \hspace{0.3cm}T_{\rm V} = \sigma_{\rm V} \hspace{0.15cm}\underline {= 0.4\,{\rm µ s}}\hspace{0.05cm}.$$ | \hspace{0.3cm}\Rightarrow \hspace{0.3cm}T_{\rm V} = \sigma_{\rm V} \hspace{0.15cm}\underline {= 0.4\,{\rm µ s}}\hspace{0.05cm}.$$ | ||
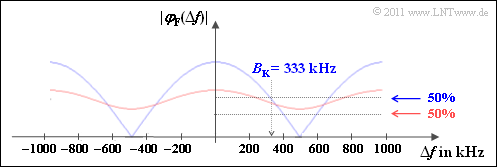
| − | '''(4)''' The frequency correlation function is the Fourier transform of ${\it \Phi}_{\rm V}(\tau) = \delta(\tau) + \delta(\tau \, – \tau_0)$: | + | '''(4)''' The frequency correlation function is the Fourier transform of ${\it \Phi}_{\rm V}(\tau) = \delta(\tau) + \delta(\tau \, – \tau_0)$: |
:$$\varphi_{\rm F}(\Delta f) = 1 + {\rm exp}(-{\rm j} \cdot 2\pi \cdot \Delta f \cdot \tau_0) = 1 + {\rm cos}(2\pi \cdot \Delta f \cdot \tau_0) -{\rm j} \cdot {\rm sin}(2\pi \cdot \Delta f \cdot \tau_0) $$ | :$$\varphi_{\rm F}(\Delta f) = 1 + {\rm exp}(-{\rm j} \cdot 2\pi \cdot \Delta f \cdot \tau_0) = 1 + {\rm cos}(2\pi \cdot \Delta f \cdot \tau_0) -{\rm j} \cdot {\rm sin}(2\pi \cdot \Delta f \cdot \tau_0) $$ | ||
[[File:P_ID2186__Mob_Z_2_7d.png|right|frame|Frequency correlation function and coherence bandwidth]] | [[File:P_ID2186__Mob_Z_2_7d.png|right|frame|Frequency correlation function and coherence bandwidth]] | ||
:$$\Rightarrow \hspace{0.3cm} |\varphi_{\rm F}(\Delta f)| = \sqrt{2 + 2 \cdot {\rm cos}(2\pi \cdot \Delta f \cdot \tau_0) }\hspace{0.05cm}.$$ | :$$\Rightarrow \hspace{0.3cm} |\varphi_{\rm F}(\Delta f)| = \sqrt{2 + 2 \cdot {\rm cos}(2\pi \cdot \Delta f \cdot \tau_0) }\hspace{0.05cm}.$$ | ||
| − | *The maximum at $\ | + | *The maximum at $\Delta f = 0$ is equal to $2$. |
*Therefore the equation to determine $B_{\rm K}$ is | *Therefore the equation to determine $B_{\rm K}$ is | ||
| − | $$|\varphi_{\rm F}(B_{\rm K})| = 1 \hspace{0.3cm} | + | :$$|\varphi_{\rm F}(B_{\rm K})| = 1 \hspace{0.3cm} |
| − | + | \Rightarrow \hspace{0.3cm}|\varphi_{\rm F}(B_{\rm K})|^2 = 1 $$ | |
| − | + | :$$ \Rightarrow \hspace{0.3cm}2 + 2 \cdot {\rm cos}(2\pi \cdot B_{\rm K} \cdot \tau_0) = 1 \hspace{0.3cm} | |
| − | + | \Rightarrow \hspace{0.3cm}{\rm cos}(2\pi \cdot B_{\rm K} \cdot \tau_0) = -0.5 \hspace{0.3cm} $$ | |
| − | $$\Rightarrow \hspace{0.3cm}2\pi \cdot B_{\rm K} \cdot \tau_0 = \frac{2\pi}{3}\hspace{0.3cm} \Rightarrow \hspace{0.3cm}B_{\rm K} = \frac{1}{3\tau_0} = 333\,{\rm kHz}\hspace{0.05cm}.$$ | + | :$$\Rightarrow \hspace{0.3cm}2\pi \cdot B_{\rm K} \cdot \tau_0 = \frac{2\pi}{3}\hspace{0.3cm} \Rightarrow \hspace{0.3cm}B_{\rm K} = \frac{1}{3\tau_0} = 333\,{\rm kHz}\hspace{0.05cm}.$$ |
| + | |||
| + | <u>Solution 1</u> is correct. The graph (blue curve) illustrates the result. | ||
| − | |||
'''(5)''' For channel ${\rm B}$ the corresponding equations are | '''(5)''' For channel ${\rm B}$ the corresponding equations are | ||
| − | :$${\it \Phi}_{\rm V}(\tau) \hspace{-0.1cm} \ = \ \hspace{-0.1cm} 1^2 \cdot \delta(\tau) + (-0.5)^2 \cdot \delta(\tau - \tau_0) \hspace{0.05cm},\hspace{0.05cm} | + | :$${\it \Phi}_{\rm V}(\tau) \hspace{-0.1cm} \ = \ \hspace{-0.1cm} 1^2 \cdot \delta(\tau) + (-0.5)^2 \cdot \delta(\tau - \tau_0) \hspace{0.05cm},\hspace{0.05cm}$$ |
| − | \varphi_{\rm F}(\Delta f) \hspace{-0.1cm} \ = \ \hspace{-0.1cm} 1 + 0.25 \cdot {\rm cos}(2\pi \cdot \Delta f \cdot \tau_0) -{\rm j} \cdot 0.25 \cdot {\rm sin}(2\pi \cdot \Delta f \cdot \tau_0)\hspace{0.05cm},$$ | + | :$$\varphi_{\rm F}(\Delta f) \hspace{-0.1cm} \ = \ \hspace{-0.1cm} 1 + 0.25 \cdot {\rm cos}(2\pi \cdot \Delta f \cdot \tau_0) -{\rm j} \cdot 0.25 \cdot {\rm sin}(2\pi \cdot \Delta f \cdot \tau_0)\hspace{0.05cm},$$ |
| − | :$$|\varphi_{\rm F}(\Delta f)| \hspace{-0.1cm} \ = \ | + | :$$|\varphi_{\rm F}(\Delta f)| \hspace{-0.1cm} \ = \ \sqrt{\frac{17}{16} + \frac{1}{2} \cdot {\rm cos}(2\pi \cdot \Delta f \cdot \tau_0) }\hspace{0.3cm}$$ |
| − | \Rightarrow \hspace{0.3cm}{\rm Max}\hspace{0.1cm}|\varphi_{\rm F}(\Delta f)| = 1.25\hspace{0.05cm},\hspace{0.2cm}{\rm Min}\hspace{0.1cm}|\varphi_{\rm F}(\Delta f)| = 0.75\hspace{0.05cm}.$$ | + | :$$\Rightarrow \hspace{0.3cm}{\rm Max}\hspace{0.1cm}|\varphi_{\rm F}(\Delta f)| = 1.25\hspace{0.05cm},\hspace{0.2cm}{\rm Min}\hspace{0.1cm}|\varphi_{\rm F}(\Delta f)| = 0.75\hspace{0.05cm}.$$ |
| − | + | You can see from this result that the $50\%$–coherence bandwidth cannot be specified here ⇒ <u>solution 4</u> is correct. | |
| − | |||
| − | This result is the reason why there are different definitions for the coherence | + | This result is the reason why there are different definitions for the coherence bandwidth in the literature, for example |
| − | * the $90\%$–coherence bandwidth (in the example $B_{\rm K, \hspace{0.03cm} 90\%} =184 \ \ \rm kHz$), | + | * the $90\%$–coherence bandwidth $($in the example $B_{\rm K, \hspace{0.03cm} 90\%} =184 \ \ \rm kHz$$)$, |
| − | * the very simple approximation $B_{\rm K}\hspace{0.01cm}'$ given above (in the example $B_{\rm K}\hspace{0.01cm}' =1 \ \ \rm MHz$ | + | * the very simple approximation $B_{\rm K}\hspace{0.01cm}'$ given above $($in the example $B_{\rm K}\hspace{0.01cm}' =1 \ \ \rm MHz)$. |
| − | You can see from these numerical values that all the information on this is very vague and that the individual | + | You can see from these numerical values that all the information on this topic is very vague and that the individual "coherence bandwidths" can be very different. |
{{ML-Fuß}} | {{ML-Fuß}} | ||
| − | [[Category: | + | [[Category:Mobile Communications: Exercises|^2.3 The GWSSUS Channel Model^]] |
Latest revision as of 13:41, 17 February 2022
For the GWSSUS model, two parameters are given, which both statistically capture the resulting delay $\tau$. More information on the topic "Multipath Propagation" can be found in the section Parameters of the GWSSUS model of the theory part.
- The delay spread $T_{\rm V}$ is by definition equal to the standard deviation of the random variable $\tau$.
This can be determined from the probability density function $f_{\rm V}(\tau)$. The PDF $f_{\rm V}(\tau)$ has the same shape as the delay power-spectral density ${\it \Phi}_{\rm V}(\tau)$. - The coherence bandwidth $B_{\rm K}$ describes the same situation in the frequency domain.
It is defined as the value of $\Delta f$ at which the magnitude of the frequency correlation function $\varphi_{\rm F}(\Delta f)$ first drops to half its maximum value:
- $$|\varphi_{\rm F}(\Delta f = B_{\rm K})| \stackrel {!}{=} {1}/{2} \cdot |\varphi_{\rm F}(\Delta f = 0)| \hspace{0.05cm}.$$
The relationship between ${\it \Phi}_{\rm V}(\tau)$ and $\varphi_{\rm F}(\Delta f)$ is given by the Fourier transform:
- $$\varphi_{\rm F}(\Delta f) \hspace{0.2cm} {\bullet\!\!-\!\!\!-\!\!\!-\!\!\circ} \hspace{0.2cm} {\it \Phi}_{\rm V}(\tau)\hspace{0.05cm}.$$
- Both definitions are only partially suitable for a time-invariant channel.
- For a time-invariant two-path channel (i.e. one with constant path weights according to the above graph), the following approximation for the coherence bandwidth is often used:
- $$B_{\rm K}\hspace{0.01cm}' = \frac{1}{\tau_{\rm max} - \tau_{\rm min}} \hspace{0.05cm}.$$
In this task we want to clarify
- why there are different definitions for the coherence bandwidth in the literature,
- which connection exists between $B_{\rm K}$ and $B_{\rm K}\hspace{0.01cm}'$, and
- which definitions make sense for which boundary conditions.
Notes:
- This exercise belongs to the chapter The GWSSUS Channel Model.
- This task also refers to some theory pages in chapter Multi-Path Reception in Mobile Communications.
Questionnaire
Solution
- That's why both channels have the same value:
- $$B_{\rm K}\hspace{0.01cm}' \ \ \underline {= 1000 \ \rm kHz}.$$
(2) The graphs refer to the impulse response $h(\tau)$.
- To obtain the delay–power-spectral density, the weights must be squared:
- $${\it \Phi}_{\rm V}(\tau) = 1^2 \cdot \delta(\tau) + G^2 \cdot \delta(\tau - \tau_0) \hspace{0.05cm}.$$
- The integral of ${\it \Phi}_{\rm V}(\tau)$ is therefore $1 + G^2$.
- The probability density function $\rm (PDF)$, however, must have "area $1$" $($i.e., the sum of the two Dirac weights must be $1)$. From this follows:
- $$f_{\rm V}(\tau) = \frac{1}{1 + G^2} \cdot \delta(\tau) + \frac{G^2}{1 + G^2} \cdot \delta(\tau - \tau_0) \hspace{0.05cm}.$$
- Only solution 3 is correct.
- The first option does not describe the PDF $f_{\rm V}(\tau)$, but the impulse response $h(\tau)$.
- The second equation specifies the delay power-spectral density ${\it \Phi}_{\rm V}(\tau)$.
(3) For channel $\rm A$ the two impulse weights are equal. This means that the mean value $m_{\rm V}$ and the standard deviation $\sigma_{\rm V} = T_{\rm V}$ can be computed simply:
- $$m_{\rm V} = \frac{\tau_0}{2} \hspace{0.15cm} {= 0.5\,{\rm µ s}}\hspace{0.05cm}, \hspace{0.2cm}T_{\rm V} = \sigma_{\rm V} =\frac{\tau_0}{2} \hspace{0.15cm}\underline {= 0.5\,{\rm µ s}} \hspace{0.05cm}.$$
For channel $\rm B$ the Dirac weights are $1/(1+0.5^2) = 0.8$ $($for $\tau = 0)$ and $0.2$ $($for $\tau = 1 \ \rm µ s)$.
- According to the basic laws of statistics, the non-central first and second order moments are:
- $$m_{\rm 1} \hspace{-0.1cm} \ = \ \hspace{-0.1cm} 0.8 \cdot 0 + 0.2 \cdot 1\,{\rm µ s} = 0.2\,{\rm µ s} \hspace{0.05cm},\hspace{0.5cm} m_{\rm 2} \hspace{-0.1cm} \ = \ \hspace{-0.1cm} 0.8 \cdot 0^2 + 0.2 \cdot (1\,{\rm µ s})^2 = 0.2\,({\rm µ s})^2 \hspace{0.05cm}.$$
- To get the result you are looking for you can use the Steiner's Theorem:
- $$\sigma_{\rm V}^2 = m_{\rm 2} - m_{\rm 1}^2 = 0.2\,({\rm µ s})^2 - (0.2\,{\rm µ s})^2 = 0.16\,({\rm µ s})^2 \hspace{0.3cm}\Rightarrow \hspace{0.3cm}T_{\rm V} = \sigma_{\rm V} \hspace{0.15cm}\underline {= 0.4\,{\rm µ s}}\hspace{0.05cm}.$$
(4) The frequency correlation function is the Fourier transform of ${\it \Phi}_{\rm V}(\tau) = \delta(\tau) + \delta(\tau \, – \tau_0)$:
- $$\varphi_{\rm F}(\Delta f) = 1 + {\rm exp}(-{\rm j} \cdot 2\pi \cdot \Delta f \cdot \tau_0) = 1 + {\rm cos}(2\pi \cdot \Delta f \cdot \tau_0) -{\rm j} \cdot {\rm sin}(2\pi \cdot \Delta f \cdot \tau_0) $$
- $$\Rightarrow \hspace{0.3cm} |\varphi_{\rm F}(\Delta f)| = \sqrt{2 + 2 \cdot {\rm cos}(2\pi \cdot \Delta f \cdot \tau_0) }\hspace{0.05cm}.$$
- The maximum at $\Delta f = 0$ is equal to $2$.
- Therefore the equation to determine $B_{\rm K}$ is
- $$|\varphi_{\rm F}(B_{\rm K})| = 1 \hspace{0.3cm} \Rightarrow \hspace{0.3cm}|\varphi_{\rm F}(B_{\rm K})|^2 = 1 $$
- $$ \Rightarrow \hspace{0.3cm}2 + 2 \cdot {\rm cos}(2\pi \cdot B_{\rm K} \cdot \tau_0) = 1 \hspace{0.3cm} \Rightarrow \hspace{0.3cm}{\rm cos}(2\pi \cdot B_{\rm K} \cdot \tau_0) = -0.5 \hspace{0.3cm} $$
- $$\Rightarrow \hspace{0.3cm}2\pi \cdot B_{\rm K} \cdot \tau_0 = \frac{2\pi}{3}\hspace{0.3cm} \Rightarrow \hspace{0.3cm}B_{\rm K} = \frac{1}{3\tau_0} = 333\,{\rm kHz}\hspace{0.05cm}.$$
Solution 1 is correct. The graph (blue curve) illustrates the result.
(5) For channel ${\rm B}$ the corresponding equations are
- $${\it \Phi}_{\rm V}(\tau) \hspace{-0.1cm} \ = \ \hspace{-0.1cm} 1^2 \cdot \delta(\tau) + (-0.5)^2 \cdot \delta(\tau - \tau_0) \hspace{0.05cm},\hspace{0.05cm}$$
- $$\varphi_{\rm F}(\Delta f) \hspace{-0.1cm} \ = \ \hspace{-0.1cm} 1 + 0.25 \cdot {\rm cos}(2\pi \cdot \Delta f \cdot \tau_0) -{\rm j} \cdot 0.25 \cdot {\rm sin}(2\pi \cdot \Delta f \cdot \tau_0)\hspace{0.05cm},$$
- $$|\varphi_{\rm F}(\Delta f)| \hspace{-0.1cm} \ = \ \sqrt{\frac{17}{16} + \frac{1}{2} \cdot {\rm cos}(2\pi \cdot \Delta f \cdot \tau_0) }\hspace{0.3cm}$$
- $$\Rightarrow \hspace{0.3cm}{\rm Max}\hspace{0.1cm}|\varphi_{\rm F}(\Delta f)| = 1.25\hspace{0.05cm},\hspace{0.2cm}{\rm Min}\hspace{0.1cm}|\varphi_{\rm F}(\Delta f)| = 0.75\hspace{0.05cm}.$$
You can see from this result that the $50\%$–coherence bandwidth cannot be specified here ⇒ solution 4 is correct.
This result is the reason why there are different definitions for the coherence bandwidth in the literature, for example
- the $90\%$–coherence bandwidth $($in the example $B_{\rm K, \hspace{0.03cm} 90\%} =184 \ \ \rm kHz$$)$,
- the very simple approximation $B_{\rm K}\hspace{0.01cm}'$ given above $($in the example $B_{\rm K}\hspace{0.01cm}' =1 \ \ \rm MHz)$.
You can see from these numerical values that all the information on this topic is very vague and that the individual "coherence bandwidths" can be very different.