Difference between revisions of "Aufgaben:Exercise 3.13: Threshold Decision vs. DFE vs. Maximum Likelihood"
(Die Seite wurde neu angelegt: „ {{quiz-Header|Buchseite=Digitalsignalübertragung/Viterbi–Empfänger}} Datei:P_ID1479__Dig_A_3_13.png|right|frame|Fehlerwahrscheinlichkeitsvergleich SW -…“) |
|||
| (42 intermediate revisions by 6 users not shown) | |||
| Line 1: | Line 1: | ||
| − | {{quiz-Header|Buchseite= | + | {{quiz-Header|Buchseite=Digital_Signal_Transmission/Viterbi_Receiver}} |
| + | '''Bitte diese Aufgabe sehr genau kontrollieren. Da habe ich etliche Änderungen vorgenommen.''' | ||
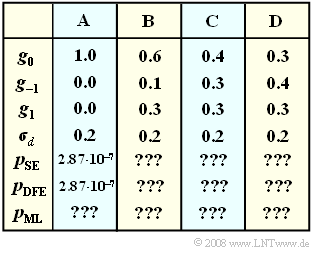
| − | [[File:P_ID1479__Dig_A_3_13.png|right|frame| | + | [[File:P_ID1479__Dig_A_3_13.png|right|frame|Error probabilities in comparison: <br>$\bullet$ Threshold Decision $\rm (SE)$, <br>$\bullet$ Decision Feedback Equalization $\text{(DFE)}$, <br>$\bullet$ Maximum Likelihood Detection $\text{(ML)}$]] |
| − | + | Error probabilities of different receiver types are to be compared. Considered are: | |
| − | * Schwellenwertentscheidung | + | * Threshold Decision $($German: "Schwellenwertentscheidung" ⇒ "$\rm SE$"$)$ ⇒ error probability $p_{\rm SE}$, |
| − | * | + | * Decision Feedback Equalization $\rm (DFE)$ ⇒ error probability $p_{\rm DFE}$ and |
| − | * Maximum& | + | * Maximum Likelihood Detection $\rm (ML)$ ⇒ error probability $p_{\rm ML}$. |
| − | |||
| − | + | In the table given are four different parameter sets $\rm A$, $\rm B$, $\rm C$ and $\rm D$: | |
| − | + | *The "main value" $g_0$ of the basic detection pulse, | |
| − | + | *the "precursor" $g_{\rm –1}$, | |
| − | + | *the "postcursor" (trailer) $g_1$, | |
| − | + | *the rms value $\sigma_d$ of the detection noise component $d_{\rm N}(t)$ before the respective decision. | |
| − | |||
| − | |||
| − | |||
| − | + | ||
| − | :$$p_{\rm SE } =p_{\rm U,\hspace{0. | + | Bipolar amplitude coefficients are assumed, so that, for example, for the worst-case error probability $($German: "ungünstigste Fehlerwahrscheinlichkeit" ⇒ "$\rm U$"$)$ of the receiver with threshold decision, the following applies: |
| + | :$$p_{\rm U,\hspace{0.15cm} SE } = \left\{ \begin{array}{c} {\rm Q}\big [ ({g_0-|g_{-1}|-|g_{1}|})/{\sigma_d} \big ]\\ | ||
| + | \\{\rm Q}(0) = 0.5 \end{array} \right.\quad | ||
| + | \begin{array}{*{1}c} {\rm with }\hspace{0.15cm}{\rm open }\hspace{0.15cm}{\rm eye }, | ||
| + | \\ \\{\rm with }\hspace{0.15cm}{\rm closed }\hspace{0.15cm}{\rm eye }. \\ \end{array}\begin{array}{*{20}c} | ||
| + | \\ | ||
| + | \end{array}$$ | ||
| + | |||
| + | For the Nyquist system $\rm A$, the mean error probability is exactly the same, viz. | ||
| + | :$$p_{\rm SE } =p_{\rm U,\hspace{0.15cm} SE } = {\rm Q}\left( {g_0}/{\sigma_d} \right)= {\rm | ||
Q}(5) \approx 2.87 \cdot 10^{-7}\hspace{0.05cm}.$$ | Q}(5) \approx 2.87 \cdot 10^{-7}\hspace{0.05cm}.$$ | ||
| − | + | For the other system variants $\rm B$, $\rm C$ and $\rm D$ considered here, the intersymbol interferences are so strong and the given noise rms value is so small that the following approximation can be applied: | |
| − | :$$p_{\rm SE } \approx {1}/{4} \cdot p_{\rm U,\hspace{0. | + | :$$p_{\rm SE } \approx {1}/{4} \cdot p_{\rm U,\hspace{0.1cm} SE } |
| − | = {1}/{4} \cdot {\rm Q}\left( \frac {{\rm Max }\hspace{0.05cm}[0, \hspace{0.05cm}g_0-|g_{-1}|-|g_{1}|]}{\sigma_d} \right)\hspace{0.05cm}.$$ | + | = {1}/{4} \cdot {\rm Q}\left( \frac {{\rm Max }\hspace{0.05cm}\big [0, \hspace{0.05cm}g_0-|g_{-1}|-|g_{1}|\big ]}{\sigma_d} \right)\hspace{0.05cm}.$$ |
| − | + | Except for the Nyquist system $\rm A$ $($here $p_{\rm DFE} = p_{\rm SE})$, the following approximation applies to the DFE receiver instead: | |
| − | :$$p_{\rm DFE } \approx {1}/{2} \cdot p_{\rm U,\hspace{0. | + | :$$p_{\rm DFE } \approx {1}/{2} \cdot p_{\rm U,\hspace{0.1cm} DFE } |
| − | = {1}/{2} \cdot {\rm Q}\left( \frac{{\rm Max }\hspace{0.05cm}[0, \hspace{0.05cm}g_0-|g_{-1}|]}{\sigma_d} \right)\hspace{0.05cm}.$$ | + | = {1}/{2} \cdot {\rm Q}\left( \frac{{\rm Max }\hspace{0.05cm}\big [0, \hspace{0.05cm}g_0-|g_{-1}|\big ]}{\sigma_d} \right)\hspace{0.05cm}.$$ |
| − | + | In contrast, it was shown in the [[Digital_Signal_Transmission/Viterbi_Receiver#Bit_error_probability_with_maximum_likelihood_decision|"last theory section"]] for this chapter that for a receiver with ML decision, the following approximation holds: | |
:$$p_{\rm ML } | :$$p_{\rm ML } | ||
= {\rm Q}\left( \frac{{\rm Max }\hspace{0.05cm}[g_{\nu}]}{\sigma_d} \right)\hspace{0.05cm}.$$ | = {\rm Q}\left( \frac{{\rm Max }\hspace{0.05cm}[g_{\nu}]}{\sigma_d} \right)\hspace{0.05cm}.$$ | ||
| − | + | ||
| − | * | + | |
| − | * | + | Notes: |
| − | * | + | *The exercise belongs to the chapter [[Digital_Signal_Transmission/Viterbi_Receiver|"Viterbi Receiver"]]. |
| + | |||
| + | *Reference is also made to the chapters [[Digital_Signal_Transmission/Linear_Nyquist_Equalization|"Linear Nyquist Equalization"]] and [[Digital_Signal_Transmission/Decision_Feedback|"Decision Feedback"]]. | ||
| + | |||
| + | * You can determine the numerical values of the Q-function using the interaction module [[Applets:Komplementäre_Gaußsche_Fehlerfunktionen|"Complementary Gaussian Error Functions"]]. | ||
| + | |||
| + | * To apply the algorithm given in the theory section for two precursors, you would have to make the following renamings <br>$($which, however, has no meaning for the calculation of the error probabilities$)$: | ||
:$$g_{1 }\hspace{0.1cm}\Rightarrow \hspace{0.1cm}g_{0 },\hspace{0.4cm} | :$$g_{1 }\hspace{0.1cm}\Rightarrow \hspace{0.1cm}g_{0 },\hspace{0.4cm} | ||
g_{0 }\hspace{0.1cm}\Rightarrow \hspace{0.1cm}g_{-1 },\hspace{0.4cm} | g_{0 }\hspace{0.1cm}\Rightarrow \hspace{0.1cm}g_{-1 },\hspace{0.4cm} | ||
| Line 44: | Line 56: | ||
\hspace{0.05cm}.$$ | \hspace{0.05cm}.$$ | ||
| − | |||
| + | ===Questions=== | ||
| + | <quiz display=simple> | ||
| + | {What is the error probability for system $\rm A$ with maximum likelihood detection $\rm (ML)$? | ||
| + | |type="{}"} | ||
| + | $\hspace{0.2cm} p_{\rm ML} \ = \ $ { 2.87 3% } $\ \cdot 10^{\rm –7} $ | ||
| + | |||
| + | {What error probabilities are to be expected with system $\rm B$? | ||
| + | |type="{}"} | ||
| + | $\hspace{0.25cm} p_{\rm SE} \ = \ $ { 4 3% } $\ \% $ | ||
| + | $p_{\rm DFE} \ = \ $ { 0.31 3% } $\ \% $ | ||
| + | $\hspace{0.2cm} p_{\rm ML} \ = \ $ { 0.135 3% } $\ \% $ | ||
| − | + | {What are the error probabilities for system $\rm C$? | |
| − | + | |type="{}"} | |
| − | { | + | $\hspace{0.25cm} p_{\rm SE} \ = \ $ { 12.5 3% } $\ \% $ |
| − | |type=" | + | $p_{\rm DFE} \ = \ $ { 15.0 3% } $\ \% $ |
| − | + | $\hspace{0.2cm} p_{\rm ML} \ = \ $ { 2.27 3% } $\ \% $ | |
| − | |||
| − | { | + | {What error probabilities are to be expected with system $\rm D$? |
|type="{}"} | |type="{}"} | ||
| − | $\ | + | $\hspace{0.25cm} p_{\rm SE} \ = \ $ { 25.0 3% } $\ \% $ |
| + | $p_{\rm DFE} \ = \ $ { 35.0 3% } $\ \% $ | ||
| + | $\hspace{0.2cm} p_{\rm ML} \ = \ $ { 2.27 3% } $\ \% $ | ||
</quiz> | </quiz> | ||
| − | === | + | ===Solution=== |
{{ML-Kopf}} | {{ML-Kopf}} | ||
| − | '''1 | + | '''(1)''' Without intersymbol interference $\text{(system A)}$, the DFE and ML receivers do not improve over the simple threshold decision: |
| − | '''2 | + | :$$ p_{\rm DFE } = p_{\rm ML } = p_{\rm SE } \hspace{0.15cm}\underline {\approx 2.87 \cdot 10^{-7}} |
| − | '''3 | + | \hspace{0.05cm}.$$ |
| − | + | ||
| − | + | ||
| − | ''' | + | '''(2)''' With $g_0 = 0.6$, $g_{\rm –1} = 0.1$ and $g_1 = 0.3$, $\text{(system B)}$, one obtains approximately: |
| − | ''' | + | :$$p_{\rm SE } \ \approx \ {1}/{4} \cdot {\rm Q}\left( \frac{0.6-0.1-0.3}{0.2} \right)= {1}/{4} \cdot{\rm Q}(1) \hspace{0.15cm}\underline {\approx 4\% |
| + | \hspace{0.05cm}},$$ | ||
| + | :$$ p_{\rm DFE } \ \approx \ {1}/{2} \cdot {\rm Q}\left( \frac{0.6-0.1}{0.2} \right)= {1}/{2} \cdot {\rm Q}(2.5) \hspace{0.15cm}\underline {\approx | ||
| + | 0.31\%} \hspace{0.05cm},$$ | ||
| + | :$$ p_{\rm ML } \ \approx \ {\rm Q}\left( \frac{0.6}{0.2} \right) = {\rm Q}(3) \hspace{0.15cm}\underline {\approx 0.135\%} | ||
| + | \hspace{0.05cm}.$$ | ||
| + | |||
| + | |||
| + | '''(3)''' With $g_0 = 0.4$ and $g_1 = g_{\rm –1} = 0.3$ $\text{(system C)}$, one obtains approximately: | ||
| + | :$$p_{\rm SE } \ \approx \ {1}/{4} \cdot{\rm Q}(0) \hspace{0.15cm}\underline {= 12.5\%} \hspace{0.3cm}\Rightarrow \hspace{0.3cm}{\rm closed }\hspace{0.15cm}{\rm eye } | ||
| + | \hspace{0.05cm},$$ | ||
| + | :$$ p_{\rm DFE } \ \approx \ {1}/{2} \cdot {\rm Q}\left( \frac{0.4-0.3}{0.2} \right)= {1}/{2} \cdot {\rm Q}(0.5) \hspace{0.15cm}\underline {\approx | ||
| + | 15\% \hspace{0.05cm}},$$ | ||
| + | :$$ p_{\rm ML } \ \approx \ {\rm Q}\left( \frac{0.4}{0.2} \right) = {\rm Q}(2) \hspace{0.15cm}\underline {\approx | ||
| + | 2.27\%} | ||
| + | \hspace{0.05cm}.$$ | ||
| + | |||
| + | *Interesting is – and not a calculation error – that the DFE is worse than the conventional threshold decision when the error probability is $10\%$ or more. | ||
| + | *See also the solution for subtask '''(4)'''. | ||
| + | |||
| + | |||
| + | '''(4)''' With system $\text{D}$, the DFE receiver also has a closed eye. | ||
| + | *$p_{\rm DFE}$ is greater than $p_{\rm SE}$, since the worst-case symbol sequence now occurs more frequently. According to the given simple approximation holds: | ||
| + | :$$p_{\rm SE } = {1}/{4} \cdot{\rm Q}(0) = 0.125\hspace{0.05cm}, \hspace{0.2cm} | ||
| + | p_{\rm DFE } = {1}/{2} \cdot{\rm Q}(0) \hspace{0.15cm}\underline {= 0.250} | ||
| + | \hspace{0.05cm}.$$ | ||
| + | |||
| + | *On the other hand, with an exact calculation one obtains: | ||
| + | :$$p_{\rm SE } \ = \ {1}/{4} \cdot {\rm Q}\left( \frac{0.3-0.4-0.3}{0.2}\right) | ||
| + | + {1}/{4} \cdot{\rm Q}\left( \frac{0.3-0.4+0.3}{0.2}\right)+ \ {1}/{4} \cdot {\rm Q}\left( \frac{0.3+0.4-0.3}{0.2}\right) | ||
| + | +{1}/{4} \cdot{\rm Q}\left( \frac{0.3+0.4+0.3}{0.2}\right)$$ | ||
| + | :$$ \Rightarrow \hspace{0.3cm}p_{\rm SE } \ = \ {1}/{4} \cdot \left[ {\rm Q}(-2) + {\rm Q}(1) +{\rm Q}(2) +{\rm Q}(5) \right] | ||
| + | ={1}/{4} \cdot \left[ 1+ {\rm Q}(1) +{\rm Q}(5) \right] | ||
| + | \hspace{0.05cm}.$$ | ||
| + | |||
| + | *Because of ${\rm Q}(–2) + {\rm Q}(2) = 1$ and ${\rm Q}(5) \approx 0$ we obtain $p_{\rm SE} \approx 25.5\%$. | ||
| + | |||
| + | *The same applies to the DFE receiver: | ||
| + | :$$p_{\rm DFE } \ = \ {1}/{2} \cdot {\rm Q}\left( \frac{0.3-0.4}{0.2}\right) | ||
| + | + {1}/{2} \cdot{\rm Q}\left( \frac{0.3+0.4}{0.2}\right)= \ {1}/{2} \cdot \left[ {\rm Q}(-0.5) + {\rm Q}(3.5) | ||
| + | \right] \approx\frac{1- {\rm Q}(0.5)}{2}\hspace{0.15cm}\underline {= 35\%} | ||
| + | \hspace{0.05cm}.$$ | ||
| + | |||
| + | *In contrast, the error probability $p_{\rm ML}$ of a maximum likelihood receiver is still ${\rm Q}(2) \hspace{0.15cm} \underline {= 2.27\%}$. | ||
| + | |||
| + | *The order of the basic detection pulse values is (almost) irrelevant for the error probability of the Viterbi receiver. | ||
{{ML-Fuß}} | {{ML-Fuß}} | ||
| − | [[Category: | + | [[Category:Digital Signal Transmission: Exercises|^3.8 Viterbi Receiver^]] |
Latest revision as of 13:21, 13 July 2022
Bitte diese Aufgabe sehr genau kontrollieren. Da habe ich etliche Änderungen vorgenommen.
Error probabilities of different receiver types are to be compared. Considered are:
- Threshold Decision $($German: "Schwellenwertentscheidung" ⇒ "$\rm SE$"$)$ ⇒ error probability $p_{\rm SE}$,
- Decision Feedback Equalization $\rm (DFE)$ ⇒ error probability $p_{\rm DFE}$ and
- Maximum Likelihood Detection $\rm (ML)$ ⇒ error probability $p_{\rm ML}$.
In the table given are four different parameter sets $\rm A$, $\rm B$, $\rm C$ and $\rm D$:
- The "main value" $g_0$ of the basic detection pulse,
- the "precursor" $g_{\rm –1}$,
- the "postcursor" (trailer) $g_1$,
- the rms value $\sigma_d$ of the detection noise component $d_{\rm N}(t)$ before the respective decision.
Bipolar amplitude coefficients are assumed, so that, for example, for the worst-case error probability $($German: "ungünstigste Fehlerwahrscheinlichkeit" ⇒ "$\rm U$"$)$ of the receiver with threshold decision, the following applies:
- $$p_{\rm U,\hspace{0.15cm} SE } = \left\{ \begin{array}{c} {\rm Q}\big [ ({g_0-|g_{-1}|-|g_{1}|})/{\sigma_d} \big ]\\ \\{\rm Q}(0) = 0.5 \end{array} \right.\quad \begin{array}{*{1}c} {\rm with }\hspace{0.15cm}{\rm open }\hspace{0.15cm}{\rm eye }, \\ \\{\rm with }\hspace{0.15cm}{\rm closed }\hspace{0.15cm}{\rm eye }. \\ \end{array}\begin{array}{*{20}c} \\ \end{array}$$
For the Nyquist system $\rm A$, the mean error probability is exactly the same, viz.
- $$p_{\rm SE } =p_{\rm U,\hspace{0.15cm} SE } = {\rm Q}\left( {g_0}/{\sigma_d} \right)= {\rm Q}(5) \approx 2.87 \cdot 10^{-7}\hspace{0.05cm}.$$
For the other system variants $\rm B$, $\rm C$ and $\rm D$ considered here, the intersymbol interferences are so strong and the given noise rms value is so small that the following approximation can be applied:
- $$p_{\rm SE } \approx {1}/{4} \cdot p_{\rm U,\hspace{0.1cm} SE } = {1}/{4} \cdot {\rm Q}\left( \frac {{\rm Max }\hspace{0.05cm}\big [0, \hspace{0.05cm}g_0-|g_{-1}|-|g_{1}|\big ]}{\sigma_d} \right)\hspace{0.05cm}.$$
Except for the Nyquist system $\rm A$ $($here $p_{\rm DFE} = p_{\rm SE})$, the following approximation applies to the DFE receiver instead:
- $$p_{\rm DFE } \approx {1}/{2} \cdot p_{\rm U,\hspace{0.1cm} DFE } = {1}/{2} \cdot {\rm Q}\left( \frac{{\rm Max }\hspace{0.05cm}\big [0, \hspace{0.05cm}g_0-|g_{-1}|\big ]}{\sigma_d} \right)\hspace{0.05cm}.$$
In contrast, it was shown in the "last theory section" for this chapter that for a receiver with ML decision, the following approximation holds:
- $$p_{\rm ML } = {\rm Q}\left( \frac{{\rm Max }\hspace{0.05cm}[g_{\nu}]}{\sigma_d} \right)\hspace{0.05cm}.$$
Notes:
- The exercise belongs to the chapter "Viterbi Receiver".
- Reference is also made to the chapters "Linear Nyquist Equalization" and "Decision Feedback".
- You can determine the numerical values of the Q-function using the interaction module "Complementary Gaussian Error Functions".
- To apply the algorithm given in the theory section for two precursors, you would have to make the following renamings
$($which, however, has no meaning for the calculation of the error probabilities$)$:
- $$g_{1 }\hspace{0.1cm}\Rightarrow \hspace{0.1cm}g_{0 },\hspace{0.4cm} g_{0 }\hspace{0.1cm}\Rightarrow \hspace{0.1cm}g_{-1 },\hspace{0.4cm} g_{-1 }\hspace{0.1cm}\Rightarrow \hspace{0.1cm}g_{-2 } \hspace{0.05cm}.$$
Questions
Solution
- $$ p_{\rm DFE } = p_{\rm ML } = p_{\rm SE } \hspace{0.15cm}\underline {\approx 2.87 \cdot 10^{-7}} \hspace{0.05cm}.$$
(2) With $g_0 = 0.6$, $g_{\rm –1} = 0.1$ and $g_1 = 0.3$, $\text{(system B)}$, one obtains approximately:
- $$p_{\rm SE } \ \approx \ {1}/{4} \cdot {\rm Q}\left( \frac{0.6-0.1-0.3}{0.2} \right)= {1}/{4} \cdot{\rm Q}(1) \hspace{0.15cm}\underline {\approx 4\% \hspace{0.05cm}},$$
- $$ p_{\rm DFE } \ \approx \ {1}/{2} \cdot {\rm Q}\left( \frac{0.6-0.1}{0.2} \right)= {1}/{2} \cdot {\rm Q}(2.5) \hspace{0.15cm}\underline {\approx 0.31\%} \hspace{0.05cm},$$
- $$ p_{\rm ML } \ \approx \ {\rm Q}\left( \frac{0.6}{0.2} \right) = {\rm Q}(3) \hspace{0.15cm}\underline {\approx 0.135\%} \hspace{0.05cm}.$$
(3) With $g_0 = 0.4$ and $g_1 = g_{\rm –1} = 0.3$ $\text{(system C)}$, one obtains approximately:
- $$p_{\rm SE } \ \approx \ {1}/{4} \cdot{\rm Q}(0) \hspace{0.15cm}\underline {= 12.5\%} \hspace{0.3cm}\Rightarrow \hspace{0.3cm}{\rm closed }\hspace{0.15cm}{\rm eye } \hspace{0.05cm},$$
- $$ p_{\rm DFE } \ \approx \ {1}/{2} \cdot {\rm Q}\left( \frac{0.4-0.3}{0.2} \right)= {1}/{2} \cdot {\rm Q}(0.5) \hspace{0.15cm}\underline {\approx 15\% \hspace{0.05cm}},$$
- $$ p_{\rm ML } \ \approx \ {\rm Q}\left( \frac{0.4}{0.2} \right) = {\rm Q}(2) \hspace{0.15cm}\underline {\approx 2.27\%} \hspace{0.05cm}.$$
- Interesting is – and not a calculation error – that the DFE is worse than the conventional threshold decision when the error probability is $10\%$ or more.
- See also the solution for subtask (4).
(4) With system $\text{D}$, the DFE receiver also has a closed eye.
- $p_{\rm DFE}$ is greater than $p_{\rm SE}$, since the worst-case symbol sequence now occurs more frequently. According to the given simple approximation holds:
- $$p_{\rm SE } = {1}/{4} \cdot{\rm Q}(0) = 0.125\hspace{0.05cm}, \hspace{0.2cm} p_{\rm DFE } = {1}/{2} \cdot{\rm Q}(0) \hspace{0.15cm}\underline {= 0.250} \hspace{0.05cm}.$$
- On the other hand, with an exact calculation one obtains:
- $$p_{\rm SE } \ = \ {1}/{4} \cdot {\rm Q}\left( \frac{0.3-0.4-0.3}{0.2}\right) + {1}/{4} \cdot{\rm Q}\left( \frac{0.3-0.4+0.3}{0.2}\right)+ \ {1}/{4} \cdot {\rm Q}\left( \frac{0.3+0.4-0.3}{0.2}\right) +{1}/{4} \cdot{\rm Q}\left( \frac{0.3+0.4+0.3}{0.2}\right)$$
- $$ \Rightarrow \hspace{0.3cm}p_{\rm SE } \ = \ {1}/{4} \cdot \left[ {\rm Q}(-2) + {\rm Q}(1) +{\rm Q}(2) +{\rm Q}(5) \right] ={1}/{4} \cdot \left[ 1+ {\rm Q}(1) +{\rm Q}(5) \right] \hspace{0.05cm}.$$
- Because of ${\rm Q}(–2) + {\rm Q}(2) = 1$ and ${\rm Q}(5) \approx 0$ we obtain $p_{\rm SE} \approx 25.5\%$.
- The same applies to the DFE receiver:
- $$p_{\rm DFE } \ = \ {1}/{2} \cdot {\rm Q}\left( \frac{0.3-0.4}{0.2}\right) + {1}/{2} \cdot{\rm Q}\left( \frac{0.3+0.4}{0.2}\right)= \ {1}/{2} \cdot \left[ {\rm Q}(-0.5) + {\rm Q}(3.5) \right] \approx\frac{1- {\rm Q}(0.5)}{2}\hspace{0.15cm}\underline {= 35\%} \hspace{0.05cm}.$$
- In contrast, the error probability $p_{\rm ML}$ of a maximum likelihood receiver is still ${\rm Q}(2) \hspace{0.15cm} \underline {= 2.27\%}$.
- The order of the basic detection pulse values is (almost) irrelevant for the error probability of the Viterbi receiver.