Aufgaben:Exercise 3.1: Spectrum of the Exponential Pulse

• Return to book

Exponentialimpuls

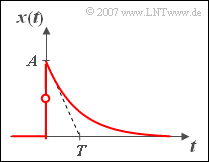
In this task, a causal signal  $x(t)$ is considered

• which rises abruptly from zero to  $A$  at time  $t = 0$ , and
• decreases exponentially with the time constant  $T$  for  $t > 0$ :

$$x(t) = A \cdot {\rm e}^{ - t/T} .$$

At the saltus at time  $t = 0$ ,   $x(t = 0) = A/2$.

Use the following parameters for the numerical calculations:

$$A = 3 \hspace{0.1cm} {\rm V}, \hspace{0.4cm} T = 1 \hspace{0.1cm} {\rm ms} .$$

The spectral function  $X(f)$  to be calculated will be complex and therefore can be

• by real and imaginary part, but also
• by magnitude and phase

can be represented. Use the notation

$$X( f ) = \left| {X( f )} \right| \cdot {\rm e}^{ - {\rm j} \hspace{0.05cm}\cdot \hspace{0.05cm} \varphi( f )} .$$

Hints:

• This exercise belongs to the chapter  Fourier Transform and Its Inverse.

Questions

1

Calculate the spectral function  $X(f)$. What spectral value results at the frequency  $f = 0$?

$\text{Re}[X(f=0)] \ = \ $  $\rm mV/Hz$

$\text{Im}[X(f=0)] \ = \ $  $\rm mV/Hz$

2

What are the real and imaginary parts of  $X(f)$  using  $f_0 = 1/(2\pi T)$. What are the values when  $f = f_0$?

$\text{Re}[X(f=f_0)] \ = \ $  $\rm mV/Hz$

$\text{Im}[X(f=f_0)] \ = \ $  $\rm mV/Hz$

3

Calculate the magnitude function  $|X(f)|$. Which values result at the frequency  $f = f_0$  and at very high frequencies?

$|X(f=f_0)| \hspace{0.25cm} = \ $  $\rm mV/Hz$

$|X(f\rightarrow \infty)| \ = \ $  $\rm mV/Hz$

4

Calculate the phase function  $\varphi(f)$. What values result for this at the frequency  $f = f_0$  and at very high frequencies?

$\varphi(f=f_0) \hspace{0.25cm} = \ $  $\rm rad$

$\varphi(f \rightarrow \infty) \ = \ $  $\rm rad$

Solution

Solution

(1)  With the first Fourier integral we get:

$$X( f ) = \int_0^\infty {A \cdot {\rm e}^{ - t\left( {1/T + {\rm j \hspace{0.05cm} \cdot \hspace{0.05cm}}2\pi f} \right)} } {\rm d}t = \left. {\frac{ { - A}}{ {1/T + {\rm j}2\pi f}} \cdot {\rm e}^{ - t\left( {1/T + {\rm j}2\pi f} \right)} } \right|_0^\infty .$$

• The upper integral limit  $(t \rightarrow \infty)$  gives zero, the lower limit  $(t = 0)$  gives the value  $1$. Thus:

$$X(f) = \frac{ {A \cdot T}}{ {1 + {\rm j}2\pi fT}}\hspace{0.3 cm}\Rightarrow\hspace{0.3 cm} X( {f = 0}) = A \cdot T{ = 3 \cdot 10^{ - 3}\; {\rm V/Hz}} \hspace{0.15 cm}\underline{ = 3 \; {\rm mV/Hz}}.$$

• At the frequency  $f = 0$ , the spectrum is therefore purely real:

$$\text{Re}[X(f=0)] \hspace{0.15 cm}\underline{ = 3 \; {\rm mV/Hz}}  \hspace{1.15 cm}\text{Im}[X(f=0)] \hspace{0.15 cm}\underline{ =0}.$$

(2)  With the abbreviations  $X_0 = A \cdot T$  and  $f_0 = 1/(2\pi T)$  the spectral function is:

$$X( f) = \frac{ {X_0 }}{ {1 +{\rm j} \cdot f/f_0 }} = \frac{ {X_0 }}{ {1 + \left( {f/f_0 } \right)^2 }} \cdot \left( {1 - {\rm j} \cdot f/f_0 } \right).$$

Divided into real and imaginary parts, this gives:

$${\mathop{\rm Re}\nolimits} [ {X(f)}] = \frac{ {X_0 }}{{1 + \left( {f/f_0 } \right)^2 }}, \hspace{0.5 cm}{\mathop{\rm Im}\nolimits} [ {X(f)}] = - \frac{ {X_0 \cdot f/f_0 }}{ {1 + \left( {f/f_0 } \right)^2 }}.$$

At the frequency  $f_0$ 

• the real part is equal to  $X_0/2 \hspace{0.15 cm}\underline{ = 1.5 \; {\rm mV/Hz}},$
• the imaginary part is equal to  $–X_0/2 \hspace{0.15 cm}\underline{ = \hspace{0.1 cm}-1.5 \; {\rm mV/Hz}}.$

Betragsspektrum des Exponentialimpulses

(3)  The magnitude of a complex-valued function, which is a quotient, is equal to the quotient of the magnitudes of the numerator and denominator.

• Thus one obtains:

$$ \left| {X( f)} \right| =\frac{ {X_0 }}{ {\left| 1 +{\rm j} \cdot f/ {f_0 } \right|}} = \frac{ {X_0 }}{{\sqrt {1 + \left( {f/f_0 } \right)^2 } }},$$

$$\Rightarrow \hspace{0.5 cm} \left| {X( {f = f_0} )} \right| = { {X_0 }}/{ {\sqrt 2 }}\hspace{0.15 cm}\underline{ = 2.12 \;{\rm mV/Hz}}.$$

• At very high frequencies  $(f \rightarrow \infty)$  the magnitude isalmost zero (see sketch).

(4)  The general rule for the phase function is:

$$\varphi ( f ) = \arctan \left( {\frac{ { - {\mathop{\rm Im}\nolimits}[{X(f)} ]}}{{ {\mathop{\rm Re}\nolimits} [ {X(f)} ]}}} \right) = \arctan \left( {f/f_0 } \right).$$

• For $f = f_0$ this gives  $\arctan(1)= \pi /4 \hspace{0.15 cm}\underline{\approx 0.785}$.
• For very large values of  $f$  the phase function approaches  $\arctan(\infty) = \pi /2 \hspace{0.15 cm}\underline{ \approx 1.571}$  an.
• Both specifications are to be understood in radians.