Difference between revisions of "Aufgaben:Exercise 4.11Z: OOK and BPSK once again"
| Line 1: | Line 1: | ||
| − | {{quiz-Header|Buchseite= | + | {{quiz-Header|Buchseite=Digital_Signal_Transmission/Carrier_Frequency_Systems_with_Coherent_Demodulation}} |
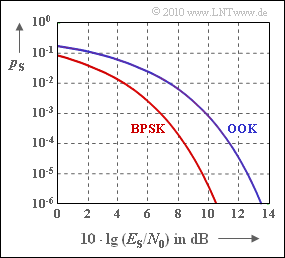
| − | [[File:P_ID2061__Dig_Z_4_11.png|right|frame| | + | [[File:P_ID2061__Dig_Z_4_11.png|right|frame|Error probabilities of <i>On–Off Keying</i> and <i>Binary Phase Shift Keying</i>]] |
| − | + | The error probabilities $p_{\rm S}$ of the digital modulation methods <i>On–Off Keying</i> (OOK) and <i>Binary Phase Shift Keying</i> (BPSK) are given here without derivation. For example, one obtains with the so-called Q function | |
:$${\rm Q} (x) = \frac{\rm 1}{\sqrt{\rm 2\pi}}\cdot \int_{\it | :$${\rm Q} (x) = \frac{\rm 1}{\sqrt{\rm 2\pi}}\cdot \int_{\it | ||
x}^{+\infty}\rm e^{\it -u^{\rm 2}/\rm 2}\,d \it u$$ | x}^{+\infty}\rm e^{\it -u^{\rm 2}/\rm 2}\,d \it u$$ | ||
| − | + | for the AWGN channel – identified by $E_{\rm S}/N_0$ – and other optimal conditions (e.g. coherent demodulation) | |
| − | * | + | * for <i>On–Off Keying</i> (OOK), often also called <i>Amplitude Shift Keying</i> (2–ASK): |
:$$p_{\rm S} = {\rm Q}\left ( \sqrt{{E_{\rm S}}/{N_0 }} \hspace{0.1cm}\right | :$$p_{\rm S} = {\rm Q}\left ( \sqrt{{E_{\rm S}}/{N_0 }} \hspace{0.1cm}\right | ||
) \hspace{0.05cm},$$ | ) \hspace{0.05cm},$$ | ||
| Line 16: | Line 16: | ||
| − | + | These symbol error probabilities (at the same time the bit error probabilities) are shown in the graph. | |
| − | + | For example, for $10 \cdot {\rm lg} \, E_{\rm S}/N_0 = 10 \ \rm dB$ one obtains according to the exact functions: | |
:$$p_{\rm S} = 7.83 \cdot 10^{-4}\,\,{\rm (OOK)}\hspace{0.05cm},\hspace{0.5cm} | :$$p_{\rm S} = 7.83 \cdot 10^{-4}\,\,{\rm (OOK)}\hspace{0.05cm},\hspace{0.5cm} | ||
p_{\rm S} = 3.87 \cdot 10^{-6}\,\,{\rm (BPSK)}\hspace{0.05cm}.$$ | p_{\rm S} = 3.87 \cdot 10^{-6}\,\,{\rm (BPSK)}\hspace{0.05cm}.$$ | ||
| − | + | In order to achieve $p_{\rm S} = 10^{\rm -5}$ with BPSK, $10 \cdot {\rm lg} \, E_{\rm S}/N_0 ≥ 9.6 \ \rm dB$ must hold. | |
| Line 28: | Line 28: | ||
| − | '' | + | ''Notes:'' |
| − | * | + | * The exercise belongs to the chapter [[Digital_Signal_Transmission/Carrier_Frequency_Systems_with_Coherent_Demodulation|"Carrier Frequency Systems with Coherent Demodulation"]]. |
| − | * | + | * You can also find the derivations in the chapter [[Digital_Signal_Transmission/Linear_Digital_Modulation_-_Coherent_Demodulation|"Linear Digital Modulation – Coherent Demodulation"]]. |
| − | * | + | * For the complementary Gaussian error function, use the following upper bound: |
:$${\rm Q}(x) \approx \frac{1}{\sqrt{2\pi} \cdot x} \cdot {\rm e}^{-x^2/2} | :$${\rm Q}(x) \approx \frac{1}{\sqrt{2\pi} \cdot x} \cdot {\rm e}^{-x^2/2} | ||
\hspace{0.05cm}.$$ | \hspace{0.05cm}.$$ | ||
| Line 38: | Line 38: | ||
| − | === | + | ===Questions=== |
<quiz display=simple> | <quiz display=simple> | ||
| − | { | + | {Calculate the '''OOK''' symbol error probability for $10 \cdot {\rm lg} \, E_{\rm S}/N_0 = 10 \ \rm dB$ using the upper bound. |
|type="{}"} | |type="{}"} | ||
$p_{\rm S}\ = \ $ { 85 3% } $\ \cdot 10^{\rm –5}$ | $p_{\rm S}\ = \ $ { 85 3% } $\ \cdot 10^{\rm –5}$ | ||
| − | { | + | {What is the '''BPSK''' symbol error probability for $10 \cdot {\rm lg} \, E_{\rm S}/N_0 = 10 \ \rm dB$? |
|type="{}"} | |type="{}"} | ||
$p_{\rm S}\ = \ $ { 0.405 3% } $\ \cdot 10^{\rm –5}$ | $p_{\rm S}\ = \ $ { 0.405 3% } $\ \cdot 10^{\rm –5}$ | ||
| − | { | + | {For '''OOK''', give the minimum value of $E_{\rm S}/N_0$ $($in $\rm dB)$ required for $p_{\rm S} = 10^{\rm -5}$. |
|type="{}"} | |type="{}"} | ||
${\rm Minimum} \big[10 \cdot {\rm lg} \, E_{\rm S}/N_0 \big ] \ = \ $ { 12.6 3% } $\ \rm dB$ | ${\rm Minimum} \big[10 \cdot {\rm lg} \, E_{\rm S}/N_0 \big ] \ = \ $ { 12.6 3% } $\ \rm dB$ | ||
</quiz> | </quiz> | ||
| − | === | + | ===Solution=== |
{{ML-Kopf}} | {{ML-Kopf}} | ||
| − | '''(1)''' | + | '''(1)''' From $10 \cdot {\rm lg} \, E_{\rm S}/N_0 = 10 \ \rm dB$ it follows that $E_{\rm S}/N_0 = 10$ and thus |
:$$p_{\rm S} = {\rm Q}\left ( \sqrt{10} \right ) \approx | :$$p_{\rm S} = {\rm Q}\left ( \sqrt{10} \right ) \approx | ||
\frac{\rm 1}{\sqrt{\rm 20\pi} }\cdot \rm e^{-5 } \underline{=85 \cdot 10^{-5}}\hspace{0.05cm}.$$ | \frac{\rm 1}{\sqrt{\rm 20\pi} }\cdot \rm e^{-5 } \underline{=85 \cdot 10^{-5}}\hspace{0.05cm}.$$ | ||
| − | * | + | *The actual value according to the data section is $78.3 \cdot 10^{\rm -5}$. |
| − | * | + | *So the given equation is actually an upper bound for ${\rm Q}(x)$. |
| − | * | + | *The relative error when using this approximation instead of the exact function ${\rm Q}(x)$ is less than $10\%$ in this case. |
| − | '''(2)''' | + | '''(2)''' For BPSK, the corresponding equation is: |
:$$p_{\rm S} = {\rm Q}\left ( \sqrt{20} \right ) \approx | :$$p_{\rm S} = {\rm Q}\left ( \sqrt{20} \right ) \approx | ||
\frac{\rm 1}{\sqrt{\rm 40\pi} }\cdot \rm e^{-10 } \underline{=0.405 \cdot 10^{-5}}\hspace{0.05cm}.$$ | \frac{\rm 1}{\sqrt{\rm 40\pi} }\cdot \rm e^{-10 } \underline{=0.405 \cdot 10^{-5}}\hspace{0.05cm}.$$ | ||
| − | * | + | *Now the relative error using the approximation is only $5\%$. |
| − | * | + | *In general: The smaller the error probability, the better the approximation. |
| − | '''(3)''' | + | '''(3)''' According to the specification, a (logarithmic) value of $9.6 \ \rm dB$ is required for BPSK. |
| − | * | + | *With the OOK, the logarithmic value must be increased by about $3 \ \rm dB$ ⇒ $10 \cdot {\rm lg} \, E_{\rm S}/N_0 \ \underline {\approx 12.6 \ \rm dB}$. |
{{ML-Fuß}} | {{ML-Fuß}} | ||
Revision as of 15:58, 19 July 2022
The error probabilities $p_{\rm S}$ of the digital modulation methods On–Off Keying (OOK) and Binary Phase Shift Keying (BPSK) are given here without derivation. For example, one obtains with the so-called Q function
- $${\rm Q} (x) = \frac{\rm 1}{\sqrt{\rm 2\pi}}\cdot \int_{\it x}^{+\infty}\rm e^{\it -u^{\rm 2}/\rm 2}\,d \it u$$
for the AWGN channel – identified by $E_{\rm S}/N_0$ – and other optimal conditions (e.g. coherent demodulation)
- for On–Off Keying (OOK), often also called Amplitude Shift Keying (2–ASK):
- $$p_{\rm S} = {\rm Q}\left ( \sqrt{{E_{\rm S}}/{N_0 }} \hspace{0.1cm}\right ) \hspace{0.05cm},$$
- für Binary Phase Shift Keying (BPSK):
- $$p_{\rm S} = {\rm Q}\left ( \sqrt{{2 \cdot E_{\rm S}}/{N_0 }} \hspace{0.1cm}\right ) \hspace{0.05cm}.$$
These symbol error probabilities (at the same time the bit error probabilities) are shown in the graph.
For example, for $10 \cdot {\rm lg} \, E_{\rm S}/N_0 = 10 \ \rm dB$ one obtains according to the exact functions:
- $$p_{\rm S} = 7.83 \cdot 10^{-4}\,\,{\rm (OOK)}\hspace{0.05cm},\hspace{0.5cm} p_{\rm S} = 3.87 \cdot 10^{-6}\,\,{\rm (BPSK)}\hspace{0.05cm}.$$
In order to achieve $p_{\rm S} = 10^{\rm -5}$ with BPSK, $10 \cdot {\rm lg} \, E_{\rm S}/N_0 ≥ 9.6 \ \rm dB$ must hold.
Notes:
- The exercise belongs to the chapter "Carrier Frequency Systems with Coherent Demodulation".
- You can also find the derivations in the chapter "Linear Digital Modulation – Coherent Demodulation".
- For the complementary Gaussian error function, use the following upper bound:
- $${\rm Q}(x) \approx \frac{1}{\sqrt{2\pi} \cdot x} \cdot {\rm e}^{-x^2/2} \hspace{0.05cm}.$$
Questions
Solution
- $$p_{\rm S} = {\rm Q}\left ( \sqrt{10} \right ) \approx \frac{\rm 1}{\sqrt{\rm 20\pi} }\cdot \rm e^{-5 } \underline{=85 \cdot 10^{-5}}\hspace{0.05cm}.$$
- The actual value according to the data section is $78.3 \cdot 10^{\rm -5}$.
- So the given equation is actually an upper bound for ${\rm Q}(x)$.
- The relative error when using this approximation instead of the exact function ${\rm Q}(x)$ is less than $10\%$ in this case.
(2) For BPSK, the corresponding equation is:
- $$p_{\rm S} = {\rm Q}\left ( \sqrt{20} \right ) \approx \frac{\rm 1}{\sqrt{\rm 40\pi} }\cdot \rm e^{-10 } \underline{=0.405 \cdot 10^{-5}}\hspace{0.05cm}.$$
- Now the relative error using the approximation is only $5\%$.
- In general: The smaller the error probability, the better the approximation.
(3) According to the specification, a (logarithmic) value of $9.6 \ \rm dB$ is required for BPSK.
- With the OOK, the logarithmic value must be increased by about $3 \ \rm dB$ ⇒ $10 \cdot {\rm lg} \, E_{\rm S}/N_0 \ \underline {\approx 12.6 \ \rm dB}$.