Difference between revisions of "Aufgaben:Exercise 4.12: Power-Spectral Density of a Binary Signal"
From LNTwww
m (Text replacement - "Category:Aufgaben zu Stochastische Signaltheorie" to "Category:Theory of Stochastic Signals: Exercises") |
|||
| (6 intermediate revisions by 2 users not shown) | |||
| Line 1: | Line 1: | ||
| − | {{quiz-Header|Buchseite= | + | {{quiz-Header|Buchseite=Theory_of_Stochastic_Signals/Power-Spectral_Density |
}} | }} | ||
| − | [[File:P_ID405__Sto_A_4_12.png|right|300px|frame| | + | [[File:P_ID405__Sto_A_4_12.png|right|300px|frame|Binary square-wave signals]] |
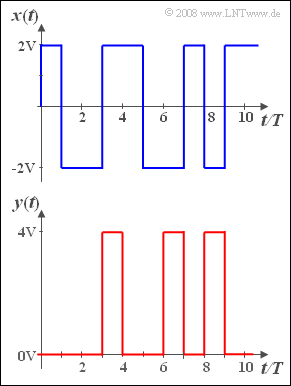
| − | + | We consider a rectangular binary signal $x(t)$ with equal probability amplitude values $+2\hspace{0.05cm}\rm V$ and $-2\hspace{0.05cm}\rm V$. | |
| − | * | + | *The symbol duration is $T = 1 \hspace{0.05cm}\rm µ s$. |
| − | *In [[Aufgaben: | + | *In [[Aufgaben:Exercise_4.10:_Binary_and_Quaternary|Exercise 4.10]] it has already been shown that the associated ACF is restricted to the range of $-T \le \tau\le +T$ and is triangular in this range: |
| − | :$$\varphi_x (\tau) = | + | :$$\varphi_x (\tau) = 4 \hspace{0.05cm}{\rm V}^2 \cdot (1 - | \tau |/{T}).$$ |
| − | * | + | *It is assumed here that the individual symbols are statistically independent of each other. |
| − | + | The signal $y(t)$ sketched below is also binary and rectangular with the same symbol duration $T = 1 \hspace{0.05cm}\rm µ s$. | |
| − | * | + | *But the possible amplitude values are now $0\hspace{0.05cm}\rm V$ and $4\hspace{0.05cm}\rm V$. |
| − | * | + | * The amplitude value $4\hspace{0.05cm}\rm V$ occurs less frequently than $0\hspace{0.05cm}\rm V$. It holds: |
| − | :$${\rm Pr}(x(t) = 4 \hspace{0.05cm} {\rm V}) = p\hspace{0.5cm} {\rm | + | :$${\rm Pr}(x(t) = 4 \hspace{0.05cm} {\rm V}) = p\hspace{0.5cm} {\rm with}\hspace{0.5cm} 0 < p \le 0.25.$$ |
| Line 25: | Line 25: | ||
| − | + | ||
| − | * | + | Hints: |
| + | *This exercise belongs to the chapter [[Theory_of_Stochastic_Signals/Power-Spectral_Density|Power-Spectral Density]]. | ||
| − | * | + | *Note the following Fourier correspondence, where ${\rm \Delta} (t)$ a triangular pulse symmetric about $t= 0$ with ${\rm \Delta} (t= 0) = 1$ and ${\rm \Delta} (t) = 0$ for $|t| \ge T$ denotes: |
| − | :$${\rm \Delta} (t) \hspace{0.3cm} \circ | + | :$${\rm \Delta} (t) \hspace{0.3cm} \circ\!\!\!\!\!\!\!\!\bullet\, \hspace{0.3cm} T \cdot {\rm si}^2 ( \pi f T).$$ |
| − | * | + | *Further, the notation ${\rm si}(x) = \sin(x)/x$ holds with the following integral value: |
:$$\int^1_0 {\rm si}^2 ( \pi \cdot u) \, {\rm d}u \ \approx 0.456.$$ | :$$\int^1_0 {\rm si}^2 ( \pi \cdot u) \, {\rm d}u \ \approx 0.456.$$ | ||
| Line 36: | Line 37: | ||
| − | === | + | ===Questions=== |
<quiz display=simple> | <quiz display=simple> | ||
| − | { | + | {Give the power-spectral density ${\it \Phi}_x(f)$ of the bipolar random signal $x(t)$ ; |
| − | + | What PSD values result für $f= 0$, $f = 500 \hspace{0.05cm}\rm kHz$ and $f = 1 \hspace{0.05cm}\rm MHz$? | |
|type="{}"} | |type="{}"} | ||
${\it \Phi}_x(f = 0) \ = \ $ { 4 3% } $\ \cdot 10^{-6} \ \rm V^2 /Hz$ | ${\it \Phi}_x(f = 0) \ = \ $ { 4 3% } $\ \cdot 10^{-6} \ \rm V^2 /Hz$ | ||
| − | ${\it \Phi}_x(f = 500 \hspace{0.08cm}\rm kHz) \ = | + | ${\it \Phi}_x(f = 500 \hspace{0.08cm}\rm kHz) \ = \ $ { 1.62 3% } $\ \cdot 10^{-6} \ \rm V^2 /Hz$ |
| − | ${\it \Phi}_x(f = 1 \hspace{0.08cm}\rm MHz) \ = | + | ${\it \Phi}_x(f = 1 \hspace{0.08cm}\rm MHz) \ = \ $ { 0. } $\ \cdot 10^{-6} \ \rm V^2 /Hz$ |
| − | { | + | {Calculate the ACF $\varphi_y(\tau)$ of the unipolar random signal $y(t)$. What ACF values result with $p = 0.25$ for $\tau =0$, $\tau =T$ and $\tau =2T$ ? |
|type="{}"} | |type="{}"} | ||
$\varphi_y(\tau = 0) \ = \ $ { 4 3% } $\ \rm V^2$ | $\varphi_y(\tau = 0) \ = \ $ { 4 3% } $\ \rm V^2$ | ||
| Line 54: | Line 55: | ||
| − | { | + | {Calculate the associated power-spectral density ${\it \Phi}_y(f)$. What PSD value results für $f = 500 \hspace{0.05cm}\rm kHz$ ? |
|type="{}"} | |type="{}"} | ||
${\it \Phi}_x(f = 500 \hspace{0.08cm}\rm kHz) \ = \ $ { 1.216 3% } $\ \cdot 10^{-6} \ \rm V^2 /Hz$ | ${\it \Phi}_x(f = 500 \hspace{0.08cm}\rm kHz) \ = \ $ { 1.216 3% } $\ \cdot 10^{-6} \ \rm V^2 /Hz$ | ||
| − | { | + | {What is the average signal power $P_{\rm M}$ $($related to the resistor $1 \hspace{0.08cm}\rm \Omega)$ displayed by a meter that only detects power components up to $1 \hspace{0.05cm}\rm MHz$ ? |
|type="{}"} | |type="{}"} | ||
$P_{\rm M} \ = \ $ { 3.736 3% } $\ \rm V^2$ | $P_{\rm M} \ = \ $ { 3.736 3% } $\ \rm V^2$ | ||
| − | |||
| Line 69: | Line 69: | ||
===Musterlösung=== | ===Musterlösung=== | ||
{{ML-Kopf}} | {{ML-Kopf}} | ||
| − | [[File: P_ID406__Sto_A_4_12_a.png|right|frame| | + | [[File: P_ID406__Sto_A_4_12_a.png|right|frame|Power-spectral density with DC component]] |
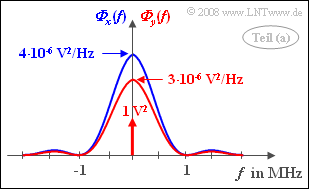
| − | '''(1)''' | + | '''(1)''' The PSD is the Fourier transform of the ACF. |
| − | * | + | *With the Fourier correspondence on the data side and $x_0 = 2\hspace{0.05cm}\rm V$ one obtains: |
:$${\it \Phi}_x(f)= x_{\rm 0}^2 \cdot T \cdot {\rm si}^2(\pi f T).$$ | :$${\it \Phi}_x(f)= x_{\rm 0}^2 \cdot T \cdot {\rm si}^2(\pi f T).$$ | ||
| − | * | + | *The PSD values we are looking for are: |
:$${\it \Phi}_x(f = 0)\hspace{0.15cm}\underline {=4} \cdot 10^{-6} \rm V^2 /Hz,$$ | :$${\it \Phi}_x(f = 0)\hspace{0.15cm}\underline {=4} \cdot 10^{-6} \rm V^2 /Hz,$$ | ||
:$${\it \Phi}_x(f = 500 \hspace{0.05cm}\rm kHz)\hspace{0.15cm}\underline {=1.62} \cdot 10^{-6} \rm V^2 /Hz,$$ | :$${\it \Phi}_x(f = 500 \hspace{0.05cm}\rm kHz)\hspace{0.15cm}\underline {=1.62} \cdot 10^{-6} \rm V^2 /Hz,$$ | ||
:$${\it \Phi}_x(f = 1 \hspace{0.05cm}\rm MHz)\hspace{0.15cm}\underline {=0}.$$ | :$${\it \Phi}_x(f = 1 \hspace{0.05cm}\rm MHz)\hspace{0.15cm}\underline {=0}.$$ | ||
| − | * | + | *At $f = 1 \hspace{0.05cm}\rm MHz $ the power-spectral density has the first zero. |
| − | [[File:P_ID407__Sto_A_4_12_b.png|right|frame| | + | [[File:P_ID407__Sto_A_4_12_b.png|right|frame|Auto-correlation function with DC component ]] |
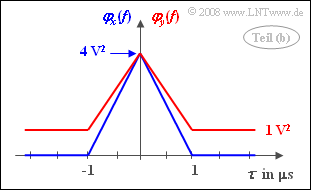
| − | '''(2)''' | + | '''(2)''' Due to the rectangular waveform, the triangular shape of the ACF does not change in principle. |
| − | * | + | *The ACF value at $\tau = 0$ again gives the second order moment. |
| − | * | + | *With $p = 0.25$ one obtains: |
:$$\varphi_y( 0) = {1}/{4}\cdot {(\rm 4\hspace{0.05cm}V)}^2 + {3}/{4}\cdot {(\rm 0\hspace{0.05cm}V)}^2 \hspace{0.15cm}\underline{= {\rm 4\,V^2}}.$$ | :$$\varphi_y( 0) = {1}/{4}\cdot {(\rm 4\hspace{0.05cm}V)}^2 + {3}/{4}\cdot {(\rm 0\hspace{0.05cm}V)}^2 \hspace{0.15cm}\underline{= {\rm 4\,V^2}}.$$ | ||
| − | * | + | *From $\tau =T$ the ACF is constantly equal to $m_y^2$. |
| − | * | + | *With probability $p = 0.25$ and $m_y = p \cdot {\rm 4\hspace{0.05cm}V} + (1-p)\cdot {\rm 0\hspace{0.05cm}V} = 1 \, \rm V$ obtains from $\tau =T$ the constant value $\varphi_y( \tau >T) \hspace{0.15cm}\underline {=\rm 1\,V^2}$. |
| − | '''(3)''' | + | '''(3)''' The ACF can also be represented as follows: |
:$$\varphi_y(\tau) = 1\hspace{0.05cm}{\rm V}^2 + 3\hspace{0.05cm}{\rm V}^2 \cdot \Delta (\tau).$$ | :$$\varphi_y(\tau) = 1\hspace{0.05cm}{\rm V}^2 + 3\hspace{0.05cm}{\rm V}^2 \cdot \Delta (\tau).$$ | ||
| − | * | + | *The ACF DC component $($with $1\hspace{0.05cm}{\rm V}^2)$ leads to a Dirac function in the PSD at $f = 0$ ⇒ see sketch for subtask '''(1)'''. |
| − | * | + | *The triangularförm ACF term causes a continuous PSD component corresponding to the $\rm si^2$-form: |
| − | :$${\it \Phi}_y(f)= 1{\rm V}^2 \cdot {\rm \delta | + | :$${\it \Phi}_y(f)= 1{\rm V}^2 \cdot {\rm \delta } (f) + 3 \cdot 10^{-6}\ {\rm V^2\hspace{-0.1cm}/Hz} \cdot {\rm si}^2(\pi f T).$$ |
| − | + | *For $f = 500 \hspace{0.05cm}\rm kHz$ ⇒ $f \cdot T =0.5$ the PSD value results to ${\underline {1.216} \cdot 10^{-6} \rm V^2 /Hz}$. | |
| − | * | + | '''(4)''' The power can be calculated as an integral over the PSD. |
| − | + | *Taking into account the spectral limitation to $ 1 \hspace{0.05cm}\rm MHz$ one obtains with the substitution $u =f \cdot T$: | |
| − | + | :$$P_{\rm M} = 1{\rm V}^2 + 3 \cdot 10^{-6} {{\rm V^2} /{\rm Hz}} \cdot \int^{\rm 1 MHz}_{-\rm 1 MHz} {\rm si}^2(\pi f T)\hspace{0.1cm}{\rm d}f = 1{\rm V}^2 + 3 V^2 \cdot 2 \cdot \int^{1}_{\rm 0} {\rm si}^2(\pi u)\hspace{0.1cm}{\rm d}u = (1 + 3\cdot 2 \cdot 0.456)\,{\rm V^2} \hspace{0.15cm}\underline{= 3.736 \, {\rm V^2}}. $$ | |
| − | + | *If, on the other hand, all spectral components were covered, the average power would result in $\varphi_y( \tau= 0) = 4 \, {\rm V^2}$. | |
| − | '''(4)''' | ||
| − | * | ||
| − | :$$P_{\rm M} = 1{\rm V}^2 | ||
| − | |||
| − | * | ||
{{ML-Fuß}} | {{ML-Fuß}} | ||
| − | [[Category:Theory of Stochastic Signals: Exercises|^4.5 | + | [[Category:Theory of Stochastic Signals: Exercises|^4.5 Power-Spectral Density^]] |
Latest revision as of 18:16, 7 March 2022
We consider a rectangular binary signal $x(t)$ with equal probability amplitude values $+2\hspace{0.05cm}\rm V$ and $-2\hspace{0.05cm}\rm V$.
- The symbol duration is $T = 1 \hspace{0.05cm}\rm µ s$.
- In Exercise 4.10 it has already been shown that the associated ACF is restricted to the range of $-T \le \tau\le +T$ and is triangular in this range:
- $$\varphi_x (\tau) = 4 \hspace{0.05cm}{\rm V}^2 \cdot (1 - | \tau |/{T}).$$
- It is assumed here that the individual symbols are statistically independent of each other.
The signal $y(t)$ sketched below is also binary and rectangular with the same symbol duration $T = 1 \hspace{0.05cm}\rm µ s$.
- But the possible amplitude values are now $0\hspace{0.05cm}\rm V$ and $4\hspace{0.05cm}\rm V$.
- The amplitude value $4\hspace{0.05cm}\rm V$ occurs less frequently than $0\hspace{0.05cm}\rm V$. It holds:
- $${\rm Pr}(x(t) = 4 \hspace{0.05cm} {\rm V}) = p\hspace{0.5cm} {\rm with}\hspace{0.5cm} 0 < p \le 0.25.$$
Hints:
- This exercise belongs to the chapter Power-Spectral Density.
- Note the following Fourier correspondence, where ${\rm \Delta} (t)$ a triangular pulse symmetric about $t= 0$ with ${\rm \Delta} (t= 0) = 1$ and ${\rm \Delta} (t) = 0$ for $|t| \ge T$ denotes:
- $${\rm \Delta} (t) \hspace{0.3cm} \circ\!\!\!\!\!\!\!\!\bullet\, \hspace{0.3cm} T \cdot {\rm si}^2 ( \pi f T).$$
- Further, the notation ${\rm si}(x) = \sin(x)/x$ holds with the following integral value:
- $$\int^1_0 {\rm si}^2 ( \pi \cdot u) \, {\rm d}u \ \approx 0.456.$$
Questions
Musterlösung
(1) The PSD is the Fourier transform of the ACF.
- With the Fourier correspondence on the data side and $x_0 = 2\hspace{0.05cm}\rm V$ one obtains:
- $${\it \Phi}_x(f)= x_{\rm 0}^2 \cdot T \cdot {\rm si}^2(\pi f T).$$
- The PSD values we are looking for are:
- $${\it \Phi}_x(f = 0)\hspace{0.15cm}\underline {=4} \cdot 10^{-6} \rm V^2 /Hz,$$
- $${\it \Phi}_x(f = 500 \hspace{0.05cm}\rm kHz)\hspace{0.15cm}\underline {=1.62} \cdot 10^{-6} \rm V^2 /Hz,$$
- $${\it \Phi}_x(f = 1 \hspace{0.05cm}\rm MHz)\hspace{0.15cm}\underline {=0}.$$
- At $f = 1 \hspace{0.05cm}\rm MHz $ the power-spectral density has the first zero.
(2) Due to the rectangular waveform, the triangular shape of the ACF does not change in principle.
- The ACF value at $\tau = 0$ again gives the second order moment.
- With $p = 0.25$ one obtains:
- $$\varphi_y( 0) = {1}/{4}\cdot {(\rm 4\hspace{0.05cm}V)}^2 + {3}/{4}\cdot {(\rm 0\hspace{0.05cm}V)}^2 \hspace{0.15cm}\underline{= {\rm 4\,V^2}}.$$
- From $\tau =T$ the ACF is constantly equal to $m_y^2$.
- With probability $p = 0.25$ and $m_y = p \cdot {\rm 4\hspace{0.05cm}V} + (1-p)\cdot {\rm 0\hspace{0.05cm}V} = 1 \, \rm V$ obtains from $\tau =T$ the constant value $\varphi_y( \tau >T) \hspace{0.15cm}\underline {=\rm 1\,V^2}$.
(3) The ACF can also be represented as follows:
- $$\varphi_y(\tau) = 1\hspace{0.05cm}{\rm V}^2 + 3\hspace{0.05cm}{\rm V}^2 \cdot \Delta (\tau).$$
- The ACF DC component $($with $1\hspace{0.05cm}{\rm V}^2)$ leads to a Dirac function in the PSD at $f = 0$ ⇒ see sketch for subtask (1).
- The triangularförm ACF term causes a continuous PSD component corresponding to the $\rm si^2$-form:
- $${\it \Phi}_y(f)= 1{\rm V}^2 \cdot {\rm \delta } (f) + 3 \cdot 10^{-6}\ {\rm V^2\hspace{-0.1cm}/Hz} \cdot {\rm si}^2(\pi f T).$$
- For $f = 500 \hspace{0.05cm}\rm kHz$ ⇒ $f \cdot T =0.5$ the PSD value results to ${\underline {1.216} \cdot 10^{-6} \rm V^2 /Hz}$.
(4) The power can be calculated as an integral over the PSD.
- Taking into account the spectral limitation to $ 1 \hspace{0.05cm}\rm MHz$ one obtains with the substitution $u =f \cdot T$:
- $$P_{\rm M} = 1{\rm V}^2 + 3 \cdot 10^{-6} {{\rm V^2} /{\rm Hz}} \cdot \int^{\rm 1 MHz}_{-\rm 1 MHz} {\rm si}^2(\pi f T)\hspace{0.1cm}{\rm d}f = 1{\rm V}^2 + 3 V^2 \cdot 2 \cdot \int^{1}_{\rm 0} {\rm si}^2(\pi u)\hspace{0.1cm}{\rm d}u = (1 + 3\cdot 2 \cdot 0.456)\,{\rm V^2} \hspace{0.15cm}\underline{= 3.736 \, {\rm V^2}}. $$
- If, on the other hand, all spectral components were covered, the average power would result in $\varphi_y( \tau= 0) = 4 \, {\rm V^2}$.