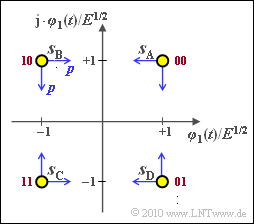
Exercise 4.13: Four-level QAM
We now consider a quadrature amplitude modulation with $M = 4$ symbols and the (normalized) signal space points
- $$\boldsymbol{ s}_{\rm A} = (+1, +1)\hspace{0.05cm},\hspace{0.2cm}\boldsymbol{ s}_{\rm B} = (-1, +1)\hspace{0.05cm},\hspace{0.2cm} \boldsymbol{ s}_{\rm C} = (-1, -1)\hspace{0.05cm},\hspace{0.2cm}\boldsymbol{ s}_{\rm D} = (+1, -1) \hspace{0.05cm}.$$
The symbols are equally probable. Thus, averaging can be omitted to calculate the mean symbol error probability.
For example:
- $$p_{\rm S} = {\rm Pr}({\cal{E}}) = {\rm Pr}( \boldsymbol{ s}_{\rm B} \cup \boldsymbol{ s}_{\rm C} \cup \boldsymbol{ s}_{\rm D} \hspace{0.15cm}{\rm decided} \hspace{0.05cm}|\hspace{0.05cm} \boldsymbol{ s}_{\rm A}\hspace{0.15cm} {\rm sent}) \hspace{0.05cm}.$$
The assignment of the symbols to bit-duples can also be taken from the graphic (red labels). Gray coding is assumed here.
Notes:
- The exercise belongs to the chapter "Carrier Frequency Systems with Coherent Demodulation".
- Reference is made in particular to the section "Quadrature amplitude modulation" (QAM).
- For subtask (4), the (discrete-time) AWGN channel with variance $\sigma_n^2 = N_0/2$ is assumed.
- For the probability that a symbol is falsified horizontally or vertically by the noise signal $n$, with the complementary Gaussian error function $\rm Q(x)$ holds:
- $$p = {\rm Pr}( n < -x_0) = {\rm Pr}( n > + x_0) = {\rm Q}(x_0 / \sigma_n) \hspace{0.05cm}.$$
Questions
Solution
- $$p_{\rm S} \hspace{-0.1cm} \ = \ \hspace{-0.1cm} {\rm Pr}({\cal{E}}) = {\rm Pr}( {\cal{E}} \hspace{0.05cm}|\hspace{0.05cm} \boldsymbol{ s}_{\rm A}\hspace{0.15cm} {\rm sent})= {\rm Pr}( \boldsymbol{ s}_{\rm B} \cup \boldsymbol{ s}_{\rm C} \cup \boldsymbol{ s}_{\rm D} \hspace{0.15cm}{\rm decided} \hspace{0.05cm}|\hspace{0.05cm} \boldsymbol{ s}_{\rm A}\hspace{0.15cm} {\rm sent}) \hspace{0.05cm}.$$
- In contrast, for the (improved) "Union Bound" in the present example:
- $$p_{\rm UB} \hspace{-0.1cm} \ = \ \hspace{-0.1cm} {\rm Pr}( \boldsymbol{ s}_{\rm B} \cup \boldsymbol{ s}_{\rm C} \hspace{0.15cm}{\rm decided} \hspace{0.05cm}|\hspace{0.05cm} \boldsymbol{ s}_{\rm A}\hspace{0.15cm} {\rm sent}) +{\rm Pr}( \boldsymbol{ s}_{\rm C} \cup \boldsymbol{ s}_{\rm D} \hspace{0.15cm}{\rm decided} \hspace{0.05cm}|\hspace{0.05cm} \boldsymbol{ s}_{\rm A}\hspace{0.15cm} {\rm sent}) = 2p = \underline{0.2} \hspace{0.05cm}.$$
(2) The two probabilities that make up the "Union Bound" additive can be interpreted geometrically as follows:
- ${\rm Pr}(\boldsymbol{s}_{\rm B} \cup \boldsymbol{s}_{\rm C} | \boldsymbol{s}_{\rm A})$ iis the probability that the receiving point is located in the left half-plane
⇒ the AWGN noise component $n_1$ is negative and greater in magnitude than $\sqrt {E}$. - ${\rm Pr}(\boldsymbol{s}_{\rm C} \cup \boldsymbol{s}_{\rm D} | \boldsymbol{s}_{\rm A})$ is the probability that the receiving point lies in the lower half-plane
⇒ the AWGN noise component $n_2$ is negative and greater in magnitude than $\sqrt {E}$.
Thus, the "Union Bound" considers the third quadrant twice. It is relatively easy to compensate for this error here:
- $$p_{\rm S} \hspace{-0.1cm} \ = \ \hspace{-0.1cm} p_{\rm UB} - {\rm Pr}( \boldsymbol{ s}_{\rm C} \hspace{0.15cm}{\rm decided} \hspace{0.05cm}|\hspace{0.05cm} \boldsymbol{ s}_{\rm A}\hspace{0.15cm} {\rm sent}) = 2 p - {\rm Pr}\left [ ( n_1 < -\sqrt{E})\cap ( n_2 < -\sqrt{E})\right ] = 2p - p^2 = \underline{0.19} \hspace{0.05cm}.$$
Here it is considered that the noise components $n_1$ and $n_2$ are independent of each other.
(3) Wie in der Teilaufgabe (2) nachgewiesen wurde, gelten für die einzelnen Verfälschungswahrscheinlichkeiten:
- Quadrant 2: ${\rm Pr}(\boldsymbol{s}_{\rm B} \ {\rm empfangen} \ | \ \boldsymbol{s}_{\rm A} \ {\rm gesendet}) = 0.09$,
- Quadrant 3: ${\rm Pr}(\boldsymbol{s}_{\rm C} \ {\rm empfangen} \ | \ \boldsymbol{s}_{\rm A} \ {\rm gesendet}) = 0.01$,
- Quadrant 4: ${\rm Pr}(\boldsymbol{s}_{\rm D} \ {\rm empfangen} \ | \ \boldsymbol{s}_{\rm A} \ {\rm gesendet}) = 0.09$.
Für die mittlere Bitfehlerwahrscheinlichkeit erhält man somit:
- $$p_{\rm B} = { 1}/{ 2} \cdot \big [ 1 \cdot 0.09 + 2 \cdot 0.01 + 1 \cdot 0.09\big ]= \underline{0.1} = p \hspace{0.05cm}.$$
- Berücksichtigt ist, dass der Quadrant 2 und der Quadrant 4 jeweils nur zu einem Bitfehler führt, der Quadrant 3 dagegen zu zweien.
- Der Faktor $1/2$ berücksichtigt wieder, dass jeweils ein Symbol zwei Binärzeichen (Bit) beinhaltet.
(4) Die Bitfehlerwahrscheinlichkeit ist nach der Lösung zur Teilaufgabe (2) gleich der Wahrscheinlichkeit, dass die beiden Rauschkomponenten gewisse Grenzen überschreiten:
- $$p_{\rm B} = {\rm Pr}( n_1 < -\sqrt{E}) = {\rm Pr}( n_2 < -\sqrt{E}) \hspace{0.05cm}.$$
- Beim AWGN–Kanal lautet diese Wahrscheinlichkeit mit der Varianz $\sigma_n^2 = N_0/2$:
- $$p_{\rm B} = {\rm Q} \left ( { { \sqrt{E}}/{ \sigma_n} }\right ) = {\rm Q} \left ( \sqrt{ { {2E}}/{ N_0} }\right ) \hspace{0.05cm}.$$
- Die mittlere Energie pro Symbol kann am einfachsten durch Mittelung über die quadratischen Abstände der Signalraumpunkte vom Ursprung bestimmt werden. Daraus ergibt sich $E_{\rm S} = 2E$.
- Die mittlere Energie pro Bit ist halb so groß: $E_{\rm B} = E_{\rm S}/2 = E$. Daraus folgt:
- $$p_{\rm B} = {\rm Q} \left ( \sqrt{ { {2E_{\rm B}}}/{ N_0} }\right ) \hspace{0.05cm}.$$
- Richtig ist also der zweite Lösungsvorschlag.
- Zum gleichen Ergebnis kommt man auch, wenn man die 4–QAM wie im Kapitel Struktur des optimalen Empfängers des Buches "Modulationsverfahren" als zwei orthogonale (das heißt: sich nicht störende) BPSK–Systeme über den gleichen Kanal betrachtet.