Difference between revisions of "Aufgaben:Exercise 4.14: ACF and CCF for Square Wave Signals"
m (Text replacement - "Category:Aufgaben zu Stochastische Signaltheorie" to "Category:Theory of Stochastic Signals: Exercises") |
|||
| (7 intermediate revisions by 2 users not shown) | |||
| Line 1: | Line 1: | ||
| − | {{quiz-Header|Buchseite= | + | {{quiz-Header|Buchseite=Theory_of_Stochastic_Signals/Cross-Correlation_Function_and_Cross_Power_Density |
}} | }} | ||
| − | [[File:P_ID436__Sto_A_4_14.png|right|framed| | + | [[File:P_ID436__Sto_A_4_14.png|right|framed|ACF and CCF for rectangular signals]] |
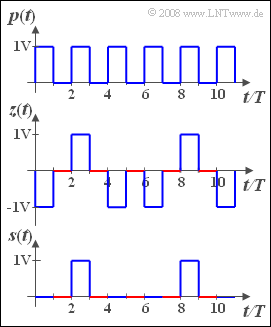
| − | + | We consider a periodic square wave signal $p(t)$ corresponding to the top sketch with the two possible amplitude values $0 \hspace{0.05cm} \rm V$ and $1 \hspace{0.05cm} \rm V$ and the rectangle duration $T$. Thus, the period duration is $T_0 = 2T$. | |
| − | + | Below this is drawn the random signal $z(t)$: | |
| − | + | *This is $z(t)=0 \hspace{0.05cm} \rm V$ between $(2i-1)\cdot T$ and $2i \cdot T$ respectively (highlighted in red in the figure). | |
| − | *In | + | *In the intervals drawn in blue between $2i \cdot T$ and $(2i+1) \cdot T$ the signal value is two-point distributed $(\pm 1 \hspace{0.05cm} \rm V)$. |
| − | + | The probability that in the intervals shown in blue $z(t)=+1 \hspace{0.05cm} \rm V$ holds is generally equal $p$ and independent of the previously selected values. | |
| − | + | The lowest signal in the adjacent graph can be constructed from the first two. It holds: | |
:$$s(t) = {1}/{2} \cdot \big[p(t) + z(t)\big].$$ | :$$s(t) = {1}/{2} \cdot \big[p(t) + z(t)\big].$$ | ||
| − | *In | + | *In the time intervals drawn in red between $(2i-1) \cdot T$ and $2i \cdot T$ $(i$ integer$)$ holds $s(t)=0 \hspace{0.05cm} \rm V$, since here both $p(t)$ and $z(t)$ are equal to zero. |
| − | *In | + | *In the intervening intervals, the amplitude value is two-point distributed between $0 \hspace{0.05cm} \rm V$ and $1 \hspace{0.05cm} \rm V$, where the value $1 \hspace{0.05cm} \rm V$ occurs again with probability $p$. |
| + | *Or in other words: The signals $z(t)$ and $s(t)$ are equivalent pattern signals of the identical random process with bipolar $(-1 \hspace{0.05cm} \rm V, \ +1 \hspace{0.05cm} \rm V)$ resp. unipolar $(0 \hspace{0.05cm} \rm V, \ 1 \hspace{0.05cm} \rm V)$ signal representation. | ||
| − | |||
| Line 26: | Line 26: | ||
| + | Hints: | ||
| + | *The exercise belongs to the chapter [[Theory_of_Stochastic_Signals/Cross-Correlation_Function_and_Cross_Power_Density|Cross-Correlation Function and Cross Power-Spectral Density]]. | ||
| + | *Refer is also made to the chapter [[Theory_of_Stochastic_Signals/Auto-Correlation_Function|Auto-Correlation Function]]. | ||
| + | *Sketch the sought correlation functions in each case in the range from $-7T$ to $+7T$. | ||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | + | ===Questions=== | |
| − | |||
| − | === | ||
<quiz display=simple> | <quiz display=simple> | ||
| − | { | + | {Calculate the ACF $\varphi_z(\tau)$ and sketch it for $p = 0.25$. What values result for $\tau = 0$, $\tau = 3T$ and $\tau = 6T$? |
|type="{}"} | |type="{}"} | ||
$\varphi_z(\tau= 0) \ = \ $ { 0.5 3% } $\ \rm V^2$ | $\varphi_z(\tau= 0) \ = \ $ { 0.5 3% } $\ \rm V^2$ | ||
| − | $\varphi_z(\tau= 3T) \ = | + | $\varphi_z(\tau= 3T) \ = \ $ { 0. } $\ \rm V^2$ |
| − | $\varphi_z(\tau= 6T) \ = | + | $\varphi_z(\tau= 6T) \ = \ $ { 0.125 3% } $\ \rm V^2$ |
| − | { | + | {Now, using the result from '''(1)''' calculate the ACF $\varphi_p(\tau)$. What values result for $\tau = 0$, $\tau = 3T$ and $\tau = 6T$? |
|type="{}"} | |type="{}"} | ||
| − | $\varphi_p(\tau= 0) \ = | + | $\varphi_p(\tau= 0) \ = \ $ { 0.5 3% } $\ \rm V^2$ |
| − | $\varphi_p(\tau= 3T) \ = | + | $\varphi_p(\tau= 3T) \ = \ $ { 0. } $\ \rm V^2$ |
| − | $\varphi_p(\tau= 6T) \ = | + | $\varphi_p(\tau= 6T) \ = \ $ { 0.5 3% } $\ \rm V^2$ |
| − | { | + | {It holds again $p = 0.25$. Calculate the cross-correlation function $\varphi_{pz}(\tau)$ for $\tau = 0$, $\tau = 3T$ and $\tau = 6T$ ? |
|type="{}"} | |type="{}"} | ||
| − | $\varphi_{pz}(\tau= 0) \ = | + | $\varphi_{pz}(\tau= 0) \ = \ $ { -0.26--0.24 } $\ \rm V^2$ |
| − | $\varphi_{pz}(\tau= 3T) \ = | + | $\varphi_{pz}(\tau= 3T) \ = \ $ { 0. } $\ \rm V^2$ |
| − | $\varphi_{pz}(\tau= 6T) \ = | + | $\varphi_{pz}(\tau= 6T) \ = \ $ { -0.26--0.24 } $\ \rm V^2$ |
| − | { | + | {What ACF $\varphi_c(\tau)$ results in general for the sum $c(t) = a(t) + b(t)$ ? |
|type="()"} | |type="()"} | ||
- $\varphi_c(\tau) = \varphi_a(\tau) + \varphi_b(\tau)$. | - $\varphi_c(\tau) = \varphi_a(\tau) + \varphi_b(\tau)$. | ||
| − | + $\varphi_c(\tau) = \varphi_a(\tau) + | + | + $\varphi_c(\tau) = \varphi_a(\tau) + \varphi_{ab}(\tau) + \varphi_{ba}(\tau) + \varphi_b(\tau)$. |
- $\varphi_c(\tau) = \varphi_a(\tau) \star \varphi_b(\tau)$. | - $\varphi_c(\tau) = \varphi_a(\tau) \star \varphi_b(\tau)$. | ||
| − | { | + | {Calculate the ACF $\varphi_s(\tau)$, taking into account the result of '''(4)'''. What values result with $p = 0.25$ for $\tau = 0$, $\tau = 3T$ and $\tau = 6T$ ? |
|type="{}"} | |type="{}"} | ||
| − | $\varphi_s(\tau= 0) \ = | + | $\varphi_s(\tau= 0) \ = \ $ { 0.125 3% } $\ \rm V^2$ |
| − | $\varphi_s(\tau= 3T) \ = | + | $\varphi_s(\tau= 3T) \ = \ $ { 0. } $\ \rm V^2$ |
| − | $\varphi_s(\tau= 6T) \ = | + | $\varphi_s(\tau= 6T) \ = \ $ { -0.03175--0.03075 } $\ \rm V^2$ |
</quiz> | </quiz> | ||
| − | === | + | ===Solution=== |
{{ML-Kopf}} | {{ML-Kopf}} | ||
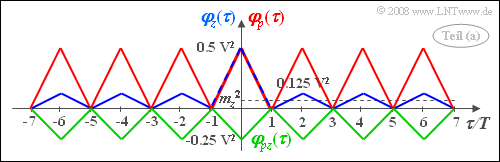
| − | '''(1)''' | + | '''(1)''' The ACF value at $\tau = 0$ gives the average power: |
:$$\varphi_z ( \tau = 0) = {1}/{2} \cdot (1 {\rm V})^2 \hspace{0.15cm}\underline{= 0.5 {\rm V}^2}.$$ | :$$\varphi_z ( \tau = 0) = {1}/{2} \cdot (1 {\rm V})^2 \hspace{0.15cm}\underline{= 0.5 {\rm V}^2}.$$ | ||
| − | * | + | *For $\tau = \pm T$, $\underline{\tau = \pm 3T}$, ... results $\varphi_z ( \tau)\hspace{0.15cm}\underline{ = 0}$. |
| + | *For intermediate values $\tau = \pm 2T$, $\tau = \pm 4T$, $\underline{\tau = \pm 6T}$, ... applies: | ||
| + | :$$\varphi_z ( \tau) = \frac {1 {\rm V}^2}{2} \left(p \hspace{0.02cm} \cdot \hspace{0.02cm}p \hspace{0.2cm} + \hspace{0.2cm}p \hspace{0.02cm}\cdot \hspace{0.02cm}(p-1) \hspace{0.2cm}+\hspace{0.2cm} (p-1)\hspace{0.02cm} \cdot \hspace{0.02cm}p \hspace{0.2cm}+\hspace{0.2cm} (p-1)\hspace{0.02cm} \cdot \hspace{0.02cm}(p-1)\right) = \hspace{0.1cm}\text{...} \hspace{0.1cm}= 0.5\, {\rm V}^2 \cdot (1-2p)^2 .$$ | ||
| − | * | + | *Here $p$ stands for $p \cdot (+1)$ and $(p-1)$ for $(1-p) \cdot (-1)$, i.e. probability times normalized amplitude value, respectively. |
| − | + | [[File:P_ID437__Sto_A_4_14_a.png|frame|right|Auto-correlation functions and cross-correlation function]] | |
| + | *With $p = 0.25$ one gets $\varphi_z ( \tau = \pm 6 T) \hspace{0.15cm}\underline{=0.125 \rm V^2}$. | ||
| − | |||
| − | |||
| − | |||
| − | + | The blue curve shows $\varphi_z(\tau)$ for $p = 0.25$ in the range of $-7T \le \tau \le +7T$: | |
| − | * | + | *Because of the rectangular signal waveform, a sum of triangular functions is obtained. |
| − | * | + | *For $p = 0.5$ the outer (smaller) triangles would disappear. |
<br clear=all> | <br clear=all> | ||
| − | '''(2)''' | + | '''(2)''' The ACF $\varphi_p(\tau)$ of the unipolar periodic signal $p(t)$ is in the generalized representation of '''(1)''' ⇒ ACF $\varphi_z(\tau)$ as a special case for $p = 1$ . |
| − | * | + | *Now one obtains a periodic ACF (see red curve in the above sketch) with |
:$$\varphi_p ( \tau = 0) = \varphi_p ( \tau = \pm 2 T) = \varphi_p ( \tau = \pm 4 T) = \hspace{0.1cm} \text{...} \hspace{0.1cm}\hspace{0.15cm}\underline{= 0.5 {\rm V}^2},$$ | :$$\varphi_p ( \tau = 0) = \varphi_p ( \tau = \pm 2 T) = \varphi_p ( \tau = \pm 4 T) = \hspace{0.1cm} \text{...} \hspace{0.1cm}\hspace{0.15cm}\underline{= 0.5 {\rm V}^2},$$ | ||
:$$\varphi_p ( \tau = \pm T) = \varphi_p ( \tau = \pm 3T) = \hspace{0.1cm} ... \hspace{0.1cm}\hspace{0.15cm}\underline{= 0}.$$ | :$$\varphi_p ( \tau = \pm T) = \varphi_p ( \tau = \pm 3T) = \hspace{0.1cm} ... \hspace{0.1cm}\hspace{0.15cm}\underline{= 0}.$$ | ||
| Line 103: | Line 100: | ||
| − | '''(3)''' | + | '''(3)''' For the cross-correlation function results for $\tau = \pm T$, $\underline{\tau = \pm 3T}$, ... always the value zero. |
| − | * | + | *In contrast, the CCF values for $\tau = \pm 2T$, $\tau = \pm 2T$, ... identical to those for $\tau = 0$: |
| − | :$$\varphi_{pz} ( \tau = 0) | + | :$$\varphi_{pz} ( \tau = 0) = \varphi_{pz} ( \tau = \pm 2 T) = \varphi_{pz} ( \tau = \pm 4 T) = \hspace{0.1cm} \text{...} \hspace{0.1cm}= \frac {1 {\rm V}^2}{2} \left( p - (1-p)\right) = \frac {2p -1}{2}\, {\rm V}^2 .$$ |
| − | * | + | *You get the following results with $p = 0.25$ (see green curve in above sketch): |
:$$\varphi_{pz} ( \tau = 0)\hspace{0.15cm}\underline{= -0.25 {\rm V}^2},\hspace{0.5cm} | :$$\varphi_{pz} ( \tau = 0)\hspace{0.15cm}\underline{= -0.25 {\rm V}^2},\hspace{0.5cm} | ||
\varphi_{pz} ( \tau = 3T)\hspace{0.15cm}\underline{= 0},\hspace{0.5cm} | \varphi_{pz} ( \tau = 3T)\hspace{0.15cm}\underline{= 0},\hspace{0.5cm} | ||
\varphi_{pz} ( \tau = 6T)\hspace{0.15cm}\underline{= -0.25 {\rm V}^2}.$$ | \varphi_{pz} ( \tau = 6T)\hspace{0.15cm}\underline{= -0.25 {\rm V}^2}.$$ | ||
| − | * | + | *With $p = 1$ on the other hand $z(t) \equiv p(t)$ would hold and so of course $\varphi_{pz}(\tau) \equiv \varphi_{p}(\tau) \equiv \varphi_{z}(\tau)$. |
| − | * | + | *For the special case $p = 0.5$ there would be no correlation between $p(t)$ and $z(t)$ and thus $\varphi_{pz}(\tau) \equiv 0$. |
| − | '''(4)''' | + | '''(4)''' Substituting $c(t) = a(t) + b(t)$ into the general ACF definition yields: |
:$$\varphi_c ( \tau ) = \overline{c(t)\hspace{0.02cm} \cdot \hspace{0.02cm} c(t + \tau)} = \overline{a(t)\hspace{0.02cm} \cdot \hspace{0.02cm} a(t + \tau)} \hspace{0.1cm}+\hspace{0.1cm}\overline{a(t)\hspace{0.02cm} \cdot \hspace{0.02cm} b(t + \tau)} +\overline{b(t)\hspace{0.02cm} \cdot \hspace{0.02cm} a(t + \tau)} \hspace{0.1cm}+\hspace{0.1cm}\overline{b(t)\hspace{0.02cm} \cdot \hspace{0.02cm} b(t + \tau)}. $$ | :$$\varphi_c ( \tau ) = \overline{c(t)\hspace{0.02cm} \cdot \hspace{0.02cm} c(t + \tau)} = \overline{a(t)\hspace{0.02cm} \cdot \hspace{0.02cm} a(t + \tau)} \hspace{0.1cm}+\hspace{0.1cm}\overline{a(t)\hspace{0.02cm} \cdot \hspace{0.02cm} b(t + \tau)} +\overline{b(t)\hspace{0.02cm} \cdot \hspace{0.02cm} a(t + \tau)} \hspace{0.1cm}+\hspace{0.1cm}\overline{b(t)\hspace{0.02cm} \cdot \hspace{0.02cm} b(t + \tau)}. $$ | ||
| − | :$$\Rightarrow | + | :$$\Rightarrow \hspace{0.5cm} \varphi_c ( \tau ) = \varphi_{a} ( \tau ) \hspace{0.1cm} + \hspace{0.1cm} \varphi_{ab} ( \tau ) \hspace{0.1cm} + \hspace{0.1cm} \varphi_{ba} ( \tau ) \hspace{0.1cm} + \hspace{0.1cm}\varphi_{a} ( \tau ). $$ |
| − | * | + | *The correct solution is thus the <u>proposed solution 2</u>. |
| − | * | + | *The proposed solution 1 is true only if $a(t)$ and $b(t)$ are uncorrelated. |
| − | * | + | *The last proposition: The convolution operation is always false. |
| − | * | + | *A similar equation would result only if we consider the PDF $f_c(c)$ of the sum $c(t) = a(t) + b(t)$ and $a(t)$ and $b(t)$ are statistically independent: |
| + | :$$f_c (c) = f_a (a) \star f_b (b) .$$ | ||
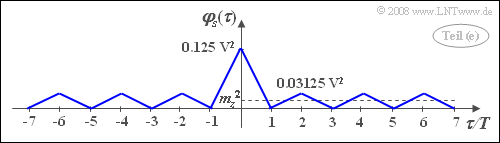
| − | '''(5)''' | + | '''(5)''' Using the result from '''(4)''' and taking into account the factor $1/2$ we get: |
| − | :$$\varphi_s ( \tau ) = {1}/{4} \cdot \big[ \varphi_{p} ( \tau ) \hspace{0.1cm} + \hspace{0.1cm} \varphi_{z} ( \tau ) \hspace{0.1cm} + \hspace{0.1cm} 2 \cdot \varphi_{pz} ( \tau ) | + | :$$\varphi_s ( \tau ) = {1}/{4} \cdot \big[ \varphi_{p} ( \tau ) \hspace{0.1cm} + \hspace{0.1cm} \varphi_{z} ( \tau ) \hspace{0.1cm} + \hspace{0.1cm} 2 \cdot \varphi_{pz} ( \tau ) \big] . $$ |
| − | * | + | *This already takes into account that the CCF between $p(t)$ and $z(t)$ is an even function, so that $\varphi_{pz}(\tau) = \varphi_{zp}(\tau)$ holds. |
| − | * | + | *For $\tau = 0$ one therefore obtains in general with the above results: |
| − | :$$\varphi_s( \tau = 0) = | + | :$$\varphi_s( \tau = 0) = {1}/{4} \cdot \left( 0.5 {\rm V}^2 +0.5 {\rm V}^2 + 2 \cdot \frac{2p-1}{2} {\rm V}^2\right) .$$ |
| − | * | + | *With $p = 0.25$ we get $\varphi_{pz} ( \tau = 0 ) = 0.125\rm V^2$. This result is plausible. On average, only in every eighth interval $s(t)=1 \hspace{0.05cm} \rm V$; otherwise $s(t)=0 \hspace{0.05cm} \rm V$. |
| − | * | + | *For even multiples of $T$ holds: |
| − | :$$ | + | :$$ \varphi_s ( \tau = \pm 2 T) = \varphi_s ( \tau = \pm 4 T) = \hspace{0.1cm} \text{ ...} \hspace{0.1cm} = \frac {0.5 {\rm V}^2}{4} \left( (1-2p)^2 +1 + 2 \cdot (2p -1)\right) = 0.5 \, {\rm V}^2 \hspace{0.02cm} \cdot \hspace{0.02cm} p^2.$$ |
| − | * | + | *With $p = 0.5$ we obtain for this the value $0.03125 \hspace{0.1cm}{\rm V}^2$. All ACF values at odd multiples of $T$ are zero again. |
| − | * | + | *This gives the outlined ACF–curve. |
| − | [[File:P_ID441__Sto_A_4_14_e.png|framed|right| | + | [[File:P_ID441__Sto_A_4_14_e.png|framed|right|ACF of a unipolar rectangular signal]] |
| − | + | Thus, the numerical values we are looking for are: | |
:$$\varphi_{s} ( \tau = 0)\hspace{0.15cm}\underline{= 0.125 {\rm V}^2},$$ | :$$\varphi_{s} ( \tau = 0)\hspace{0.15cm}\underline{= 0.125 {\rm V}^2},$$ | ||
:$$\varphi_{s} ( \tau = 3T)\hspace{0.15cm}\underline{= 0},$$ | :$$\varphi_{s} ( \tau = 3T)\hspace{0.15cm}\underline{= 0},$$ | ||
:$$\varphi_{s} ( \tau = 6T)\hspace{0.15cm}\underline{= -0.03125 {\rm V}^2}.$$ | :$$\varphi_{s} ( \tau = 6T)\hspace{0.15cm}\underline{= -0.03125 {\rm V}^2}.$$ | ||
| − | + | A comparison with the sketch for subtask '''(1)''' shows that the binary signal $s(t)$ has the same ACF as the ternary signal $z(t)$ except for the factor $1/4$. | |
{{ML-Fuß}} | {{ML-Fuß}} | ||
| − | [[Category:Theory of Stochastic Signals: Exercises|^4.6 | + | [[Category:Theory of Stochastic Signals: Exercises|^4.6 CCF and Cross Power-Spectral Density^]] |
Latest revision as of 18:57, 26 March 2022
We consider a periodic square wave signal $p(t)$ corresponding to the top sketch with the two possible amplitude values $0 \hspace{0.05cm} \rm V$ and $1 \hspace{0.05cm} \rm V$ and the rectangle duration $T$. Thus, the period duration is $T_0 = 2T$.
Below this is drawn the random signal $z(t)$:
- This is $z(t)=0 \hspace{0.05cm} \rm V$ between $(2i-1)\cdot T$ and $2i \cdot T$ respectively (highlighted in red in the figure).
- In the intervals drawn in blue between $2i \cdot T$ and $(2i+1) \cdot T$ the signal value is two-point distributed $(\pm 1 \hspace{0.05cm} \rm V)$.
The probability that in the intervals shown in blue $z(t)=+1 \hspace{0.05cm} \rm V$ holds is generally equal $p$ and independent of the previously selected values.
The lowest signal in the adjacent graph can be constructed from the first two. It holds:
- $$s(t) = {1}/{2} \cdot \big[p(t) + z(t)\big].$$
- In the time intervals drawn in red between $(2i-1) \cdot T$ and $2i \cdot T$ $(i$ integer$)$ holds $s(t)=0 \hspace{0.05cm} \rm V$, since here both $p(t)$ and $z(t)$ are equal to zero.
- In the intervening intervals, the amplitude value is two-point distributed between $0 \hspace{0.05cm} \rm V$ and $1 \hspace{0.05cm} \rm V$, where the value $1 \hspace{0.05cm} \rm V$ occurs again with probability $p$.
- Or in other words: The signals $z(t)$ and $s(t)$ are equivalent pattern signals of the identical random process with bipolar $(-1 \hspace{0.05cm} \rm V, \ +1 \hspace{0.05cm} \rm V)$ resp. unipolar $(0 \hspace{0.05cm} \rm V, \ 1 \hspace{0.05cm} \rm V)$ signal representation.
Hints:
- The exercise belongs to the chapter Cross-Correlation Function and Cross Power-Spectral Density.
- Refer is also made to the chapter Auto-Correlation Function.
- Sketch the sought correlation functions in each case in the range from $-7T$ to $+7T$.
Questions
Solution
- $$\varphi_z ( \tau = 0) = {1}/{2} \cdot (1 {\rm V})^2 \hspace{0.15cm}\underline{= 0.5 {\rm V}^2}.$$
- For $\tau = \pm T$, $\underline{\tau = \pm 3T}$, ... results $\varphi_z ( \tau)\hspace{0.15cm}\underline{ = 0}$.
- For intermediate values $\tau = \pm 2T$, $\tau = \pm 4T$, $\underline{\tau = \pm 6T}$, ... applies:
- $$\varphi_z ( \tau) = \frac {1 {\rm V}^2}{2} \left(p \hspace{0.02cm} \cdot \hspace{0.02cm}p \hspace{0.2cm} + \hspace{0.2cm}p \hspace{0.02cm}\cdot \hspace{0.02cm}(p-1) \hspace{0.2cm}+\hspace{0.2cm} (p-1)\hspace{0.02cm} \cdot \hspace{0.02cm}p \hspace{0.2cm}+\hspace{0.2cm} (p-1)\hspace{0.02cm} \cdot \hspace{0.02cm}(p-1)\right) = \hspace{0.1cm}\text{...} \hspace{0.1cm}= 0.5\, {\rm V}^2 \cdot (1-2p)^2 .$$
- Here $p$ stands for $p \cdot (+1)$ and $(p-1)$ for $(1-p) \cdot (-1)$, i.e. probability times normalized amplitude value, respectively.
- With $p = 0.25$ one gets $\varphi_z ( \tau = \pm 6 T) \hspace{0.15cm}\underline{=0.125 \rm V^2}$.
The blue curve shows $\varphi_z(\tau)$ for $p = 0.25$ in the range of $-7T \le \tau \le +7T$:
- Because of the rectangular signal waveform, a sum of triangular functions is obtained.
- For $p = 0.5$ the outer (smaller) triangles would disappear.
(2) The ACF $\varphi_p(\tau)$ of the unipolar periodic signal $p(t)$ is in the generalized representation of (1) ⇒ ACF $\varphi_z(\tau)$ as a special case for $p = 1$ .
- Now one obtains a periodic ACF (see red curve in the above sketch) with
- $$\varphi_p ( \tau = 0) = \varphi_p ( \tau = \pm 2 T) = \varphi_p ( \tau = \pm 4 T) = \hspace{0.1cm} \text{...} \hspace{0.1cm}\hspace{0.15cm}\underline{= 0.5 {\rm V}^2},$$
- $$\varphi_p ( \tau = \pm T) = \varphi_p ( \tau = \pm 3T) = \hspace{0.1cm} ... \hspace{0.1cm}\hspace{0.15cm}\underline{= 0}.$$
(3) For the cross-correlation function results for $\tau = \pm T$, $\underline{\tau = \pm 3T}$, ... always the value zero.
- In contrast, the CCF values for $\tau = \pm 2T$, $\tau = \pm 2T$, ... identical to those for $\tau = 0$:
- $$\varphi_{pz} ( \tau = 0) = \varphi_{pz} ( \tau = \pm 2 T) = \varphi_{pz} ( \tau = \pm 4 T) = \hspace{0.1cm} \text{...} \hspace{0.1cm}= \frac {1 {\rm V}^2}{2} \left( p - (1-p)\right) = \frac {2p -1}{2}\, {\rm V}^2 .$$
- You get the following results with $p = 0.25$ (see green curve in above sketch):
- $$\varphi_{pz} ( \tau = 0)\hspace{0.15cm}\underline{= -0.25 {\rm V}^2},\hspace{0.5cm} \varphi_{pz} ( \tau = 3T)\hspace{0.15cm}\underline{= 0},\hspace{0.5cm} \varphi_{pz} ( \tau = 6T)\hspace{0.15cm}\underline{= -0.25 {\rm V}^2}.$$
- With $p = 1$ on the other hand $z(t) \equiv p(t)$ would hold and so of course $\varphi_{pz}(\tau) \equiv \varphi_{p}(\tau) \equiv \varphi_{z}(\tau)$.
- For the special case $p = 0.5$ there would be no correlation between $p(t)$ and $z(t)$ and thus $\varphi_{pz}(\tau) \equiv 0$.
(4) Substituting $c(t) = a(t) + b(t)$ into the general ACF definition yields:
- $$\varphi_c ( \tau ) = \overline{c(t)\hspace{0.02cm} \cdot \hspace{0.02cm} c(t + \tau)} = \overline{a(t)\hspace{0.02cm} \cdot \hspace{0.02cm} a(t + \tau)} \hspace{0.1cm}+\hspace{0.1cm}\overline{a(t)\hspace{0.02cm} \cdot \hspace{0.02cm} b(t + \tau)} +\overline{b(t)\hspace{0.02cm} \cdot \hspace{0.02cm} a(t + \tau)} \hspace{0.1cm}+\hspace{0.1cm}\overline{b(t)\hspace{0.02cm} \cdot \hspace{0.02cm} b(t + \tau)}. $$
- $$\Rightarrow \hspace{0.5cm} \varphi_c ( \tau ) = \varphi_{a} ( \tau ) \hspace{0.1cm} + \hspace{0.1cm} \varphi_{ab} ( \tau ) \hspace{0.1cm} + \hspace{0.1cm} \varphi_{ba} ( \tau ) \hspace{0.1cm} + \hspace{0.1cm}\varphi_{a} ( \tau ). $$
- The correct solution is thus the proposed solution 2.
- The proposed solution 1 is true only if $a(t)$ and $b(t)$ are uncorrelated.
- The last proposition: The convolution operation is always false.
- A similar equation would result only if we consider the PDF $f_c(c)$ of the sum $c(t) = a(t) + b(t)$ and $a(t)$ and $b(t)$ are statistically independent:
- $$f_c (c) = f_a (a) \star f_b (b) .$$
(5) Using the result from (4) and taking into account the factor $1/2$ we get:
- $$\varphi_s ( \tau ) = {1}/{4} \cdot \big[ \varphi_{p} ( \tau ) \hspace{0.1cm} + \hspace{0.1cm} \varphi_{z} ( \tau ) \hspace{0.1cm} + \hspace{0.1cm} 2 \cdot \varphi_{pz} ( \tau ) \big] . $$
- This already takes into account that the CCF between $p(t)$ and $z(t)$ is an even function, so that $\varphi_{pz}(\tau) = \varphi_{zp}(\tau)$ holds.
- For $\tau = 0$ one therefore obtains in general with the above results:
- $$\varphi_s( \tau = 0) = {1}/{4} \cdot \left( 0.5 {\rm V}^2 +0.5 {\rm V}^2 + 2 \cdot \frac{2p-1}{2} {\rm V}^2\right) .$$
- With $p = 0.25$ we get $\varphi_{pz} ( \tau = 0 ) = 0.125\rm V^2$. This result is plausible. On average, only in every eighth interval $s(t)=1 \hspace{0.05cm} \rm V$; otherwise $s(t)=0 \hspace{0.05cm} \rm V$.
- For even multiples of $T$ holds:
- $$ \varphi_s ( \tau = \pm 2 T) = \varphi_s ( \tau = \pm 4 T) = \hspace{0.1cm} \text{ ...} \hspace{0.1cm} = \frac {0.5 {\rm V}^2}{4} \left( (1-2p)^2 +1 + 2 \cdot (2p -1)\right) = 0.5 \, {\rm V}^2 \hspace{0.02cm} \cdot \hspace{0.02cm} p^2.$$
- With $p = 0.5$ we obtain for this the value $0.03125 \hspace{0.1cm}{\rm V}^2$. All ACF values at odd multiples of $T$ are zero again.
- This gives the outlined ACF–curve.
Thus, the numerical values we are looking for are:
- $$\varphi_{s} ( \tau = 0)\hspace{0.15cm}\underline{= 0.125 {\rm V}^2},$$
- $$\varphi_{s} ( \tau = 3T)\hspace{0.15cm}\underline{= 0},$$
- $$\varphi_{s} ( \tau = 6T)\hspace{0.15cm}\underline{= -0.03125 {\rm V}^2}.$$
A comparison with the sketch for subtask (1) shows that the binary signal $s(t)$ has the same ACF as the ternary signal $z(t)$ except for the factor $1/4$.