Difference between revisions of "Aufgaben:Exercise 5.4: Walsh Functions (PCCF, PACF)"
| Line 69: | Line 69: | ||
===Musterlösung=== | ===Musterlösung=== | ||
{{ML-Kopf}} | {{ML-Kopf}} | ||
| − | '''1 | + | '''(1)''' <u>Alle Vorschläge</u> sind richtig: |
| + | *Die Matrix $ {\mathbf{H}_{4}}$ ist die linke obere Teilmatrix von $ {\mathbf{H}_{8}}$. | ||
| + | *Die Spreizfolgen ergeben sich aus den Zeilen 2, 3 und 4 von $ {\mathbf{H}_{4}}$, und stimmen mit den angegebenen Folgen überein. | ||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | ''' | + | '''(2)''' Richtig sind die <u>Lösungsvorschläge 1, 2 und 3</u>: |
| − | $$\ | + | *Entsprechend den Gleichungen im Angabenteil gilt: |
| − | $$\ | + | :$${\it \varphi}_{12}(\lambda = 0) = 1/4 \cdot \left [ (+1) \cdot (+1) + (-1) \cdot (+1) + (+1) \cdot (-1) + (-1) \cdot (-1) \right ] = 0\hspace{0.05cm},$$ |
| − | + | :$${\it \varphi}_{13}(\lambda = 0) = 1/4\cdot \left [ (+1) \cdot (+1) + (-1) \cdot (-1) + (+1) \cdot (-1) + (-1) \cdot (+1) \right ] = 0\hspace{0.05cm},$$ | |
| − | $$\ | + | :$${\it \varphi}_{23}(\lambda = 0) =1/4 \cdot \left [ (+1) \cdot (+1) + (+1) \cdot (-1) + (-1) \cdot (-1) + (-1) \cdot (+1) \right ] = 0\hspace{0.05cm}.$$ |
| − | + | *Auch für größere Werte von $J$ ist für $i ≠ j$ der PKKF–Wert stets $φ_{ij}(λ = 0)= 0$. | |
| − | + | *Daraus folgt: Bei synchronem CDMA stören sich die Teilnehmer nicht. | |
| − | |||
| − | |||
| − | In der | + | '''(3)''' Richtig sind die <u>Lösungsvorschläge 1 und 2</u>: |
| + | *Für alle Werte von $λ$ ist dieie PKKF $φ_{12}(λ) = 0$, wie die folgenden Zeilen zeigen: | ||
| + | :$$\langle w_\nu^{(1)}\rangle = {+\hspace{-0.05cm}1}\hspace{0.15cm} {-\hspace{-0.05cm}1} \hspace{0.15cm} {+\hspace{-0.05cm}1}\hspace{0.15cm} {-\hspace{-0.05cm}1} \hspace{0.05cm},$$ $$\langle w_\nu^{(2)}\rangle = {+\hspace{-0.05cm}1}\hspace{0.15cm} {+\hspace{-0.05cm}1} \hspace{0.15cm} {-\hspace{-0.05cm}1}\hspace{0.15cm} {-\hspace{-0.05cm}1} \hspace{0.05cm}, \hspace{0.3cm}{\rm Produkt\hspace{0.1cm} mit \hspace{0.1cm}}\langle w_\nu^{(1)}\rangle: {+\hspace{-0.05cm}1}\hspace{0.15cm} {-\hspace{-0.05cm}1} \hspace{0.15cm} {-\hspace{-0.05cm}1}\hspace{0.15cm} {+\hspace{-0.05cm}1} \hspace{0.05cm},$$ | ||
| + | :$$\langle w_{\nu+1}^{(2)}\rangle = {-\hspace{-0.05cm}1}\hspace{0.15cm} {+\hspace{-0.05cm}1} \hspace{0.15cm} {+\hspace{-0.05cm}1}\hspace{0.15cm} {-\hspace{-0.05cm}1} \hspace{0.05cm}, \hspace{0.3cm}{\rm Produkt\hspace{0.1cm} mit \hspace{0.1cm}}\langle w_\nu^{(1)}\rangle: {-\hspace{-0.05cm}1}\hspace{0.15cm} {-\hspace{-0.05cm}1} \hspace{0.15cm} {+\hspace{-0.05cm}1}\hspace{0.15cm} {+\hspace{-0.05cm}1} \hspace{0.05cm},$$ | ||
| + | :$$\langle w_{\nu+2}^{(2)}\rangle = {-\hspace{-0.05cm}1}\hspace{0.15cm} {-\hspace{-0.05cm}1} \hspace{0.15cm} {+\hspace{-0.05cm}1}\hspace{0.15cm} {+\hspace{-0.05cm}1} \hspace{0.05cm},\hspace{0.3cm}{\rm Produkt\hspace{0.1cm} mit \hspace{0.1cm}}\langle w_\nu^{(1)}\rangle: {-\hspace{-0.05cm}1}\hspace{0.15cm} {+\hspace{-0.05cm}1} \hspace{0.15cm} {+\hspace{-0.05cm}1}\hspace{0.15cm} {-\hspace{-0.05cm}1} \hspace{0.05cm},$$ | ||
| + | :$$\langle w_{\nu+3}^{(2)}\rangle = {+\hspace{-0.05cm}1}\hspace{0.15cm} {-\hspace{-0.05cm}1} \hspace{0.15cm} {-\hspace{-0.05cm}1}\hspace{0.15cm} {+\hspace{-0.05cm}1} \hspace{0.05cm},\hspace{0.3cm}{\rm Produkt\hspace{0.1cm} mit \hspace{0.1cm}}\langle w_\nu^{(1)}\rangle: {+\hspace{-0.05cm}1}\hspace{0.15cm} {+\hspace{-0.05cm}1} \hspace{0.15cm} {-\hspace{-0.05cm}1}\hspace{0.15cm} {-\hspace{-0.05cm}1} \hspace{0.05cm},$$ | ||
| + | :$$\langle w_{\nu+4}^{(2)}\rangle = {+\hspace{-0.05cm}1}\hspace{0.15cm} {+\hspace{-0.05cm}1} \hspace{0.15cm} {-\hspace{-0.05cm}1}\hspace{0.15cm} {-\hspace{-0.05cm}1} = \langle w_\nu^{(2)}\rangle \hspace{0.05cm}.$$ | ||
| + | *Das gleiche gilt für die PKKF $φ_{13}(λ)$. | ||
| + | *Dagegen erhält man für die PKKF zwischen den Folgen $ \langle w_\nu^{(2)}\rangle$ und $ \langle w_\nu^{(3)}\rangle$: | ||
| + | [[File:P_ID1890__Mod_A_5_4c.png|right|frame|Verschiedene PKKF– und PAKF–Kurven]] | ||
| + | :$${\it \varphi}_{23}(\lambda ) = \left\{ \begin{array}{c}0 \\+1\\ -1 \\ \end{array} \right. \begin{array}{*{10}c} {\rm{f\ddot{u}r}} \\ {\rm{f\ddot{u}r}} \\ {\rm{f\ddot{u}r}} \\ \end{array} \begin{array}{*{20}c} \lambda = 0, \pm 2, \pm 4,\pm 6, ... \hspace{0.05cm}, \\ \hspace{0.14cm} \lambda = ... \hspace{0.05cm} , -3, +1, +5, ... \hspace{0.05cm}, \\ \hspace{0.14cm} \lambda = ... \hspace{0.05cm} , -5, -1, +3, ... \hspace{0.05cm}. \\ \end{array}$$ | ||
| + | *Das bedeutet: Wird das Signal von Teilnehmer 3 gegenüber Teilnehmer 2 um ein Spreizchip verzögert oder umgekehrt, so lassen sich die Teilnehmer nicht mehr trennen und es kommt zu einer signifikanten Erhöhung der Fehlerwahrscheinlichkeit. | ||
| + | *In der Grafik sind die PKKF–Kurven gestrichelt eingezeichnet (violett und rot). | ||
| − | |||
| − | + | '''(4)''' Richtig sind die <u>Aussagen 1, 2 und 4</u>: | |
| − | '''4 | + | * Da die Walsh–Funktion Nr. 1 periodisch ist mit $T_0 = 2T_c$, ist auch die PAKF periodisch mit $λ = 2$. |
| − | + | *Die zweite Aussage ist richtig, wie die folgende Rechnung zeigt (grüner Kurvenzug): | |
| − | Die zweite Aussage ist richtig, wie die folgende Rechnung zeigt (grüner Kurvenzug): | + | :$${\it \varphi}_{11}(\lambda = 0) = 1/4 \cdot \left [ (+1) \cdot (+1) + (-1) \cdot (-1) + (+1) \cdot (+1) + (-1) \cdot (-1) \right ] = 1\hspace{0.05cm},$$ |
| − | $${\it \varphi}_{11}(\lambda = 0) = | + | :$${\it \varphi}_{11}(\lambda = 1) = 1/4 \cdot \left [ (+1) \cdot (-1) + (+1) \cdot (-1) + (+1) \cdot (-1) + (+1) \cdot (-1) \right ] = -1\hspace{0.05cm}.$$ |
| − | $${\it \varphi}_{11}(\lambda = 1) = | + | *Da sich die beiden Walsh–Funktionen Nr. 2 und 3 nur durch eine Verschiebung um $T_c$ unterscheiden und sich eine Phase in der PAKF prinzipiell nicht auswirkt, ist tatsächlich entsprechend dem letzten Lösungsvorschlag $φ_{33}(λ) = φ_{22}(λ)$. Diese beiden PAKF–Funktionen sind blau eingezeichnet. |
| − | Da sich die beiden Walsh–Funktionen Nr. 2 und 3 nur durch eine Verschiebung um $T_c$ unterscheiden und sich eine Phase in der PAKF prinzipiell nicht auswirkt, ist tatsächlich entsprechend dem letzten Lösungsvorschlag $φ_{33}(λ) = φ_{22}(λ)$. Diese beiden PAKF–Funktionen sind blau eingezeichnet. | + | *Dagegen unterscheidet sich $φ_{22}(λ)$ von $φ_{11}(λ)$ durch eine andere Periodizität: $φ_{22}(λ) = φ_{33}(λ)$ ist doppelt so breit wie $φ_{11}(λ)$. |
| − | |||
| − | Dagegen unterscheidet sich $φ_{22}(λ)$ von $φ_{11}(λ)$ durch eine andere Periodizität: $φ_{22}(λ) = φ_{33}(λ)$ ist doppelt so breit wie $φ_{11}(λ)$. | ||
{{ML-Fuß}} | {{ML-Fuß}} | ||
Revision as of 13:57, 2 August 2017
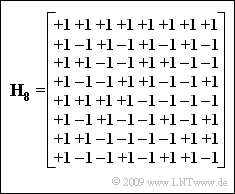
Häufig verwendet man zur Bandspreizung und Bandstauchung so genannte Walsh–Funktionen, die mittels der Hadamard–Matrix konstruiert werden können. Ausgehend von der Matrix
- $${\mathbf{H}_{2}} = \left[ \begin{array}{ccc} +1 & +1 \\ +1 & -1 \end{array} \right] $$
lassen sich durch folgende Rekursiont die weiteren Hadamard–Matrizen $ {\mathbf{H}_{4}}$, $ {\mathbf{H}_{8}}$, usw. herleiten:
- $$ {\mathbf{H}_{2J}} = \left[ \begin{array}{ccc} \mathbf{H}_J & \mathbf{H}_J \\ \mathbf{H}_J & -\mathbf{H}_J \end{array} \right] \hspace{0.05cm}.$$
Die Grafik zeigt die Matrix $ {\mathbf{H}_{8}}$ für den Spreizfaktor $J = 8$. Daraus lassen sich die Spreizfolgen
- $$ \langle w_\nu^{(1)}\rangle = {+\hspace{-0.05cm}1}\hspace{0.15cm} {-\hspace{-0.05cm}1} \hspace{0.15cm} {+\hspace{-0.05cm}1}\hspace{0.15cm} {-\hspace{-0.05cm}1} \hspace{0.15cm}{+\hspace{-0.05cm}1}\hspace{0.15cm} {-\hspace{-0.05cm}1} \hspace{0.15cm}{+\hspace{-0.05cm}1}\hspace{0.15cm} {-\hspace{-0.05cm}1} \hspace{0.05cm},$$
- $$ \langle w_\nu^{(2)}\rangle = {+\hspace{-0.05cm}1}\hspace{0.15cm} {+\hspace{-0.05cm}1} \hspace{0.15cm} {-\hspace{-0.05cm}1}\hspace{0.15cm} {-\hspace{-0.05cm}1} \hspace{0.15cm}{+\hspace{-0.05cm}1}\hspace{0.15cm} {+\hspace{-0.05cm}1} \hspace{0.15cm}{-\hspace{-0.05cm}1}\hspace{0.15cm} {-\hspace{-0.05cm}1} \hspace{0.05cm},$$
- $$...$$
- $$\langle w_\nu^{(7)}\rangle = {+\hspace{-0.05cm}1}\hspace{0.15cm} {-\hspace{-0.05cm}1} \hspace{0.15cm} {-\hspace{-0.05cm}1}\hspace{0.15cm} {+\hspace{-0.05cm}1} \hspace{0.15cm}{+\hspace{-0.05cm}1}\hspace{0.15cm} {-\hspace{-0.05cm}1} \hspace{0.15cm}{-\hspace{-0.05cm}1}\hspace{0.15cm} {+\hspace{-0.05cm}1} \hspace{0.05cm}.$$
für sieben CDMA–Teilnehmer ablesen. Die Spreizfolge $ \langle w_\nu^{(0)}\rangle$ entsprechend der ersten Zeile in der Hadamard–Matrix wird meistens nicht vergeben, da sie nicht spreizt.
Die Fragen beziehen sich meist auf den Spreizfaktor $J = 4$. Damit können entsprechend mit den Spreizfolgen $ \langle w_\nu^{(1)}\rangle$, $ \langle w_\nu^{(2)}\rangle$ und $ \langle w_\nu^{(3)}\rangle$ maximal drei CDMA–Teilnehmer versorgt werden, die sich aus der zweiten, dritten und vierten Zeile der Matrix $ {\mathbf{H}_{4}}$ ergeben.
Hinsichtlich der Korrelationsfunktionen soll in dieser Aufgabe folgende Nomenklatur gelten:
- Die periodische Kreuzkorrelationsfunktion (PKKF) zwischen den Folgen $ \langle w_\nu^{(i)}\rangle$〉 und $ \langle w_\nu^{(j)}\rangle$ wird mit $φ_{ij}(λ)$ bezeichnet. Hierbei gilt:
- $${\it \varphi}_{ij}(\lambda) = {\rm E}\left [ w_{\nu}^{(i)} \cdot w_{\nu+ \lambda}^{(j)} \right ] \hspace{0.05cm}.$$
- Ist die PKKF $φ_{ij} \equiv 0$ (das heißt: $φ_{ij}(λ) = 0$ für alle Werte von $λ$), so stören sich die CDMA–Teilnehmer nicht, auch wenn zwei Teilnehmer unterschiedliche Laufzeiten aufweisen.
- Gilt zumindest $φ_{ij}({\it λ} = 0) = 0$, so kommt es zumindest bei synchronem CDMA–Betrieb (keine oder gleiche Laufzeiten aller Teilnehmer) zu keinen Interferenzen.
- Die periodische Autokorrelationsfunktion (PAKF) der Walsh–Funktion $ \langle w_\nu^{(i)}\rangle$ wird mit $φ_{ii}(λ)$ bezeichnet, und es gilt:
- $${\it \varphi}_{ii}(\lambda) = {\rm E}\left [ w_{\nu}^{(i)} \cdot w_{\nu+ \lambda}^{(i)} \right ] \hspace{0.05cm}.$$
Hinweise:
- Die Aufgabe gehört zum Kapitel Spreizfolgen für CDMA.
- Bezug genommen wird insbesondere auf den Abschnitt Walsh–Funktionen im Theorieteil.
- Wir möchten Sie gerne auch auf das Interaktionsmodul Walsh-Funktionen hinweisen.
- Sollte die Eingabe des Zahlenwertes „0” erforderlich sein, so geben Sie bitte „0.” ein.
- Die Abszisse ist auf die Chipdauer $T_c$ normiert. Das bedeutet, dass $λ = 1$ eigentlich eine Verschiebung um die Verzögerungszeit $τ = T_c$ beschreibt.
Fragebogen
Musterlösung
- Die Matrix $ {\mathbf{H}_{4}}$ ist die linke obere Teilmatrix von $ {\mathbf{H}_{8}}$.
- Die Spreizfolgen ergeben sich aus den Zeilen 2, 3 und 4 von $ {\mathbf{H}_{4}}$, und stimmen mit den angegebenen Folgen überein.
(2) Richtig sind die Lösungsvorschläge 1, 2 und 3:
- Entsprechend den Gleichungen im Angabenteil gilt:
- $${\it \varphi}_{12}(\lambda = 0) = 1/4 \cdot \left [ (+1) \cdot (+1) + (-1) \cdot (+1) + (+1) \cdot (-1) + (-1) \cdot (-1) \right ] = 0\hspace{0.05cm},$$
- $${\it \varphi}_{13}(\lambda = 0) = 1/4\cdot \left [ (+1) \cdot (+1) + (-1) \cdot (-1) + (+1) \cdot (-1) + (-1) \cdot (+1) \right ] = 0\hspace{0.05cm},$$
- $${\it \varphi}_{23}(\lambda = 0) =1/4 \cdot \left [ (+1) \cdot (+1) + (+1) \cdot (-1) + (-1) \cdot (-1) + (-1) \cdot (+1) \right ] = 0\hspace{0.05cm}.$$
- Auch für größere Werte von $J$ ist für $i ≠ j$ der PKKF–Wert stets $φ_{ij}(λ = 0)= 0$.
- Daraus folgt: Bei synchronem CDMA stören sich die Teilnehmer nicht.
(3) Richtig sind die Lösungsvorschläge 1 und 2:
- Für alle Werte von $λ$ ist dieie PKKF $φ_{12}(λ) = 0$, wie die folgenden Zeilen zeigen:
- $$\langle w_\nu^{(1)}\rangle = {+\hspace{-0.05cm}1}\hspace{0.15cm} {-\hspace{-0.05cm}1} \hspace{0.15cm} {+\hspace{-0.05cm}1}\hspace{0.15cm} {-\hspace{-0.05cm}1} \hspace{0.05cm},$$ $$\langle w_\nu^{(2)}\rangle = {+\hspace{-0.05cm}1}\hspace{0.15cm} {+\hspace{-0.05cm}1} \hspace{0.15cm} {-\hspace{-0.05cm}1}\hspace{0.15cm} {-\hspace{-0.05cm}1} \hspace{0.05cm}, \hspace{0.3cm}{\rm Produkt\hspace{0.1cm} mit \hspace{0.1cm}}\langle w_\nu^{(1)}\rangle: {+\hspace{-0.05cm}1}\hspace{0.15cm} {-\hspace{-0.05cm}1} \hspace{0.15cm} {-\hspace{-0.05cm}1}\hspace{0.15cm} {+\hspace{-0.05cm}1} \hspace{0.05cm},$$
- $$\langle w_{\nu+1}^{(2)}\rangle = {-\hspace{-0.05cm}1}\hspace{0.15cm} {+\hspace{-0.05cm}1} \hspace{0.15cm} {+\hspace{-0.05cm}1}\hspace{0.15cm} {-\hspace{-0.05cm}1} \hspace{0.05cm}, \hspace{0.3cm}{\rm Produkt\hspace{0.1cm} mit \hspace{0.1cm}}\langle w_\nu^{(1)}\rangle: {-\hspace{-0.05cm}1}\hspace{0.15cm} {-\hspace{-0.05cm}1} \hspace{0.15cm} {+\hspace{-0.05cm}1}\hspace{0.15cm} {+\hspace{-0.05cm}1} \hspace{0.05cm},$$
- $$\langle w_{\nu+2}^{(2)}\rangle = {-\hspace{-0.05cm}1}\hspace{0.15cm} {-\hspace{-0.05cm}1} \hspace{0.15cm} {+\hspace{-0.05cm}1}\hspace{0.15cm} {+\hspace{-0.05cm}1} \hspace{0.05cm},\hspace{0.3cm}{\rm Produkt\hspace{0.1cm} mit \hspace{0.1cm}}\langle w_\nu^{(1)}\rangle: {-\hspace{-0.05cm}1}\hspace{0.15cm} {+\hspace{-0.05cm}1} \hspace{0.15cm} {+\hspace{-0.05cm}1}\hspace{0.15cm} {-\hspace{-0.05cm}1} \hspace{0.05cm},$$
- $$\langle w_{\nu+3}^{(2)}\rangle = {+\hspace{-0.05cm}1}\hspace{0.15cm} {-\hspace{-0.05cm}1} \hspace{0.15cm} {-\hspace{-0.05cm}1}\hspace{0.15cm} {+\hspace{-0.05cm}1} \hspace{0.05cm},\hspace{0.3cm}{\rm Produkt\hspace{0.1cm} mit \hspace{0.1cm}}\langle w_\nu^{(1)}\rangle: {+\hspace{-0.05cm}1}\hspace{0.15cm} {+\hspace{-0.05cm}1} \hspace{0.15cm} {-\hspace{-0.05cm}1}\hspace{0.15cm} {-\hspace{-0.05cm}1} \hspace{0.05cm},$$
- $$\langle w_{\nu+4}^{(2)}\rangle = {+\hspace{-0.05cm}1}\hspace{0.15cm} {+\hspace{-0.05cm}1} \hspace{0.15cm} {-\hspace{-0.05cm}1}\hspace{0.15cm} {-\hspace{-0.05cm}1} = \langle w_\nu^{(2)}\rangle \hspace{0.05cm}.$$
- Das gleiche gilt für die PKKF $φ_{13}(λ)$.
- Dagegen erhält man für die PKKF zwischen den Folgen $ \langle w_\nu^{(2)}\rangle$ und $ \langle w_\nu^{(3)}\rangle$:
- $${\it \varphi}_{23}(\lambda ) = \left\{ \begin{array}{c}0 \\+1\\ -1 \\ \end{array} \right. \begin{array}{*{10}c} {\rm{f\ddot{u}r}} \\ {\rm{f\ddot{u}r}} \\ {\rm{f\ddot{u}r}} \\ \end{array} \begin{array}{*{20}c} \lambda = 0, \pm 2, \pm 4,\pm 6, ... \hspace{0.05cm}, \\ \hspace{0.14cm} \lambda = ... \hspace{0.05cm} , -3, +1, +5, ... \hspace{0.05cm}, \\ \hspace{0.14cm} \lambda = ... \hspace{0.05cm} , -5, -1, +3, ... \hspace{0.05cm}. \\ \end{array}$$
- Das bedeutet: Wird das Signal von Teilnehmer 3 gegenüber Teilnehmer 2 um ein Spreizchip verzögert oder umgekehrt, so lassen sich die Teilnehmer nicht mehr trennen und es kommt zu einer signifikanten Erhöhung der Fehlerwahrscheinlichkeit.
- In der Grafik sind die PKKF–Kurven gestrichelt eingezeichnet (violett und rot).
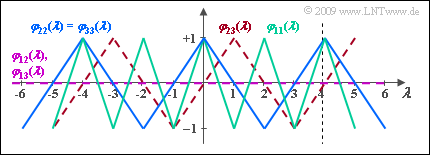
(4) Richtig sind die Aussagen 1, 2 und 4:
- Da die Walsh–Funktion Nr. 1 periodisch ist mit $T_0 = 2T_c$, ist auch die PAKF periodisch mit $λ = 2$.
- Die zweite Aussage ist richtig, wie die folgende Rechnung zeigt (grüner Kurvenzug):
- $${\it \varphi}_{11}(\lambda = 0) = 1/4 \cdot \left [ (+1) \cdot (+1) + (-1) \cdot (-1) + (+1) \cdot (+1) + (-1) \cdot (-1) \right ] = 1\hspace{0.05cm},$$
- $${\it \varphi}_{11}(\lambda = 1) = 1/4 \cdot \left [ (+1) \cdot (-1) + (+1) \cdot (-1) + (+1) \cdot (-1) + (+1) \cdot (-1) \right ] = -1\hspace{0.05cm}.$$
- Da sich die beiden Walsh–Funktionen Nr. 2 und 3 nur durch eine Verschiebung um $T_c$ unterscheiden und sich eine Phase in der PAKF prinzipiell nicht auswirkt, ist tatsächlich entsprechend dem letzten Lösungsvorschlag $φ_{33}(λ) = φ_{22}(λ)$. Diese beiden PAKF–Funktionen sind blau eingezeichnet.
- Dagegen unterscheidet sich $φ_{22}(λ)$ von $φ_{11}(λ)$ durch eine andere Periodizität: $φ_{22}(λ) = φ_{33}(λ)$ ist doppelt so breit wie $φ_{11}(λ)$.