Difference between revisions of "Digital Signal Transmission/Error Probability with Intersymbol Interference"
| Line 82: | Line 82: | ||
By comparing these images, the following statements can be verified: | By comparing these images, the following statements can be verified: | ||
*With the cutoff frequency $f_{\rm G} \cdot T = 0.8$ (upper graph), only minorintersymbol interferences result at the detection times $($at multiples of $T)$. Due to the Gaussian low-pass here primarily the corners of the transmitted signal $s(t)$ are rounded.<br> | *With the cutoff frequency $f_{\rm G} \cdot T = 0.8$ (upper graph), only minorintersymbol interferences result at the detection times $($at multiples of $T)$. Due to the Gaussian low-pass here primarily the corners of the transmitted signal $s(t)$ are rounded.<br> | ||
| − | *In contrast, in the lower image $(f_{\rm G} \cdot T = 0.4)$ | + | *In contrast, in the lower image $(f_{\rm G} \cdot T = 0.4)$ the effects of the intersymbol interferences are clearly visible. At the detection times $(\nu \cdot T)$, the signal component of the detection signal $d_{\rm S}(\nu \cdot T)$ shown in blue can assume six different values (grid lines drawn).<br> |
| − | * | + | *The noise component $d_{\rm N}(t)$ – recognizable as the difference between the yellow curve and the blue circles – is statistically larger on average with $f_{\rm G} \cdot T = 0.8$ than with $f_{\rm G} \cdot T = 0.4$.<br> |
| − | * | + | *This result can be explained by the right graph of $\text{example 1}$, which shows the power-spectral density of the noise component $d_{\rm N}(t)$: |
:$${\it \Phi}_{d{\rm N} }(f) = {N_0}/{2} \cdot \vert H_{\rm G}(f) \vert^2 = | :$${\it \Phi}_{d{\rm N} }(f) = {N_0}/{2} \cdot \vert H_{\rm G}(f) \vert^2 = | ||
{N_0}/{2} \cdot {\rm exp}\left [- | {N_0}/{2} \cdot {\rm exp}\left [- | ||
\frac{2\pi f^2}{(2f_{\rm G})^2} \right ] .$$ | \frac{2\pi f^2}{(2f_{\rm G})^2} \right ] .$$ | ||
| − | * | + | *The integral over ${\it \Phi}_{d{\rm N} }(f)$ – i.e. the noise power $\sigma_d^2$ – is twice as large for $f_{\rm G} \cdot T = 0.8$ (purple curve) than with $f_{\rm G} \cdot T = 0.4$ (red curve).}} |
== Definition and statements of the eye diagram== | == Definition and statements of the eye diagram== | ||
<br> | <br> | ||
| − | + | The above mentioned facts can also be explained by the eye diagram. | |
{{BlaueBox|TEXT= | {{BlaueBox|TEXT= | ||
| − | $\text{Definition:}$ | + | $\text{Definition:}$ The '''eye diagram''' (or <i>eye pattern</i>) is the sum of all superimposed sections of the detection signal, whose duration is an integer multiple of the symbol duration $T$. }} |
{{GraueBox|TEXT= | {{GraueBox|TEXT= | ||
| − | $\text{ | + | $\text{Example 3:}$ We assume a redundancy-free binary bipolar NRZ rectangular signal $s(t)$ and the Gaussian low-pass filter with cutoff frequency $f_{\rm G} \cdot T = 0.4$. |
| − | [[File:P ID1375 Dig T 3 2 S2 version1.png|right|frame| | + | [[File:P ID1375 Dig T 3 2 S2 version1.png|right|frame|Eye diagrams with and without noise|class=fit]] |
| − | <br> | + | <br>Shown are the eye diagrams after the Gaussian low-pass, |
| − | * | + | *left with noise ⇒ signal $d(t)$, |
| − | * | + | *on the right without taking noise into account ⇒ signal $d_{\rm S}(t)$. |
| − | + | This diagram has a certain resemblance to an eye, which led to its naming. | |
<br clear=all> | <br clear=all> | ||
| − | + | This representation allows important statements about the quality of a digital transmission system: | |
| − | * | + | *Only the eye diagram of the signal $d(t)$ can be displayed metrologically on an oscilloscope, which is triggered with the clock signal. From this eye diagram (left graph), for example, the noise rms value $\sigma_d$ can be read – or rather: estimated – werden.<br> |
| − | * | + | *The eye diagram without noise (right graph) refers to the signal component of the detection signal $d_{\rm S}(t)$ and can only be determined by means of a computer simulation. For an implemented system, this eye diagram cannot be displayed, since the noise component $d_{\rm N}(t)$ cannot be eliminated.<br> |
| − | * | + | *In both diagrams, $2048$ eye lines were drawn in each case. In the right graph, however, only $2^5 = 32$ eye lines are distinguishable because the present basic transmitter pulse $g_d(t)$ is limited to the time range $\vert t\vert \le 2T$ (see [[Digital_Signal_Transmission/Error_Probability_with_Intersymbol_Interference#Gaussian_receiver_filter|graph in example 1]] with $f_{\rm G} \cdot T = 0.4$, red curve).<br> |
| − | * | + | *The inner eye lines determine the '''vertical eye opening''' $\ddot{o}(T_{\rm D})$. The smaller this is, the greater is the influence of intersymbol interference. For a (intersymbol interference free) Nyquist system the vertical eye opening is maximum. Normalized to the transmitted amplitude, $\ddot{o}(T_{\rm D})/s_0 = 2$ is then valid. <br> |
| − | * | + | *With symmetrical basic pulse, the detection time $T_{\rm D} = 0$ is optimal. With a different value $($for example $T_{\rm D} = T/10) $, $\ddot{o}(T_{\rm D})$ would be somewhat smaller and thus the error probability would be significantly larger. This case is indicated by the purple–dashed vertical line in the right graph.}} |
| − | == | + | == Mean error probability== |
<br> | <br> | ||
| − | + | As with the previous graphs in this chapter, we assume the following: | |
| − | *NRZ | + | *NRZ rectangles with amplitude $s_0$, AWGN noise with $N_0$, where |
:$$10 \cdot {\rm lg}\hspace{0.1cm} \frac{s_0^2 \cdot T}{N_0}\approx | :$$10 \cdot {\rm lg}\hspace{0.1cm} \frac{s_0^2 \cdot T}{N_0}\approx | ||
13\,{\rm dB}\hspace{0.3cm}\Rightarrow \hspace{0.3cm} | 13\,{\rm dB}\hspace{0.3cm}\Rightarrow \hspace{0.3cm} | ||
\frac{N_0}{s_0^2 \cdot T} = 0.05\hspace{0.05cm}.$$ | \frac{N_0}{s_0^2 \cdot T} = 0.05\hspace{0.05cm}.$$ | ||
| − | * | + | *Gaussian receiver filter with cutoff frequency $f_{\rm G} \cdot T = 0.4$: |
:$$\sigma_d^2 = \frac{(N_0 /T)\cdot (f_{\rm G}\cdot T)}{\sqrt{2}}= \frac{0.05 \cdot | :$$\sigma_d^2 = \frac{(N_0 /T)\cdot (f_{\rm G}\cdot T)}{\sqrt{2}}= \frac{0.05 \cdot | ||
s_0^2\cdot0.4}{\sqrt{2}} \hspace{0.3cm} \Rightarrow \hspace{0.3cm} \sigma_d = \sqrt{0.0141}\cdot s_0 | s_0^2\cdot0.4}{\sqrt{2}} \hspace{0.3cm} \Rightarrow \hspace{0.3cm} \sigma_d = \sqrt{0.0141}\cdot s_0 | ||
\approx 0.119 \cdot s_0 \hspace{0.05cm}.$$ | \approx 0.119 \cdot s_0 \hspace{0.05cm}.$$ | ||
| − | * | + | * Let $g_d(\nu \cdot T) \approx 0$ be valid for $|\nu| \ge 2$. The other basic detection pulse values are given as follows: |
:$$g_0 = g_d(t=0) \approx 0.68 \cdot s_0, \hspace{0.5cm}g_1 = g_d(t=T) \approx 0.16 \cdot s_0, \hspace{0.2cm} g_{-1} = g_d(t=-T) \approx | :$$g_0 = g_d(t=0) \approx 0.68 \cdot s_0, \hspace{0.5cm}g_1 = g_d(t=T) \approx 0.16 \cdot s_0, \hspace{0.2cm} g_{-1} = g_d(t=-T) \approx | ||
0.16 \cdot s_0\hspace{0.05cm}.$$ | 0.16 \cdot s_0\hspace{0.05cm}.$$ | ||
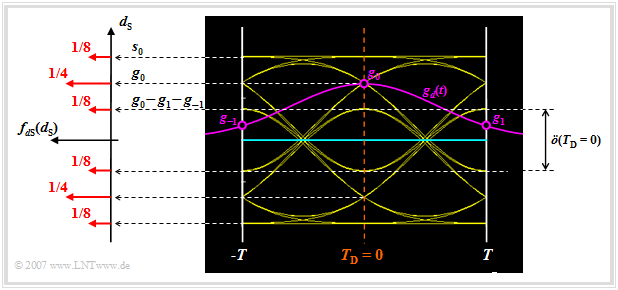
| − | [[File:P ID1377 Dig T 3 2 S3 version1.png|center|frame| | + | [[File:P ID1377 Dig T 3 2 S3 version1.png|center|frame|Eye diagram and PDF of the signal component|class=fit]] |
| − | + | et us now analyze the possible values for the signal component of the detection signal $d_{\rm S}(t)$ at the detection times: | |
| − | * | + | *Of the total $32$ eye lines, four lines intersect the ordinate $(t = 0)$ at $g_0 + 2 \cdot g_1 = s_0$. These lines belong to the amplitude coefficients "$\text{...}\hspace{0.05cm} +\hspace{-0.1cm}1,\hspace{0.05cm} {\it +\hspace{-0.05cm}1},\hspace{0.05cm} +\hspace{-0.05cm}1\hspace{0.05cm} \text{...}$". Here, the "middle" coefficient $a_{\nu = 0}$ is highlighted in italics.<br> |
| − | * | + | *The four eye lines, each representing the coefficients "$\text{...}\hspace{0.05cm} -\hspace{-0.1cm}1,\hspace{0.05cm} {\it +\hspace{-0.05cm}1},\hspace{0.05cm} -\hspace{-0.05cm}1,\hspace{0.05cm} \text{...}$" result in the useful sample $d_{\rm S}(T_{\rm D} = 0) =g_0 - 2 \cdot g_1 = 0.36 \cdot s_0$.<br> |
*Dagegen tritt der Nutzabtastwert $d_{\rm S}(T_{\rm D} = 0) =g_0 = 0.68 \cdot s_0$ doppelt so häufig auf. Dieser geht entweder auf die Koeffizienten "$\text{...}\hspace{0.05cm} +\hspace{-0.1cm}1,\hspace{0.05cm} {\it +\hspace{-0.05cm}1},\hspace{0.05cm} -\hspace{-0.05cm}1\hspace{0.05cm} \text{...}$" oder auf "$\text{...}\hspace{0.05cm} -\hspace{-0.1cm}1,\hspace{0.05cm} {\it +\hspace{-0.05cm}1},\hspace{0.05cm} +\hspace{-0.05cm}1\hspace{0.05cm} \text{...}$" zurück.<br> | *Dagegen tritt der Nutzabtastwert $d_{\rm S}(T_{\rm D} = 0) =g_0 = 0.68 \cdot s_0$ doppelt so häufig auf. Dieser geht entweder auf die Koeffizienten "$\text{...}\hspace{0.05cm} +\hspace{-0.1cm}1,\hspace{0.05cm} {\it +\hspace{-0.05cm}1},\hspace{0.05cm} -\hspace{-0.05cm}1\hspace{0.05cm} \text{...}$" oder auf "$\text{...}\hspace{0.05cm} -\hspace{-0.1cm}1,\hspace{0.05cm} {\it +\hspace{-0.05cm}1},\hspace{0.05cm} +\hspace{-0.05cm}1\hspace{0.05cm} \text{...}$" zurück.<br> | ||
*Für die $16$ Augenlinien, welche die Ordinate $T_{\rm D} = 0$ unterhalb der Entscheiderschwelle $E = 0$ schneiden, ergeben sich genau spiegelbildliche Verhältnisse.<br><br> | *Für die $16$ Augenlinien, welche die Ordinate $T_{\rm D} = 0$ unterhalb der Entscheiderschwelle $E = 0$ schneiden, ergeben sich genau spiegelbildliche Verhältnisse.<br><br> | ||
Revision as of 16:59, 25 April 2022
Contents
Gaussian receiver filter
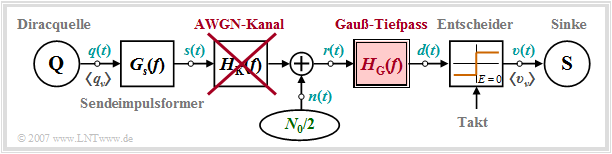
We start from the sketched block diagram. The following configuration is assumed for quantitative consideration of the intersymbol interference:
- Rectangular NRZ basic transmission pulse $g_s(t)$ with height $s_0$ and duration $T$,
- Gaussian-shaped receiver filter with cutoff frequency $f_{\rm G}$ (Note: In this section, we often also denote the exponential function by $\rm exp [ . ]$):
- $$H_{\rm E}(f) = H_{\rm G}(f) = {\rm exp}\left [- \frac{\pi \cdot f^2}{(2f_{\rm G})^2} \right ] \hspace{0.2cm} \bullet\!\!-\!\!\!-\!\!\!-\!\!\circ \hspace{0.2cm}h_{\rm E}(t) = h_{\rm G}(t) = {\rm exp}\left [- \pi \cdot (2 f_{\rm G} t)^2\right ] \hspace{0.05cm}.$$
- AWGN channel, that is, $H_{\rm K}(f) = 1 $ and ${\it \Phi}_n(f) = N_0/2$.
Based on the assumptions made here, the following holds for the basic detection pulse:
- $$g_d(t) = g_s(t) \star h_{\rm G}(t) = 2 f_{\rm G} \cdot s_0 \cdot \int_{t-T/2}^{t+T/2} {\rm e}^{- \pi \hspace{0.05cm}\cdot\hspace{0.05cm} (2 \hspace{0.05cm}\cdot\hspace{0.05cm} f_{\rm G}\hspace{0.05cm}\cdot\hspace{0.05cm} \tau )^2} \,{\rm d} \tau \hspace{0.05cm}.$$
The integration leads to the following equivalent results:
- $$g_d(t) = s_0 \cdot \big [ {\rm Q} \left ( 2 \cdot \sqrt {2 \pi} \cdot f_{\rm G}\cdot ( t - {T}/{2})\right )- {\rm Q} \left ( 2 \cdot \sqrt {2 \pi} \cdot f_{\rm G}\cdot ( t + {T}/{2} )\right ) \big ],$$
- $$g_d(t) = s_0 \cdot\big [ {\rm erfc} \left ( 2 \cdot \sqrt {\pi} \cdot f_{\rm G}\cdot ( t - {T}/{2})\right )- {\rm erfc} \left ( 2 \cdot \sqrt {\pi} \cdot f_{\rm G}\cdot ( t + {T}/{2} )\right ) \big ]\hspace{0.05cm}.$$
Here, two variants of the complementary Gaussian error function are used, viz.
- $${\rm Q} (x) = \frac{\rm 1}{\sqrt{\rm 2\pi}}\int_{\it x}^{+\infty}\rm e^{\it -u^{\rm 2}/\rm 2}\,d {\it u} \hspace{0.05cm},\hspace{0.5cm} {\rm erfc} (\it x) = \frac{\rm 2}{\sqrt{\rm \pi}}\int_{\it x}^{+\infty}\rm e^{\it -u^{\rm 2}}\,d \it u \hspace{0.05cm}.$$
The module Complementary Gaussian Error Functions provides the numerical values of ${\rm Q} (x)$ and $0.5 \cdot {\rm erfc} (x)$.
The noise power at the output of the Gaussian receiver filter $H_{\rm G}(f)$ is equal to
- $$\sigma_d^2 = \frac{N_0}{2} \cdot \int_{-\infty}^{+\infty} |H_{\rm G}(f)|^2 \,{\rm d} f = \frac{N_0\cdot f_{\rm G}}{\sqrt{2}}\hspace{0.05cm}.$$
From these two equations one can already see:
- The smaller the cutoff frequency $f_{\rm G}$ of the Gaussian low-pass filter, the smaller the noise rms value $\sigma_d$ and consequently the better the noise performance.
- However, a small cutoff frequency leads to a strong deviation of the basic detection pulse $g_d(t)$ from the square wave form and thus to intersymbol interference.
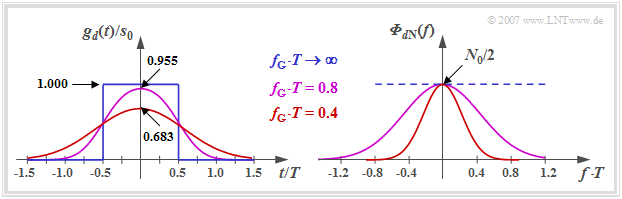
$\text{Example 1:}$ The left graph shows the basic detection pulse $g_d(t)$ at the output of a Gaussian low-pass filter $H_{\rm G}(f)$ with the cutoff frequency $f_{\rm G}$ when an NRZ rectangular pulse (blue curve) is applied at the input.
One can see from this plot:
- The Gaussian low-pass filter $H_{\rm G}(f)$ causes the detection pulse $g_d(t)$ to be reduced and broadened compared to the transmitted pulse $g_s(t)$ ⇒ time dispersion.
- This pulse deformation is the stronger, the smaller the cutoff frequency $f_{\rm G}$ is. For example, with $f_{\rm G} \cdot T = 0.4$ (red curve) the pulse maximum is already reduced to about $68\%$.
- In the limiting case $f_{\rm G} \to \infty$ the Gaussian low-pass has no effect ⇒ $g_d(t) = g_s(t)$. However, in this case, no noise limitation is effective at all, as can be seen from the right figure.
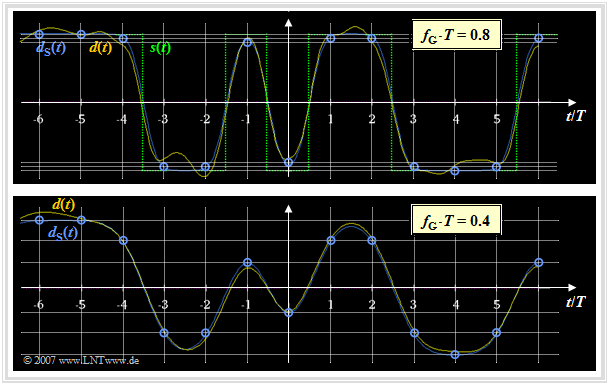
$\text{Example 2:}$ The same preconditions apply as for the last example. The graph shows the detection signal $d(t)$ after the Gaussian low-pass (before the decision) for two different cutoff frequencies, namely $f_{\rm G} \cdot T = 0.8$ and $f_{\rm G} \cdot T = 0.4$
Shown equally in both diagrams (but admittedly difficult to see as a screen capture) are:
- the component $d_{\rm S}(\nu \cdot T)$ without considering the noise (blue circles at the detection times),
- the total detection signal $d(t)$ including the noise component (yellow),
- the transmitted signal $s(t)$ as reference signal (green dotted in the upper graph; equally valid for the lower graph).
By comparing these images, the following statements can be verified:
- With the cutoff frequency $f_{\rm G} \cdot T = 0.8$ (upper graph), only minorintersymbol interferences result at the detection times $($at multiples of $T)$. Due to the Gaussian low-pass here primarily the corners of the transmitted signal $s(t)$ are rounded.
- In contrast, in the lower image $(f_{\rm G} \cdot T = 0.4)$ the effects of the intersymbol interferences are clearly visible. At the detection times $(\nu \cdot T)$, the signal component of the detection signal $d_{\rm S}(\nu \cdot T)$ shown in blue can assume six different values (grid lines drawn).
- The noise component $d_{\rm N}(t)$ – recognizable as the difference between the yellow curve and the blue circles – is statistically larger on average with $f_{\rm G} \cdot T = 0.8$ than with $f_{\rm G} \cdot T = 0.4$.
- This result can be explained by the right graph of $\text{example 1}$, which shows the power-spectral density of the noise component $d_{\rm N}(t)$:
- $${\it \Phi}_{d{\rm N} }(f) = {N_0}/{2} \cdot \vert H_{\rm G}(f) \vert^2 = {N_0}/{2} \cdot {\rm exp}\left [- \frac{2\pi f^2}{(2f_{\rm G})^2} \right ] .$$
- The integral over ${\it \Phi}_{d{\rm N} }(f)$ – i.e. the noise power $\sigma_d^2$ – is twice as large for $f_{\rm G} \cdot T = 0.8$ (purple curve) than with $f_{\rm G} \cdot T = 0.4$ (red curve).
Definition and statements of the eye diagram
The above mentioned facts can also be explained by the eye diagram.
$\text{Definition:}$ The eye diagram (or eye pattern) is the sum of all superimposed sections of the detection signal, whose duration is an integer multiple of the symbol duration $T$.
$\text{Example 3:}$ We assume a redundancy-free binary bipolar NRZ rectangular signal $s(t)$ and the Gaussian low-pass filter with cutoff frequency $f_{\rm G} \cdot T = 0.4$.
Shown are the eye diagrams after the Gaussian low-pass,
- left with noise ⇒ signal $d(t)$,
- on the right without taking noise into account ⇒ signal $d_{\rm S}(t)$.
This diagram has a certain resemblance to an eye, which led to its naming.
This representation allows important statements about the quality of a digital transmission system:
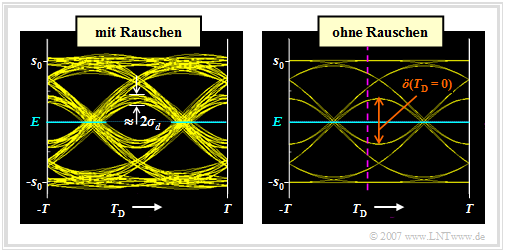
- Only the eye diagram of the signal $d(t)$ can be displayed metrologically on an oscilloscope, which is triggered with the clock signal. From this eye diagram (left graph), for example, the noise rms value $\sigma_d$ can be read – or rather: estimated – werden.
- The eye diagram without noise (right graph) refers to the signal component of the detection signal $d_{\rm S}(t)$ and can only be determined by means of a computer simulation. For an implemented system, this eye diagram cannot be displayed, since the noise component $d_{\rm N}(t)$ cannot be eliminated.
- In both diagrams, $2048$ eye lines were drawn in each case. In the right graph, however, only $2^5 = 32$ eye lines are distinguishable because the present basic transmitter pulse $g_d(t)$ is limited to the time range $\vert t\vert \le 2T$ (see graph in example 1 with $f_{\rm G} \cdot T = 0.4$, red curve).
- The inner eye lines determine the vertical eye opening $\ddot{o}(T_{\rm D})$. The smaller this is, the greater is the influence of intersymbol interference. For a (intersymbol interference free) Nyquist system the vertical eye opening is maximum. Normalized to the transmitted amplitude, $\ddot{o}(T_{\rm D})/s_0 = 2$ is then valid.
- With symmetrical basic pulse, the detection time $T_{\rm D} = 0$ is optimal. With a different value $($for example $T_{\rm D} = T/10) $, $\ddot{o}(T_{\rm D})$ would be somewhat smaller and thus the error probability would be significantly larger. This case is indicated by the purple–dashed vertical line in the right graph.
Mean error probability
As with the previous graphs in this chapter, we assume the following:
- NRZ rectangles with amplitude $s_0$, AWGN noise with $N_0$, where
- $$10 \cdot {\rm lg}\hspace{0.1cm} \frac{s_0^2 \cdot T}{N_0}\approx 13\,{\rm dB}\hspace{0.3cm}\Rightarrow \hspace{0.3cm} \frac{N_0}{s_0^2 \cdot T} = 0.05\hspace{0.05cm}.$$
- Gaussian receiver filter with cutoff frequency $f_{\rm G} \cdot T = 0.4$:
- $$\sigma_d^2 = \frac{(N_0 /T)\cdot (f_{\rm G}\cdot T)}{\sqrt{2}}= \frac{0.05 \cdot s_0^2\cdot0.4}{\sqrt{2}} \hspace{0.3cm} \Rightarrow \hspace{0.3cm} \sigma_d = \sqrt{0.0141}\cdot s_0 \approx 0.119 \cdot s_0 \hspace{0.05cm}.$$
- Let $g_d(\nu \cdot T) \approx 0$ be valid for $|\nu| \ge 2$. The other basic detection pulse values are given as follows:
- $$g_0 = g_d(t=0) \approx 0.68 \cdot s_0, \hspace{0.5cm}g_1 = g_d(t=T) \approx 0.16 \cdot s_0, \hspace{0.2cm} g_{-1} = g_d(t=-T) \approx 0.16 \cdot s_0\hspace{0.05cm}.$$
et us now analyze the possible values for the signal component of the detection signal $d_{\rm S}(t)$ at the detection times:
- Of the total $32$ eye lines, four lines intersect the ordinate $(t = 0)$ at $g_0 + 2 \cdot g_1 = s_0$. These lines belong to the amplitude coefficients "$\text{...}\hspace{0.05cm} +\hspace{-0.1cm}1,\hspace{0.05cm} {\it +\hspace{-0.05cm}1},\hspace{0.05cm} +\hspace{-0.05cm}1\hspace{0.05cm} \text{...}$". Here, the "middle" coefficient $a_{\nu = 0}$ is highlighted in italics.
- The four eye lines, each representing the coefficients "$\text{...}\hspace{0.05cm} -\hspace{-0.1cm}1,\hspace{0.05cm} {\it +\hspace{-0.05cm}1},\hspace{0.05cm} -\hspace{-0.05cm}1,\hspace{0.05cm} \text{...}$" result in the useful sample $d_{\rm S}(T_{\rm D} = 0) =g_0 - 2 \cdot g_1 = 0.36 \cdot s_0$.
- Dagegen tritt der Nutzabtastwert $d_{\rm S}(T_{\rm D} = 0) =g_0 = 0.68 \cdot s_0$ doppelt so häufig auf. Dieser geht entweder auf die Koeffizienten "$\text{...}\hspace{0.05cm} +\hspace{-0.1cm}1,\hspace{0.05cm} {\it +\hspace{-0.05cm}1},\hspace{0.05cm} -\hspace{-0.05cm}1\hspace{0.05cm} \text{...}$" oder auf "$\text{...}\hspace{0.05cm} -\hspace{-0.1cm}1,\hspace{0.05cm} {\it +\hspace{-0.05cm}1},\hspace{0.05cm} +\hspace{-0.05cm}1\hspace{0.05cm} \text{...}$" zurück.
- Für die $16$ Augenlinien, welche die Ordinate $T_{\rm D} = 0$ unterhalb der Entscheiderschwelle $E = 0$ schneiden, ergeben sich genau spiegelbildliche Verhältnisse.
Die möglichen Werte $d_{\rm S}(T_{\rm D})$ und deren Auftrittswahrscheinlichkeiten findet man in obiger Grafik auf der linken Seite in der Wahrscheinlichkeitsdichtefunktion (WDF) der Detektionsnutzabtastwerte wieder:
- $$f_{d{\rm S}}(d_{\rm S}) = {1}/{8} \cdot \delta (d_{\rm S} - s_0)+ {1}/{4} \cdot \delta (d_{\rm S} - 0.68 \cdot s_0)+ {1}/{8} \cdot \delta (d_{\rm S} - 0.36 \cdot s_0)+ $$
$$\hspace{2.15cm} + \hspace{0.2cm} {1}/{8} \cdot \delta (d_{\rm S} + s_0)+{1}/{4} \cdot \delta (d_{\rm S} + 0.68 \cdot s_0)+{1}/{8} \cdot \delta (d_{\rm S} + 0.36 \cdot s_0)\hspace{0.05cm}.$$
Damit kann die (mittlere) Symbolfehlerwahrscheinlichkeit des impulsinterferenzbehafteten Systems angegeben werden. Unter Ausnutzung der Symmetrie erhält man mit $\sigma_d/s_0 = 0.119$:
- $$p_{\rm S} = {1}/{4} \cdot {\rm Q} \left( \frac{s_0}{ \sigma_d} \right)+ {1}/{2} \cdot {\rm Q} \left( \frac{0.68 \cdot s_0}{ \sigma_d} \right)+{1}/{4} \cdot {\rm Q} \left( \frac{0.36 \cdot s_0}{ \sigma_d} \right)$$
- $$\Rightarrow \hspace{0.3cm}p_{\rm S} \approx {1}/{4} \cdot {\rm Q}(8.40) +{1}/{2} \cdot {\rm Q}(5.71)+ {1}/{4} \cdot {\rm Q}(3.02)\approx {1}/{4} \cdot 2.20 \cdot 10^{-17}+ {1}/{2} \cdot 1.65 \cdot 10^{-9}+ {1}/{4} \cdot 1.26 \cdot 10^{-3} \approx 3.14 \cdot 10^{-4} \hspace{0.05cm}.$$
$\text{Fazit:}$ Anhand dieses Zahlenbeispiels erkennt man:
- Bei Vorhandensein von Impulsinterferenzen wird die (mittlere) Symbolfehlerwahrscheinlichkeit $p_{\rm S}$ im Wesentlichen durch die inneren Augenlinien bestimmt.
- Der Rechenaufwand zur Bestimmung der Fehlerwahrscheinlichkeit $p_{\rm S}$ kann sehr groß werden, insbesondere dann, wenn die Impulsinterferenzen von sehr vielen Grundimpulswerten $g_\nu$ herrühren.
$\text{Beispiel 4:}$
- Sind die Grundimpulswerte $g_{-5}, \text{...} \ , g_{+5}$ von Null verschieden und $E \ne 0$, so ist zur Bestimmung der Fehlerwahrscheinlichkeit $p_{\rm S}$ eine Mittelung über $2^{11} = 2048$ Augenlinien erforderlich.
- Sind dagegen nur die Grundimpulswerte $g_{-1}, \ g_0, \ g_{+1}$ von Null verschieden und wird zudem die Symmetrie bezüglich der Schwelle $E = 0$ berücksichtigt, so reduziert sich der Aufwand auf die Mittelung über vier Terme.
- Gilt zusätzlich die Symmetrie $g_{-1} = g_{+1}$ wie bei den obigen Zahlenwerten, so kann auch die Symmetrie bezüglich $T_{\rm D}$ ausgenutzt werden und es genügt die Mittelung über drei Terme.
Ungünstigste Fehlerwahrscheinlichkeit
In der Vergangenheit wurden eine Vielzahl von Näherungen für die mittlere Fehlerwahrscheinlichkeit angegeben, unter Anderem:
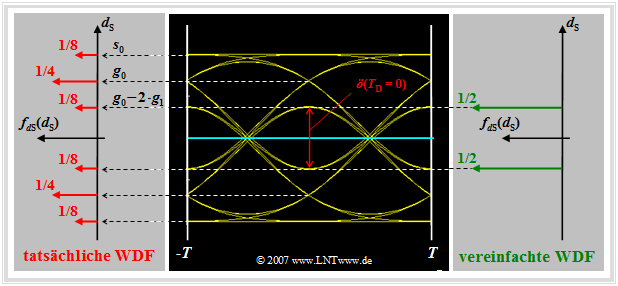
$\text{Definition:}$ Als eine sehr einfache Näherung für die tatsächliche Fehlerwahrscheinlichkeit $p_{\rm S}$ verwendet man häufig die ungünstigste Fehlerwahrscheinlichkeit (englisch: Worst-Case Error Probability)
- $$p_{\rm U} = {\rm Q} \left( \frac{\ddot{o}(T_{\rm D})/2}{ \sigma_d} \right) \hspace{0.05cm}.$$
Für deren Berechnung wird stets von den ungünstigsten Symbolfolgen ausgegangen. Das bedeutet:
- Die tatsächliche WDF der Nutzabtastwerte (linke Grafik: sechs rote Diracs) wird durch eine vereinfachte WDF mit nur den inneren Diracfunktionen (rechte Grafik: zwei grüne Diracs) ersetzt.
- Für die halbe vertikale Augenöffnung gilt mit den Grundimpulswerten $g_\nu = g_d( T_{\rm D}+ \nu \cdot T)$ allgemein:
- $$\ddot{o}(T_{\rm D})/{ 2}= g_0 - \sum_{\nu = 1}^{n} \vert g_{\nu} \vert- \sum_{\nu = 1}^{v} \vert g_{-\nu} \vert \hspace{0.05cm}.$$
Diese Gleichung kann wie folgt interpretiert werden:
- $g_0 = g_d( T_{\rm D})$ ist der so genannte Hauptwert des Grundimpulses. Bei Nyquistsystemen gilt stets $\ddot{o}(T_{\rm D})/{ 2}= g_0$. Im Folgenden wird (meist) $T_{\rm D}= 0$ gesetzt.
- Die erste Summe beschreibt die Impulsinterferenzen der $n$ Nachläufer vorangegangener Impulse. Stillschweigend vorausgesetzt wird $g_\nu = 0$ für $\nu \gt n$.
- Die zweite Summe berücksichtigt den Einfluss der $v$ Vorläufer nachfolgender Impulse unter der Voraussetzung $g_{-\nu} = 0$ für $\nu \gt v$.
- Sind alle Impulsvor– und –nachläufer positiv, so lauten die beiden ungünstigsten Symbolfolgen "$\text{...}\hspace{0.05cm} -\hspace{-0.1cm}1,\hspace{0.05cm} -\hspace{-0.05cm}1,\hspace{0.05cm} {\it +\hspace{-0.05cm}1},\hspace{0.05cm} -\hspace{-0.05cm}1,\hspace{0.05cm} -\hspace{-0.05cm}1\hspace{0.05cm} \text{...}$" und "$\text{...}\hspace{0.05cm} +\hspace{-0.1cm}1,\hspace{0.05cm} +\hspace{-0.05cm}1,\hspace{0.05cm} {\it -\hspace{-0.05cm}1},\hspace{0.05cm} +\hspace{-0.05cm}1,\hspace{0.05cm} +\hspace{-0.05cm}1\hspace{0.05cm} \text{...}$" (der Koeffizient $a_{\nu = 0}$ ist jeweils kursiv). Diese Angaben treffen zum Beispiel für das hier betrachtete gaußförmige Empfangsfilter zu.
- Sind einige Grundimpulswerte negativ, so wird dies in obiger Gleichung durch die Betragsbildung berücksichtigt. Es ergeben sich dann andere "Worst–Case"–Folgen als gerade genannt.
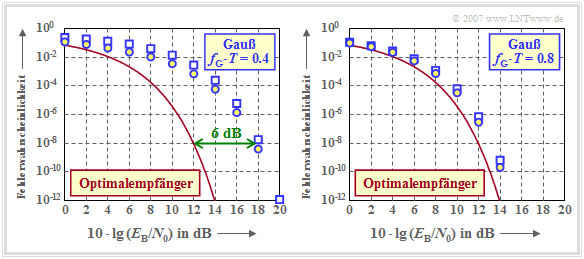
$\text{Beispiel 5:}$ Die Grafik zeigt die Fehlerwahrscheinlichkeiten des AWGN–Kanals in Abhängigkeit des (logarithmierten) Quotienten $E_{\rm B}/N_0$, nämlich
- die mittlere Fehlerwahrscheinlichkeit $p_{\rm S}$ bei gaußförmigem Empfangsfilter (blaue Kreise),
- die ungünstigste Fehlerwahrscheinlichkeit $p_{\rm U}$ bei gaußförmigem Empfangsfilter (blaue Rechtecke),
- die kleinstmögliche Fehlerwahrscheinlichkeit gemäß der Seite Optimaler Binärempfänger (rote Kurve).
Die Energie pro Bit ist dabei gleich $E_{\rm B} = s_0^2 \cdot T$ (NRZ–Rechteck–Sendeimpulse).
Die linke Grafik gilt für die (normierte) Grenzfrequenz $f_{\rm G} \cdot T = 0.4$, die rechte für ein breitbandigeres Empfangsfilter mit $f_{\rm G} \cdot T = 0.8$. Die Ergebnisse können wie folgt interpretiert werden:
- Die ungünstigste Fehlerwahrscheinlichkeit $p_{\rm U}$ ist stets eine obere Schranke für die tatsächliche Symbolfehlerwahrscheinlichkeit $p_{\rm S}$. Je kleiner der Einfluss der Impulsinterferenzen ist (große Grenzfrequenz), um so näher liegen $p_{\rm S}$ und $p_{\rm U}$ zusammen. Beim Optimalempfänger gilt $p_{\rm S} = p_{\rm U}.$
- Bei gaußförmigem Empfangsfilter mit $f_{\rm G} \cdot T \ge 0.3$ werden die Impulsinterferenzen allein durch die Nachbarimpulse hervorgerufen $(g_2 = g_3 = \text{...} \approx 0)$, so dass für $p_{\rm S}$ auch eine untere Schranke angegeben werden kann:
- $${p_{\rm U} }/{ 4} \le p_{\rm S} \le p_{\rm U} \hspace{0.05cm}.$$
- Die starken Impulsinterferenzen eines gaußförmigen Empfangsfilters mit $f_{\rm G} \cdot T = 0.4$ führen dazu, dass gegenüber dem Optimalempfänger ein um $6 \ \rm dB$ größeres $E_{\rm B}/N_0$ aufgewendet werden muss (vierfache Leistung), damit die Fehlerwahrscheinlichkeit den Wert $10^{-8}$ nicht überschreitet.
- Der horizontale Abstand zwischen der blauen $p_{\rm S}$–Kurve (markiert durch Kreise) und der roten Vergleichskurve ist aber nicht konstant. Bei $p_{\rm S} = 10^{-2}$ beträgt der Abstand nur $4 \ \rm dB$.
- Die rechte Grafik zeigt, dass mit $f_{\rm G} \cdot T = 0.8$ der Abstand zum Vergleichssystem weniger als $1 \ \rm dB$ beträgt. Auf der nächsten Seite wird gezeigt, dass bei einem gaußförmigen Empfangsfilter die (normierte) Grenzfrequenz $f_{\rm G} \cdot T \approx 0.8$ das Optimum darstellt.
Optimierung der Grenzfrequenz
Für die Systemoptimierung und den Systemvergleich erweist es sich als zweckmäßig,
- anstelle der ungünstigsten Fehlerwahrscheinlichkeit $p_{\rm U}$
- das ungünstigste Signal–zu–Rausch–Leistungsverhältnis (S/N-Verhältnis) zu verwenden:
- $$\rho_{\rm U} = [\ddot{o}(T_{\rm D})]^2/ \sigma_d^2.$$
Bei Gaußscher Störung besteht folgender Zusammenhang:
- $$p_{\rm U} = {\rm Q} \left( \sqrt{\rho_{\rm U}} \right) \hspace{0.05cm}.$$
Die mittlere Symbolfehlerwahrscheinlichkeit $p_{\rm S}$ kann formal über die Q–Funktion ebenfalls durch ein S/N–Verhältnis ausgedrückt werden:
- $$\rho_d = \left[{\rm Q}^{-1} \left( p_{\rm S} \right)\right]^2 \hspace{0.05cm}.$$
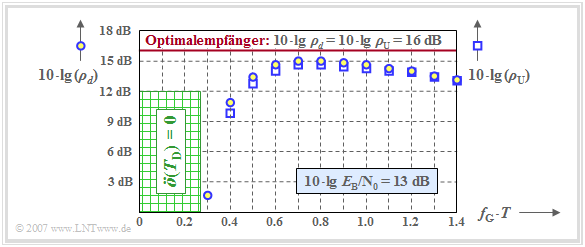
Die Grafik zeigt die beiden Größen $\rho_d$ und $\rho_{\rm U}$ in logarithmischer Form abhängig von der normierten Grenzfrequenz $f_{\rm G} \cdot T$ eines gaußförmigen Empfangsfilters, wobei $10 \cdot {\rm lg}\hspace{0.1cm} E_{\rm B}/N_0 = 13 \ \rm dB$ zugrunde liegt.
- Die blau umrandeten Kreise gelten für $10 \cdot {\rm lg}\hspace{0.1cm} \rho_d$ ⇒ "mittleres" Detektions–SNR,
- Die blau umrandeten Quadrate markieren $10 \cdot {\rm lg}\hspace{0.1cm} \rho_{\rm U}$ ⇒ "ungünstigstes" SNR.
Zum Vergleich ist als rote horizontale Linie auch das Ergebnis für den optimalen Binärempfänger eingezeichnet. Für diesen gilt:
- $$\rho_d = \rho_{\rm U} = {2 \cdot E_{\rm B}}/{ N_0}\hspace{0.3cm}\Rightarrow \hspace{0.3cm} 10 \cdot {\rm lg}\hspace{0.1cm} \rho_d = 10 \cdot {\rm lg}\hspace{0.1cm} \rho_{\rm U} \approx 16\,{\rm dB} \hspace{0.05cm}.$$
Man erkennt aus der Darstellung:
- Das Optimierungskriterium $\rho_d$ führt zur optimalen Grenzfrequenz $f_\text{G, opt} \cdot T = 0.8$. Eine kleinere Grenzfrequenz hat stärkere Impulsinterferenzen zur Folge (kleinere Augenöffnung), eine größere Grenzfrequenz bewirkt einen größeren Rauscheffektivwert $\sigma_d$.
- Ein solches gaußförmiges Empfangsfilter mit $f_\text{G, opt} \cdot T \approx 0.8$ führt zum Störabstand $10 \cdot {\rm lg}\hspace{0.1cm} \rho_d \approx 15 \ \rm dB$ und damit zur Fehlerwahrscheinlichkeit $p_{\rm S} \approx 10^{-8}$. Zum Vergleich: Für den optimalen Empfänger (an den Sender angepasste Impulsantwort) ergeben sich $10 \cdot {\rm lg}\hspace{0.1cm} \rho_d \approx 16 \ \rm dB$ und $p_{\rm S} \approx 10^{-10}$.
- Die Grafik zeigt aber auch, dass das sehr viel einfachere Optimierungskriterien $ \rho_{\rm U}$ $($bzw. $ p_{\rm U})$ näherungsweise zur gleichen optimalen Grenzfrequenz $f_\text{G, opt} \cdot T = 0.8$ führt. Für diese Grenzfrequenz erhält man $10 \cdot {\rm lg}\hspace{0.1cm} \rho_{\rm U} \approx 14.7 \ \rm dB$ sowie die ungünstigste Fehlerwahrscheinlichkeit $p_{\rm U} \approx 3 \cdot 10^{-8}$.
- Ist die Grenzfrequenz $f_\text{G} \cdot T < 0.27$, so ergibt sich für die vertikale Augenöffnung immer $\ddot{o}(T_{\rm D}) = 0$. Man spricht von einem geschlossenen Auge. Dies hat zur Folge, dass einige ungünstige Impulsfolgen auch ohne Rauschen immer falsch entschieden würden. Es tritt ein systematischer Fehler auf.
- Weitere Untersuchungen haben gezeigt, dass das Optimierungskriterium $ \rho_{\rm U}$ auch bei kleinerem $E_{\rm B}/N_0$ ausreichend ist. Bei einem verzerrungsfreien Kanal ⇒ $H_{\rm K}(f) = 1$, ergibt sich somit die optimale Grenzfrequenz des Gaußtiefpasses stets zu $f_\text{G, opt} \cdot T \approx 0.8$, zumindest bei realitätsnaher Betrachtungsweise.
Alle Aussagen dieses Kapitels können mit dem interaktiven Applet Augendiagramm und Augenöffnung nachvollzogen werden.
Aufgaben zum Kapitel
Aufgabe 3.2: Augendiagramm nach Gaußtiefpass
Aufgabe 3.2Z: Optimale Grenzfrequenz bei Gauß-Tiefpass