Difference between revisions of "Aufgaben:Exercise 4.4: Pointer Diagram for DSB-AM"
m (Guenter verschob die Seite 4.4 Zeigerdiagramm beim ZSB-AM nach 4.4 Zeigerdiagramm bei ZSB-AM) |
|||
| (37 intermediate revisions by 5 users not shown) | |||
| Line 1: | Line 1: | ||
| − | {{quiz-Header|Buchseite= | + | {{quiz-Header|Buchseite=Signal_Representation/Analytical_Signal_and_Its_Spectral_Function |
}} | }} | ||
| − | [[File:P_ID718__Sig_A_4_4.png|250px|right| | + | [[File:P_ID718__Sig_A_4_4.png|250px|right|frame|Spectrum of the analytical signal]] |
| − | + | We assume a cosine-shaped source signal $q(t)$ with | |
| − | + | *amplitude $A_{\rm N} = 0.8 \ \text{V}$ and | |
| + | *frequency $f_{\rm N}= 10 \ \text{kHz}$. | ||
| + | |||
| + | |||
| + | The frequency conversion is done by means of [[Modulation_Methods/Zweiseitenband-Amplitudenmodulation#ZSB-Amplitudenmodulation_mit_Tr.C3.A4ger|"Double-Sideband Amplitude Modulation with Carrier"]]. | ||
| + | |||
| + | The modulated signal $s(t)$ is with the (normalised) carrier $z(t) = \text{cos}(\omega_{\rm T} \cdot t)$ and the DC component $q_0 = 1 \ \text{V}$: | ||
| − | $$\begin{align*} s(t) & = \left(q_0 + q(t)\right) \cdot z(t)= \left({\rm 1 \hspace{0.05cm} | + | :$$\begin{align*} s(t) & = \left(q_0 + q(t)\right) \cdot z(t)= \left({\rm 1 \hspace{0.05cm} |
V} + {\rm 0.8 \hspace{0.05cm}V}\cdot {\cos} ( \omega_{\rm N}\cdot t)\right) | V} + {\rm 0.8 \hspace{0.05cm}V}\cdot {\cos} ( \omega_{\rm N}\cdot t)\right) | ||
\cdot {\cos} ( \omega_{\rm T}\cdot t) = \\ & = q_0 \cdot {\cos} ( \omega_{\rm T}\cdot t) + | \cdot {\cos} ( \omega_{\rm T}\cdot t) = \\ & = q_0 \cdot {\cos} ( \omega_{\rm T}\cdot t) + | ||
| − | + | {A_{\rm N}}/{2} \cdot {\cos} ( (\omega_{\rm T}+ \omega_{\rm N}) \cdot t) | |
| − | + | + | + {A_{\rm N}}/{2} \cdot {\cos} ( (\omega_{\rm T}- \omega_{\rm N}) \cdot t).\end{align*}$$ |
| − | + | The first term describes the carrier, the second term the so-called upper sideband $\rm (OSB)$ and the last term the lower sideband $\rm (USB)$. | |
| − | |||
| − | |||
| − | + | The sketch shows the spectrum $S_+(f)$ of the corresponding analytical signal for $f_{\rm T} = 50 \ \text{kHz}$. You can see | |
| − | + | *the carrier (red), | |
| − | + | *the upper sideband (blue), and | |
| − | + | *the lower sideband (grün). | |
| − | |||
| − | |||
| − | |||
| − | |||
| − | === | + | In subtask '''(5)''' the magnitude of $s_+(t)$ is asked for. This is the length of the resulting pointer. |
| + | |||
| + | |||
| + | |||
| + | |||
| + | |||
| + | ''Hints:'' | ||
| + | *This exercise belongs to the chapter [[Signal_Representation/Analytical_Signal_and_Its_Spectral_Function|Analytical Signal and its Spectral Function]]. | ||
| + | *The interactive applet [[Applets:Physical_Signal_%26_Analytic_Signal|Physical and Analytical Signal]] illustrates the topic covered here. | ||
| + | *In this task we use the following nomenclature because of the German original: | ||
| + | #The index $\rm N$ stands for "source signal" ⇒ (German: "Nachrichtenignal"). | ||
| + | #The index $\rm T$ stands for "carrier" ⇒ (German: "Trägersignal"). | ||
| + | #$\rm OSB$ denotes the "upper sideband" ⇒ (German: "oberes Seitenband"). | ||
| + | #$\rm USB$ denotes the "lower sideband" ⇒ (German: "unteres Seitenband"). | ||
| + | |||
| + | ===Questions=== | ||
<quiz display=simple> | <quiz display=simple> | ||
| − | { | + | {What is the analytical signal $s_+(t)$. What is its magnitude at time $t = 0$? |
|type="{}"} | |type="{}"} | ||
| − | $\text{Re}[s_+(t=0)] =$ { 1.8 } V | + | $\text{Re}[s_+(t=0)]\ = \ $ { 1.8 3% } $\text{V}$ |
| − | $\text{Im}[s_+(t=0)] =$ { 0 } V | + | $\text{Im}[s_+(t=0)]\ = \ $ { 0. } $\text{V}$ |
| − | { | + | {Which of the following statements are true? |
|type="[]"} | |type="[]"} | ||
| − | + $s_+(t)$ | + | + $s_+(t)$ results from $s(t)$, if $\cos(\text{...})$ is replaced by ${\rm e}^{{\rm j}(\text{...})}$ . |
| − | - | + | - If $s(t)$ is an even time function, $s_+(t)$ is purely real. |
| − | - | + | - At no time does the imaginary part of $s_+(t)$ disappear. |
| − | { | + | {What is the value of the analytical signal at time $t = 5 \ {\rm µ}\text{s}$? |
|type="{}"} | |type="{}"} | ||
| − | $\text{Re}[s_+(t=5 \ | + | $\text{Re}[s_+(t=5 \ {\rm µ} \text{s})]\ = \ $ { 0. } $\text{V}$ |
| − | $\text{Im}[s_+(t=5 \ | + | $\text{Im}[s_+(t=5 \ {\rm µ} \text{s})]\ = \ $ { 1.761 3% } $\text{V}$ |
| − | { | + | {What is the value of $s_+(t)$ at time $t = 20 \ {\rm µ}\text{s}$? |
|type="{}"} | |type="{}"} | ||
| − | $\text{Re}[s_+(t=20 \ | + | $\text{Re}[s_+(t=20 \ {\rm µ} \text{s})]\ = \ $ { 1.236 3% } $\text{V}$ |
| − | $\text{Im}[s_+(t=20 \ | + | $\text{Im}[s_+(t=20 \ {\rm µ} \text{s})]\ = \ $ { 0. } $\text{V}$ |
| − | { | + | {What is the smallest possible pointer length? At what time $t_{\text{min}}$ does this value occur for the first time? |
|type="{}"} | |type="{}"} | ||
| − | $|s_+(t)|_{\text{min}} =$ { 0.2 3% } V | + | $|s_+(t)|_{\text{min}}\ = \ $ { 0.2 3% } $\text{V}$ |
| − | $t_{\text{min}} =$ { 50 } | + | $t_{\text{min}}\ = \ $ { 50 3% } ${\rm µ} \text{s}$ |
| Line 62: | Line 77: | ||
| − | === | + | ===Solution=== |
{{ML-Kopf}} | {{ML-Kopf}} | ||
| − | '''1 | + | '''(1)''' By inverse Fourier transform of $S_+(f)$ taking into account the "Shifting Theorem": |
| − | + | ||
| − | $$s_{+}(t) = {\rm 1 \hspace{0.05cm} V} \cdot {\rm e}^{{\rm | + | :$$s_{+}(t) = {\rm 1 \hspace{0.05cm} V} \cdot {\rm e}^{{\rm |
j}\hspace{0.05cm} \omega_{\rm 50}\hspace{0.05cm} t } + {\rm 0.4 | j}\hspace{0.05cm} \omega_{\rm 50}\hspace{0.05cm} t } + {\rm 0.4 | ||
\hspace{0.05cm} V} \cdot {\rm e}^{{\rm j}\hspace{0.05cm} | \hspace{0.05cm} V} \cdot {\rm e}^{{\rm j}\hspace{0.05cm} | ||
| Line 73: | Line 88: | ||
40}\hspace{0.05cm} t }.$$ | 40}\hspace{0.05cm} t }.$$ | ||
| − | + | [[File:EN_Sig_A_4_4_ML.png|center|frame|Three different analytical signals]] | |
| + | |||
| + | *The expression describes the sum of three pointers rotating at different circular velocities. | ||
| + | *In the above equation, for example, $\omega_{60} = 2\pi (f_{\rm T} + f_{\rm N}) = 2\pi \cdot 60 \ \text{kHz}$. | ||
| + | *At time $t = 0$ all three pointers point in the direction of the real axis (see left graph). | ||
| + | *One obtains the <u>real value</u> $s_+(t = 0) \;\underline{= 1.8 \ \text{V}}$. | ||
| + | |||
| + | <br clear=all> | ||
| + | '''(2)''' The <u>first statement</u> is correct and results from the "Hilbert transform". On the other hand, the next two statements are'nt correct: | ||
| + | *$s_+(t)$ is always a complex time function with exception of the limiting case $s(t) \equiv 0$. | ||
| + | *However, every complex function also has purely real values at some points in time. | ||
| + | *The "pointer group" always rotates in a mathematically positive direction. | ||
| + | *If the sum vector crosses the real axis, the imaginary part disappears at this point and $s_+(t)$ is purely real. | ||
| + | |||
| − | |||
| − | |||
| − | |||
| − | '''3 | + | '''(3)''' The period duration of the carrier signal is $T_0 = 1/f_T = 20 \ {\rm µ} \text{s}$. |
| + | *After $t = 5 \ {\rm µ} \text{s}$ (see middle graph) the carrier has thus rotated by $90^{\circ}$. | ||
| + | *The blue pointer $\rm (OSB)$ rotates $20\%$ faster, the green one $\rm (USB)$ $20\%$ slower than the red rotary pointer (carrier signal): | ||
| − | $$ | + | :$$s_{+}({\rm 5 \hspace{0.05cm} {\rm µ} s}) = {\rm 1 |
\hspace{0.05cm} V} \cdot {\rm e}^{{\rm j}\hspace{0.05cm} 2 \pi | \hspace{0.05cm} V} \cdot {\rm e}^{{\rm j}\hspace{0.05cm} 2 \pi | ||
\hspace{0.03cm} \cdot \hspace{0.08cm}50 \hspace{0.03cm} \cdot | \hspace{0.03cm} \cdot \hspace{0.08cm}50 \hspace{0.03cm} \cdot | ||
| Line 89: | Line 116: | ||
}+ {\rm 0.4 \hspace{0.05cm} V} \cdot {\rm e}^{{\rm | }+ {\rm 0.4 \hspace{0.05cm} V} \cdot {\rm e}^{{\rm | ||
j}\hspace{0.05cm} 2 \pi \hspace{0.03cm} \cdot \hspace{0.08cm}40 | j}\hspace{0.05cm} 2 \pi \hspace{0.03cm} \cdot \hspace{0.08cm}40 | ||
| − | \hspace{0.03cm} \cdot \hspace{0.08cm}0.005 } | + | \hspace{0.03cm} \cdot \hspace{0.08cm}0.005 } = {\rm 1 |
\hspace{0.05cm} V} \cdot {\rm e}^{{\rm j}\hspace{0.05cm} 90^\circ | \hspace{0.05cm} V} \cdot {\rm e}^{{\rm j}\hspace{0.05cm} 90^\circ | ||
}+ {\rm 0.4 \hspace{0.05cm} V} \cdot {\rm e}^{{\rm | }+ {\rm 0.4 \hspace{0.05cm} V} \cdot {\rm e}^{{\rm | ||
j}\hspace{0.05cm} 108^\circ }+{\rm 0.4 \hspace{0.05cm} V} \cdot | j}\hspace{0.05cm} 108^\circ }+{\rm 0.4 \hspace{0.05cm} V} \cdot | ||
| − | {\rm e}^{{\rm j}\hspace{0.05cm} 72^\circ }. | + | {\rm e}^{{\rm j}\hspace{0.05cm} 72^\circ }.$$ |
| − | + | *Thus, the angles travelled in $ 5 \ {\rm µ} \text{s}$ by OSB and USB are $108^{\circ}$ and $72^{\circ}$ respectively. | |
| + | *Since at this time the real parts of OSB and USB compensate, $s_+(t=5 \ {\rm µ} \text{s})$ is <u>purely imaginary</u> and we obtain: | ||
| − | $${\rm Im}\left[s_{+}(t = {\rm 5 \hspace{0.05cm} \ | + | :$${\rm Im}\left[s_{+}(t = {\rm 5 \hspace{0.05cm} {\rm µ} s})\right] = |
{\rm 1 \hspace{0.05cm} V} + 2 \cdot {\rm 0.4 \hspace{0.05cm} | {\rm 1 \hspace{0.05cm} V} + 2 \cdot {\rm 0.4 \hspace{0.05cm} | ||
V}\cdot \cos (18^\circ ) \hspace{0.15 cm}\underline{= {\rm 1.761 \hspace{0.05cm} V}}.$$ | V}\cdot \cos (18^\circ ) \hspace{0.15 cm}\underline{= {\rm 1.761 \hspace{0.05cm} V}}.$$ | ||
| − | '''4 | + | |
| + | |||
| + | '''(4)''' After one rotation of the red carrier, i.e. at time $t$ = $T_0 = 20 \ {\rm µ} \text{s}$, the blue pointer has already covered $72^{\circ}$ more and the green pointer correspondingly $72^{\circ}$ less. The sum of the three pointers is again <u>real</u> and results in accordance with the graph on the right: | ||
| − | $${\rm Re}\left[s_{+}({\rm 20 \hspace{0.05cm} \ | + | :$${\rm Re}\left[s_{+}({\rm 20 \hspace{0.05cm} {\rm µ} s})\right] = |
{\rm 1 \hspace{0.05cm} V} + 2 \cdot {\rm 0.4 \hspace{0.05cm} | {\rm 1 \hspace{0.05cm} V} + 2 \cdot {\rm 0.4 \hspace{0.05cm} | ||
| − | V}\cdot \cos (72^\circ ) \hspace{0.15 cm}\underline{= {\rm 1. | + | V}\cdot \cos (72^\circ ) \hspace{0.15 cm}\underline{= {\rm 1.236 \hspace{0.05cm} V}}.$$ |
| + | |||
| − | '''5 | + | '''(5)''' The magnitude is minimum when the pointers of the two sidebands are offset from the carrier by $180^{\circ}$ . It follows: |
| − | $$|s_{+}(t)|_{\rm min} = {\rm 1 \hspace{0.05cm} V} - 2 \cdot {\rm | + | :$$|s_{+}(t)|_{\rm min} = {\rm 1 \hspace{0.05cm} V} - 2 \cdot {\rm |
0.4 \hspace{0.05cm} V} \hspace{0.15 cm}\underline{= {\rm 0.2 \hspace{0.05cm} V}}.$$ | 0.4 \hspace{0.05cm} V} \hspace{0.15 cm}\underline{= {\rm 0.2 \hspace{0.05cm} V}}.$$ | ||
| − | + | Within one period $T_0$ of the carrier, a phase offset of $\pm72^{\circ}$ occurs with respect to the pointers of the two sidebands. From this follows: | |
| + | :$$t_{\text{min}} = 180^{\circ}/72^{\circ} \cdot T_0 = 2.5 \cdot T_0 \;\underline{= 50 \ {\rm µ} \text{s}}.$$ | ||
{{ML-Fuß}} | {{ML-Fuß}} | ||
__NOEDITSECTION__ | __NOEDITSECTION__ | ||
| − | [[Category: | + | [[Category:Signal Representation: Exercises|^4.2 Analytical Signal and its Spectral Function^]] |
Latest revision as of 14:29, 7 May 2021
We assume a cosine-shaped source signal $q(t)$ with
- amplitude $A_{\rm N} = 0.8 \ \text{V}$ and
- frequency $f_{\rm N}= 10 \ \text{kHz}$.
The frequency conversion is done by means of "Double-Sideband Amplitude Modulation with Carrier".
The modulated signal $s(t)$ is with the (normalised) carrier $z(t) = \text{cos}(\omega_{\rm T} \cdot t)$ and the DC component $q_0 = 1 \ \text{V}$:
- $$\begin{align*} s(t) & = \left(q_0 + q(t)\right) \cdot z(t)= \left({\rm 1 \hspace{0.05cm} V} + {\rm 0.8 \hspace{0.05cm}V}\cdot {\cos} ( \omega_{\rm N}\cdot t)\right) \cdot {\cos} ( \omega_{\rm T}\cdot t) = \\ & = q_0 \cdot {\cos} ( \omega_{\rm T}\cdot t) + {A_{\rm N}}/{2} \cdot {\cos} ( (\omega_{\rm T}+ \omega_{\rm N}) \cdot t) + {A_{\rm N}}/{2} \cdot {\cos} ( (\omega_{\rm T}- \omega_{\rm N}) \cdot t).\end{align*}$$
The first term describes the carrier, the second term the so-called upper sideband $\rm (OSB)$ and the last term the lower sideband $\rm (USB)$.
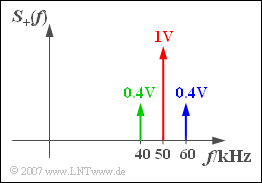
The sketch shows the spectrum $S_+(f)$ of the corresponding analytical signal for $f_{\rm T} = 50 \ \text{kHz}$. You can see
- the carrier (red),
- the upper sideband (blue), and
- the lower sideband (grün).
In subtask (5) the magnitude of $s_+(t)$ is asked for. This is the length of the resulting pointer.
Hints:
- This exercise belongs to the chapter Analytical Signal and its Spectral Function.
- The interactive applet Physical and Analytical Signal illustrates the topic covered here.
- In this task we use the following nomenclature because of the German original:
- The index $\rm N$ stands for "source signal" ⇒ (German: "Nachrichtenignal").
- The index $\rm T$ stands for "carrier" ⇒ (German: "Trägersignal").
- $\rm OSB$ denotes the "upper sideband" ⇒ (German: "oberes Seitenband").
- $\rm USB$ denotes the "lower sideband" ⇒ (German: "unteres Seitenband").
Questions
Solution
- $$s_{+}(t) = {\rm 1 \hspace{0.05cm} V} \cdot {\rm e}^{{\rm j}\hspace{0.05cm} \omega_{\rm 50}\hspace{0.05cm} t } + {\rm 0.4 \hspace{0.05cm} V} \cdot {\rm e}^{{\rm j}\hspace{0.05cm} \omega_{\rm 60} \hspace{0.05cm} t }+ {\rm 0.4 \hspace{0.05cm} V} \cdot {\rm e}^{{\rm j}\hspace{0.05cm} \omega_{\rm 40}\hspace{0.05cm} t }.$$
- The expression describes the sum of three pointers rotating at different circular velocities.
- In the above equation, for example, $\omega_{60} = 2\pi (f_{\rm T} + f_{\rm N}) = 2\pi \cdot 60 \ \text{kHz}$.
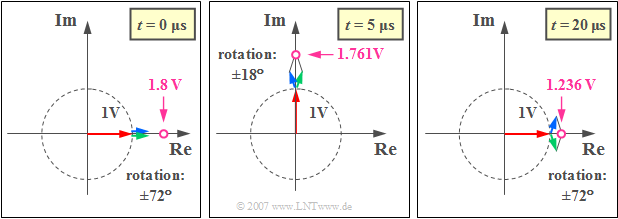
- At time $t = 0$ all three pointers point in the direction of the real axis (see left graph).
- One obtains the real value $s_+(t = 0) \;\underline{= 1.8 \ \text{V}}$.
(2) The first statement is correct and results from the "Hilbert transform". On the other hand, the next two statements are'nt correct:
- $s_+(t)$ is always a complex time function with exception of the limiting case $s(t) \equiv 0$.
- However, every complex function also has purely real values at some points in time.
- The "pointer group" always rotates in a mathematically positive direction.
- If the sum vector crosses the real axis, the imaginary part disappears at this point and $s_+(t)$ is purely real.
(3) The period duration of the carrier signal is $T_0 = 1/f_T = 20 \ {\rm µ} \text{s}$.
- After $t = 5 \ {\rm µ} \text{s}$ (see middle graph) the carrier has thus rotated by $90^{\circ}$.
- The blue pointer $\rm (OSB)$ rotates $20\%$ faster, the green one $\rm (USB)$ $20\%$ slower than the red rotary pointer (carrier signal):
- $$s_{+}({\rm 5 \hspace{0.05cm} {\rm µ} s}) = {\rm 1 \hspace{0.05cm} V} \cdot {\rm e}^{{\rm j}\hspace{0.05cm} 2 \pi \hspace{0.03cm} \cdot \hspace{0.08cm}50 \hspace{0.03cm} \cdot \hspace{0.08cm}0.005 } + {\rm 0.4 \hspace{0.05cm} V} \cdot {\rm e}^{{\rm j}\hspace{0.05cm} 2 \pi \hspace{0.03cm} \cdot \hspace{0.08cm}60 \hspace{0.03cm} \cdot \hspace{0.08cm}0.005 }+ {\rm 0.4 \hspace{0.05cm} V} \cdot {\rm e}^{{\rm j}\hspace{0.05cm} 2 \pi \hspace{0.03cm} \cdot \hspace{0.08cm}40 \hspace{0.03cm} \cdot \hspace{0.08cm}0.005 } = {\rm 1 \hspace{0.05cm} V} \cdot {\rm e}^{{\rm j}\hspace{0.05cm} 90^\circ }+ {\rm 0.4 \hspace{0.05cm} V} \cdot {\rm e}^{{\rm j}\hspace{0.05cm} 108^\circ }+{\rm 0.4 \hspace{0.05cm} V} \cdot {\rm e}^{{\rm j}\hspace{0.05cm} 72^\circ }.$$
- Thus, the angles travelled in $ 5 \ {\rm µ} \text{s}$ by OSB and USB are $108^{\circ}$ and $72^{\circ}$ respectively.
- Since at this time the real parts of OSB and USB compensate, $s_+(t=5 \ {\rm µ} \text{s})$ is purely imaginary and we obtain:
- $${\rm Im}\left[s_{+}(t = {\rm 5 \hspace{0.05cm} {\rm µ} s})\right] = {\rm 1 \hspace{0.05cm} V} + 2 \cdot {\rm 0.4 \hspace{0.05cm} V}\cdot \cos (18^\circ ) \hspace{0.15 cm}\underline{= {\rm 1.761 \hspace{0.05cm} V}}.$$
(4) After one rotation of the red carrier, i.e. at time $t$ = $T_0 = 20 \ {\rm µ} \text{s}$, the blue pointer has already covered $72^{\circ}$ more and the green pointer correspondingly $72^{\circ}$ less. The sum of the three pointers is again real and results in accordance with the graph on the right:
- $${\rm Re}\left[s_{+}({\rm 20 \hspace{0.05cm} {\rm µ} s})\right] = {\rm 1 \hspace{0.05cm} V} + 2 \cdot {\rm 0.4 \hspace{0.05cm} V}\cdot \cos (72^\circ ) \hspace{0.15 cm}\underline{= {\rm 1.236 \hspace{0.05cm} V}}.$$
(5) The magnitude is minimum when the pointers of the two sidebands are offset from the carrier by $180^{\circ}$ . It follows:
- $$|s_{+}(t)|_{\rm min} = {\rm 1 \hspace{0.05cm} V} - 2 \cdot {\rm 0.4 \hspace{0.05cm} V} \hspace{0.15 cm}\underline{= {\rm 0.2 \hspace{0.05cm} V}}.$$
Within one period $T_0$ of the carrier, a phase offset of $\pm72^{\circ}$ occurs with respect to the pointers of the two sidebands. From this follows:
- $$t_{\text{min}} = 180^{\circ}/72^{\circ} \cdot T_0 = 2.5 \cdot T_0 \;\underline{= 50 \ {\rm µ} \text{s}}.$$