Difference between revisions of "Aufgaben:Exercise 5.2Z: DFT of a Triangular Pulse"
| (23 intermediate revisions by 5 users not shown) | |||
| Line 1: | Line 1: | ||
| − | {{quiz-Header|Buchseite= | + | {{quiz-Header|Buchseite=Signal_Representation/Discrete_Fourier_Transform_(DFT) |
}} | }} | ||
| − | [[File:P_ID1140__Sig_Z_5_2.png|right| | + | [[File:P_ID1140__Sig_Z_5_2.png|right|frame|Discretisation of a triangular pulse]] |
| − | + | Consider the sketched triangular pulse | |
:$$x(t) = \left\{ \begin{array}{l} A \cdot \left( 1 - {|t|}/{T} \right ) \\ | :$$x(t) = \left\{ \begin{array}{l} A \cdot \left( 1 - {|t|}/{T} \right ) \\ | ||
\hspace{0.25cm} 0 \\ \end{array} \right.\quad | \hspace{0.25cm} 0 \\ \end{array} \right.\quad | ||
| − | \begin{array}{*{10}c} {\rm{ | + | \begin{array}{*{10}c} {\rm{for}} |
| − | \\ {\rm{ | + | \\ {\rm{for}} \\ \end{array}\begin{array}{*{10}c} |
{\left| \hspace{0.005cm} t\hspace{0.05cm} \right| \le T,} \\ | {\left| \hspace{0.005cm} t\hspace{0.05cm} \right| \le T,} \\ | ||
{\left|\hspace{0.005cm} t \hspace{0.05cm} \right| > T.} \\ | {\left|\hspace{0.005cm} t \hspace{0.05cm} \right| > T.} \\ | ||
\end{array}$$ | \end{array}$$ | ||
| − | + | The signal parameters have the following values: | |
| − | + | * amplitude $A = 4 \ \text{V}$, | |
| − | + | * equivalent pulse duration $\Delta t = T = 1 \, \text{ms}$. | |
| − | |||
| − | |||
| − | + | The spectrum $X(f)$ is obtained by applying [[Signal_Representation/Fourier_Transform_and_its_Inverse#The_first_Fourier_integral|the first Fourier Integral]]: | |
| + | :$$X(f) = A \cdot T \cdot {\rm si}^2(\pi f T)\hspace{0.5cm}\text{with}\hspace{0.5cm}{\rm si}(x)=\sin(x)/x\hspace{0.05cm}.$$ | ||
| − | + | The spectral function is now to be approximated by a Discrete Fourier Transform $\rm (DFT)$ with $N = 8$ , where the $N$ coefficients for the time domain ⇒ $d(0)$, ... , $d(7)$ can be taken from the graph. | |
| + | |||
| + | The corresponding spectral coefficients $D(0)$, ... , $D(7)$ are to be determined. For the indices $\mu = 0$, ... , $N–1$ applies: | ||
:$$D(\mu) = \frac{1}{N} \cdot \sum_{\nu = 0 }^{N-1} | :$$D(\mu) = \frac{1}{N} \cdot \sum_{\nu = 0 }^{N-1} | ||
| − | d(\nu)\cdot {w}^{\hspace{0.05cm}\nu \hspace{0. | + | d(\nu)\cdot {w}^{\hspace{0.05cm}\nu \hspace{0.05cm} \cdot \hspace{0.05cm}\mu} \hspace{0.05cm}.$$ |
| − | + | ||
| + | If we denote the distance between two samples in the time domain by $T_{\rm A}$ and the corresponding frequency distance between two lines by $f_{\rm A}$, following relationship applies: | ||
:$$N \cdot f_{\rm A} \cdot T_{\rm A} = 1 \hspace{0.05cm}.$$ | :$$N \cdot f_{\rm A} \cdot T_{\rm A} = 1 \hspace{0.05cm}.$$ | ||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | === | + | |
| + | |||
| + | |||
| + | |||
| + | ''Hints:'' | ||
| + | *This task belongs to the chapter [[Signal_Representation/Discrete_Fourier_Transform_(DFT)|Discrete Fourier Transformation (DFT)]]. | ||
| + | |||
| + | *The topic dealt with here is also dealt with in the interactive applet [[Applets:Discrete_Fouriertransform_and_Inverse|Discrete Fourier Transform and Inverse]]. | ||
| + | |||
| + | |||
| + | |||
| + | |||
| + | |||
| + | |||
| + | |||
| + | ===Question=== | ||
<quiz display=simple> | <quiz display=simple> | ||
| − | { | + | {Give the time coefficients. What are the values of $d(0)$, $d(3)$ and $d(6)$? |
|type="{}"} | |type="{}"} | ||
| − | $d(0)$ | + | $d(0)\ = \ $ { 4 3% } $\text{V}$ |
| − | $d(3)$ | + | $d(3)\ = \ $ { 1 3% } $\text{V}$ |
| − | $d(6)$ | + | $d(6)\ = \ $ { 2 3% } $\text{V}$ |
| − | { | + | {What is the distance $T_{\rm A}$ between two time samples? |
|type="{}"} | |type="{}"} | ||
| − | $T_{\rm A}$ | + | $T_{\rm A}\ = \ ${ 0.25 3% } $\text{ms}$ |
| − | { | + | {What is the distance $f_{\rm A}$ between two DFT frequency samples? |
|type="{}"} | |type="{}"} | ||
| − | $f_{\rm A}$ | + | $f_{\rm A}\ = \ ${ 0.5 3% } $\text{kHz}$ |
| − | { | + | {Calculate the coefficient $D(0)$ and interpret the result. |
|type="{}"} | |type="{}"} | ||
| − | $D(0)$ | + | $D(0)\ = \ $ { 2 3% } $\text{V}$ |
| − | { | + | {Calculate the coefficient $D(2)$ and interpret the result, also in terms of the coefficients $D(4)$ and $D(6)$. |
|type="{}"} | |type="{}"} | ||
| − | $D(2)$ | + | $D(2)\ = \ ${ 0. } $\text{V}$ |
| − | { | + | {Calculate and interpret the DFT coefficient $D(7)$. |
|type="{}"} | |type="{}"} | ||
| − | $D(7)$ | + | $D(7)\ = \ $ { 0.854 3% } $\text{V}$ |
| Line 74: | Line 86: | ||
</quiz> | </quiz> | ||
| − | === | + | ===Solution=== |
{{ML-Kopf}} | {{ML-Kopf}} | ||
| − | '''1 | + | '''(1)''' From the graph the following values result with $A = 4 \ {\rm V}$ : |
| − | :$$ | + | :$${d(0) = 4\,{\rm V}, \hspace{0.1cm}d(1) = d(7) = 3\,{\rm V}, \hspace{0.1cm} |
\hspace{0.1cm}d(2) = d(6) = 2\,{\rm V}, \hspace{0.1cm} | \hspace{0.1cm}d(2) = d(6) = 2\,{\rm V}, \hspace{0.1cm} | ||
\hspace{0.1cm}d(3) = d(5) = 1\,{\rm V}, \hspace{0.1cm} | \hspace{0.1cm}d(3) = d(5) = 1\,{\rm V}, \hspace{0.1cm} | ||
\hspace{0.1cm}d(4) = 0}\hspace{0.05cm}. $$ | \hspace{0.1cm}d(4) = 0}\hspace{0.05cm}. $$ | ||
| + | :$$\Rightarrow \hspace{0.15 cm}\underline{d(0) = 4\,{\rm V}, \hspace{0.1cm}d(3) = 1\,{\rm V}, | ||
| + | \hspace{0.1cm}d(6) = 2\,{\rm V}. \hspace{0.1cm}} | ||
| + | \hspace{0.05cm} $$ | ||
| + | |||
| + | |||
| + | '''(2)''' According to the diagram $T_{\rm A} = T/4$. | ||
| + | *With $T = 1 \ \text{ms}$ one obtains $\underline{T_{\rm A} = 0.25 \ \text{ms}}$. | ||
| − | |||
| − | '''3 | + | |
| + | '''(3)''' For the distances of the samples in the time and frequency domain applies: | ||
:$$N \cdot f_{\rm A} \cdot T_{\rm A} = 1 \hspace{0.3cm}\Rightarrow \hspace{0.3cm}f_{\rm | :$$N \cdot f_{\rm A} \cdot T_{\rm A} = 1 \hspace{0.3cm}\Rightarrow \hspace{0.3cm}f_{\rm | ||
A}= \frac{1}{ 8 \cdot 0.25\, {\rm ms}}\hspace{0.15 cm}\underline{= 0.5\, {\rm kHz}}\hspace{0.05cm}.$$ | A}= \frac{1}{ 8 \cdot 0.25\, {\rm ms}}\hspace{0.15 cm}\underline{= 0.5\, {\rm kHz}}\hspace{0.05cm}.$$ | ||
| − | '''4 | + | |
| + | '''(4)''' With $N = 8$ and $\mu = 0$ , it follows from the DFT equation: | ||
:$$D(0) = \frac{1}{8}\cdot \sum_{\nu = 0 }^{7} | :$$D(0) = \frac{1}{8}\cdot \sum_{\nu = 0 }^{7} | ||
d(\nu) = \frac{1\,{\rm V}}{8}\cdot (4+3+2+1+0+1+2+3)\hspace{0.15 cm}\underline{= 2 \,{\rm V}}\hspace{0.05cm}.$$ | d(\nu) = \frac{1\,{\rm V}}{8}\cdot (4+3+2+1+0+1+2+3)\hspace{0.15 cm}\underline{= 2 \,{\rm V}}\hspace{0.05cm}.$$ | ||
| − | + | *The DFT value $D(0)$ describes the spectral value at $f = 0$, where the following relation holds: | |
:$$X(f=0) = \frac{D(0)}{f_{\rm A}}= \frac{ 2\,{\rm V}}{0.5\,{\rm kHz}}= 4 \cdot 10^{-3}\,{\rm V/Hz}\hspace{0.05cm}.$$ | :$$X(f=0) = \frac{D(0)}{f_{\rm A}}= \frac{ 2\,{\rm V}}{0.5\,{\rm kHz}}= 4 \cdot 10^{-3}\,{\rm V/Hz}\hspace{0.05cm}.$$ | ||
| − | + | *This value agrees with the theoretical value $(A \cdot T)$ . | |
| − | '''5 | + | |
| + | |||
| + | '''(5)''' With $N = 8$ and $\mu = 2$ we obtain: | ||
:$$D(2) = \frac{1}{8}\cdot \sum_{\nu = 0 }^{7} | :$$D(2) = \frac{1}{8}\cdot \sum_{\nu = 0 }^{7} | ||
d(\nu)\cdot {\rm e}^{-{\rm j} \hspace{0.05cm}\cdot \hspace{0.05cm} (\pi /2) \hspace{0.05cm}\cdot \hspace{0.05cm} \nu} = | d(\nu)\cdot {\rm e}^{-{\rm j} \hspace{0.05cm}\cdot \hspace{0.05cm} (\pi /2) \hspace{0.05cm}\cdot \hspace{0.05cm} \nu} = | ||
\frac{1}{8}\cdot \sum_{\nu = 0 }^{7} | \frac{1}{8}\cdot \sum_{\nu = 0 }^{7} | ||
| − | d(\nu)\cdot (-{\rm j})^{\nu} | + | d(\nu)\cdot (-{\rm j})^{\nu} \hspace{0.3cm} |
| − | + | \Rightarrow \hspace{0.3cm} = \frac{1\,{\rm V}}{8}\cdot (4-3\cdot{\rm j}-2+{\rm j}-{\rm j}-2+3\cdot{\rm j})\hspace{0.15 cm}\underline{= 0}\hspace{0.05cm}.$$ | |
| − | + | This result could have been predicted without calculation: | |
| + | *The DFT coefficients $D(\mu)$ are at the same time the Fourier coefficients of the function $x_{\rm Per}(t)$ periodised at the distance $T_{\rm P} = 2T$. <br>This is shown as a dashed line in the graph on the information page. | ||
| + | *Due to symmetry properties, however, all even Fourier coefficients of the function $x_{\rm Per}(t)$ are equal to zero ⇒ $D(4)\hspace{0.15cm}\underline{=0},$ $D(6)\hspace{0.15cm}\underline{=0}$. | ||
| − | '''6 | + | |
| + | |||
| + | '''(6)''' The coefficient $D(7)$ describes the periodised spectral function at the frequency $f = 7 \cdot f_{\rm A}$. Due to periodicity and symmetry property holds: | ||
:$$D(7) = D(-1) = D^{\star}(1) \hspace{0.05cm}.$$ | :$$D(7) = D(-1) = D^{\star}(1) \hspace{0.05cm}.$$ | ||
| − | + | Preferably, we calculate this DFT coefficient: | |
:$$D(1) = \frac{1}{8}\cdot \sum_{\nu = 0 }^{7} | :$$D(1) = \frac{1}{8}\cdot \sum_{\nu = 0 }^{7} | ||
d(\nu)\cdot {\rm e}^{-{\rm j} \hspace{0.05cm}\cdot \hspace{0.05cm} (\pi /4) \hspace{0.05cm}\cdot \hspace{0.05cm} \nu} = | d(\nu)\cdot {\rm e}^{-{\rm j} \hspace{0.05cm}\cdot \hspace{0.05cm} (\pi /4) \hspace{0.05cm}\cdot \hspace{0.05cm} \nu} = | ||
| − | \frac{1\,{\rm V}}{8}\cdot | + | \frac{1\,{\rm V}}{8}\cdot \left(4 +3\cdot \frac{1 - {\rm j}}{\sqrt{2}}-2\cdot {\rm j}+ \frac{-1 - {\rm j}}{\sqrt{2}}-{\rm j}+ |
| − | \frac{-1 + {\rm j}}{\sqrt{2}}-{\rm j}+ 2\cdot {\rm j}+3\cdot \frac{1 - {\rm j}}{\sqrt{2}}\right) | + | \frac{-1 + {\rm j}}{\sqrt{2}}-{\rm j}+ 2\cdot {\rm j}+3\cdot \frac{1 - {\rm j}}{\sqrt{2}}\right)$$ |
| − | + | :$$\Rightarrow \; \; D(1) = \frac{2 + \sqrt{2}}{4} \approx 0.854{\rm V}\hspace{0.05cm}.$$ | |
| − | + | Since $D(1)$ is purely real, $D(7) = D(1) \; \underline{= 0.854 \ {\rm V}}$. | |
| + | |||
| + | This gives for the corresponding values of the continuous spectral function: | ||
:$$X(f=-f_{\rm A}) = X(f=+f_{\rm A}) =\frac{D(1)}{f_{\rm A}}= 1.708 \cdot 10^{-3}\,{\rm V/Hz}\hspace{0.05cm}.$$ | :$$X(f=-f_{\rm A}) = X(f=+f_{\rm A}) =\frac{D(1)}{f_{\rm A}}= 1.708 \cdot 10^{-3}\,{\rm V/Hz}\hspace{0.05cm}.$$ | ||
| − | + | *Because of the implicit periodic continuation by the DFT, the value calculated in this way does not exactly match the actual value $(4 \cdot A \cdot T/\pi^2 = 1.621 · 10^{-3}\text{ V/Hz})$. | |
| + | *The relative error is approx. $5.3\%$. | ||
{{ML-Fuß}} | {{ML-Fuß}} | ||
__NOEDITSECTION__ | __NOEDITSECTION__ | ||
| − | [[Category: | + | [[Category:Signal Representation: Exercises|^5.2 Discrete Fourier Transform^]] |
Latest revision as of 16:59, 16 May 2021
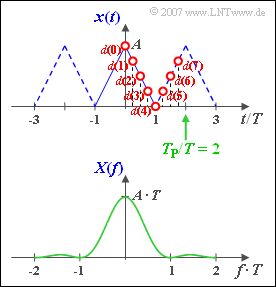
Consider the sketched triangular pulse
- $$x(t) = \left\{ \begin{array}{l} A \cdot \left( 1 - {|t|}/{T} \right ) \\ \hspace{0.25cm} 0 \\ \end{array} \right.\quad \begin{array}{*{10}c} {\rm{for}} \\ {\rm{for}} \\ \end{array}\begin{array}{*{10}c} {\left| \hspace{0.005cm} t\hspace{0.05cm} \right| \le T,} \\ {\left|\hspace{0.005cm} t \hspace{0.05cm} \right| > T.} \\ \end{array}$$
The signal parameters have the following values:
- amplitude $A = 4 \ \text{V}$,
- equivalent pulse duration $\Delta t = T = 1 \, \text{ms}$.
The spectrum $X(f)$ is obtained by applying the first Fourier Integral:
- $$X(f) = A \cdot T \cdot {\rm si}^2(\pi f T)\hspace{0.5cm}\text{with}\hspace{0.5cm}{\rm si}(x)=\sin(x)/x\hspace{0.05cm}.$$
The spectral function is now to be approximated by a Discrete Fourier Transform $\rm (DFT)$ with $N = 8$ , where the $N$ coefficients for the time domain ⇒ $d(0)$, ... , $d(7)$ can be taken from the graph.
The corresponding spectral coefficients $D(0)$, ... , $D(7)$ are to be determined. For the indices $\mu = 0$, ... , $N–1$ applies:
- $$D(\mu) = \frac{1}{N} \cdot \sum_{\nu = 0 }^{N-1} d(\nu)\cdot {w}^{\hspace{0.05cm}\nu \hspace{0.05cm} \cdot \hspace{0.05cm}\mu} \hspace{0.05cm}.$$
If we denote the distance between two samples in the time domain by $T_{\rm A}$ and the corresponding frequency distance between two lines by $f_{\rm A}$, following relationship applies:
- $$N \cdot f_{\rm A} \cdot T_{\rm A} = 1 \hspace{0.05cm}.$$
Hints:
- This task belongs to the chapter Discrete Fourier Transformation (DFT).
- The topic dealt with here is also dealt with in the interactive applet Discrete Fourier Transform and Inverse.
Question
Solution
- $${d(0) = 4\,{\rm V}, \hspace{0.1cm}d(1) = d(7) = 3\,{\rm V}, \hspace{0.1cm} \hspace{0.1cm}d(2) = d(6) = 2\,{\rm V}, \hspace{0.1cm} \hspace{0.1cm}d(3) = d(5) = 1\,{\rm V}, \hspace{0.1cm} \hspace{0.1cm}d(4) = 0}\hspace{0.05cm}. $$
- $$\Rightarrow \hspace{0.15 cm}\underline{d(0) = 4\,{\rm V}, \hspace{0.1cm}d(3) = 1\,{\rm V}, \hspace{0.1cm}d(6) = 2\,{\rm V}. \hspace{0.1cm}} \hspace{0.05cm} $$
(2) According to the diagram $T_{\rm A} = T/4$.
- With $T = 1 \ \text{ms}$ one obtains $\underline{T_{\rm A} = 0.25 \ \text{ms}}$.
(3) For the distances of the samples in the time and frequency domain applies:
- $$N \cdot f_{\rm A} \cdot T_{\rm A} = 1 \hspace{0.3cm}\Rightarrow \hspace{0.3cm}f_{\rm A}= \frac{1}{ 8 \cdot 0.25\, {\rm ms}}\hspace{0.15 cm}\underline{= 0.5\, {\rm kHz}}\hspace{0.05cm}.$$
(4) With $N = 8$ and $\mu = 0$ , it follows from the DFT equation:
- $$D(0) = \frac{1}{8}\cdot \sum_{\nu = 0 }^{7} d(\nu) = \frac{1\,{\rm V}}{8}\cdot (4+3+2+1+0+1+2+3)\hspace{0.15 cm}\underline{= 2 \,{\rm V}}\hspace{0.05cm}.$$
- The DFT value $D(0)$ describes the spectral value at $f = 0$, where the following relation holds:
- $$X(f=0) = \frac{D(0)}{f_{\rm A}}= \frac{ 2\,{\rm V}}{0.5\,{\rm kHz}}= 4 \cdot 10^{-3}\,{\rm V/Hz}\hspace{0.05cm}.$$
- This value agrees with the theoretical value $(A \cdot T)$ .
(5) With $N = 8$ and $\mu = 2$ we obtain:
- $$D(2) = \frac{1}{8}\cdot \sum_{\nu = 0 }^{7} d(\nu)\cdot {\rm e}^{-{\rm j} \hspace{0.05cm}\cdot \hspace{0.05cm} (\pi /2) \hspace{0.05cm}\cdot \hspace{0.05cm} \nu} = \frac{1}{8}\cdot \sum_{\nu = 0 }^{7} d(\nu)\cdot (-{\rm j})^{\nu} \hspace{0.3cm} \Rightarrow \hspace{0.3cm} = \frac{1\,{\rm V}}{8}\cdot (4-3\cdot{\rm j}-2+{\rm j}-{\rm j}-2+3\cdot{\rm j})\hspace{0.15 cm}\underline{= 0}\hspace{0.05cm}.$$
This result could have been predicted without calculation:
- The DFT coefficients $D(\mu)$ are at the same time the Fourier coefficients of the function $x_{\rm Per}(t)$ periodised at the distance $T_{\rm P} = 2T$.
This is shown as a dashed line in the graph on the information page. - Due to symmetry properties, however, all even Fourier coefficients of the function $x_{\rm Per}(t)$ are equal to zero ⇒ $D(4)\hspace{0.15cm}\underline{=0},$ $D(6)\hspace{0.15cm}\underline{=0}$.
(6) The coefficient $D(7)$ describes the periodised spectral function at the frequency $f = 7 \cdot f_{\rm A}$. Due to periodicity and symmetry property holds:
- $$D(7) = D(-1) = D^{\star}(1) \hspace{0.05cm}.$$
Preferably, we calculate this DFT coefficient:
- $$D(1) = \frac{1}{8}\cdot \sum_{\nu = 0 }^{7} d(\nu)\cdot {\rm e}^{-{\rm j} \hspace{0.05cm}\cdot \hspace{0.05cm} (\pi /4) \hspace{0.05cm}\cdot \hspace{0.05cm} \nu} = \frac{1\,{\rm V}}{8}\cdot \left(4 +3\cdot \frac{1 - {\rm j}}{\sqrt{2}}-2\cdot {\rm j}+ \frac{-1 - {\rm j}}{\sqrt{2}}-{\rm j}+ \frac{-1 + {\rm j}}{\sqrt{2}}-{\rm j}+ 2\cdot {\rm j}+3\cdot \frac{1 - {\rm j}}{\sqrt{2}}\right)$$
- $$\Rightarrow \; \; D(1) = \frac{2 + \sqrt{2}}{4} \approx 0.854{\rm V}\hspace{0.05cm}.$$
Since $D(1)$ is purely real, $D(7) = D(1) \; \underline{= 0.854 \ {\rm V}}$.
This gives for the corresponding values of the continuous spectral function:
- $$X(f=-f_{\rm A}) = X(f=+f_{\rm A}) =\frac{D(1)}{f_{\rm A}}= 1.708 \cdot 10^{-3}\,{\rm V/Hz}\hspace{0.05cm}.$$
- Because of the implicit periodic continuation by the DFT, the value calculated in this way does not exactly match the actual value $(4 \cdot A \cdot T/\pi^2 = 1.621 · 10^{-3}\text{ V/Hz})$.
- The relative error is approx. $5.3\%$.