Aufgaben:Exercise 2.1: Linear? Or Non-Linear?

• Return to book

Interconnected system

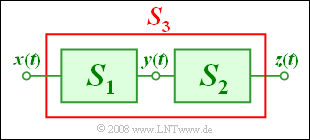
We consider the sketched arrangement with input  $x(t)$  and output $z(t)$:

• The system $S_1$  is describable by the following equation:

$$y(t) = x(t) + {1 \, \rm V}^{\rm -1} \cdot x^2(t) .$$

• Nothing else is known about the system  $S_2$  with input  $y(t)$  and output  $z(t)$ .

• The system $S_3$  is the interconnection of  $S_1$  and  $S_2$.

An oscillation with frequency  $f_0 = 5 \ \rm kHz$  is applied to the input:

$$x(t) = {2 \, \rm V} \cdot {\rm cos}(2\pi f_0 t ) .$$

Hence, at the output of the overall system  $S_3$ the following is obtained:

$$z(t) = {1 \, \rm V} \cdot {\rm sin}(2\pi f_0 t ) .$$

Please note:

• The exercise belongs to the chapter  Classification of Distortions.

• The following trigonometric relation is given:

$$\cos^2(\alpha) = {1}/{2} \cdot \big[ 1 + \cos(2\alpha)\big].$$

Questions

1

What is the signal $y(t)$? What is the signal value at time zero?

$y(t = 0) \ = \ $ $\ \rm V$

2

What correct conclusions could be drawn by an observer who only knows the signals $x(t)$  and  $z(t)$  but not the structure of  $S_3$?

	$S_3$  is an ideal system.
	$S_3$  is a distortion-free system.
	$S_3$  is a linearly distorting system.
	$S_3$  is a non-linearly distorting system.

3

What conclusions would the observer have to draw if all the information from the information page is known to them?

	$S_2$  is a distortion-free system.
	$S_2$  is a linearly distorting system.
	$S_2$  is a non-linearly distorting system.

4

What signal $z(t)$  could arise as a result with the input frequency $f_0 = 10 \ \rm kHz$ ?

	The signal  $z(t)$  is zero for all times.
	A signal of the form $z(t) = A \cdot {\rm cos}(2\pi \cdot 10 \ {\rm kHz} \cdot t ) ,$ with $A \ne 0.$
	A signal of the form $z(t) = A \cdot {\rm cos}(2\pi \cdot 20 \ {\rm kHz} \cdot t ) ,$ with $A \ne 0.$

Solution

Solution

(1)  The following holds due to the characteristic curve with linear and quadratic components:

$$y(t) = {2 \, \rm V} \cdot {\rm cos}(2\pi f_0 t ) + {1 \, \rm V}^{\rm -1} \cdot ({2 \, \rm V})^2 \cdot {\rm cos}^2(2\pi f_0 t ) = {2 \, \rm V} \cdot \big[ 1 + {\rm cos}(2\pi \cdot f_0 \cdot t ) +{\rm cos}(2\pi \cdot 2f_0 \cdot t ) \big].$$

• Therefore, the signal value 6 V occurs at time  $t= 0$ .

(2)  Möglich sind die Alternativen 2 und 3:

• Ein ideales System kommt wegen  $z(t) ≠ x(t)$  nicht in Frage.
• Bei nur einer Eingangsfrequenz  $(f_0 = 5 \ \rm kHz)$  im Testsignal ist keine Aussage möglich, ob eine zweite Frequenzkomponente mit  $f \ne f_0$  ebenfalls um  $\alpha = 0.5$  gedämpft und um  $\tau = T_0/4 = 50 \ µ\rm s$  verzögert würde.
• Ergäbe sich für die zweite Frequenz  $\alpha = 0.5$  und  $\tau = T_0/4 = 50 \ µ \rm s$, so könnte ein verzerrungsfreies System vorliegen.
• Ergäbe sich für die zweite Frequenzkomponente  $\alpha \ne 0.5$  und/oder  $\tau \ne T_0/4$, so wäre das System linear verzerrend.
• Die letzte Alternative müsste der Beobachter – obwohl teilweise zutreffend – logischerweise verneinen.

(3)  Richtig ist der Lösungsvorschlag 2:

• Der Beobachter würde erkennen, dass  $S_2$  ein linear verzerrendes System ist.
• Bei einem verzerrungsfreien System müsste  $z(t)$  zusätzlich noch eine Gleichkomponente und eine   $10 \ \rm kHz$–Komponente beinhalten,
• bei einem nichtlinear verzerrenden System noch größere Frequenzanteile  $($bei Vielfachen von  $10 \ \rm kHz)$.

(4)  In diesem Fall würde gelten:

$$y(t) = {2 \, \rm V} \cdot \big[ 1 + {\rm cos}(2\pi \cdot 10 \ {\rm kHz} \cdot t ) +{\rm cos}(2\pi \cdot 20 \ {\rm kHz} \cdot t ) \big].$$

• Das heißt:   $Y(f)$  würde Spektrallinien bei  $f = 0$,  $10 \ \rm kHz$  und  $20 \ \rm kHz$  aufweisen.

• Die auf der Angabenseite beschriebene Messung mit  $f_0 = 5 \ \rm kHz$  hat aber gezeigt, dass  $H_2(f = 0) = H_2(f = 10 \ {\rm kHz}) = 0$  gelten muss.
• Die einzig mögliche Signalform ist somit

$$z(t) = {2 \, \rm V} \cdot H_2 (f = {20 \, \rm kHz})\cdot {\rm cos}(2\pi \cdot {20 \, \rm kHz} \cdot t ) .$$

• Möglich sind also die Lösungsvorschläge 1 und 3, je nachdem, ob das System  $S_2$  die Frequenz  $20 \ {\rm kHz}$  unterdrückt oder durchlässt.