Aufgaben:Exercise 2.2: Distortion Power

• Return to book

Input signal and output signals

A rectangular pulse $x(t)$  with amplitude $1 \hspace{0.08cm} \rm V$  and duration $4 \hspace{0.08cm} \rm ms$  is applied to the input of a communication system. Then, the pulse $y_1(t)$ , whose signal parameters can be taken from the middle sketch, is measured at the system output.

At the output of another system  $S_2$ , the signal $y_2(t)$  shown in the lower sketch is obtained with the same input signal $x(t)$ .

Let the following definition apply to the error signal used in this task:

$$\varepsilon(t) = y(t) - \alpha \cdot x(t - \tau) .$$

The parameters $\alpha$  and  $\tau$  are to be determined such that the distortion power (the mean squared error) is minimal. For this, the following holds:

$$P_{\rm V} = \overline{\varepsilon^2(t)} = \frac{1}{T_{\rm M}} \cdot \int\limits_{ ( T_{\rm M})} {\varepsilon^2(t) }\hspace{0.1cm}{\rm d}t$$

These definitions already take into account that a frequency-independent damping just as a runtime which is constant for all frequencies does not contribute to the distortion.

The integration interval has to be chosen appropriately in each case:

• Use the interval  $0$ ... $4 \hspace{0.08cm} \rm ms$  for $y_1(t)$  and the interval  $1 \hspace{0.08cm} {\rm ms}$ ... $5 \hspace{0.08cm} \rm ms$ for   $y_2(t)$ .
• Thus, the measurement time is $T_{\rm M} = 4 \hspace{0.08cm} \rm ms$ in both cases.
• It is obvious that with respect to $y_1(t)$  the parameters  $\alpha = 1$  and  $\tau = 0$  respectively result in the minimum distortion power.

In general, the so-called signal–to–distortion–power ratio is given by the following formula

$$\rho_{\rm V} = \frac{ \alpha^2 \cdot P_{x}}{P_{\rm V}} \hspace{0.05cm}.$$

Here,

• $P_x$  denotes the power of the signal $x(t)$, and
• $\alpha^2 \cdot P_x$  denotes the power of $y(t) = \alpha \cdot x(t - \tau)$, that would arise as aresult in the absence of distortion.

Usually, – as also in this task– this S/N-ratio  $\rho_{\rm V}$  is given logarithmically in  $\rm dB$ .

Please note:

• The exercise belongs to the chapter  Classification of the Distortions.
• In particular, consider the pages 

Quantitative measure for the signal distortions  and also  

Berücksichtigung von Dämpfung und Laufzeit.

Questions

1

Determine the distortion power of the system  $S_1$.

$P_{\rm V1} \ = \ $ $\ \cdot 10^{-3} \ {\rm V}^2$

2

Compute the signal–to–distortion–power ratio for system  $S_1$.

$10 \cdot {\rm lg} \ \rho_\text{V1} \ = \ $ $\ \rm dB$

3

What parameters  $\alpha$  and  $\tau$  should be used to calculate the distortion power of the system  $S_2$ ?
Justify your result.

$\alpha \ = \ $

$\tau \ = \ $ $\ \rm ms$

4

Determine the distortion power of the system  $S_2$.

$P_{\rm V2} \ = \ $ $\ \cdot 10^{-3} \ {\rm V}^2$

5

Compute the signal–to–distortion–power ratio for the system  $S_2$.
Interpret the different results.

$10 \cdot {\rm lg} \ \rho_\text{V2} \ = \ $ $\ \rm dB$

Solution

Solution

Resulting error signals

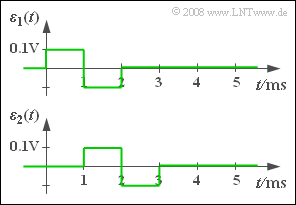
(1)  The error signal  $\varepsilon_1(t)$ shown in the graph is obtained with the given parameters $\alpha = 1$  and  $\tau= 0$ . The distortion power is thus equal to:

$$P_{\rm V1} = \frac{ {1 \, \rm ms}}{4 \, \rm ms} \cdot \big[ ({0.1 \, \rm V})^2 + ({-0.1 \, \rm V})^2\big]\hspace{0.3cm}\Rightarrow \hspace{0.3cm}P_{\rm V1} \hspace{0.15cm}\underline{ = 5 \cdot 10^{-3} \, \rm V^2}.$$

(2)  The power of the input signal is:

$$P_{x} = \frac{1}{4 \, \rm ms} \cdot ({1 \, \rm V})^2 \cdot {4 \, \rm ms}\hspace{0.15cm}{ = {1 \, \rm V^2}}.$$

• The following is obtained for the signal–to–distortion–power ratio with the result from  (1) :

$$\rho_{\rm V1} = \frac{ P_{x}}{P_{\rm V1}}= \frac{ {1 \, \rm V^2}}{0.005 \, \rm V^2}\hspace{0.05cm}\rm = 200\hspace{0.3cm} \Rightarrow \hspace{0.3cm} 10 \cdot {\rm lg}\hspace{0.1cm}\rho_{\rm V1}\hspace{0.15cm}\underline{ = {23.01 \, \rm dB}}.$$

(3)  The sketch on the information sheet makes it clear that even without the distortions occuring – but due to attenuation and runtime alone – the signal $y(t)$  would differ significantly from $x(t)$ .

• The following would arise as a result:  $y(t) = 0.5 \cdot x(t-1\ {\rm ms})$ .

• If someone does not immediately perceive these values from the graph, then first the error signal

$$\varepsilon_2(t) = y_2(t) - \alpha \cdot x(t - \tau)$$

and afterwards the mean squared error for very (infinitely) many $\alpha$–  and  $\tau$–values would have to be determined, in doing so the integration interval is to be adjusted to $\tau$  in each case.

• Then, the smallest possible result would also be obtained for $\alpha \; \underline{= 0.5}$  and  $\tau \; \underline{= 1 \ \rm ms}$ . However, for this optimization of $\alpha$  and  $\tau$  the useage of a computer program should be granted.

(4)  The above sketch shows that $\varepsilon_2(t)$  is equal to the error signal $\varepsilon_1(t)$  except for a shift by $1 \ \rm ms$ . Considering the integration interval $1 \ {\rm ms}$ ... $5 \ {\rm ms}$  the same distortion power is obtained:

$$P_{\rm V2} = P_{\rm V1} \hspace{0.15cm}\underline{ = 5 \cdot 10^{-3} \, \rm V^2}.$$

(5)  According to the information sheet the following holds:

$$\rho_{\rm V2} = \frac{ \alpha^2 \cdot P_{x}}{P_{\rm V2}}= \frac{ 0.5^2 \cdot {1 \, \rm V^2}}{0.005 \, \rm V^2}\hspace{0.05cm}\rm = 50\hspace{0.3cm} \Rightarrow \hspace{0.3cm} 10 \cdot {\rm lg}\hspace{0.1cm}\rho_{\rm V2} \hspace{0.15cm}\underline{= {16.99 \, \rm dB}}.$$

• Despite the same distortion power $10 \cdot {\rm lg}\hspace{0.1cm}\rho_{\rm V2}$  is less than  $10 \cdot {\rm lg}\hspace{0.1cm}\rho_{\rm V1}$  by about $6 \ \rm dB$ .
• The signal $y_2(t)$  is thus significantly less favourable in terms of SNR than $y_1(t)$.
• It is considered that now the power of the output signal is only a quarter of the input power due to  $\alpha = 0.5$ .
• If this attenuation at the output was to be compensated by amplifying it by $1/\alpha$, the distortion power would indeed increase by $\alpha^2$.

• The signal-to-distortion-power ratio $\rho_{\rm V2}$ would, however, remain the same because the "useful signal" would also be increased by the same value.