Aufgaben:Exercise 3.2Z: Laplace and Fourier

• Return to book

Four causal time signals

The Fourier transformation can be applied to any deterministic signal  $x(t)$ . Then, the following holds for the spectral function:

$$X(f) = \int_{-\infty}^{ +\infty} { x(t) \hspace{0.05cm}\cdot \hspace{0.05cm} {\rm e}^{-{\rm j}\hspace{0.05cm}\cdot \hspace{0.05cm}2\pi f t}}\hspace{0.1cm}{\rm d}t\hspace{0.05cm}\hspace{0.05cm} .$$

For power-limited signals – characteristics:   infinite energy – $X(f)$  also includes distributions (Dirac functions).

For all causal signals (and only for these), the Laplace transformation is applicable:

$$X_{\rm L}(p) = \int_{0}^{ \infty} { x(t) \hspace{0.05cm}\cdot \hspace{0.05cm} {\rm e}^{-p t}}\hspace{0.1cm}{\rm d}t\hspace{0.05cm}\hspace{0.05cm} .$$

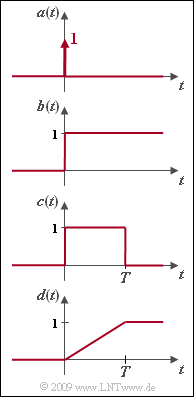
In the diagram you can see several causal time functions that will be covered in this exercise:

• the Dirac function  $a(t)$,

• the step function  $b(t)$,

• the rectangular function  $c(t)$,

• the ramp function  $d(t)$.

The  Fourier transform theorems  gelten meist (allerdings nicht immer) auch für die Laplace–Transformation, wobei  $p ={\rm j} \cdot 2 \pi f$  zu setzen ist:

• Zum Beispiel lautet der  shifting theorem  in Laplace– bzw. Fourier–Darstellung:

$$x(t- \tau) \quad \circ\!\!-\!\!\!-^{\hspace{-0.25cm}\rm L}\!\!\!-\!\!\hspace{-0.05cm}\bullet\quad X_{\rm L}(p)\cdot {\rm e}^{-p \hspace{0.05cm} \cdot \hspace{0.05cm}\tau}\hspace{0.05cm} ,$$

$$x(t- \tau) \quad \circ\!\!-\!\!\!-^{\hspace{-0.05cm}}\!\!\!-\!\!\hspace{-0.05cm}\bullet\quad X(f)\cdot {\rm e}^{-{\rm j}\hspace{0.05cm} \cdot \hspace{0.05cm}2\pi f \hspace{0.05cm} \cdot \hspace{0.05cm}\tau}\hspace{0.05cm} .$$

• Dagegen ergeben sich beim  integration theorem  Unterschiede:

$$\int {x(\tau)} \hspace{0.1cm}{\rm d}\tau \quad \circ\!\!-\!\!\!-^{\hspace{-0.25cm}\rm L}\!\!\!-\!\!\hspace{-0.05cm}\bullet\quad X_{\rm L}(p)\cdot \frac{1}{p}\hspace{0.05cm} ,$$

$$\int {x(\tau)} \hspace{0.1cm}{\rm d}\tau \quad \circ\!\!-\!\!\!-^{\hspace{-0.05cm}}\!\!\!-\!\!\hspace{-0.05cm}\bullet\quad X(f)\cdot \left [ {1}/{2} \cdot{\rm \delta } (f) + \frac{1}{{\rm j} \cdot 2\pi f} \right ] \hspace{0.05cm} .$$

Please note:

• The exercise belongs to the chapter  Laplace Transform and p-Transfer Function.
• Das Lernvideo   Fourier transform theorems  könnte hilfreich sein .

Questions

1

Wie lauten die Spektraltransformationen des Signals  $a(t) = \delta(t)$?

	$A_{\rm L}(p) = 1$.
	$A(f) = \delta(f)$.
	$A(f) = 1$.

2

Wie lauten die Spektraltransformationen der Sprungfunktion  $b(t) = \gamma(t)$?

	$B_{\rm L}(p) = 1/p$.
	$B(f) = 1/({\rm j} \cdot 2 \pi f)$
	$B(f) = 1/2 \cdot \delta(f) - {\rm j}/(2 \pi f)$.

3

Wie lauten die Spektraltransformationen der Rechteckfunktion  $c(t)$?

	$C_{\rm L}(p) = {\rm si}(pT)$.
	$C_{\rm L}(p) = \big [1-{\rm e}^{-p\hspace{0.05cm} \cdot \hspace{0.05cm}T} \big ]/p$.
	$C(f) = C_{\rm L}(p)$  mit  $p = 2 \pi f$.

4

Wie lauten die Spektraltransformationen der Rampenfunktion  $d(t)$?

	$D_{\rm L}(p) = \big[1-{\rm e}^{-p\hspace{0.05cm} \cdot \hspace{0.05cm}T}\big]/(p^2T)$.
	$D_{\rm L}(p) = 1-{\rm e}^{-p\hspace{0.05cm} \cdot \hspace{0.05cm}T}$.
	$D(f) = D_{\rm L}(p)$  mit  $p = 2 \pi f$.

Solution

Solution

(1)  Richtig sind die Lösungsvorschläge 1 und 3:

• Berücksichtigt man, dass die Diracfunktion nur bei  $t= 0$  ungleich Null ist und das Integral über den Dirac den Wert  $1$  liefert, solange das Integrationsintervall den Zeitpunkt  $t= 0$  einschließt, so erhält man:

$$A(f) = 1, \hspace{0.2cm}A_{\rm L}(p) = 1 \hspace{0.05cm} .$$

(2)  Richtig sind wieder die Lösungsvorschläge 1 und 3:

• Die Sprungfunktion  $b(t) = \gamma(t)$  ist das Integral über die Diracfunktion  $a(t) = \delta(t)$   ⇒   man kann den  integration theorem  anwenden:

$$b(t) = \int_{-\infty}^t {a(\tau)} \hspace{0.1cm}{\rm d}\tau \hspace{0.3cm}\Rightarrow \hspace{0.3cm} B_{\rm L}(p) =A_{\rm L}(p)\cdot {1}/{p} = {1}/{p}\hspace{0.05cm} ,$$

$$B(f) = A(f)\cdot \left [ {1}/{2} \cdot{\rm \delta } (f) + \frac{1}{{\rm j} \cdot 2\pi f} \right ] = {1}/{2} \cdot{\rm \delta } (f) + \frac{1}{{\rm j} \cdot 2\pi f}\hspace{0.05cm} .$$

(3)  Richtig sind die Lösungsvorschläge 2 und 3:

• Nachdem die (kausale) Rechteckfunktion als Differenz zweier Sprungfunktionen dargestellt werden kann, erhält man mit dem  shifting theorem:

$$c(t)= b(t) - b(t-T) \hspace{0.3cm} \Rightarrow \hspace{0.3cm} C_{\rm L}(p) =B_{\rm L}(p)- B_{\rm L}(p) \cdot {\rm e}^{-p \hspace{0.05cm} \cdot \hspace{0.05cm}T} = {1}/{p} \cdot \big [ 1- {\rm e}^{-p \hspace{0.05cm} \cdot \hspace{0.05cm}T} \big ] \hspace{0.05cm} .$$

• Da die Rechteckfunktion eine endliche Energie besitzt, gilt für das  Fourier spectrum:

$$C(f) = C_{\rm L}(p)\Bigg |_{\hspace{0.1cm} p\hspace{0.05cm}=\hspace{0.05cm}{\rm j \hspace{0.05cm}2\pi \it f}} = \frac{1}{{\rm j} \cdot 2\pi f} \cdot \big [ 1- {\rm e}^{-{\rm j} \hspace{0.05cm}\cdot \hspace{0.05cm} 2\pi f T} \big ] \hspace{0.05cm}.$$

• Nach einigen trigonometrischen Umformungen kann hierfür auch geschrieben werden:

$$C(f) = T \cdot {\rm si} (2 \pi f{T})+ {\rm j} \cdot \frac{{\rm cos} (2 \pi f{T})-1}{2\pi f} \hspace{0.05cm}.$$

(4)  Richtig ist der Lösungsvorschlag 1, da gilt:

$$d(t) = \frac{1}{T} \cdot \int\limits_{-\infty}^t {c(\tau)} \hspace{0.1cm}{\rm d}\tau \hspace{0.3cm}\Rightarrow \hspace{0.3cm} D_{\rm L}(p) =C_{\rm L}(p)\cdot \frac{1}{p \cdot T} = \frac{1- {\rm e}^{-p \hspace{0.05cm}\cdot \hspace{0.05cm}T}}{p^2 \cdot T}\hspace{0.05cm} .$$

• Da sich  $d(t)$  bis ins Unendliche erstreckt, ist der einfache Zusammenhang zwischen  $D_{\rm L}(p)$  und  $D(f)$  gemäß Lösungsvorschlag 3 nicht gegeben.
• $D(f)$  beinhaltet vielmehr auch eine Diracfunktion bei der Frequenz  $f = 0$.