Difference between revisions of "Aufgaben:Exercise 4.6: k-parameters and alpha-parameters"
| (20 intermediate revisions by 4 users not shown) | |||
| Line 1: | Line 1: | ||
| − | {{quiz-Header|Buchseite= | + | {{quiz-Header|Buchseite=Linear_and_Time_Invariant_Systems/Properties_of_Balanced_Copper_Pairs |
}} | }} | ||
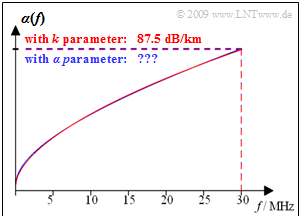
| − | [[File: | + | [[File:EN_LZI_A_4_6.png|right|frame|Attenuation function per unit length, <br>valid for "copper twin wire" (0.5 mm)]] |
| − | + | For symmetrical copper twisted pairs, the following empirical formula can be found in [PW95], which is valid for the frequency range 0≤f≤30 MHz: | |
:$$\alpha_{\rm I} (f) = k_1 + k_2 \cdot (f/f_0)^{k_3} , \hspace{0.15cm} | :$$\alpha_{\rm I} (f) = k_1 + k_2 \cdot (f/f_0)^{k_3} , \hspace{0.15cm} | ||
f_0 = 1\,{\rm MHz} .$$ | f_0 = 1\,{\rm MHz} .$$ | ||
| − | + | In contrast, the attenuation function per unit length of a coaxial cable is usually given in the following form: | |
:αII(f)=α0+α1⋅f+α2⋅√f. | :αII(f)=α0+α1⋅f+α2⋅√f. | ||
| − | + | Especially for the calculation of impulse response and rectangular response it is advantageous also for the copper twisted pairs to choose the second representation form with the cable parameters α0, α1 and α2 instead of the representation with k1, k2 and k3. | |
| − | + | For the conversion, one proceeds as follows: | |
| − | * | + | * From above equations, it is obvious that the coefficient characterizing the DC signal attenuation is α0=k1. |
| − | * | + | * To determine α1 and α2, it is assumed that the mean square error should be minimum in the range of a given bandwidth B: |
| − | :$${\rm E}[\varepsilon^2(f)] = \int_{0}^{ | + | :$${\rm E}\big[\varepsilon^2(f)\big] = \int_{0}^{ |
B} \left [ \alpha_{\rm II} (f) - \alpha_{\rm I} (f)\right ]^2 | B} \left [ \alpha_{\rm II} (f) - \alpha_{\rm I} (f)\right ]^2 | ||
\hspace{0.1cm}{\rm d}f \hspace{0.3cm}\Rightarrow | \hspace{0.1cm}{\rm d}f \hspace{0.3cm}\Rightarrow | ||
\hspace{0.3cm}{\rm Minimum} | \hspace{0.3cm}{\rm Minimum} | ||
\hspace{0.05cm} .$$ | \hspace{0.05cm} .$$ | ||
| − | * | + | * The difference ε2(f) and the mean square error ${\rm E}\big[\varepsilon^2(f)\big]$ are obtained as follows: |
| − | :$$\varepsilon^2(f) = \ | + | :$$\varepsilon^2(f) = \big [ \alpha_1 \cdot f + \alpha_2 \cdot \sqrt {f} - k_2 \cdot (f/f_0)^{k_3}\big ]^2 |
=\alpha_1^2 \hspace{0.05cm}\cdot\hspace{0.05cm} f^2 + 2 \alpha_1 \alpha_2 \hspace{0.05cm}\cdot\hspace{0.05cm} f^{1.5} + | =\alpha_1^2 \hspace{0.05cm}\cdot\hspace{0.05cm} f^2 + 2 \alpha_1 \alpha_2 \hspace{0.05cm}\cdot\hspace{0.05cm} f^{1.5} + | ||
\alpha_1^2 \hspace{0.05cm}\cdot\hspace{0.05cm} f + k_2^2\hspace{0.05cm}\cdot\hspace{0.05cm} \frac{f^{2k_3}}{f_0^{2k_3}} - 2 k_2 \alpha_1 \hspace{0.05cm}\cdot\hspace{0.05cm} | \alpha_1^2 \hspace{0.05cm}\cdot\hspace{0.05cm} f + k_2^2\hspace{0.05cm}\cdot\hspace{0.05cm} \frac{f^{2k_3}}{f_0^{2k_3}} - 2 k_2 \alpha_1 \hspace{0.05cm}\cdot\hspace{0.05cm} | ||
\frac{f^{k_3+1}} {f_0^{k_3}}-{2 k_2 \alpha_2} \hspace{0.05cm}\cdot\hspace{0.05cm} \frac{f^{k_3+0.5}}{f_0^{k_3}}$$ | \frac{f^{k_3+1}} {f_0^{k_3}}-{2 k_2 \alpha_2} \hspace{0.05cm}\cdot\hspace{0.05cm} \frac{f^{k_3+0.5}}{f_0^{k_3}}$$ | ||
:$$\Rightarrow | :$$\Rightarrow | ||
| − | \hspace{0.3cm}{\rm E}[\varepsilon^2(f)] = \alpha_1^2 | + | \hspace{0.3cm}{\rm E}\big[\varepsilon^2(f)\big] = \alpha_1^2 |
\hspace{0.05cm}\cdot\hspace{0.05cm}\frac{B^3}{3} + \frac{4}{5} \hspace{0.05cm}\cdot\hspace{0.05cm}\alpha_1 \alpha_2 \hspace{0.05cm}\cdot\hspace{0.05cm}B^{2.5} + | \hspace{0.05cm}\cdot\hspace{0.05cm}\frac{B^3}{3} + \frac{4}{5} \hspace{0.05cm}\cdot\hspace{0.05cm}\alpha_1 \alpha_2 \hspace{0.05cm}\cdot\hspace{0.05cm}B^{2.5} + | ||
\alpha_1^2 \hspace{0.05cm}\cdot\hspace{0.05cm} \frac{B^2}{2} + \frac{k_2^2}{2k_3 +1} \hspace{0.05cm}\cdot\hspace{0.05cm} | \alpha_1^2 \hspace{0.05cm}\cdot\hspace{0.05cm} \frac{B^2}{2} + \frac{k_2^2}{2k_3 +1} \hspace{0.05cm}\cdot\hspace{0.05cm} | ||
| Line 32: | Line 32: | ||
\hspace{0.05cm}\cdot\hspace{0.05cm} | \hspace{0.05cm}\cdot\hspace{0.05cm} | ||
$$ | $$ | ||
| − | : | + | :This equation contains the cable parameters α1, α2, k2 and k3 to be calculated as well as the bandwidth B, within which the approximation should be valid. |
| − | * | + | * By setting the derivatives of ${\rm E}\big[\varepsilon^2(f)\big]$ to α1 and α2 to zero, two equations are obtained for the best possible coefficients α1 and α2 that minimize the mean square error. These can be represented in the following form: |
| − | :$$\frac{{\rm d}\,{\rm E}[\varepsilon^2(f)]}{{\rm d}\,{\alpha_1}} = 0 \hspace{0.2cm} | + | :$$\frac{{\rm d}\,{\rm E}\big[\varepsilon^2(f)\big]}{{\rm d}\,{\alpha_1}} = 0 \hspace{0.2cm} |
\Rightarrow | \Rightarrow | ||
\hspace{0.2cm} \alpha_1 + C_1 \cdot \alpha_2 + C_2 = 0 \hspace{0.05cm} | \hspace{0.2cm} \alpha_1 + C_1 \cdot \alpha_2 + C_2 = 0 \hspace{0.05cm} | ||
,$$ | ,$$ | ||
| − | :$$\frac{{\rm d}\,{\rm E}[\varepsilon^2(f)]}{{\rm d}\,{\alpha_2}} = | + | :$$\frac{{\rm d}\,{\rm E}\big[\varepsilon^2(f)\big]}{{\rm d}\,{\alpha_2}} = |
0 \hspace{0.2cm} | 0 \hspace{0.2cm} | ||
\Rightarrow | \Rightarrow | ||
\hspace{0.2cm} \alpha_1 + D_1 \cdot \alpha_2 + D_2 = 0 \hspace{0.05cm} | \hspace{0.2cm} \alpha_1 + D_1 \cdot \alpha_2 + D_2 = 0 \hspace{0.05cm} | ||
. $$ | . $$ | ||
| − | * | + | * From the equation C1⋅α2+C2=D1⋅α2+D2, the coefficient α2 can be calculated and then the coefficient α1 can be calculated from each of the two equations above. |
| − | + | The graph shows the attenuation function per unit length for a copper twin wire with $\text{0.5 mm}$ diameter, whose k–parameters are: | |
:$$k_1 = 4.4\, {\rm dB}/{\rm km} \hspace{0.05cm}, \hspace{0.2cm} | :$$k_1 = 4.4\, {\rm dB}/{\rm km} \hspace{0.05cm}, \hspace{0.2cm} | ||
k_2 = 10.8\, {\rm dB}/{\rm km}\hspace{0.05cm}, \hspace{0.2cm}k_3 = 0.60\hspace{0.05cm} | k_2 = 10.8\, {\rm dB}/{\rm km}\hspace{0.05cm}, \hspace{0.2cm}k_3 = 0.60\hspace{0.05cm} | ||
\hspace{0.05cm}.$$ | \hspace{0.05cm}.$$ | ||
| − | * | + | *The red curve shows the function α(f) calculated with this parameters. For f=30 MHz the attenuation function per unit length is $\alpha(f)= 87.5 \ \rm dB/km$. |
| − | * | + | *The blue curve gives the approximation with the α–coefficients. This is almost indistinguishable from the red curve within the drawing accuracy. |
| Line 58: | Line 58: | ||
| − | + | Notes: | |
| − | * | + | *The exercise belongs to the chapter [[Linear_and_Time_Invariant_Systems/Eigenschaften_von_Kupfer–Doppeladern|Properties of Balanced Copper Pairs]]. |
| − | * | + | |
| − | + | *You can use the (German language) interactive SWF applet [[Applets:Dämpfung_von_Kupferkabeln|"Dämpfung von Kupferkabeln"]] ⇒ "Attenuation of copper cables" . | |
| − | *[PW95] | + | *[PW95] denotes the following literature reference: Pollakowski, P.; Wellhausen, H.-W.: Eigenschaften symmetrischer Ortsanschlusskabel im Frequenzbereich bis 30 MHz. Deutsche Telekom AG, Forschungs- und Technologiezentrum Darmstadt, 1995. |
| − | :Pollakowski, P.; Wellhausen, H.-W.: | ||
| − | === | + | ===Questions=== |
<quiz display=simple> | <quiz display=simple> | ||
| − | { | + | {Calculate the parameters C1 and C2 of the equation α1+C1⋅α2+C2=0 resulting from the derivative ${\rm dE\big[\text{...}\big]/d}\alpha_1$. <br>Which results are correct? |
|type="[]"} | |type="[]"} | ||
+ C1=6/5⋅B−0.5, | + C1=6/5⋅B−0.5, | ||
| Line 79: | Line 78: | ||
| − | { | + | {Calculate the parameters D1 and D2 of the equation α1+D1⋅α2+D2=0 resulting from the derivative ${\rm dE\big[\text{...}\big]/d}\alpha_2$. <br> Which results are correct? |
|type="[]"} | |type="[]"} | ||
- D1=6/5⋅B−0.5, | - D1=6/5⋅B−0.5, | ||
| Line 89: | Line 88: | ||
| − | { | + | {Calculate the coefficients α1 and α2 for the given k2 and k3. <br>Which of the following statements are true? |
|type="[]"} | |type="[]"} | ||
| − | + | + | + For k3=1.0, α1=k2/f0 and α2=0. |
| − | + | + | + For k3=0.5, α1=0 and α2=k2/f0.50. |
| − | { | + | {Determine the coefficients α1 and α2 numerically for the approximation bandwidth B=30 MHz. |
|type="{}"} | |type="{}"} | ||
α1 = { 0.761 3% } dB/(km ⋅ MHz) | α1 = { 0.761 3% } dB/(km ⋅ MHz) | ||
| Line 101: | Line 100: | ||
| − | { | + | {Using the α–parameters, calculate the attenuation function per unit length for the frequency f=30 MHz. |
|type="{}"} | |type="{}"} | ||
αII(f=30 MHz) = { 88.1 3% } dB/km | αII(f=30 MHz) = { 88.1 3% } dB/km | ||
| Line 109: | Line 108: | ||
</quiz> | </quiz> | ||
| − | === | + | ===Solution=== |
{{ML-Kopf}} | {{ML-Kopf}} | ||
| − | '''(1)''' | + | '''(1)''' <u>Solutions 1 and 6</u> are correct: |
| − | $$\frac{{\rm d}\,{\rm E}[\varepsilon^2(f)]}{{\rm d}\,{\alpha_1}} = | + | *The derivative of the given expected value with respect to α1 gives: |
| + | :$$\frac{{\rm d}\,{\rm E}[\varepsilon^2(f)]}{{\rm d}\,{\alpha_1}} = | ||
\frac{2}{3}\cdot B^3 \cdot \alpha_1 + \frac{4}{5}\cdot B^{2.5} \cdot \alpha_2 | \frac{2}{3}\cdot B^3 \cdot \alpha_1 + \frac{4}{5}\cdot B^{2.5} \cdot \alpha_2 | ||
- \frac{2 k_2 }{k_3 | - \frac{2 k_2 }{k_3 | ||
+ 2} \cdot \frac{B^{k_3+2}}{f_0^{k_3}}= 0 | + 2} \cdot \frac{B^{k_3+2}}{f_0^{k_3}}= 0 | ||
\hspace{0.05cm} .$$ | \hspace{0.05cm} .$$ | ||
| − | + | *By setting it to zero and dividing by 2B2/3, we obtain: | |
| − | $$\alpha_1 + \frac{6}{5}\cdot B^{-0.5} \cdot \alpha_2 | + | :$$\alpha_1 + \frac{6}{5}\cdot B^{-0.5} \cdot \alpha_2 |
- \frac{3 k_2 }{k_3 | - \frac{3 k_2 }{k_3 | ||
+2} \cdot \frac{B^{k_3-1}}{f_0^{k_3}}= 0 | +2} \cdot \frac{B^{k_3-1}}{f_0^{k_3}}= 0 | ||
| − | + | \hspace{0.3cm} | |
| − | + | \Rightarrow \hspace{0.3cm} C_1 = \frac{6}{5}\cdot B^{-0.5} \hspace{0.05cm} , | |
\hspace{0.5cm} C_2 = | \hspace{0.5cm} C_2 = | ||
- \frac{3 k_2 }{k_3 | - \frac{3 k_2 }{k_3 | ||
+2} \cdot \frac{B^{k_3-1}}{f_0^{k_3}} | +2} \cdot \frac{B^{k_3-1}}{f_0^{k_3}} | ||
\hspace{0.05cm} .$$ | \hspace{0.05cm} .$$ | ||
| − | |||
| − | '''(2)''' | + | |
| − | $$\frac{{\rm d}\,{\rm E}[\varepsilon^2(f)]}{{\rm d}\,{\alpha_2}} = | + | '''(2)''' <u>Solutions 2 and 5</u> are correct: |
| + | *Using the same procedure as in subtask '''(1)''', we obtain: | ||
| + | :$$\frac{{\rm d}\,{\rm E}[\varepsilon^2(f)]}{{\rm d}\,{\alpha_2}} = | ||
\frac{4}{5}\cdot B^{2.5} \cdot \alpha_1 + B^{2} \cdot \alpha_2 | \frac{4}{5}\cdot B^{2.5} \cdot \alpha_1 + B^{2} \cdot \alpha_2 | ||
- \frac{2 k_2 }{k_3 | - \frac{2 k_2 }{k_3 | ||
+ 1.5} \cdot \frac{B^{k_3+1.5}}{f_0^{k_3}}= 0$$ | + 1.5} \cdot \frac{B^{k_3+1.5}}{f_0^{k_3}}= 0$$ | ||
| − | $$\Rightarrow \hspace{0.3cm} \alpha_1 + \frac{5}{4}\cdot B^{-0.5} \cdot \alpha_2 | + | :$$\Rightarrow \hspace{0.3cm} \alpha_1 + \frac{5}{4}\cdot B^{-0.5} \cdot \alpha_2 |
- \frac{2.5 \cdot k_2 }{k_3 | - \frac{2.5 \cdot k_2 }{k_3 | ||
+1.5} \cdot \frac{B^{k_3-1}}{f_0^{k_3}}= 0 | +1.5} \cdot \frac{B^{k_3-1}}{f_0^{k_3}}= 0 | ||
| − | + | \hspace{0.3cm} | |
| − | + | \Rightarrow \hspace{0.3cm}D_1 = \frac{5}{4}\cdot B^{-0.5} \hspace{0.05cm} , | |
\hspace{0.3cm}D_2 = | \hspace{0.3cm}D_2 = | ||
- \frac{2.5 \cdot k_2 }{k_3 | - \frac{2.5 \cdot k_2 }{k_3 | ||
| Line 146: | Line 147: | ||
| − | '''(3)''' | + | |
| − | $$\alpha_2 = \frac{D_2 - C_2}{C_1 - D_1} = \frac{- \frac{2.5 \cdot k_2 }{k_3 | + | '''(3)''' <u>Both solutions</u> are correct: |
| + | |||
| + | *From C1⋅α2+C2=D1⋅α2+D2 we obtain a linear equation for α2. With the result from '''(2)''' we can write: | ||
| + | :$$\alpha_2 = \frac{D_2 - C_2}{C_1 - D_1} = \frac{- \frac{2.5 \cdot k_2 }{k_3 | ||
+1.5} \cdot \frac{B^{k_3-1}}{f_0^{k_3}} + \frac{3 k_2 }{k_3 +2} | +1.5} \cdot \frac{B^{k_3-1}}{f_0^{k_3}} + \frac{3 k_2 }{k_3 +2} | ||
\cdot \frac{B^{k_3-1}}{f_0^{k_3}}}{{6}/{5}\cdot B^{-0.5} - | \cdot \frac{B^{k_3-1}}{f_0^{k_3}}}{{6}/{5}\cdot B^{-0.5} - | ||
| Line 154: | Line 158: | ||
{5}/{4})(k_3 +1.5)(k_3 +2)} \cdot | {5}/{4})(k_3 +1.5)(k_3 +2)} \cdot | ||
\frac{B^{k_3-0.5}}{f_0^{k_3}}$$ | \frac{B^{k_3-0.5}}{f_0^{k_3}}$$ | ||
| − | $$ \Rightarrow \hspace{0.3cm}\alpha_2 = 10 \cdot (B/f_0)^{k_3 | + | :$$ \Rightarrow \hspace{0.3cm}\alpha_2 = 10 \cdot (B/f_0)^{k_3 |
-0.5}\cdot \frac{1-k_3}{(k_3 + 1.5)(k_3 + | -0.5}\cdot \frac{1-k_3}{(k_3 + 1.5)(k_3 + | ||
2)}\cdot \frac {k_2}{\sqrt{f_0}} | 2)}\cdot \frac {k_2}{\sqrt{f_0}} | ||
\hspace{0.05cm} .$$ | \hspace{0.05cm} .$$ | ||
| − | + | *For the parameter α1 then holds: | |
| − | $$\alpha_1 = - C_1 \cdot \alpha_2 - C_2 = | + | :$$\alpha_1 = - C_1 \cdot \alpha_2 - C_2 = |
-\frac{6}{5}\cdot B^{-0.5} \cdot 10 \cdot (B/f_0)^{k_3 | -\frac{6}{5}\cdot B^{-0.5} \cdot 10 \cdot (B/f_0)^{k_3 | ||
-0.5}\cdot \frac{1-k_3}{(k_3 + 1.5)(k_3 + | -0.5}\cdot \frac{1-k_3}{(k_3 + 1.5)(k_3 + | ||
2)}\cdot \frac {k_2}{\sqrt{f_0}} +\frac{3 k_2 }{k_3 +2} | 2)}\cdot \frac {k_2}{\sqrt{f_0}} +\frac{3 k_2 }{k_3 +2} | ||
| − | \cdot \frac{B^{k_3-1}}{f_0^{k_3}}= | + | \cdot \frac{B^{k_3-1}}{f_0^{k_3}}$$ |
| + | :$$ \Rightarrow \hspace{0.3cm}\alpha_1 = (B/f_0)^{k_3 -1}\cdot | ||
\frac{-12 \cdot (1-k_3) + 3 \cdot (k_3 + 1.5)}{(k_3 + 1.5)(k_3 + | \frac{-12 \cdot (1-k_3) + 3 \cdot (k_3 + 1.5)}{(k_3 + 1.5)(k_3 + | ||
| − | 2)} \cdot \frac {k_2}{f_0} | + | 2)} \cdot \frac {k_2}{f_0} \hspace{0.3cm} \Rightarrow \hspace{0.3cm}\alpha_1 =15 \cdot (B/f_0)^{k_3 |
| − | |||
-1}\cdot \frac{k_3 -0.5}{(k_3 + 1.5)(k_3 + | -1}\cdot \frac{k_3 -0.5}{(k_3 + 1.5)(k_3 + | ||
2)}\cdot \frac {k_2}{f_0}\hspace{0.05cm} .$$ | 2)}\cdot \frac {k_2}{f_0}\hspace{0.05cm} .$$ | ||
| − | + | ||
| − | $$\alpha_1 = (B/f_0)^{k_3 | + | *Regardless of the bandwidth, we obtain for k3=1: |
| + | :$$\alpha_1 = (B/f_0)^{k_3 | ||
-1}\cdot \frac{15 \cdot (k_3 -0.5)}{(k_3 + 1.5)(k_3 + | -1}\cdot \frac{15 \cdot (k_3 -0.5)}{(k_3 + 1.5)(k_3 + | ||
2)}\cdot \frac {k_2}{f_0} = \frac{15 \cdot 0.5}{2.5 \cdot 3}\cdot \frac {k_2}{f_0} | 2)}\cdot \frac {k_2}{f_0} = \frac{15 \cdot 0.5}{2.5 \cdot 3}\cdot \frac {k_2}{f_0} | ||
\hspace{0.15cm}\underline{ = {k_2}/{f_0}}\hspace{0.05cm} | \hspace{0.15cm}\underline{ = {k_2}/{f_0}}\hspace{0.05cm} | ||
,$$ | ,$$ | ||
| − | $$ \alpha_2 = (B/f_0)^{k_3 | + | :$$ \alpha_2 = (B/f_0)^{k_3 |
-0.5}\cdot \frac{10 \cdot (1-k_3)}{(k_3 + 1.5)(k_3 + | -0.5}\cdot \frac{10 \cdot (1-k_3)}{(k_3 + 1.5)(k_3 + | ||
2)}\cdot \frac {k_2}{\sqrt{f_0}}\hspace{0.15cm}\underline{= 0} \hspace{0.05cm} | 2)}\cdot \frac {k_2}{\sqrt{f_0}}\hspace{0.15cm}\underline{= 0} \hspace{0.05cm} | ||
.$$ | .$$ | ||
| − | + | *In contrast, for k3=0.5: | |
| − | $$\alpha_1 = (B/f_0)^{k_3 | + | :$$\alpha_1 = (B/f_0)^{k_3 |
-1}\cdot \frac{15 \cdot (k_3 -0.5)}{(k_3 + 1.5)(k_3 + | -1}\cdot \frac{15 \cdot (k_3 -0.5)}{(k_3 + 1.5)(k_3 + | ||
2)}\cdot \frac {k_2}{f_0} \hspace{0.15cm}\underline{= 0}\hspace{0.05cm} | 2)}\cdot \frac {k_2}{f_0} \hspace{0.15cm}\underline{= 0}\hspace{0.05cm} | ||
,$$ | ,$$ | ||
| − | $$ \alpha_2 = (B/f_0)^{k_3 | + | :$$ \alpha_2 = (B/f_0)^{k_3 |
-0.5}\cdot \frac{10 \cdot (1-k_3)}{(k_3 + 1.5)(k_3 + | -0.5}\cdot \frac{10 \cdot (1-k_3)}{(k_3 + 1.5)(k_3 + | ||
2)}\cdot \frac {k_2}{\sqrt{f_0}}= \frac{10 \cdot 0.5}{2 \cdot 2.5}\cdot \frac {k_2}{\sqrt{f_0}} = \hspace{0.15cm}\underline{ {k_2}/{\sqrt{f_0}}} \hspace{0.05cm} | 2)}\cdot \frac {k_2}{\sqrt{f_0}}= \frac{10 \cdot 0.5}{2 \cdot 2.5}\cdot \frac {k_2}{\sqrt{f_0}} = \hspace{0.15cm}\underline{ {k_2}/{\sqrt{f_0}}} \hspace{0.05cm} | ||
| Line 190: | Line 195: | ||
| − | '''(4)''' | + | |
| − | $$\alpha_1 = (B/f_0)^{k_3 | + | '''(4)''' For the two coefficients, with k2=10.8 dB/km, k3=0.6 dB/km and B/f0=30: |
| + | :$$\alpha_1 = (B/f_0)^{k_3 | ||
-1}\cdot \frac{15 \cdot (k_3 -0.5)}{(k_3 + 1.5)(k_3 + | -1}\cdot \frac{15 \cdot (k_3 -0.5)}{(k_3 + 1.5)(k_3 + | ||
2)}\cdot \frac {k_2}{f_0} = 30^{-0.4}\cdot \frac{15 \cdot 0.1}{2.1 \cdot 2.6}\cdot | 2)}\cdot \frac {k_2}{f_0} = 30^{-0.4}\cdot \frac{15 \cdot 0.1}{2.1 \cdot 2.6}\cdot | ||
| Line 199: | Line 205: | ||
\hspace{0.05cm} | \hspace{0.05cm} | ||
,$$ | ,$$ | ||
| − | $$ \alpha_2 = (B/f_0)^{k_3 | + | :$$ \alpha_2 = (B/f_0)^{k_3 |
-0.5}\cdot \frac{10 \cdot (1-k_3)}{(k_3 + 1.5)(k_3 + | -0.5}\cdot \frac{10 \cdot (1-k_3)}{(k_3 + 1.5)(k_3 + | ||
| − | 2)}\cdot \frac {k_2}{\sqrt{f_0}}= | + | 2)}\cdot \frac {k_2}{\sqrt{f_0}}= \frac {k_2}{\sqrt{f_0}} |
= 30^{0.1}\cdot \frac{10 \cdot 0.4}{2.1 \cdot 2.6}\cdot \frac | = 30^{0.1}\cdot \frac{10 \cdot 0.4}{2.1 \cdot 2.6}\cdot \frac | ||
{10.8 \, {\rm dB/km} }{1 \, {\rm MHz^{0.5}}} | {10.8 \, {\rm dB/km} }{1 \, {\rm MHz^{0.5}}} | ||
\hspace{0.15cm}\underline{ \approx 11.1\, | \hspace{0.15cm}\underline{ \approx 11.1\, | ||
| − | {{\rm dB} }/{({\rm km \cdot MHz | + | {{\rm dB} }/{({\rm km \cdot \sqrt{MHz}}})}\hspace{0.05cm} |
.$$ | .$$ | ||
| − | '''(5)''' | + | |
| − | $$\alpha_{\rm II}(f = 30 \, {\rm MHz}) = \alpha_0 + \alpha_1 \cdot f + \alpha_2 \cdot \sqrt {f} | + | '''(5)''' According to the given equation $\alpha_{\rm II}(f)$ thus also holds: |
| − | = \ | + | :$$\alpha_{\rm II}(f = 30 \, {\rm MHz}) = \alpha_0 + \alpha_1 \cdot f + \alpha_2 \cdot \sqrt {f} |
| − | \ | + | = \big [ \hspace{0.05cm} 4.4 + 0.761 \cdot 30 + 11.1 \cdot \sqrt {30}\hspace{0.05cm} |
| + | \big ]\frac | ||
{\rm dB}{\rm km } | {\rm dB}{\rm km } | ||
\hspace{0.15cm}\underline{\approx 88.1\, {\rm dB}/{\rm km }} | \hspace{0.15cm}\underline{\approx 88.1\, {\rm dB}/{\rm km }} | ||
| Line 220: | Line 227: | ||
| − | [[Category: | + | [[Category:Linear and Time-Invariant Systems: Exercises|^4.3 Balanced Copper Twisted Pair^]] |
Latest revision as of 18:11, 23 November 2021
For symmetrical copper twisted pairs, the following empirical formula can be found in [PW95], which is valid for the frequency range 0≤f≤30 MHz:
- αI(f)=k1+k2⋅(f/f0)k3,f0=1MHz.
In contrast, the attenuation function per unit length of a coaxial cable is usually given in the following form:
- αII(f)=α0+α1⋅f+α2⋅√f.
Especially for the calculation of impulse response and rectangular response it is advantageous also for the copper twisted pairs to choose the second representation form with the cable parameters α0, α1 and α2 instead of the representation with k1, k2 and k3.
For the conversion, one proceeds as follows:
- From above equations, it is obvious that the coefficient characterizing the DC signal attenuation is α0=k1.
- To determine α1 and α2, it is assumed that the mean square error should be minimum in the range of a given bandwidth B:
- E[ε2(f)]=∫B0[αII(f)−αI(f)]2df⇒Minimum.
- The difference ε2(f) and the mean square error E[ε2(f)] are obtained as follows:
- ε2(f)=[α1⋅f+α2⋅√f−k2⋅(f/f0)k3]2=α21⋅f2+2α1α2⋅f1.5+α21⋅f+k22⋅f2k3f2k30−2k2α1⋅fk3+1fk30−2k2α2⋅fk3+0.5fk30
- ⇒E[ε2(f)]=α21⋅B33+45⋅α1α2⋅B2.5+α21⋅B22+k222k3+1⋅B2k3+1f2k30−2k2α1k3+2⋅
- This equation contains the cable parameters α1, α2, k2 and k3 to be calculated as well as the bandwidth B, within which the approximation should be valid.
- By setting the derivatives of E[ε2(f)] to α1 and α2 to zero, two equations are obtained for the best possible coefficients α1 and α2 that minimize the mean square error. These can be represented in the following form:
- dE[ε2(f)]dα1=0⇒α1+C1⋅α2+C2=0,
- dE[ε2(f)]dα2=0⇒α1+D1⋅α2+D2=0.
- From the equation C1⋅α2+C2=D1⋅α2+D2, the coefficient α2 can be calculated and then the coefficient α1 can be calculated from each of the two equations above.
The graph shows the attenuation function per unit length for a copper twin wire with 0.5 mm diameter, whose k–parameters are:
- k1=4.4dB/km,k2=10.8dB/km,k3=0.60.
- The red curve shows the function α(f) calculated with this parameters. For f=30 MHz the attenuation function per unit length is α(f)=87.5 dB/km.
- The blue curve gives the approximation with the α–coefficients. This is almost indistinguishable from the red curve within the drawing accuracy.
Notes:
- The exercise belongs to the chapter Properties of Balanced Copper Pairs.
- You can use the (German language) interactive SWF applet "Dämpfung von Kupferkabeln" ⇒ "Attenuation of copper cables" .
- [PW95] denotes the following literature reference: Pollakowski, P.; Wellhausen, H.-W.: Eigenschaften symmetrischer Ortsanschlusskabel im Frequenzbereich bis 30 MHz. Deutsche Telekom AG, Forschungs- und Technologiezentrum Darmstadt, 1995.
Questions
Solution
- The derivative of the given expected value with respect to α1 gives:
- dE[ε2(f)]dα1=23⋅B3⋅α1+45⋅B2.5⋅α2−2k2k3+2⋅Bk3+2fk30=0.
- By setting it to zero and dividing by 2B2/3, we obtain:
- α1+65⋅B−0.5⋅α2−3k2k3+2⋅Bk3−1fk30=0⇒C1=65⋅B−0.5,C2=−3k2k3+2⋅Bk3−1fk30.
(2) Solutions 2 and 5 are correct:
- Using the same procedure as in subtask (1), we obtain:
- dE[ε2(f)]dα2=45⋅B2.5⋅α1+B2⋅α2−2k2k3+1.5⋅Bk3+1.5fk30=0
- ⇒α1+54⋅B−0.5⋅α2−2.5⋅k2k3+1.5⋅Bk3−1fk30=0⇒D1=54⋅B−0.5,D2=−2.5⋅k2k3+1.5⋅Bk3−1fk30.
(3) Both solutions are correct:
- From C1⋅α2+C2=D1⋅α2+D2 we obtain a linear equation for α2. With the result from (2) we can write:
- α2=D2−C2C1−D1=−2.5⋅k2k3+1.5⋅Bk3−1fk30+3k2k3+2⋅Bk3−1fk306/5⋅B−0.5−5/4⋅B−0.5=−2.5⋅k2⋅(k3+2)+3k2⋅(k3+1.5)(6/5−5/4)(k3+1.5)(k3+2)⋅Bk3−0.5fk30
- ⇒α2=10⋅(B/f0)k3−0.5⋅1−k3(k3+1.5)(k3+2)⋅k2√f0.
- For the parameter α1 then holds:
- α1=−C1⋅α2−C2=−65⋅B−0.5⋅10⋅(B/f0)k3−0.5⋅1−k3(k3+1.5)(k3+2)⋅k2√f0+3k2k3+2⋅Bk3−1fk30
- ⇒α1=(B/f0)k3−1⋅−12⋅(1−k3)+3⋅(k3+1.5)(k3+1.5)(k3+2)⋅k2f0⇒α1=15⋅(B/f0)k3−1⋅k3−0.5(k3+1.5)(k3+2)⋅k2f0.
- Regardless of the bandwidth, we obtain for k3=1:
- α1=(B/f0)k3−1⋅15⋅(k3−0.5)(k3+1.5)(k3+2)⋅k2f0=15⋅0.52.5⋅3⋅k2f0=k2/f0_,
- α2=(B/f0)k3−0.5⋅10⋅(1−k3)(k3+1.5)(k3+2)⋅k2√f0=0_.
- In contrast, for k3=0.5:
- α1=(B/f0)k3−1⋅15⋅(k3−0.5)(k3+1.5)(k3+2)⋅k2f0=0_,
- α2=(B/f0)k3−0.5⋅10⋅(1−k3)(k3+1.5)(k3+2)⋅k2√f0=10⋅0.52⋅2.5⋅k2√f0=k2/√f0_.
(4) For the two coefficients, with k2=10.8 dB/km, k3=0.6 dB/km and B/f0=30:
- α1=(B/f0)k3−1⋅15⋅(k3−0.5)(k3+1.5)(k3+2)⋅k2f0=30−0.4⋅15⋅0.12.1⋅2.6⋅10.8dB/km1MHz≈0.761dB/(km⋅MHz)_,
- α2=(B/f0)k3−0.5⋅10⋅(1−k3)(k3+1.5)(k3+2)⋅k2√f0=k2√f0=300.1⋅10⋅0.42.1⋅2.6⋅10.8dB/km1MHz0.5≈11.1dB/(km⋅√MHz)_.
(5) According to the given equation αII(f) thus also holds:
- αII(f=30MHz)=α0+α1⋅f+α2⋅√f=[4.4+0.761⋅30+11.1⋅√30]dBkm≈88.1dB/km_.