Difference between revisions of "Aufgaben:Exercise 1.3: Rectangular Functions for Transmitter and Receiver"
| Line 1: | Line 1: | ||
| − | {{quiz-Header|Buchseite= | + | {{quiz-Header|Buchseite=Digital_Signal_Transmission/Error_Probability_for_Baseband_Transmission |
}} | }} | ||
| − | [[File: P_ID1267__Dig_A_1_3.png|right|frame| | + | [[File: P_ID1267__Dig_A_1_3.png|right|frame|Three different system concepts]] |
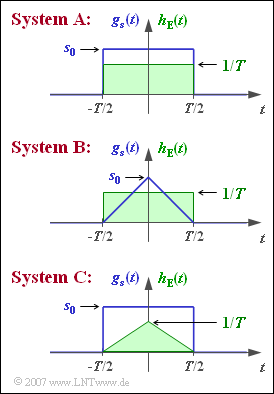
| − | + | We consider here three variants of a binary bipolar AWGN transmission system which differ with respect to the basic transmission pulse $g_{s}(t)$ as well as the impulse response $h_{\rm E}(t)$ of the receiver filter: | |
| − | * | + | *For $\text{System A}$, both $g_{s}(t)$ and $h_{\rm E}(t)$ are rectangular, only the pulse heights $(s_{\rm 0}$ and $1/T)$ are different. |
| − | * | + | * $\text{System B}$ differs from $\text{System A}$ by having a triangular-shaped basic transmission pulse with $g_{s}(t=0) = s_{\rm 0}$. |
| − | * | + | * $\text{System C}$ has the same basic transmission pulse as $\text{System A}$, while the impulse response is triangular with $h_{\rm E}(t=0) = 1/T$. |
| − | + | The absolute width of the rectangular and triangular functions considered here is $T = 10 \ \rm µ s$ each. The bit rate is $R = 100 \ \rm kbit/s$. The other system parameters are given as follows: | |
:$$s_0 = 6 \,\,\sqrt{W}\hspace{0.05cm},\hspace{0.3cm} N_{\rm 0} = 2 \cdot 10^{-5} \,\,{\rm W/Hz}\hspace{0.05cm}.$$ | :$$s_0 = 6 \,\,\sqrt{W}\hspace{0.05cm},\hspace{0.3cm} N_{\rm 0} = 2 \cdot 10^{-5} \,\,{\rm W/Hz}\hspace{0.05cm}.$$ | ||
| Line 16: | Line 16: | ||
| − | '' | + | ''Notes:'' |
| − | * | + | *The exercise belongs to the chapter [[Digital_Signal_Transmission/Error_Probability_for_Baseband_Transmission|Error Probability for Baseband Transmission]]. |
| − | * | + | *You can use the interactive applet [[Applets:Komplementäre_Gaußsche_Fehlerfunktionen|Komplementäre Gaußsche Fehlerfunktionen]] to determine error probabilities. |
| − | * | + | *Consider [[Theory_of_Stochastic_Signals/Power-Spectral_Density#Wiener-Khintchine_Theorem|Wiener-Chintchine's theorem]] when calculating the detection noise power: |
:$$ \sigma _d ^2 = \frac{N_0 }{2} \cdot \int_{ - \infty }^{ | :$$ \sigma _d ^2 = \frac{N_0 }{2} \cdot \int_{ - \infty }^{ | ||
+ \infty } {\left| {H_{\rm E}( f )} \right|^2 | + \infty } {\left| {H_{\rm E}( f )} \right|^2 | ||
| Line 28: | Line 28: | ||
| − | === | + | ===Questions=== |
<quiz display=simple> | <quiz display=simple> | ||
| − | { | + | {Calculate for $\text{System A}$ the basic detection pulse $g_{d}(t) = g_{ s}(t) \star h_{\rm E}(t)$. <br>What value $g_0 = g_{d}(t=0)$ results at time $t = 0$? |
|type="{}"} | |type="{}"} | ||
$g_0 \hspace{0.28cm} = \ $ { 6 3% } $\ \rm W^{1/2}$ | $g_0 \hspace{0.28cm} = \ $ { 6 3% } $\ \rm W^{1/2}$ | ||
| − | { | + | {From this, calculate the detection noise power $σ_{d}^2$. |
|type="{}"} | |type="{}"} | ||
$σ_{d}^{\hspace{0.02cm}2} \hspace{0.2cm} = \ $ { 1 3% } $\ \rm W$ | $σ_{d}^{\hspace{0.02cm}2} \hspace{0.2cm} = \ $ { 1 3% } $\ \rm W$ | ||
| − | { | + | {Thus, what is the bit error probability $p_{\rm B}$ for $\text{System A}$? |
|type="{}"} | |type="{}"} | ||
$p_{\rm B} \hspace{0.2cm} = \ $ { 0.987 10% } $\ \cdot 10^{-9}$ | $p_{\rm B} \hspace{0.2cm} = \ $ { 0.987 10% } $\ \cdot 10^{-9}$ | ||
| − | { | + | {Determine the corresponding quantities for $\text{System B}$ . |
|type="{}"} | |type="{}"} | ||
$g_0 \hspace{0.28cm} = \ $ { 3 3% } $\ \rm W^{1/2}$ | $g_0 \hspace{0.28cm} = \ $ { 3 3% } $\ \rm W^{1/2}$ | ||
| Line 49: | Line 49: | ||
$p_{\rm B} \hspace{0.2cm} = \ $ { 0.135 10% } $\ \cdot 10^{-2}$ | $p_{\rm B} \hspace{0.2cm} = \ $ { 0.135 10% } $\ \cdot 10^{-2}$ | ||
| − | { | + | {What are the characteristics for $\text{System C}$ ? |
|type="{}"} | |type="{}"} | ||
$g_0 \hspace{0.28cm} = \ $ { 3 3% } $\ \rm W^{1/2}$ | $g_0 \hspace{0.28cm} = \ $ { 3 3% } $\ \rm W^{1/2}$ | ||
| Line 57: | Line 57: | ||
</quiz> | </quiz> | ||
| − | === | + | ===Solution=== |
{{ML-Kopf}} | {{ML-Kopf}} | ||
| − | '''1.''' | + | '''1.''' For '''System A''', the convolution of the two equal-width rectangular functions $g_{s}(t)$ and $h_{\rm E}(t)$ leads to a triangular basic detection pulse with the maximum at $t = 0$: |
:$$g_d (t = 0) = \int_{ - T/2}^{ | :$$g_d (t = 0) = \int_{ - T/2}^{ | ||
+ T/2} { g_s(t) \cdot h_{\rm E}( t )} \hspace{0.1cm}{\rm{d}}t =s_0 | + T/2} { g_s(t) \cdot h_{\rm E}( t )} \hspace{0.1cm}{\rm{d}}t =s_0 | ||
\cdot \frac{1 }{T} \cdot T = s_0 \hspace{0.1cm}\underline { = 6 \,\,\sqrt{{\rm | \cdot \frac{1 }{T} \cdot T = s_0 \hspace{0.1cm}\underline { = 6 \,\,\sqrt{{\rm | ||
W}}}\hspace{0.05cm}.$$ | W}}}\hspace{0.05cm}.$$ | ||
| − | + | There is no intersymbol interfering because for $| t |\ge T$ the detection pulse is $g_{d}(t) = 0$. | |
| − | '''2.''' | + | '''2.''' The variance of the noise component of the detection signal – referred to here as the detection noise power – can be calculated in both the time and frequency domains. |
| − | * | + | *For the present rectangular waveform, calculation in the time domain yields faster results: |
:$$\sigma _d ^2 \ = \ \frac{N_0 }{2} \cdot \int_{ - | :$$\sigma _d ^2 \ = \ \frac{N_0 }{2} \cdot \int_{ - | ||
\infty }^{ + \infty } {\left| {h_{\rm E}( t )} \right|^2 | \infty }^{ + \infty } {\left| {h_{\rm E}( t )} \right|^2 | ||
| Line 77: | Line 77: | ||
\,\,{\rm W/Hz}}{2 \cdot 10^{-5} \,\,{\rm s}} \hspace{0.1cm}\underline {= 1\,{\rm | \,\,{\rm W/Hz}}{2 \cdot 10^{-5} \,\,{\rm s}} \hspace{0.1cm}\underline {= 1\,{\rm | ||
W}}\hspace{0.05cm}.$$ | W}}\hspace{0.05cm}.$$ | ||
| − | * | + | *The frequency domain calculation would be as follows with $H_{\rm E}(f) = {\rm si}(πfT)$: |
:$$\sigma _d ^2 = \frac{N_0 }{2} \cdot \int_{ - \infty }^{ | :$$\sigma _d ^2 = \frac{N_0 }{2} \cdot \int_{ - \infty }^{ | ||
+ \infty } {\left| {H_{\rm E}( f )} \right|^2 | + \infty } {\left| {H_{\rm E}( f )} \right|^2 | ||
| Line 85: | Line 85: | ||
| − | '''3.''' | + | '''3.''' Due to the time-limited pulse shape (this means: no intersymbol interfering!), the bipolar approach assumed here yields: |
:$$p_{\rm B} = {\rm Q} \left( \frac{s_0}{\sigma_d}\right)= {\rm Q} \left( \frac{ 6 \,\sqrt{\rm W}}{1 \,\sqrt{\rm W}}\right) | :$$p_{\rm B} = {\rm Q} \left( \frac{s_0}{\sigma_d}\right)= {\rm Q} \left( \frac{ 6 \,\sqrt{\rm W}}{1 \,\sqrt{\rm W}}\right) | ||
= {\rm Q}(6) \hspace{0.1cm}\underline {= 0.987 \cdot 10^{-9}} \hspace{0.05cm}.$$ | = {\rm Q}(6) \hspace{0.1cm}\underline {= 0.987 \cdot 10^{-9}} \hspace{0.05cm}.$$ | ||
| − | '''System A''' | + | '''System A''' represents the matched filter realization of the optimal binary receiver, so the following equations would also be applicable: |
:$$E_{\rm B} = s_0^2 \cdot T = 36\, {\rm W} \cdot 10^{-5} {\rm s}\hspace{0.3cm} | :$$E_{\rm B} = s_0^2 \cdot T = 36\, {\rm W} \cdot 10^{-5} {\rm s}\hspace{0.3cm} | ||
\Rightarrow \hspace{0.3cm} p_{\rm B} = {\rm Q} \left( \sqrt{\frac{2 \cdot E_{\rm B}}{N_0}}\right) | \Rightarrow \hspace{0.3cm} p_{\rm B} = {\rm Q} \left( \sqrt{\frac{2 \cdot E_{\rm B}}{N_0}}\right) | ||
| Line 96: | Line 96: | ||
| − | '''4.''' | + | '''4.''' Since '''System B''' uses the same receiver filter as '''System A''', the same detection noise power $σ_{d}^2 = 1 \ \rm W$ is also obtained. |
| − | * | + | *However, the basic detection pulse is now no longer triangular, but has a more pointed shape. At time $t = 0$ applies: |
:$$g_d (t = 0) = \frac{1}{T} \cdot \int_{ - T/2}^{ | :$$g_d (t = 0) = \frac{1}{T} \cdot \int_{ - T/2}^{ | ||
+ T/2} { g_s(t) } \hspace{0.1cm}{\rm{d}}t = \frac{1}{T} \cdot | + T/2} { g_s(t) } \hspace{0.1cm}{\rm{d}}t = \frac{1}{T} \cdot | ||
\frac{s_0 }{2} \cdot T = \frac{s_0 }{2}\hspace{0.1cm}\underline {= 3 \,\,\sqrt{\rm | \frac{s_0 }{2} \cdot T = \frac{s_0 }{2}\hspace{0.1cm}\underline {= 3 \,\,\sqrt{\rm | ||
W}}\hspace{0.05cm}.$$ | W}}\hspace{0.05cm}.$$ | ||
| − | * | + | *'''System B''' is also free of intersymbol interfering. Therefore, one obtains for the bit error probability: |
:$$p_{\rm B} = {\rm Q} \left( \frac{g_d (t = 0)}{\sigma_d}\right)= {\rm Q} \left( \frac{ 3 \,\sqrt{\rm W}}{1 \,\sqrt{\rm W}}\right) | :$$p_{\rm B} = {\rm Q} \left( \frac{g_d (t = 0)}{\sigma_d}\right)= {\rm Q} \left( \frac{ 3 \,\sqrt{\rm W}}{1 \,\sqrt{\rm W}}\right) | ||
= {\rm Q}(3) \hspace{0.1cm}\underline {= 0.135 \cdot 10^{-2}} \hspace{0.05cm}.$$ | = {\rm Q}(3) \hspace{0.1cm}\underline {= 0.135 \cdot 10^{-2}} \hspace{0.05cm}.$$ | ||
| − | * | + | *On the other hand, the following calculation is not applicable here: |
:$$E_{\rm B} = \int^{+\infty} _{-\infty} g_s^2(t)\,{\rm | :$$E_{\rm B} = \int^{+\infty} _{-\infty} g_s^2(t)\,{\rm | ||
d}t = 2\cdot s_0^2 \cdot \int ^{+T/2} _{0} \left( 1- \frac{2t}{T}\right)^2\,{\rm | d}t = 2\cdot s_0^2 \cdot \int ^{+T/2} _{0} \left( 1- \frac{2t}{T}\right)^2\,{\rm | ||
| Line 112: | Line 112: | ||
={\rm Q} \left( \sqrt{12}\right)={\rm Q}(3.464) \approx 3 \cdot 10^{-4} | ={\rm Q} \left( \sqrt{12}\right)={\rm Q}(3.464) \approx 3 \cdot 10^{-4} | ||
\hspace{0.05cm}.$$ | \hspace{0.05cm}.$$ | ||
| − | * | + | *One would thus compute a bit error probability that is too low, since the implicit assumption of a matched filter does not hold. |
| − | '''5.''' | + | '''5.''' For rectangular basic transmission pulse and triangular impulse response ⇒ '''System C''', the same basic detection pulse is obtained as for triangular $g_{\rm s}(t)$ and rectangular $h_{\rm E}(t)$. |
| − | * | + | *Therefore, as in '''System B''': |
:$$g_d (t = 0) = \frac{s_0}{2}\hspace{0.1cm}\underline {= 3 \,\,\sqrt{\rm | :$$g_d (t = 0) = \frac{s_0}{2}\hspace{0.1cm}\underline {= 3 \,\,\sqrt{\rm | ||
W}}\hspace{0.05cm}.$$ | W}}\hspace{0.05cm}.$$ | ||
| − | * | + | *In contrast, the detection noise power is now smaller than in systems A and B: |
:$$\sigma _d ^2 = \frac{N_0}{2} \cdot \frac{1}{T^2} \cdot \int^{+T/2} _{-T/2} \left( 1- \frac{2t}{T}\right)^2\,{\rm | :$$\sigma _d ^2 = \frac{N_0}{2} \cdot \frac{1}{T^2} \cdot \int^{+T/2} _{-T/2} \left( 1- \frac{2t}{T}\right)^2\,{\rm | ||
d}t = \frac{N_0}{6T}\hspace{0.1cm}\underline { = 0.333 \,{\rm W}}.$$ | d}t = \frac{N_0}{6T}\hspace{0.1cm}\underline { = 0.333 \,{\rm W}}.$$ | ||
| − | * | + | *This now gives us for the bit error probability: |
:$$p_{\rm B} = {\rm Q} \left( \frac{ 3 \,\sqrt{\rm W}}{0.577 \,\sqrt{\rm W}}\right) | :$$p_{\rm B} = {\rm Q} \left( \frac{ 3 \,\sqrt{\rm W}}{0.577 \,\sqrt{\rm W}}\right) | ||
\approx {\rm Q}(5.2)\hspace{0.1cm}\underline { \approx 10^{-7} } \hspace{0.05cm}.$$ | \approx {\rm Q}(5.2)\hspace{0.1cm}\underline { \approx 10^{-7} } \hspace{0.05cm}.$$ | ||
| − | * | + | *The apparent increase in error probability by a factor of about $100$ compared to subtask '''(3)''' is due to the severe mismatch compared to the matched filter. |
| − | * | + | *The improvement over subtask '''(4)''' is due to the higher signal energy. |
{{ML-Fuß}} | {{ML-Fuß}} | ||
Revision as of 17:46, 2 March 2022
We consider here three variants of a binary bipolar AWGN transmission system which differ with respect to the basic transmission pulse $g_{s}(t)$ as well as the impulse response $h_{\rm E}(t)$ of the receiver filter:
- For $\text{System A}$, both $g_{s}(t)$ and $h_{\rm E}(t)$ are rectangular, only the pulse heights $(s_{\rm 0}$ and $1/T)$ are different.
- $\text{System B}$ differs from $\text{System A}$ by having a triangular-shaped basic transmission pulse with $g_{s}(t=0) = s_{\rm 0}$.
- $\text{System C}$ has the same basic transmission pulse as $\text{System A}$, while the impulse response is triangular with $h_{\rm E}(t=0) = 1/T$.
The absolute width of the rectangular and triangular functions considered here is $T = 10 \ \rm µ s$ each. The bit rate is $R = 100 \ \rm kbit/s$. The other system parameters are given as follows:
- $$s_0 = 6 \,\,\sqrt{W}\hspace{0.05cm},\hspace{0.3cm} N_{\rm 0} = 2 \cdot 10^{-5} \,\,{\rm W/Hz}\hspace{0.05cm}.$$
Notes:
- The exercise belongs to the chapter Error Probability for Baseband Transmission.
- You can use the interactive applet Komplementäre Gaußsche Fehlerfunktionen to determine error probabilities.
- Consider Wiener-Chintchine's theorem when calculating the detection noise power:
- $$ \sigma _d ^2 = \frac{N_0 }{2} \cdot \int_{ - \infty }^{ + \infty } {\left| {H_{\rm E}( f )} \right|^2 \hspace{0.1cm}{\rm{d}}f} = \frac{N_0 }{2} \cdot \int_{ - \infty }^{ + \infty } {\left| {h_{\rm E}( t )} \right|^2 \hspace{0.1cm}{\rm{d}}t}\hspace{0.05cm}.$$
Questions
Solution
- $$g_d (t = 0) = \int_{ - T/2}^{ + T/2} { g_s(t) \cdot h_{\rm E}( t )} \hspace{0.1cm}{\rm{d}}t =s_0 \cdot \frac{1 }{T} \cdot T = s_0 \hspace{0.1cm}\underline { = 6 \,\,\sqrt{{\rm W}}}\hspace{0.05cm}.$$
There is no intersymbol interfering because for $| t |\ge T$ the detection pulse is $g_{d}(t) = 0$.
2. The variance of the noise component of the detection signal – referred to here as the detection noise power – can be calculated in both the time and frequency domains.
- For the present rectangular waveform, calculation in the time domain yields faster results:
- $$\sigma _d ^2 \ = \ \frac{N_0 }{2} \cdot \int_{ - \infty }^{ + \infty } {\left| {h_{\rm E}( t )} \right|^2 \hspace{0.1cm}{\rm{d}}t} =\frac{N_0 }{2} \cdot \int_{ - T/2 }^{ + T/2 } {\left| {h_{\rm E}( t )} \right|^2 \hspace{0.1cm}{\rm{d}}t} = \ \frac{N_0 }{2} \cdot\frac{1 }{T^2} \cdot T = \frac{N_0 }{2T} = \frac{2 \cdot 10^{-5} \,\,{\rm W/Hz}}{2 \cdot 10^{-5} \,\,{\rm s}} \hspace{0.1cm}\underline {= 1\,{\rm W}}\hspace{0.05cm}.$$
- The frequency domain calculation would be as follows with $H_{\rm E}(f) = {\rm si}(πfT)$:
- $$\sigma _d ^2 = \frac{N_0 }{2} \cdot \int_{ - \infty }^{ + \infty } {\left| {H_{\rm E}( f )} \right|^2 \hspace{0.1cm}{\rm{d}}f} = \frac{N_0 }{2} \cdot \int_{- \infty }^{ \infty } {\rm si}^2(\pi f T)\hspace{0.1cm}{\rm{d}}f = \frac{N_0 }{2T} \hspace{0.05cm}.$$
3. Due to the time-limited pulse shape (this means: no intersymbol interfering!), the bipolar approach assumed here yields:
- $$p_{\rm B} = {\rm Q} \left( \frac{s_0}{\sigma_d}\right)= {\rm Q} \left( \frac{ 6 \,\sqrt{\rm W}}{1 \,\sqrt{\rm W}}\right) = {\rm Q}(6) \hspace{0.1cm}\underline {= 0.987 \cdot 10^{-9}} \hspace{0.05cm}.$$
System A represents the matched filter realization of the optimal binary receiver, so the following equations would also be applicable:
- $$E_{\rm B} = s_0^2 \cdot T = 36\, {\rm W} \cdot 10^{-5} {\rm s}\hspace{0.3cm} \Rightarrow \hspace{0.3cm} p_{\rm B} = {\rm Q} \left( \sqrt{\frac{2 \cdot E_{\rm B}}{N_0}}\right) ={\rm Q} \left( \sqrt{\frac{2 \cdot 36 \cdot 10^{-5}\,\, {\rm Ws}}{2 \cdot 10^{-5} \,\, {\rm Ws}}}\right)={\rm Q}(6) \hspace{0.05cm}.$$
4. Since System B uses the same receiver filter as System A, the same detection noise power $σ_{d}^2 = 1 \ \rm W$ is also obtained.
- However, the basic detection pulse is now no longer triangular, but has a more pointed shape. At time $t = 0$ applies:
- $$g_d (t = 0) = \frac{1}{T} \cdot \int_{ - T/2}^{ + T/2} { g_s(t) } \hspace{0.1cm}{\rm{d}}t = \frac{1}{T} \cdot \frac{s_0 }{2} \cdot T = \frac{s_0 }{2}\hspace{0.1cm}\underline {= 3 \,\,\sqrt{\rm W}}\hspace{0.05cm}.$$
- System B is also free of intersymbol interfering. Therefore, one obtains for the bit error probability:
- $$p_{\rm B} = {\rm Q} \left( \frac{g_d (t = 0)}{\sigma_d}\right)= {\rm Q} \left( \frac{ 3 \,\sqrt{\rm W}}{1 \,\sqrt{\rm W}}\right) = {\rm Q}(3) \hspace{0.1cm}\underline {= 0.135 \cdot 10^{-2}} \hspace{0.05cm}.$$
- On the other hand, the following calculation is not applicable here:
- $$E_{\rm B} = \int^{+\infty} _{-\infty} g_s^2(t)\,{\rm d}t = 2\cdot s_0^2 \cdot \int ^{+T/2} _{0} \left( 1- \frac{2t}{T}\right)^2\,{\rm d}t = \frac{s_0^2 \cdot T }{3} = 12 \cdot 10^{-5} \,{\rm Ws}$$
- $$\Rightarrow \hspace{0.3cm} p_{\rm B} = {\rm Q} \left( \sqrt{\frac{2 \cdot E_{\rm B}}{N_0}}\right) ={\rm Q} \left( \sqrt{12}\right)={\rm Q}(3.464) \approx 3 \cdot 10^{-4} \hspace{0.05cm}.$$
- One would thus compute a bit error probability that is too low, since the implicit assumption of a matched filter does not hold.
5. For rectangular basic transmission pulse and triangular impulse response ⇒ System C, the same basic detection pulse is obtained as for triangular $g_{\rm s}(t)$ and rectangular $h_{\rm E}(t)$.
- Therefore, as in System B:
- $$g_d (t = 0) = \frac{s_0}{2}\hspace{0.1cm}\underline {= 3 \,\,\sqrt{\rm W}}\hspace{0.05cm}.$$
- In contrast, the detection noise power is now smaller than in systems A and B:
- $$\sigma _d ^2 = \frac{N_0}{2} \cdot \frac{1}{T^2} \cdot \int^{+T/2} _{-T/2} \left( 1- \frac{2t}{T}\right)^2\,{\rm d}t = \frac{N_0}{6T}\hspace{0.1cm}\underline { = 0.333 \,{\rm W}}.$$
- This now gives us for the bit error probability:
- $$p_{\rm B} = {\rm Q} \left( \frac{ 3 \,\sqrt{\rm W}}{0.577 \,\sqrt{\rm W}}\right) \approx {\rm Q}(5.2)\hspace{0.1cm}\underline { \approx 10^{-7} } \hspace{0.05cm}.$$
- The apparent increase in error probability by a factor of about $100$ compared to subtask (3) is due to the severe mismatch compared to the matched filter.
- The improvement over subtask (4) is due to the higher signal energy.