Difference between revisions of "Aufgaben:Exercise 1.5: Cosine-Square Spectrum"
m (Text replacement - "Category:Aufgaben zu Digitalsignalübertragung" to "Category:Digital Signal Transmission: Exercises") |
|||
| (6 intermediate revisions by 2 users not shown) | |||
| Line 1: | Line 1: | ||
| − | {{quiz-Header|Buchseite= | + | {{quiz-Header|Buchseite=Digital_Signal_Transmission/Properties_of_Nyquist_Systems |
}} | }} | ||
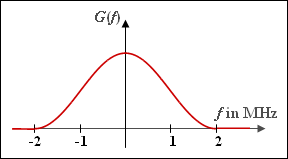
| − | [[File:P_ID1282__Dig_A_1_5.png|right|frame| | + | [[File:P_ID1282__Dig_A_1_5.png|right|frame|Cosine-square Nyquist spectrum]] |
| − | + | The spectrum G(f) with cos2–shaped course is considered according to the sketch. This satisfies the first Nyquist criterion: | |
:+∞∑k=−∞G(f−k/T)=const. | :+∞∑k=−∞G(f−k/T)=const. | ||
| − | + | Accordingly, the associated pulse g(t) has zero crossings at multiples of T, where T remains to be determined. The inverse Fourier transform of G(f) yields the equation for the time course: | |
:$$g( t )= g_0 \cdot \frac{\cos(\pi \cdot t/T)}{1 - (2 \cdot | :$$g( t )= g_0 \cdot \frac{\cos(\pi \cdot t/T)}{1 - (2 \cdot | ||
| − | t/T)^2}\cdot {\rm | + | t/T)^2}\cdot {\rm sinc}( {t}/{T})\hspace{0.5cm} \text{with}\hspace{0.5cm} {\rm sinc}(x)=\sin(\pi x)/(\pi x)\hspace{0.05cm}.$$ |
| − | + | The questions for this exercise refer to the following properties: | |
| − | * | + | *The spectral function G(f) is a special case of the cosine rolloff spectrum, which is point symmetric about the Nyquist frequency fNyq. |
| − | * | + | *The cosine rolloff spectrum is completely characterized by the corner frequencies f1 and f2. |
| − | * | + | * For |f|<f1, G(f)=g0⋅T=const., while the spectrum for |f|>f2 has no components. |
| − | * | + | *The relation between the Nyquist frequency and the corner frequencies is: |
:$$f_{\rm Nyq}= \frac{f_1 +f_2 } | :$$f_{\rm Nyq}= \frac{f_1 +f_2 } | ||
{2 }\hspace{0.05cm}.$$ | {2 }\hspace{0.05cm}.$$ | ||
| − | * | + | *The edge steepness is characterized by the so-called rolloff factor: |
:$$r = \frac{f_2 -f_1 } | :$$r = \frac{f_2 -f_1 } | ||
{f_2 +f_1 }\hspace{0.2cm}(0 \le r \le 1) \hspace{0.05cm}.$$ | {f_2 +f_1 }\hspace{0.2cm}(0 \le r \le 1) \hspace{0.05cm}.$$ | ||
| Line 23: | Line 23: | ||
| − | + | Note: The exercise belongs to the chapter [[Digital_Signal_Transmission/Properties_of_Nyquist_Systems|"Properties of Nyquist Systems"]]. | |
| − | |||
| − | |||
| − | |||
| − | === | + | ===Questions=== |
<quiz display=simple> | <quiz display=simple> | ||
| − | { | + | {What are the corner frequencies of this cosine rolloff spectrum? |
|type="{}"} | |type="{}"} | ||
f1 = { 0 3% } MHz | f1 = { 0 3% } MHz | ||
f2 = { 2 3% } MHz | f2 = { 2 3% } MHz | ||
| − | { | + | {What are the Nyquist frequency and the rolloff factor? |
|type="{}"} | |type="{}"} | ||
fNyq = { 1 3% } MHz | fNyq = { 1 3% } MHz | ||
r = { 1 3% } | r = { 1 3% } | ||
| − | { | + | {At what time interval T does g(t) have zero crossings? |
|type="{}"} | |type="{}"} | ||
T = { 0.5 3% } \ \rm µ s | T = { 0.5 3% } \ \rm µ s | ||
| − | { | + | {Which of the following statements is true? |
|type="[]"} | |type="[]"} | ||
| − | + g(t) | + | + g(t) satisfies the first Nyquist criterion because of the $\rm sinc$–term. |
| − | - g(t) | + | - g(t) has further zero crossings at \pm 0.5T, \pm 1.5T, \pm 2.5 T, \text{...} |
| − | + | + | + The cos2–spectrum also satisfies the second Nyquist criterion. |
| − | { | + | {What is the (normalized) value of the pulse at time t=T/2? |
|type="{}"} | |type="{}"} | ||
g(t=T/2)/g0 = { 0.5 3% } | g(t=T/2)/g0 = { 0.5 3% } | ||
| Line 63: | Line 60: | ||
</quiz> | </quiz> | ||
| − | === | + | ===Solution=== |
{{ML-Kopf}} | {{ML-Kopf}} | ||
| − | '''(1)''' | + | '''(1)''' The upper corner frequency can be read from the diagram: f2=2 MHz_. Since the spectrum is not constant in any range, f1=0_. |
| − | '''(2)''' | + | '''(2)''' From the given equations we obtain: |
:$$f_{\rm Nyq} = \ \frac{f_1 +f_2 } | :$$f_{\rm Nyq} = \ \frac{f_1 +f_2 } | ||
{2 }\hspace{0.1cm}\underline { = 1\,{\rm MHz}}\hspace{0.05cm},\hspace{0.5cm} r = \ \frac{f_2 -f_1 } {f_2 +f_1 }\hspace{0.1cm}\underline { = 1 }\hspace{0.05cm}.$$ | {2 }\hspace{0.1cm}\underline { = 1\,{\rm MHz}}\hspace{0.05cm},\hspace{0.5cm} r = \ \frac{f_2 -f_1 } {f_2 +f_1 }\hspace{0.1cm}\underline { = 1 }\hspace{0.05cm}.$$ | ||
| − | '''(3)''' | + | '''(3)''' The spacing of equidistant zero crossings is directly related to the Nyquist frequency: |
:f_{\rm Nyq}= \frac{1}{2T} \hspace{0.3cm} \Rightarrow \hspace{0.3cm} T= \frac{1}{2f_{\rm Nyq}}\hspace{0.1cm}\underline { = 0.5\,{\rm µ s}}\hspace{0.05cm}. | :f_{\rm Nyq}= \frac{1}{2T} \hspace{0.3cm} \Rightarrow \hspace{0.3cm} T= \frac{1}{2f_{\rm Nyq}}\hspace{0.1cm}\underline { = 0.5\,{\rm µ s}}\hspace{0.05cm}. | ||
| − | '''(4)''' | + | '''(4)''' <u>Statements 1 and 3</u> are correct: |
| − | * | + | *The first statement is correct: The function ${\rm sinc}(t/T)$ leads to zero crossings at νT(ν≠0). |
| − | * | + | *The last statement is also true: Because of g(t)=0 for t=±1.5T,±2.5T,±3.5T,... the second Nyquist criterion is also fulfilled. |
| − | * | + | *On the other hand, the middle statement is false, since g(t=T/2)≠0. |
| − | + | *The condition for the second Nyquist criterion is in the frequency domain: | |
| − | * | ||
:$$G_{\rm Per}(f) = \sum_{k = -\infty}^{+\infty} \frac {G \left ( f - | :$$G_{\rm Per}(f) = \sum_{k = -\infty}^{+\infty} \frac {G \left ( f - | ||
| − | + | {k}/{T} \right)}{\cos(\pi \cdot f \cdot T - k \cdot \pi)}= | |
{\rm const.}$$ | {\rm const.}$$ | ||
| − | * | + | *The condition is indeed fulfilled for the cos2–spectrum, as can be shown after a longer calculation. |
| + | *We restrict ourselves here to the frequency range |f·T|≤1 and set g0⋅T=1 for simplicity: | ||
:$$G_{\rm Per}(f) = \frac {\cos^2 \left [\pi/2 \cdot ( f_{\rm Nyq} | :$$G_{\rm Per}(f) = \frac {\cos^2 \left [\pi/2 \cdot ( f_{\rm Nyq} | ||
- f) \cdot T \right ]}{\cos \left [\pi \cdot ( f_{\rm Nyq} - f) | - f) \cdot T \right ]}{\cos \left [\pi \cdot ( f_{\rm Nyq} - f) | ||
| Line 93: | Line 90: | ||
+ f) \cdot T \right ]}{\cos \left [\pi \cdot ( f_{\rm Nyq} + f) | + f) \cdot T \right ]}{\cos \left [\pi \cdot ( f_{\rm Nyq} + f) | ||
\cdot T \right ]}\hspace{0.05cm}.$$ | \cdot T \right ]}\hspace{0.05cm}.$$ | ||
| − | * | + | *Further holds: |
:$$\frac {\cos^2 (x)}{\cos(2x)} = {1}/{2} \cdot \frac | :$$\frac {\cos^2 (x)}{\cos(2x)} = {1}/{2} \cdot \frac | ||
{1+\cos(2x)}{\cos(2x)}= {1}/{2} \cdot \left [1+ \frac | {1+\cos(2x)}{\cos(2x)}= {1}/{2} \cdot \left [1+ \frac | ||
| Line 101: | Line 98: | ||
- f) \cdot T \right ]} +1- \frac {1}{\cos \left [\pi \cdot ( f_{\rm Nyq} | - f) \cdot T \right ]} +1- \frac {1}{\cos \left [\pi \cdot ( f_{\rm Nyq} | ||
+ f) \cdot T \right ]}\right ]\hspace{0.05cm}.$$ | + f) \cdot T \right ]}\right ]\hspace{0.05cm}.$$ | ||
| − | * | + | * Because of $\cos \left [ \pi \cdot ( f_{\rm Nyq} \pm f) \cdot T \right] = \cos |
\left ( {\pi}/{2} \pm \pi f T \right) = \sin \left ( \pm | \left ( {\pi}/{2} \pm \pi f T \right) = \sin \left ( \pm | ||
\pi f T \right)\text{:}$ | \pi f T \right)\text{:}$ | ||
| Line 108: | Line 105: | ||
| − | '''(5)''' | + | '''(5)''' For t=T/2, the given equation yields an indeterminate value ("0 divided by 0"), which can be determined using l'Hospital's rule. |
| − | * | + | *To do this, form the derivatives of the numerator and denominator and insert the desired time t=T/2 into the result: |
| − | :$$\frac{g( t = T/2)}{g_0} = \ {{\rm | + | :$$\frac{g( t = T/2)}{g_0} = \ {{\rm sinc}( \frac{t}{T}) |
\cdot \frac{{\rm d}/{\rm d}t \left [ \cos(\pi \cdot | \cdot \frac{{\rm d}/{\rm d}t \left [ \cos(\pi \cdot | ||
t/T)\right]}{{\rm d}/{\rm d}t\left [ 1 - (2 \cdot t/T)^2\right]}} | t/T)\right]}{{\rm d}/{\rm d}t\left [ 1 - (2 \cdot t/T)^2\right]}} | ||
| − | \bigg |_{t = T/2} = \ {{\rm | + | \bigg |_{t = T/2} = \ {{\rm sinc}( \frac{t}{T}) \cdot \frac{- \pi/T \cdot |
\sin(\pi \cdot t/T)}{-2 \cdot (2\cdot t/T) \cdot (2/T)}} \bigg |_{t = T/2} = \frac {2}{\pi}\cdot | \sin(\pi \cdot t/T)}{-2 \cdot (2\cdot t/T) \cdot (2/T)}} \bigg |_{t = T/2} = \frac {2}{\pi}\cdot | ||
\frac {\pi}{4}\hspace{0.1cm}\underline { = 0.5}\hspace{0.05cm}.$$ | \frac {\pi}{4}\hspace{0.1cm}\underline { = 0.5}\hspace{0.05cm}.$$ | ||
| − | * | + | *A second solution method leads to the expression: |
| − | :$$\frac{g( t )}{g_0} = {\rm | + | :$$\frac{g( t )}{g_0} = {\rm sinc}( \frac{t}{T}) \cdot |
| − | \frac {\pi}{4} \cdot \big [ {\rm | + | \frac {\pi}{4} \cdot \big [ {\rm sinc}( t/T + 1/2) + |
| − | {\rm | + | {\rm sinc}(t/T - 1/2)\big] \hspace{0.05cm}.$$ |
| − | * | + | *The second bracket expression can be transformed as follows: |
:$$\frac {\pi}{4} \cdot \bigg [ \hspace{0.1cm}... \hspace{0.1cm} | :$$\frac {\pi}{4} \cdot \bigg [ \hspace{0.1cm}... \hspace{0.1cm} | ||
\bigg ] = \ \frac {\pi}{4} \cdot \left [ \frac {{\rm sin}(\pi | \bigg ] = \ \frac {\pi}{4} \cdot \left [ \frac {{\rm sin}(\pi | ||
| Line 134: | Line 131: | ||
\cdot t/T)(1- 2 \cdot t/T)}= \frac{\cos(\pi \cdot t/T)}{1 - (2 | \cdot t/T)(1- 2 \cdot t/T)}= \frac{\cos(\pi \cdot t/T)}{1 - (2 | ||
\cdot t/T)^2}\hspace{0.05cm}.$$ | \cdot t/T)^2}\hspace{0.05cm}.$$ | ||
| − | * | + | *It follows that both expressions are actually equal. Thus, for time t=T/2, the following is still true: |
| − | :$$\frac{g( t = T/2)}{g_0} = {\rm | + | :$$\frac{g( t = T/2)}{g_0} = {\rm sinc}( 0.5) \cdot \frac |
| − | {\pi}{4} \cdot \ | + | {\pi}{4} \cdot \big [ {\rm sinc}(1 ) + {\rm sinc}(0)\big]= \frac |
{2}{\pi}\cdot \frac {\pi}{4} = 0.5 \hspace{0.05cm}.$$ | {2}{\pi}\cdot \frac {\pi}{4} = 0.5 \hspace{0.05cm}.$$ | ||
{{ML-Fuß}} | {{ML-Fuß}} | ||
| Line 142: | Line 139: | ||
| − | [[Category:Digital Signal Transmission: Exercises|^1.3 | + | [[Category:Digital Signal Transmission: Exercises|^1.3 Nyquist System Properties^]] |
Latest revision as of 14:24, 2 May 2022
The spectrum G(f) with cos2–shaped course is considered according to the sketch. This satisfies the first Nyquist criterion:
- +∞∑k=−∞G(f−k/T)=const.
Accordingly, the associated pulse g(t) has zero crossings at multiples of T, where T remains to be determined. The inverse Fourier transform of G(f) yields the equation for the time course:
- g(t)=g0⋅cos(π⋅t/T)1−(2⋅t/T)2⋅sinc(t/T)withsinc(x)=sin(πx)/(πx).
The questions for this exercise refer to the following properties:
- The spectral function G(f) is a special case of the cosine rolloff spectrum, which is point symmetric about the Nyquist frequency fNyq.
- The cosine rolloff spectrum is completely characterized by the corner frequencies f1 and f2.
- For |f|<f1, G(f)=g0⋅T=const., while the spectrum for |f|>f2 has no components.
- The relation between the Nyquist frequency and the corner frequencies is:
- fNyq=f1+f22.
- The edge steepness is characterized by the so-called rolloff factor:
- r=f2−f1f2+f1(0≤r≤1).
Note: The exercise belongs to the chapter "Properties of Nyquist Systems".
Questions
Solution
(2) From the given equations we obtain:
- f_{\rm Nyq} = \ \frac{f_1 +f_2 } {2 }\hspace{0.1cm}\underline { = 1\,{\rm MHz}}\hspace{0.05cm},\hspace{0.5cm} r = \ \frac{f_2 -f_1 } {f_2 +f_1 }\hspace{0.1cm}\underline { = 1 }\hspace{0.05cm}.
(3) The spacing of equidistant zero crossings is directly related to the Nyquist frequency:
- f_{\rm Nyq}= \frac{1}{2T} \hspace{0.3cm} \Rightarrow \hspace{0.3cm} T= \frac{1}{2f_{\rm Nyq}}\hspace{0.1cm}\underline { = 0.5\,{\rm µ s}}\hspace{0.05cm}.
(4) Statements 1 and 3 are correct:
- The first statement is correct: The function {\rm sinc}(t/T) leads to zero crossings at \nu T (\nu \neq 0).
- The last statement is also true: Because of g(t) = 0 for t =\pm 1.5T, \pm 2.5T, \pm 3.5T, ... the second Nyquist criterion is also fulfilled.
- On the other hand, the middle statement is false, since g(t = T/2) \neq 0.
- The condition for the second Nyquist criterion is in the frequency domain:
- G_{\rm Per}(f) = \sum_{k = -\infty}^{+\infty} \frac {G \left ( f - {k}/{T} \right)}{\cos(\pi \cdot f \cdot T - k \cdot \pi)}= {\rm const.}
- The condition is indeed fulfilled for the cos^{2}–spectrum, as can be shown after a longer calculation.
- We restrict ourselves here to the frequency range | f · T | \leq 1 and set g_{0} \cdot T = 1 for simplicity:
- G_{\rm Per}(f) = \frac {\cos^2 \left [\pi/2 \cdot ( f_{\rm Nyq} - f) \cdot T \right ]}{\cos \left [\pi \cdot ( f_{\rm Nyq} - f) \cdot T \right ]}+\frac {\cos^2 \left [\pi/2 \cdot ( f_{\rm Nyq} + f) \cdot T \right ]}{\cos \left [\pi \cdot ( f_{\rm Nyq} + f) \cdot T \right ]}\hspace{0.05cm}.
- Further holds:
- \frac {\cos^2 (x)}{\cos(2x)} = {1}/{2} \cdot \frac {1+\cos(2x)}{\cos(2x)}= {1}/{2} \cdot \left [1+ \frac {1}{\cos(2x)}\right ]
- \Rightarrow \hspace{0.3cm} G_{\rm Per}(f) = {1}/{2} \cdot \left [1+ \frac {1}{\cos \left [\pi \cdot ( f_{\rm Nyq} - f) \cdot T \right ]} +1- \frac {1}{\cos \left [\pi \cdot ( f_{\rm Nyq} + f) \cdot T \right ]}\right ]\hspace{0.05cm}.
- Because of \cos \left [ \pi \cdot ( f_{\rm Nyq} \pm f) \cdot T \right] = \cos \left ( {\pi}/{2} \pm \pi f T \right) = \sin \left ( \pm \pi f T \right)\text{:}
- \Rightarrow \hspace{0.3cm} G_{\rm Per}(f) = 2 - \frac {1}{\sin (\pi f T)} + \frac {1}{\sin (\pi f T)} = 2 = {\rm const}\hspace{0.05cm}.
(5) For t = T/2, the given equation yields an indeterminate value ("0 divided by 0"), which can be determined using l'Hospital's rule.
- To do this, form the derivatives of the numerator and denominator and insert the desired time t = T/2 into the result:
- \frac{g( t = T/2)}{g_0} = \ {{\rm sinc}( \frac{t}{T}) \cdot \frac{{\rm d}/{\rm d}t \left [ \cos(\pi \cdot t/T)\right]}{{\rm d}/{\rm d}t\left [ 1 - (2 \cdot t/T)^2\right]}} \bigg |_{t = T/2} = \ {{\rm sinc}( \frac{t}{T}) \cdot \frac{- \pi/T \cdot \sin(\pi \cdot t/T)}{-2 \cdot (2\cdot t/T) \cdot (2/T)}} \bigg |_{t = T/2} = \frac {2}{\pi}\cdot \frac {\pi}{4}\hspace{0.1cm}\underline { = 0.5}\hspace{0.05cm}.
- A second solution method leads to the expression:
- \frac{g( t )}{g_0} = {\rm sinc}( \frac{t}{T}) \cdot \frac {\pi}{4} \cdot \big [ {\rm sinc}( t/T + 1/2) + {\rm sinc}(t/T - 1/2)\big] \hspace{0.05cm}.
- The second bracket expression can be transformed as follows:
- \frac {\pi}{4} \cdot \bigg [ \hspace{0.1cm}... \hspace{0.1cm} \bigg ] = \ \frac {\pi}{4} \cdot \left [ \frac {{\rm sin}(\pi \cdot t/T + \pi/2)}{\pi \cdot t/T + \pi/2} + \frac {{\rm sin}(\pi \cdot t/T - \pi/2)}{\pi \cdot t/T - \pi/2}\right] = \ \frac {1}{2} \cdot {\rm cos}(\pi \cdot t/T )\cdot \left [ \frac {1}{2 \cdot t/T + 1} - \frac {1}{ 2 \cdot t/T - 1}\right]
- \Rightarrow \hspace{0.3cm} \frac {\pi}{4} \cdot \bigg [ \hspace{0.1cm}... \hspace{0.1cm} \bigg ] = \ \frac {1}{2} \cdot {\rm cos}(\pi \cdot t/T )\cdot \frac{1- 2 \cdot t/T + 1+ 2 \cdot t/T}{(1+ 2 \cdot t/T)(1- 2 \cdot t/T)}= \frac{\cos(\pi \cdot t/T)}{1 - (2 \cdot t/T)^2}\hspace{0.05cm}.
- It follows that both expressions are actually equal. Thus, for time t = T/2, the following is still true:
- \frac{g( t = T/2)}{g_0} = {\rm sinc}( 0.5) \cdot \frac {\pi}{4} \cdot \big [ {\rm sinc}(1 ) + {\rm sinc}(0)\big]= \frac {2}{\pi}\cdot \frac {\pi}{4} = 0.5 \hspace{0.05cm}.