Difference between revisions of "Aufgaben:Exercise 5.2: Error Correlation Function"
| Line 91: | Line 91: | ||
In the calculation, it was assumed that the individual error distances are statistically independent of each other. | In the calculation, it was assumed that the individual error distances are statistically independent of each other. | ||
*However, this assumption is valid only for a special class of channel models called "renewing". | *However, this assumption is valid only for a special class of channel models called "renewing". | ||
| − | *The | + | *The burst error model considered here does not satisfy this condition. |
*The actual probability ${\rm Pr}(a = 2) = 0.1675$ therefore deviates slightly from the value calculated here $(0.1715)$. | *The actual probability ${\rm Pr}(a = 2) = 0.1675$ therefore deviates slightly from the value calculated here $(0.1715)$. | ||
Revision as of 12:54, 26 August 2022
For the characterization of digital channel models one uses among other things
- the error correlation function (ECF)
- $$\varphi_{e}(k) = {\rm E}\big[e_{\nu} \cdot e_{\nu + k}\big]\hspace{0.05cm}, \hspace{0.2cm} k \ge 0\hspace{0.05cm},$$
- the error distance probabilities
- $${\rm Pr}( a =k) \hspace{0.05cm}, \hspace{0.2cm} k \ge 1\hspace{0.05cm}.$$
Here denote:
- $〈e_{\rm \nu}〉$ is the error sequence with $e_{\rm \nu} ∈ \{0, 1\}$.
- $a$ indicates the error distance.
Two directly consecutive bit errors are thus characterized by the error distance $a = 1$.
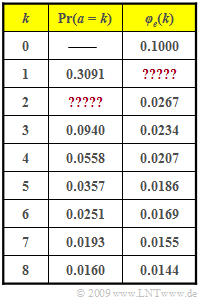
The table shows exemplary values of the error distance probabilities ${\rm Pr}(a = k)$ as well as the error correlation function $\varphi_e(k)$.
- Some data are missing in the table.
- These values are to be calculated from the given values.
Note:
- The exercise covers the subject matter of the chapter "Parameters of Digital Channel Models".
Questions
Solution
- $$\varphi_{e}(k = 0) = {\rm E}[e_{\nu}^2 ]= {\rm E}[e_{\nu} ]= p_{\rm M} \hspace{0.3cm} \Rightarrow \hspace{0.3cm}p_{\rm M}\hspace{0.15cm}\underline { = 0.1} \hspace{0.05cm}.$$
(2) The mean error distance is equal to the reciprocal of the mean error probability. That is:
- $${\rm E}\big[a\big] = 1/p_{\rm M} \ \underline {= 10}.$$
(3) According to the definition equation and "Bayes' theorem", the following result is obtained:
- $$\varphi_{e}(k = 1) \hspace{-0.1cm} \ = \ \hspace{-0.1cm} {\rm E}[e_{\nu} \cdot e_{\nu + 1}] = {\rm E}[(e_{\nu} = 1) \cdot (e_{\nu + 1}=1)]={\rm Pr}(e_{\nu + 1}=1 \hspace{0.05cm}|\hspace{0.05cm} e_{\nu} = 1) \cdot {\rm Pr}(e_{\nu} = 1) \hspace{0.05cm}.$$
- The first probability is equal to ${\rm Pr}(a = 1)$ and the second probability is equal to $p_{\rm M}$:
- $$\varphi_{e}(k = 1) = 0.3091 \cdot 0.1\hspace{0.15cm}\underline { = 0.0309} \hspace{0.05cm}.$$
(4) The ECF value $\varphi_e(k = 2)$ can be interpreted (approximately) as follows:
- $$\varphi_{e}(k = 2) ={\rm Pr}(e_{\nu + 2}=1 \hspace{0.05cm}|\hspace{0.05cm} e_{\nu} = 1) \cdot p_{\rm M} \hspace{0.3cm} \Rightarrow \hspace{0.3cm}{\rm Pr}(e_{\nu + 2}=1 \hspace{0.05cm}|\hspace{0.05cm} e_{\nu} = 1) = \frac{\varphi_{e}(k = 2)}{p_{\rm M}} = \frac{0.0267}{0.1} = 0.267\hspace{0.05cm}.$$
This probability is composed of "At time $\nu+1$ an error occurs" and "At time $\nu+1$ there is no error":
- $${\rm Pr}(e_{\nu + 2}=1 \hspace{0.05cm}|\hspace{0.05cm} e_{\nu} = 1) = {\rm Pr}( a =1) \cdot {\rm Pr}( a =1) + {\rm Pr}( a =2)\hspace{0.3cm} \Rightarrow \hspace{0.3cm}{\rm Pr}( a =2)= 0.267 - 0.3091^2 \hspace{0.15cm}\underline {= 0.1715}\hspace{0.05cm}.$$
In the calculation, it was assumed that the individual error distances are statistically independent of each other.
- However, this assumption is valid only for a special class of channel models called "renewing".
- The burst error model considered here does not satisfy this condition.
- The actual probability ${\rm Pr}(a = 2) = 0.1675$ therefore deviates slightly from the value calculated here $(0.1715)$.