Aufgaben:Exercise 3.4Z: Continuous Phase Frequency Shift Keying

• Return to book

Signals for  $\text{CP-FSK}$

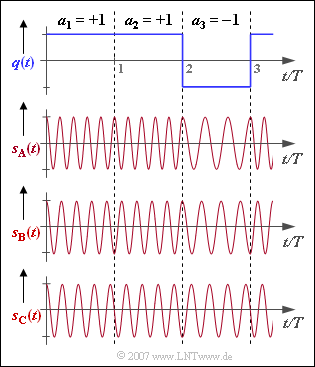
The graph shows three frequency shift keying  $\rm (FSK)$  transmitted signals which differ with respect to the frequency deviation  $\Delta f_{\rm A}$  distinguish and thus also by their modulation index

$$h = 2 \cdot \Delta f_{\rm A} \cdot T.$$

The digital source signal  $q(t)$ underlying the signals  $s_{\rm A}(t),  s_{\rm B}(t)$  and  $s_{\rm C}(t)$  is shown above.  All considered signals are normalized to amplitude  $1$  and time duration  $T$  and based on a cosine carrier with frequency  $f_{\rm T}$.

With binary FSK  $($"Binary Frequency Shift Keying"$)$  only two different frequencies occur,  each of which remains constant over a bit duration:

• $f_{1}$  $($if  $a_{\nu} = +1)$,

• $f_{2}$  $($if  $a_{\nu} = -1)$.

If the modulation index is not a multiple of  $2$,  continuous phase adjustment is required to avoid phase jumps.  This is called  "Continuous Phase Frequency Shift Keying"   $(\text{CP-FSK)}$.

An important special case is represented by binary FSK with modulation index  $h = 0.5$  which is also called  "Minimum Shift Keying"  $(\rm MSK)$.  This will be discussed in this exercise.

Hints:

• This exercise belongs to the chapter  "Radio Interface".

• Reference is made in particular to the section  "Continuous phase adjustment with FSK.

Questions

1

Which statements are true for the FSK and specifically for the MSK?

	FSK is generally a nonlinear modulation method.
	MSK can be implemented as offset QPSK and is therefore linear.
	This results in the same bit error rate as for QPSK.
	A band limitation is less disturbing than with QPSK.
	The MSK envelope is constant even with spectral shaping.

2

What frequencies  $f_{1}$  $($for amplitude coefficient  $a_{\nu} = +1)$  and $f_{2}$  $($for  $a_{\nu} = -1)$  does the signal  $s_{\rm A}(t)$ contain?

$f_{1} \cdot T \ = \ $

$f_{2} \cdot T \ = \ $

3

For the signal  $s_{\rm A}(t)$  what are the carrier frequency  $f_{\rm T}$, frequency deviation  $\delta f_{\rm A}$  and modulation index  $h$?

$f_{\rm T} \cdot T \ = \ $

$\delta f_{\rm A} \cdot T \ = \ $

$h \ = \ $

4

What is the modulation index at signal  $s_{\rm B}(t)$?

$h \ = \ $

5

What is the modulation index at signal  $s_{\rm C}(t)$?

$h \ = \ $

6

Which signals required continuous phase adjustment?

	$s_{\rm A}(t)$,
	$s_{\rm B}(t)$,
	$s_{\rm C}(t)$.

7

What signals describe  Minimum Shift Keying  (MSK)?

	$s_{\rm A}(t)$,
	$s_{\rm B}(t)$,
	$s_{\rm C}(t)$.

Solution

Solution

(1)  All statements except the third are true:

• Generally nonlinear FSK can only be demodulated coherently, while MSK can also use a noncoherent demodulation method.
• Compared to QPSK with coherent demodulation, MSK requires $3 \ \rm dB$ more $E_{\rm B}/N_{0}$ (energy per bit related to the noise power density) for the same bit error rate.
• The first zero in the power density spectrum occurs later in MSK than in QSPK, but it shows a faster asymptotic decay than in QSPK.
• The constant envelope of MSK means that nonlinearities in the transmission line do not play a role. This allows the use of simple and inexpensive power amplifiers with lower power consumption and thus longer operating times of battery-powered devices.

(2)  One can see from the graph five and three oscillations per symbol duration, respectively:

$$f_{\rm 1} \cdot T \hspace{0.15cm} \underline {= 5}\hspace{0.05cm},\hspace{0.2cm}f_{\rm 2} \cdot T \hspace{0.15cm} \underline { = 3}\hspace{0.05cm}.$$

(3)  For FSK with rectangular pulse shape, only the two instantaneous frequencies $f_{1} = f_{\rm T} + \Delta f_{\rm A}$ and $f_{2} = f_{\rm T} - \Delta f_{\rm A}$ occur.

• With the result from (2) we thus obtain:

$$f_{\rm T} \ = \ \frac{f_{\rm 1}+f_{\rm 2}}{2}\hspace{0.3cm}\Rightarrow \hspace{0.3cm}f_{\rm T} \cdot T \hspace{0.15cm} \underline {= 4}\hspace{0.05cm},$$

$$\Delta f_{\rm A} \ = \ \frac{f_{\rm 1}-f_{\rm 2}}{2}\hspace{0.3cm}\Rightarrow \hspace{0.3cm}\Delta f_{\rm A} \cdot T \hspace{0.15cm} \underline { = 1}\hspace{0.05cm},$$

$$h \ = \ 2 \cdot \Delta f_{\rm A} \cdot T \hspace{0.15cm} \underline {= 2} \hspace{0.05cm}.$$

(4)  From the graph one can see the frequencies $f_{1} \cdot T = 4.5$ and $f_{2} \cdot T = 3.5$.

• This results in the frequency deviation $\Delta f_{\rm A} \cdot T = 0.5$ and the modulation index $\underline{h = 1}$.

(5)  Here the two (normalized) frequencies $f_{1} \cdot T = 4.25$ and $f_{2} \cdot T = 3.75$ occur,

• which results in the frequency deviation $\Delta f_{\rm A} \cdot T = 0.25$ and the modulation index $\underline{h = 0.5}$ can be calculated.

(6)  Correct are the solutions 2 and 3:

• Only at $s_{\rm A}(t)$ was no phase adjustment made.
• Here, the signal waveforms in the region of the first and second bit ($a_{1} = a_{2} = +1$) are each cosinusoidal like the carrier signal (with respect to the symbol boundary).
• In contrast, in the second symbol of $s_{\rm B}(t)$ a minus-cosine-shaped course (initial phase $\phi_{0} = π$ corresponding to $180^\circ$) can be seen and in the second symbol of $s_{\rm C}(t)$ a minus-sine-shaped course ($\phi_{0} = π /2$ or $90^\circ$).
• For $s_{\rm A}(t)$ the initial phase is always $0$, for $s_{\rm B}(t)$ either $0$ or $π$, while for the signal $s_{\rm C}(t)$ with modulation index $h = 0.5$ a total of four initial phases are possible: $0^\circ, \ 90^\circ, \ 180^\circ$ and $270^\circ$.

(7)  Correct is the last proposed solution, since for this signal $h = 0.5$ holds.

• This is the smallest possible modulation index for which there is orthogonality between $f_{1}$ and $f_{2}$ within the symbol duration $T$.