Difference between revisions of "Aufgaben:Exercise 1.1Z: ISDN Connection"

From LNTwww
 
(32 intermediate revisions by 4 users not shown)
Line 1: Line 1:
−
{{quiz-Header|Buchseite=Signaldarstellung/Prinzip der Nachrichtenübertragung}}
+
{{quiz-Header|Buchseite=Signal_Representation/Principles_of_communication}}
  
−
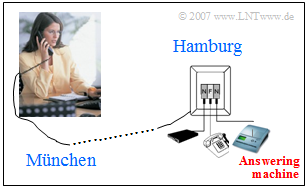
===Zusatzaufgabe zu [[Signaldarstellung/Prinzip_der_Nachrichtenübertragung|Prinzip der Nachrichtenübertragung]]===
+
[[File:EN_Sig_Z_1_1.png|right|frame|A telephone connection scenario]]
 +
We consider the scenario shown in the picture:
  
−
[[File:P_ID340__Sig_Z_1_1.png|right|Szenario einer Telefonverbindung]]
+
A woman from Munich dials a number in Hamburg with her ISDN phone.  However, she cannot reach the person she wants to talk to, so she leaves him a message on tape.
−
Wir betrachten das im Bild dargestellte Szenario: Eine Münchnerin wählt mit ihrem ISDN-Telefon eine Rufnummer in Hamburg. Sie erreicht  jedoch den gewünschten Gesprächspartner  nicht, und  hinterlässt  ihm deshalb eine Nachricht auf Band.
 
  
−
Die  verzerrungsfreie Verbindung wird durch
+
The distortion-free connection is fully described by
 +
* an attenuation coefficient   $\alpha$,
 +
* a term  $\tau$  and
 +
* the current signal-to-noise ratio (SNR).
  
−
:* einen Dämpfungsfaktor $\alpha$,
 
  
−
:* eine Laufzeit $\tau$, und
 
  
−
:* das momentane Signal-zu-Rauschverhältnis (SNR)
 
  
−
vollständig beschrieben.
 
  
−
<b>Hinweis:</b> Die Aufgabe soll einen Bezug zwischen diesem realen Szenario und den in [http://en.lntwww.de/Signaldarstellung/Prinzip_der_Nachrichtenübertragung Prinzip der Nachrichtenübertragung] genannten Funktionseinheiten eines allgemeinen Nachrichtenübertragungssystems herstellen.
 
  
  
−
===Fragebogen===
+
 
 +
 
 +
''Notes:'' &nbsp;The task shall establish a relation between this real scenario and the functional units of a general communications system mentioned in the&nbsp;[[Signal_Representation/Principles_of_Communication#Block_diagram_of_a_communications_system|theory section]]&nbsp;.
 +
 
 +
 
 +
 
 +
 
 +
===Questions===
  
 
<quiz display=simple>
 
<quiz display=simple>
−
{Welche der Aussagen sind bezüglich Quelle und Sender zutreffend?
+
{Which of the statements are true regarding source and transmitter?
 
|type="[]"}
 
|type="[]"}
−
+ Die Nachrichtenquelle ist die Anruferin. Das Quellensignal $\text{q(t)}$ ist die akustische Welle ihres Sprachsignals.
+
+ The message source is the caller.&nbsp; The source signal&nbsp; ${q(t)}$&nbsp; is the acoustic wave of her speech signal.
−
+ Die mit „Sender” bezeichnete Einheit beinhaltet unter anderem einen Signalwandler und einen Modulator.
+
+ The unit labelled&nbsp; "transmitter"&nbsp; contains, among other things, a signal converter and a modulator.
−
- Das Sendesignal $\text{s(t)}$ ist analog.
+
- The transmitted signal&nbsp; $s(t)$&nbsp; is analog.
  
  
−
{Welche der Aussagen treffen bezüglich Empfänger und Sinke zu?
+
{Which of the statements are true regarding receiver and sink?
 
|type="[]"}
 
|type="[]"}
−
- Das Empfangssignal $\text{r(t)}$ ist digital.
+
- The received signal&nbsp; $r(t)$&nbsp; is digital.
−
- Die Nachrichtensinke ist der Telefonapparat in Hamburg.
+
- The message sink is the telephone set in Hamburg.
−
+ Die Nachrichtensinke ist der Anrufbeantworter.
+
+ The message sink is the answering machine.
−
+ Es gilt $\upsilon = \alpha \cdot q(t - \tau ) + \text{n(t)}$.
+
+ The following applies:&nbsp; $v(t) = \alpha \cdot q(t - \tau ) + {n(t)}$.
−
- Es liegt ein ideales Übertragungssystem vor.
+
- There is an ideal transmission system.
  
  
Line 41: Line 46:
 
</quiz>
 
</quiz>
  
−
===Musterlösung===
+
===Solution===
 
{{ML-Kopf}}
 
{{ML-Kopf}}
−
'''1.'''  Die <u>ersten beiden Aussagen</u> sind richtig: Das akustische Sprachsignal $\text{q(t)}$ muss zunächst in ein elektrisches Signal gewandelt und anschließend für die Übertragung aufbereitet werden. Bei ISDN ist das Sendesignal $\text{s(t)}$ digital.
+
'''(1)'''&nbsp; The <u>first two statements</u> are correct:  
 +
*The speech signal&nbsp; ${q(t)}$&nbsp; must first be converted into an electrical signal and then prepared for transmission.  
 +
*For ISDN the transmitted signal&nbsp; ${s(t)}$&nbsp; is digital.
 +
 
 +
 
  
−
'''2.''' Das Empfangssignal $\text{r(t)}$ ist aufgrund des unvermeidbaren thermischen Rauschens stets analog. Die Nachrichtensinke ist der Anrufbeantworter. Bei einem idealen Übertragungssystem müsste $\upsilon = \text{q(t)}$ gelten. Aufgrund des additiven Rauschterms $\text{n(t)}$, der Dämpfung $\alpha$ und der Laufzeit $\tau$ gilt jedoch hier:
+
'''(2)'''&nbsp; Correct are the <u>solutions 3 and 4</u>:
 +
*The received signal&nbsp; ${r(t)}$&nbsp; is always analog due to the unavoidable thermal noise.  
 +
*The message sink is the answering machine.  
 +
*In an ideal transmission system&nbsp; $v(t) = {q(t)}$&nbsp; should apply.
 +
*However,&nbsp; due to the additive noise term&nbsp; ${n(t)}$,&nbsp; the attenuation&nbsp; $\alpha$&nbsp; and the delay time&nbsp; $\tau$&nbsp; the following applies here:
 
:$$v(t) = \alpha \cdot q ( t - \tau) + n(t).$$
 
:$$v(t) = \alpha \cdot q ( t - \tau) + n(t).$$
−
Richtig sind also die <u>Lösungsvorschläge 3 und 4</u>.
+
*By our definitions, this is a distortion-free system.
 +
 
 
{{ML-Fuß}}
 
{{ML-Fuß}}
  
  
−
[[Category:Aufgaben zu Signaldarstellung|^1. Grundbegriffe der Nachrichtentechnik^]]
+
[[Category:Signal Representation: Exercises|^1.1 Principles of Communication^]]

Latest revision as of 16:35, 23 January 2023

A telephone connection scenario

We consider the scenario shown in the picture:

A woman from Munich dials a number in Hamburg with her ISDN phone.  However, she cannot reach the person she wants to talk to, so she leaves him a message on tape.

The distortion-free connection is fully described by

  • an attenuation coefficient   $\alpha$,
  • a term  $\tau$  and
  • the current signal-to-noise ratio (SNR).





Notes:  The task shall establish a relation between this real scenario and the functional units of a general communications system mentioned in the theory section .



Questions

1

Which of the statements are true regarding source and transmitter?

The message source is the caller.  The source signal  ${q(t)}$  is the acoustic wave of her speech signal.
The unit labelled  "transmitter"  contains, among other things, a signal converter and a modulator.
The transmitted signal  $s(t)$  is analog.

2

Which of the statements are true regarding receiver and sink?

The received signal  $r(t)$  is digital.
The message sink is the telephone set in Hamburg.
The message sink is the answering machine.
The following applies:  $v(t) = \alpha \cdot q(t - \tau ) + {n(t)}$.
There is an ideal transmission system.


Solution

Solution

(1)  The first two statements are correct:

  • The speech signal  ${q(t)}$  must first be converted into an electrical signal and then prepared for transmission.
  • For ISDN the transmitted signal  ${s(t)}$  is digital.


(2)  Correct are the solutions 3 and 4:

  • The received signal  ${r(t)}$  is always analog due to the unavoidable thermal noise.
  • The message sink is the answering machine.
  • In an ideal transmission system  $v(t) = {q(t)}$  should apply.
  • However,  due to the additive noise term  ${n(t)}$,  the attenuation  $\alpha$  and the delay time  $\tau$  the following applies here:
$$v(t) = \alpha \cdot q ( t - \tau) + n(t).$$
  • By our definitions, this is a distortion-free system.

Retrieved from "http://en.lntwww.de/index.php?title=Aufgaben:Exercise_1.1Z:_ISDN_Connection&oldid=52096"
  • Powered by MediaWiki