Difference between revisions of "Aufgaben:Exercise 2.15: Block Error Probability with AWGN"

Latest revision as of 17:12, 13 March 2023

Incomplete table of results

Using the example of $\rm RSC \, (7, \, 3, \, 5)_8$ with parameters

$n = 7$ $($number of code symbols$)$,

$k =3$ $($number of information symbols$)$,

$t = 2$ $($correction capability$)$.

the calculation of the block error probability in "Bounded Distance Decoding" $\rm (BDD)$ shall be shown. The corresponding equation is:

$${\rm Pr(block\:error)} = {\rm Pr}(\underline{v} \ne \underline{u}) = \sum_{f = t + 1}^{n} {n \choose f} \cdot {\varepsilon_{\rm S}}^f \cdot (1 - \varepsilon_{\rm S})^{n-f} \hspace{0.05cm}.$$

⇒ The calculation is performed for the "AWGN channel" characterized by the parameter $E_{\rm B}/N_0$:

The quotient $E_{\rm B}/{N_0}$ can be expressed by the relation

$$\varepsilon = {\rm Q} \big (\sqrt{{2 \cdot R \cdot E_{\rm B}}/{N_0}} \big ) $$

into the "BSC model" where $R$ denotes the code rate $($here: $R = 3/7)$ and ${\rm Q}(x)$ indicates the "complementary Gaussian error integral".

But since in the considered code the symbols come from $\rm GF(2^3)$, the BSC model with parameter $\varepsilon$ must also still be adapted to the task.

For the falsification probability of the "m– BSC" model applies:

$$\varepsilon_{\rm S} = 1 - (1 - \varepsilon)^m \hspace{0.05cm}.$$

Here it is to be set $m = 3$ $($three bits per code symbol$)$.

⇒ For some $E_{\rm B}/N_0$ values the results are entered in the table above. The two rows with yellow background are briefly explained here:

For $10 \cdot \lg {E_{\rm B}/N_0} = 4 \ \rm dB$ we get $\varepsilon \approx {\rm Q}(1.47) \approx 0.071$ and $\varepsilon_{\rm S} \approx 0.2$. The block error probability here can most easily be calculated using the complement:

$${\rm Pr(block\:error)} = 1 - \left [ {7 \choose 0} \cdot 0.8^7 + {7 \choose 1} \cdot 0.2 \cdot 0.8^6 + {7 \choose 2} \cdot 0.2^2 \cdot 0.8^5\right ] \approx 0.148 \hspace{0.05cm}.$$

For $10 \cdot \lg {E_{\rm B}/N_0} = 12 \ \rm dB$ one gets $\varepsilon \approx 1.2 \cdot 10^{-4}$ and $\varepsilon_{\rm S} \approx 3.5 \cdot 10^{-4}$. With this very small falsification probability, the $f = 3$ term dominates, and we obtain:

$${\rm Pr(block\:error)} \approx {7 \choose 3} \cdot (3.5 \cdot 10^{-4})^3 \cdot (1- 3.5 \cdot 10^{-4})^4 \approx 1.63 \cdot 10^{-9} \hspace{0.05cm}.$$

⇒ You are to calculate the block error probabilities for the rows highlighted in red $(10 \cdot \lg {E_{\rm B}/N_0} = 5 \ \rm dB, \ 8 \rm dB$, $10 \ \rm dB)$.

The rows with blue background show some results of "Exercise 2.15Z". There ${\rm Pr}(\underline{v} ≠ \underline{u})$ is calculated for $\varepsilon_{\rm S} = 10\%, \ 1\%$ $0.1\%$.
In subtasks (4) and (5) you are to establish the relationship between the quantity $\varepsilon_{\rm S}$ and the AWGN parameter $E_{\rm B}/N_0$ thus completing the above table.

Hints:

The exercise belongs to the chapter "Error Probability and Application Areas".

We refer you here to the two interactive HTML5/JavaScript applets

Questions

${\rm Pr(block\:error)} \ = \ $

$\ \cdot 10^{-2}$

${\rm Pr(block\:error)} \ = \ $

$\ \cdot 10^{-4}$

${\rm Pr(block\:error)} \ = \ $

$\ \cdot 10^{-6}$

$\varepsilon_{\rm S} = 10^{-1} \text{:} \hspace{0.4cm} 10 \cdot \lg {E_{\rm B}/N_0} \ = \ $

$\ \rm dB$

$\varepsilon_{\rm S} = 10^{-2} \text{:} \hspace{0.4cm} 10 \cdot \lg {E_{\rm B}/N_0} \ = \ $

$\ \rm dB$

$\varepsilon_{\rm S} = 10^{-3} \text{:} \hspace{0.4cm} 10 \cdot \lg {E_{\rm B}/N_0} \ = \ $

$\ \rm dB$

Solution

(1) From the table on the information page, the BSC parameter $\varepsilon = 0.0505$ can be read.

This gives $\varepsilon_{\rm S}$ for the symbol error probability with $m = 3$:

$$1 - \varepsilon_{\rm S} = (1 - 0.0505)^3 \approx 0.856 \hspace{0.3cm}\Rightarrow \hspace{0.3cm} \varepsilon_{\rm S} \approx 0.144 \hspace{0.05cm}.$$

The fastest way to calculate the block error probability is here to use the formula

$${\rm Pr(block\:error)} \hspace{-0.15cm} \ = \ \hspace{-0.15cm} 1 - {\rm Pr}(f=0) - {\rm Pr}(f=1) - {\rm Pr}(f=2) = 1 - 1 \cdot 0.856^7 - 7 \cdot 0.144^1 \cdot 0.856^6 - 21 \cdot 0.144^2 \cdot 0.856^5$$

$$\Rightarrow \hspace{0.3cm} {\rm Pr(block\:error)} \hspace{-0.15cm} \ = \ \hspace{-0.15cm} {\rm Pr}(\underline{v} \ne \underline{u}) =1 - 0.3368 - 0.3965 - 0.2001 \hspace{0.15cm} \underline{=0.0666} \hspace{0.05cm}.$$

(2) Following the same calculation procedure as in subtask (1), we obtain with $\varepsilon_{\rm S} \approx 0.03 \ \Rightarrow \ 1 - \varepsilon_{\rm S} = 0.97$:

$${\rm Pr(block\:error)} \hspace{-0.15cm} \ = \ \hspace{-0.15cm} 1 \hspace{-0.05cm}-\hspace{-0.05cm} 1 \cdot 0.97^7 \hspace{-0.05cm}-\hspace{-0.05cm} 7 \cdot 0.03^1 \cdot 0.97^6 \hspace{-0.05cm}-\hspace{-0.05cm} 21 \cdot 0.03^2 \cdot 0.97^5 =1 \hspace{-0.05cm}-\hspace{-0.05cm} 0.8080 \hspace{-0.05cm}-\hspace{-0.05cm} 0.1749\hspace{-0.05cm}-\hspace{-0.05cm} 0.0162= 1 \hspace{-0.05cm}-\hspace{-0.05cm} 0.9991 = 9 \cdot 10^{-4} \hspace{0.05cm}.$$

You can see that here the difference between two numbers of almost the same size must be formed, so that the result could be affected by an error.

Therefore we still calculate the following quantities:

$${\rm Pr}(f=3) \hspace{-0.15cm} \ = \ \hspace{-0.15cm} {7 \choose 3} \cdot \varepsilon_{\rm S}^3 \cdot (1 - \varepsilon_{\rm S})^4 = 35 \cdot 0.03^3 \cdot 0.97^4 = 8.366 \cdot 10^{-4}\hspace{0.05cm},$$

$${\rm Pr}(f=4) \hspace{-0.15cm} \ = \ \hspace{-0.15cm} {7 \choose 4} \cdot \varepsilon_{\rm S}^4 \cdot (1 - \varepsilon_{\rm S})^3 = 35 \cdot 0.03^4 \cdot 0.97^3 = 0.259 \cdot 10^{-4}\hspace{0.05cm},$$

$${\rm Pr}(f=5) \hspace{-0.15cm} \ = \ \hspace{-0.15cm} {7 \choose 5} \cdot \varepsilon_{\rm S}^5 \cdot (1 - \varepsilon_{\rm S})^2 = 21 \cdot 0.03^5 \cdot 0.97^2 = 0.005 \cdot 10^{-4}$$

$$\Rightarrow \hspace{0.3cm} {\rm Pr(block\:error)} = {\rm Pr}(\underline{v} \ne \underline{u}) \approx {\rm Pr}(f=3) + {\rm Pr}(f=4) + {\rm Pr}(f=5) \hspace{0.15cm} \underline{=8.63 \cdot 10^{-4}} \hspace{0.05cm}.$$

The terms for $f = 6$ and $f = 7$ can be omitted here. They do not provide a relevant contribution.

(3) Here $\varepsilon_{\rm S} = 0.005 \ \Rightarrow \ 1 - \varepsilon_{\rm S} = 0.995$ is already given in the table.

The (by far) dominant term in the calculation of the block error probability is ${\rm Pr}(f = 3)$:

$${\rm Pr(block\:error)} = {\rm Pr}(\underline{v} \ne \underline{u}) \approx {\rm Pr}(f=3) = {7 \choose 3} \cdot 0.005^3 \cdot 0.995^4 \hspace{0.15cm} \underline{\approx 4.3 \cdot 10^{-6}} \hspace{0.05cm}.$$

(4) For the BSC parameter $\varepsilon$ holds with $\varepsilon_{\rm S} = 0.1$:

$$\varepsilon = 1 -(1 - \varepsilon_{\rm S})^{1/3} = 1 - 0.9^{1/3} \approx 0.0345 \hspace{0.05cm}.$$

The relation between $\varepsilon$ and $E_{\rm B}/N_0$ is:

$$\varepsilon = {\rm Q}(x)\hspace{0.05cm}, \hspace{0.5cm} x = \sqrt{2 \cdot R \cdot E_{\rm B}/N_0}\hspace{0.05cm}.$$

The inverse $x = {\rm Q}^{-1}(0.0345)$ is obtained with the applet "Complementary Gaussian Error Functions" to $x = 1.82$. This further gives:

$$E_{\rm B}/N_0 = \frac{x^2}{2R} = \frac{1.82^2}{2R \cdot 3/7} \approx 3.864 \hspace{0.3cm} \Rightarrow \hspace{0.3cm} 10 \cdot {\rm lg}\hspace{0.15cm}(E_{\rm B}/N_0) \hspace{0.15cm} \underline{\approx 5.87 \,\, {\rm dB}} \hspace{0.05cm}. $$

(5) After the same calculation one obtains

for $\varepsilon_{\rm S} = 10^{-2} \ \Rightarrow \ \varepsilon \approx 0.33 \cdot 10^{-2} \ \Rightarrow \ x = {\rm Q}^{-1}(\varepsilon) = 2.71$

$$E_{\rm B}/N_0 = \frac{x^2}{2R} = \frac{2.71^2}{2R \cdot 3/7} \approx 8.568 \hspace{0.3cm} \Rightarrow \hspace{0.3cm} 10 \cdot {\rm lg}\hspace{0.15cm}(E_{\rm B}/N_0) \hspace{0.15cm} \underline{\approx 9.32 \,\, {\rm dB}} \hspace{0.05cm}, $$

for $\varepsilon_{\rm S} = 10^{-3} \ \Rightarrow \ \varepsilon \approx 0.33 \cdot 10^{-3} \ \Rightarrow \ x = {\rm Q}^{-1}(\varepsilon) = 3.4$:

$$E_{\rm B}/N_0 = \frac{x^2}{2R} = \frac{3.4^2}{2R \cdot 3/7} \approx 13.487 \hspace{0.3cm} \Rightarrow \hspace{0.3cm} 10 \cdot {\rm lg}\hspace{0.15cm}(E_{\rm B}/N_0) \hspace{0.15cm} \underline{\approx 11.3 \,\, {\rm dB}} \hspace{0.05cm}. $$

Results for $\rm RSC \, (7, \, 3, \, 5)_8$ decoding

The graph shows

the course of the block error probability as function of $10 \cdot \lg {E_{\rm B}/N_0}$,

and the completely filled result table.

One can see the clearly less favorable (asymptotic) behavior of the (green) code $\rm RSC \, (7, \, 5, \, 3)_8$ compared to the (red) comparison code $\rm RSC \, (255, \, 223, \, 33)_8$:

For abscissa values smaller than $10 \ \rm dB$ the result is even worse than without coding.
It should be pointed out again that the $\rm RSC \, (7, \, 3, \, 5)_8$ has only little practical meaning.
It was chosen for this exercise only to be able to demonstrate with reasonable effort the calculation of the block error probability for "Bounded Distance Decoding" $\rm (BDD)$.

@@ Line 1: / Line 1: @@
-{{quiz-Header|Buchseite=Kanalcodierung/Fehlerwahrscheinlichkeit und Anwendungsgebiete}}
+{{quiz-Header|Buchseite=Channel_Coding/Error_Probability_and_Areas_of_Application}}
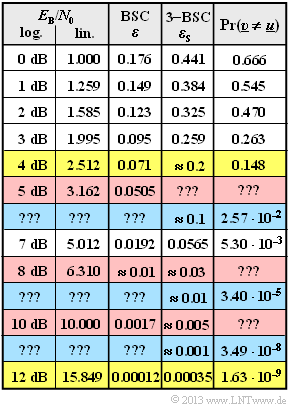
-[[File:P_ID2571__KC_A_2_15neu.png|right|frame|Unvollständige Ergebnistabelle]]
+[[File:P_ID2571__KC_A_2_15neu.png|right|frame|Incomplete table of results]]
-Am Beispiel des $\rm RSC \, (7, \, 3, \, 5)_8$ mit den Parametern
+Using the example of&nbsp; $\rm RSC \, (7, \, 3, \, 5)_8$&nbsp; with parameters
-* $n = 7$ (Anzahl der Codesymbole),
+* $n = 7$&nbsp; $($number of code symbols$)$,
-* $k =3$ (Anzahl der Informationssymbole),
-* $t = 2$ (Korrekturfähigkeit)
+* $k =3$&nbsp; $($number of information symbols$)$,
-soll die Berechnung der Blockfehlerwahrscheinlichkeit beim [[Kanalcodierung/Fehlerwahrscheinlichkeit_und_Anwendungsgebiete#Blockfehlerwahrscheinlichkeit_f.C3.BCr_RSC_und_BDD|Bounded Distance Decoding]] (BDD) gezeigt werden. Die entsprechende Gleichung lautet:
+* $t = 2$&nbsp; $($correction capability$)$.
-:$${\rm Pr(Blockfehler)}  =$$
-:$$ =
+the calculation of the block error probability in&nbsp; [[Channel_Coding/Error_Probability_and_Areas_of_Application#Block_error_probability_for_RSC_and_BDD|"Bounded Distance Decoding"]]&nbsp; $\rm (BDD)$&nbsp; shall be shown.&nbsp; The corresponding equation is:
+:$${\rm Pr(block\:error)}  = {\rm Pr}(\underline{v} \ne \underline{u}) =
 \sum_{f = t + 1}^{n} {n \choose f} \cdot {\varepsilon_{\rm S}}^f \cdot (1 - \varepsilon_{\rm S})^{n-f} \hspace{0.05cm}.$$
-Die Berechnung erfolgt für den [[Kanalcodierung/Klassifizierung_von_Signalen#AWGN.E2.80.93Kanal_bei_bin.C3.A4rem_Eingang|AWGN&ndash;Kanal]], der durch den Parameter $E_{\rm B}/N_0$ gekennzeichnet ist. Dieser Quotient lässt sich über die Beziehung
+&rArr; &nbsp; The calculation is performed for the&nbsp; [[Theory_of_Stochastic_Signals/Gaussian_Distributed_Random_Variables#Exceedance_probability|"AWGN channel"
-:$$\varepsilon = {\rm Q} \big (\sqrt{\frac{2 \cdot R \cdot E_{\rm B}}{N_0}} \big ) $$
+]]&nbsp; characterized by the parameter&nbsp; $E_{\rm B}/N_0$:
-in das [[Kanalcodierung/Klassifizierung_von_Signalen#Binary_Symmetric_Channel_.E2.80.93_BSC|BSC&ndash;Modell]] übeführen, wobei $R$ die Coderate bezeichnet (hier: $R = 3/7$) und ${\rm Q}(x)$ und [[komplementäre Gaußsche Fehlerintegral]] angibt. Da aber beim betrachteten Code die Symbole aus $\rm GF(2^3)$ entstammen, muss das BSC&ndash;Modell mit Parameter $\epsilon$ ebenfalls noch an die Aufgabenstellung adaptiert werden. Für die Verfälschungwahrscheinlichkeit des [[Kanalcodierung/Fehlerwahrscheinlichkeit_und_Anwendungsgebiete#Blockfehlerwahrscheinlichkeit_f.C3.BCr_RSC_und_BDD|m&ndash;BSC&ndash;Modells]] gilt:
+*The quotient&nbsp; $E_{\rm B}/{N_0}$&nbsp; can be expressed by the relation
+:$$\varepsilon = {\rm Q} \big (\sqrt{{2 \cdot R \cdot E_{\rm B}}/{N_0}} \big ) $$
+:into the&nbsp; [[Channel_Coding/Channel_Models_and_Decision_Structures#Binary_Symmetric_Channel_.E2.80. 93_BSC|"BSC model"]]&nbsp; where&nbsp; $R$&nbsp; denotes the code rate&nbsp; $($here: &nbsp;$R = 3/7)$&nbsp; and&nbsp; ${\rm Q}(x)$&nbsp; indicates the&nbsp; [[Theory_of_Stochastic_Signals/Gaussian_Distributed_Random_Variables#Exceedance_probability|"complementary Gaussian error integral"]].
+*But since in the considered code the symbols come from&nbsp; $\rm GF(2^3)$,&nbsp; the BSC model with parameter&nbsp; $\varepsilon$&nbsp; must also still be adapted to the task.
+*For the falsification probability of the&nbsp; [[Channel_Coding/Error_Probability_and_Areas_of_Application#Block_error_probability_for_RSC_and_BDD|"''m''&ndash; BSC"&nbsp; model]]&nbsp; applies:&nbsp;
 :$$\varepsilon_{\rm S} = 1 - (1 - \varepsilon)^m
-\hspace{0.05cm},$$
+\hspace{0.05cm}.$$
+:Here it is to be set&nbsp;  $m = 3$&nbsp; $($three bits per code symbol$)$.
-wobei hier $m = 3$ zu setzen ist (3 Bit pro Codesymbol).
-Für einige $E_{\rm B}/N_0$&ndash;Werte sind alle Ergebnisse bereits in obiger Tabelle eingetragen. Die gelb hinterlegten Zeilen werden hier kurz erläutert.
+&rArr; &nbsp; For some&nbsp; $E_{\rm B}/N_0$ values the results are entered in the table above.&nbsp; The two rows with yellow background are briefly explained here:
-* Für $10 \cdot \lg {E_{\rm B}/N_0} = 4 \ \rm dB$ ergibt sich $\epsilon \approx {\rm Q}(1.47) \approx 0.071$ und $\epsilon_{\rm S} \approx 0.2$. Der einfachste Weg zur Berechnung der Blockfehlerwahrscheinlichkeit führt hier über das Kompliment:
+* For&nbsp; $10 \cdot \lg {E_{\rm B}/N_0} = 4 \ \rm dB$&nbsp; we get&nbsp; $\varepsilon \approx {\rm Q}(1.47) \approx 0.071$&nbsp; and&nbsp; $\varepsilon_{\rm S} \approx 0.2$. &nbsp; The block error probability here can most easily be calculated using the complement:
-:$${\rm Pr(Blockfehler)}  = 1 - \big [ {7 \choose 0} \cdot 0.8^7 + {7 \choose 1} \cdot 0.2 \cdot 0.8^6 + {7 \choose 2} \cdot 0.2^2 \cdot 0.8^5\big ]
+:$${\rm Pr(block\:error)}  = 1 - \left [ {7 \choose 0} \cdot 0.8^7 + {7 \choose 1} \cdot 0.2 \cdot 0.8^6 + {7 \choose 2} \cdot 0.2^2 \cdot 0.8^5\right ]
 \approx 0.148  \hspace{0.05cm}.$$
-* Für $10 \cdot \lg {E_{\rm B}/N_0} = 12 \ \rm dB$ erhält man $\epsilon \approx 1.2 \cdot 10^{-4}$ und $\epsilon_{\rm S} \approx 3.5 \cdot 10^{-4}$. Mit dieser sehr kleinen Verfälschungswahrscheinlichkeit dominiert der $f = 3$&ndash;Term und man erhält
-:$${\rm Pr(Blockfehler)}  \approx  {7 \choose 3} \cdot (3.5 \cdot 10^{-4})^3 \cdot (1- 3.5 \cdot 10^{-4})^4
+* For&nbsp; $10 \cdot \lg {E_{\rm B}/N_0} = 12 \ \rm dB$&nbsp; one gets&nbsp; $\varepsilon \approx 1.2 \cdot 10^{-4}$&nbsp; and&nbsp; $\varepsilon_{\rm S} \approx 3.5 \cdot 10^{-4}$.&nbsp; With this very small falsification probability,&nbsp; the&nbsp; $f = 3$&nbsp; term dominates,&nbsp; and we obtain:
+:$${\rm Pr(block\:error)}  \approx  {7 \choose 3} \cdot (3.5 \cdot 10^{-4})^3 \cdot (1- 3.5 \cdot 10^{-4})^4
 \approx 1.63 \cdot 10^{-9}  \hspace{0.05cm}.$$
-In dieser Aufgabe sollen Sie für die rot hinterlegten Zeilen $(10 \cdot \lg {E_{\rm B}/N_0} = 5 \ \rm dB, \ 8 \ dB$ und $10 \ \rm dB)$ die Blockfehlerwahrscheinlichkeiten berechnen.
+&rArr; &nbsp; You are to calculate the block error probabilities for the rows highlighted in red &nbsp; $(10 \cdot \lg {E_{\rm B}/N_0} = 5 \ \rm dB, \ 8 \rm dB$,&nbsp; $10 \ \rm dB)$.
+:*The rows with blue background show some results of&nbsp; [[Aufgaben:Exercise_2.15Z:_Block_Error_Probability_once_more|"Exercise 2.15Z"]].&nbsp; There&nbsp; ${\rm Pr}(\underline{v} &ne; \underline{u})$&nbsp; is calculated for&nbsp; $\varepsilon_{\rm S} = 10\%,&nbsp; \ 1\%$&nbsp; $0.1\%$.
+:*In subtasks '''(4)''' and '''(5)''' you are to establish the relationship between the quantity&nbsp; $\varepsilon_{\rm S}$&nbsp; and the AWGN parameter&nbsp; $E_{\rm B}/N_0$&nbsp; thus completing the above table.
-Die blau hinterlegten Zeilen zeigen einige Ergebnisse der [[Aufgaben:2.15Z_Nochmals_Pr(%CF%85_%E2%89%A0_u)_f%C3%BCr_BDD|Zusatzaufgabe Z2.15]]. Dort wird ${\rm Pr}(\underline{\upsilon} &ne; \underline{u})$ für $\epsilon_{\rm S} = 10\%, \ 1\%$ und $0.1\%$ berechnet. In den Teilaufgaben (4) und (5) sollen Sie den Zusammenhang zwischen dieser Größe $\epsilon_{\rm S}$ und dem AWGN&ndash;Parameter $E_{\rm B}/N_0$ herstellen und somit die obige Tabelle vervollständigen.
+<u>Hints:</u>
+* The exercise belongs to the chapter&nbsp; [[Channel_Coding/Error_Probability_and_Areas_of_Application| "Error Probability and Application Areas"]].
-''Hinweise:''
+* We refer you here to the two interactive HTML5/JavaScript applets&nbsp;
-* Die Aufgabe gehört zum Kapitel [[Kanalcodierung/Fehlerwahrscheinlichkeit_und_Anwendungsgebiete| Fehlerwahrscheinlichkeit und Anwendungsgebiete]].
+:*[[Applets:Complementary_Gaussian_Error_Functions|"Complementary Gaussian error functions"]],&nbsp; and&nbsp;
-* Wir weisen Sie auf folgende Interaktionsmodule hin:
+:*[[Applets:Binomial_and_Poisson_Distribution_(Applet)|"Binomial and Poisson Distribution"]].
-# [[Komplementäre Gaußsche Fehlerfunktionen]]
-# [[Wahrscheinlichkeiten der Binomialverteilung]]
-* Sollte die Eingabe des Zahlenwertes &bdquo;0&rdquo; erforderlich sein, so geben Sie bitte &bdquo;0.&rdquo; ein.
@@ Line 45: / Line 57: @@
-===Fragebogen===
+===Questions===
 <quiz display=simple>
-{Wie groß ist die Blockfehlerwahrscheinlichkeit für $10 \cdot \lg {E_{\rm B}/N_0} = 5 \ \rm dB$?
+{What is the block error probability for&nbsp;  $10 \cdot \lg {E_{\rm B}/N_0} \hspace{0.15cm}\underline{= 5 \ \rm dB}$?
 |type="{}"}
-$E_{\rm B}/N_0 = 5 \ \rm dB \text{:} \hspace{0.2cm} Pr(Blockfehler) \ = \ ${ 6.66 3% } $\ 10^{-2}
+${\rm Pr(block\:error)} \ = \ ${ 6.66 3% } $\ \cdot 10^{-2}$
-{Wie groß ist die Blockfehlerwahrscheinlichkeit für $10 \cdot \lg {E_{\rm B}/N_0} = 8 \ \rm dB$?
+{What is the block error probability for&nbsp; $10 \cdot \lg {E_{\rm B}/N_0} \hspace{0.15cm}\underline{= 8 \ \rm dB}$?
 |type="{}"}
-$E_{\rm B}/N_0 = 8 \ \rm dB \text{:} \hspace{0.2cm} Pr(Blockfehler) \ = \ ${ 8.63 3% } $\ \cdot 10^{-4}$
+${\rm Pr(block\:error)} \ = \ ${ 8.63 3% } $\ \cdot 10^{-4}$
-{Wie groß ist die Blockfehlerwahrscheinlichkeit für $10 \cdot \lg {E_{\rm B}/N_0} = 10 \ \rm dB$?
+{What is the block error probability for&nbsp; $10 \cdot \lg {E_{\rm B}/N_0}\hspace{0.15cm}\underline{ = 10 \ \rm dB}$?
 |type="{}"}
-$E_{\rm B}/N_0 = 10 \ \rm dB \text{:} \hspace{0.2cm} Pr(Blockfehler) \ = \ ${ 4.3 3% } $\ \cdot 10^{-6}$
+${\rm Pr(block\:error)} \ = \ ${ 4.3 3% } $\ \cdot 10^{-6}$
-{Wie hängt $\epsilon_{\rm S} = 0.1$ mit $10 \cdot \lg {E_{\rm B}/N_0}$ zusammen? ''Hinweis:'' Verwenden Sie das angegebene Flash&ndash;Modul zur Berechnung von ${\rm Q}(x)$.
+{How is&nbsp; $\varepsilon_{\rm S} = 0.1$&nbsp; related to&nbsp; $10 \cdot \lg {E_{\rm B}/N_0}$&nbsp;? &nbsp; <u>Note:</u> &nbsp;Use the given applet to calculate&nbsp; ${\rm Q}(x)$.
 |type="{}"}
-$\epsilon_{\rm S} = 0.1 \text{:} \hspace{0.2cm} 10 \cdot \lg {E_{\rm B}/N_0} \ = \ ${ 5.4 3% } $\ \rm dB$
+$\varepsilon_{\rm S} = 10^{-1} \text{:} \hspace{0.4cm} 10 \cdot \lg {E_{\rm B}/N_0} \ = \ ${ 5.87 3% } $\ \rm dB$
-{Ermitteln Sie auch die $E_{\rm B}/N_0}&ndash;Werte (in $\rm dB$) für $\epsilon_{\rm S} = 0.01$ und $\epsilon_{\rm S} = 0.001$ und vervollständigen Sie die Tabelle.
+{Find also the&nbsp; $E_{\rm B}/N_0$ values&nbsp; $($in&nbsp; $\rm dB)$&nbsp; for&nbsp; $\varepsilon_{\rm S} = 0.01$&nbsp; and&nbsp; $\varepsilon_{\rm S} = 0.001$. Complete the table.
 |type="{}"}
-$\epsilon_{\rm S} = 0.01 \text{:} \hspace{0.2cm} 10 \cdot \lg {E_{\rm B}/N_0} \ = \ ${ 9.32 3% } $\ \rm dB$
+$\varepsilon_{\rm S} = 10^{-2} \text{:} \hspace{0.4cm} 10 \cdot \lg {E_{\rm B}/N_0} \ = \ $ { 9.32 3% } $\ \rm dB$
-$\epsilon_{\rm S} = 0.001 \text{:} \hspace{0.2cm} 10 \cdot \lg {E_{\rm B}/N_0} \ = \ ${ 11.3 3% } $\ \rm dB$
+$\varepsilon_{\rm S} = 10^{-3} \text{:} \hspace{0.4cm} 10 \cdot \lg {E_{\rm B}/N_0} \ = \ $ { 11.3 3% } $\ \rm dB$
 </quiz>
-===Musterlösung===
+===Solution===
 {{ML-Kopf}}
-'''(1)'''&nbsp;
+'''(1)'''&nbsp; From the table on the information page,&nbsp;  the BSC parameter&nbsp; $\varepsilon = 0.0505$&nbsp; can be read.
-'''(2)'''&nbsp;
+*This gives&nbsp; $\varepsilon_{\rm S}$&nbsp; for the symbol error probability with&nbsp; $m = 3$:
-'''(3)'''&nbsp;
+:$$1 - \varepsilon_{\rm S} = (1 - 0.0505)^3 \approx 0.856
-'''(4)'''&nbsp;
+\hspace{0.3cm}\Rightarrow  \hspace{0.3cm}
-'''(5)'''&nbsp;
+\varepsilon_{\rm S} \approx 0.144
-{{ML-Fuß}}
+\hspace{0.05cm}.$$
+*The fastest way to calculate the block error probability is here to use the formula
+:$${\rm Pr(block\:error)}   \hspace{-0.15cm} \ = \ \hspace{-0.15cm} 1 - {\rm Pr}(f=0) -  {\rm Pr}(f=1) - {\rm Pr}(f=2) = 1 - 1 \cdot 0.856^7 -
+\cdot 0.144^1 \cdot 0.856^6 -  21 \cdot 0.144^2 \cdot 0.856^5$$
+:$$\Rightarrow \hspace{0.3cm} {\rm Pr(block\:error)}   \hspace{-0.15cm} \ = \ \hspace{-0.15cm} {\rm Pr}(\underline{v} \ne \underline{u}) =1 - 0.3368 - 0.3965 - 0.2001 \hspace{0.15cm} \underline{=0.0666}
+\hspace{0.05cm}.$$
+'''(2)'''&nbsp; Following the same calculation procedure as in subtask&nbsp; '''(1)''',&nbsp; we obtain with&nbsp; $\varepsilon_{\rm S} \approx 0.03 \ \Rightarrow \ 1 - \varepsilon_{\rm S} = 0.97$:
+:$${\rm Pr(block\:error)}
+\hspace{-0.15cm} \ = \ \hspace{-0.15cm} 1 \hspace{-0.05cm}-\hspace{-0.05cm} 1 \cdot 0.97^7 \hspace{-0.05cm}-\hspace{-0.05cm}
+\cdot 0.03^1 \cdot 0.97^6 \hspace{-0.05cm}-\hspace{-0.05cm}  21 \cdot 0.03^2 \cdot 0.97^5 =1 \hspace{-0.05cm}-\hspace{-0.05cm} 0.8080 \hspace{-0.05cm}-\hspace{-0.05cm} 0.1749\hspace{-0.05cm}-\hspace{-0.05cm} 0.0162= 1 \hspace{-0.05cm}-\hspace{-0.05cm} 0.9991  = 9 \cdot 10^{-4}
+\hspace{0.05cm}.$$
+*You can see that here the difference between two numbers of almost the same size must be formed,&nbsp; so that the result could be affected by an error.
+*Therefore we still calculate the following quantities:
+:$${\rm Pr}(f=3) \hspace{-0.15cm} \ = \ \hspace{-0.15cm}
+{7 \choose 3} \cdot \varepsilon_{\rm S}^3 \cdot (1 - \varepsilon_{\rm S})^4 = 35 \cdot 0.03^3 \cdot 0.97^4 = 8.366 \cdot 10^{-4}\hspace{0.05cm},$$
+:$${\rm Pr}(f=4) \hspace{-0.15cm} \ = \ \hspace{-0.15cm}
+{7 \choose 4} \cdot \varepsilon_{\rm S}^4 \cdot (1 - \varepsilon_{\rm S})^3 = 35 \cdot 0.03^4 \cdot 0.97^3 = 0.259 \cdot 10^{-4}\hspace{0.05cm},$$
+:$${\rm Pr}(f=5) \hspace{-0.15cm} \ = \ \hspace{-0.15cm}
+{7 \choose 5} \cdot \varepsilon_{\rm S}^5 \cdot (1 - \varepsilon_{\rm S})^2 = 21 \cdot 0.03^5 \cdot 0.97^2 = 0.005 \cdot 10^{-4}$$
+:$$\Rightarrow  \hspace{0.3cm} {\rm Pr(block\:error)} = {\rm Pr}(\underline{v} \ne \underline{u})   \approx {\rm Pr}(f=3) +  {\rm Pr}(f=4) + {\rm Pr}(f=5)  \hspace{0.15cm} \underline{=8.63 \cdot 10^{-4}} \hspace{0.05cm}.$$
+*The terms for&nbsp; $f = 6$&nbsp; and&nbsp; $f = 7$&nbsp; can be omitted here.&nbsp; They do not provide a relevant contribution.
+'''(3)'''&nbsp; Here&nbsp; $\varepsilon_{\rm S} = 0.005 \ \Rightarrow \ 1 - \varepsilon_{\rm S} = 0.995$&nbsp; is already given in the table.
+*The&nbsp; (by far)&nbsp; dominant term in the calculation of the block error probability is&nbsp; ${\rm Pr}(f = 3)$:
+:$${\rm Pr(block\:error)} = {\rm Pr}(\underline{v} \ne \underline{u})  \approx {\rm Pr}(f=3) = {7 \choose 3} \cdot 0.005^3 \cdot 0.995^4
+\hspace{0.15cm} \underline{\approx 4.3 \cdot 10^{-6}} \hspace{0.05cm}.$$
+'''(4)'''&nbsp; For the BSC parameter&nbsp; $\varepsilon$&nbsp; holds with&nbsp; $\varepsilon_{\rm S} = 0.1$:
+:$$\varepsilon = 1 -(1 - \varepsilon_{\rm S})^{1/3} = 1 - 0.9^{1/3} \approx 0.0345
+\hspace{0.05cm}.$$
+*The relation between&nbsp; $\varepsilon$&nbsp; and&nbsp; $E_{\rm B}/N_0$&nbsp; is:
+:$$\varepsilon = {\rm Q}(x)\hspace{0.05cm}, \hspace{0.5cm} x = \sqrt{2 \cdot R \cdot E_{\rm B}/N_0}\hspace{0.05cm}.$$
+*The inverse&nbsp; $x = {\rm Q}^{-1}(0.0345)$&nbsp; is obtained with the applet&nbsp; [[Applets:QFunction|"Complementary Gaussian Error Functions"]]&nbsp; to&nbsp; $x = 1.82$.&nbsp; This further gives:
+:$$E_{\rm B}/N_0 = \frac{x^2}{2R} = \frac{1.82^2}{2R \cdot 3/7} \approx 3.864
+\hspace{0.3cm}  \Rightarrow  \hspace{0.3cm}
+\cdot {\rm lg}\hspace{0.15cm}(E_{\rm B}/N_0)
+\hspace{0.15cm} \underline{\approx 5.87 \,\, {\rm dB}} \hspace{0.05cm}. $$
+'''(5)'''&nbsp; After the same calculation one obtains
+* for&nbsp; $\varepsilon_{\rm S} = 10^{-2} \ \Rightarrow \ \varepsilon \approx 0.33 \cdot 10^{-2} \ \Rightarrow \ x = {\rm Q}^{-1}(\varepsilon) = 2.71$
+:$$E_{\rm B}/N_0 = \frac{x^2}{2R} = \frac{2.71^2}{2R \cdot 3/7} \approx 8.568
+\hspace{0.3cm}  \Rightarrow  \hspace{0.3cm}
+\cdot {\rm lg}\hspace{0.15cm}(E_{\rm B}/N_0)
+\hspace{0.15cm} \underline{\approx 9.32 \,\, {\rm dB}} \hspace{0.05cm}, $$
+* for $\varepsilon_{\rm S} = 10^{-3} \ \Rightarrow \ \varepsilon \approx 0.33 \cdot 10^{-3} \ \Rightarrow \ x = {\rm Q}^{-1}(\varepsilon) = 3.4$:
+:$$E_{\rm B}/N_0 = \frac{x^2}{2R} = \frac{3.4^2}{2R \cdot 3/7} \approx 13.487
+\hspace{0.3cm}  \Rightarrow  \hspace{0.3cm}
+\cdot {\rm lg}\hspace{0.15cm}(E_{\rm B}/N_0)
+\hspace{0.15cm} \underline{\approx 11.3 \,\, {\rm dB}} \hspace{0.05cm}. $$
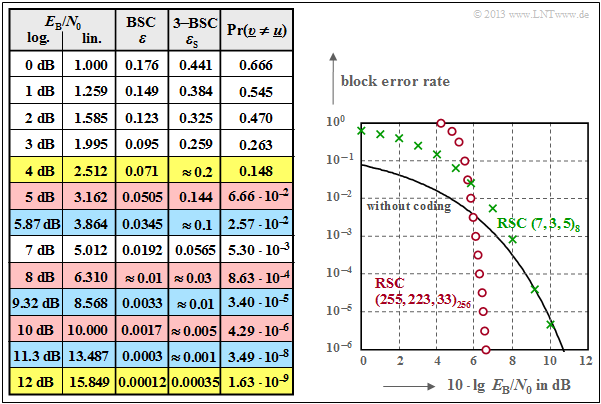
+[[File:EN_KC_A_2_15e.ng.png|right|frame|Results for $\rm RSC \, (7, \, 3, \, 5)_8$ decoding]]
+The graph shows
+*the course of the block error probability as function of $10 \cdot \lg {E_{\rm B}/N_0}$,
+* and the completely filled result table.
+One can see the clearly less favorable&nbsp; (asymptotic)&nbsp; behavior of the&nbsp; (green)&nbsp; code $\rm RSC \, (7, \, 5, \, 3)_8$ compared to the&nbsp; (red)&nbsp; comparison code $\rm RSC \, (255, \, 223, \, 33)_8$:
+# For abscissa values smaller than&nbsp; $10 \ \rm dB$&nbsp; the result is even worse than without coding.
+# It should be pointed out again that the&nbsp; $\rm RSC \, (7, \, 3, \, 5)_8$&nbsp; has only little practical meaning.
+# It was chosen for this exercise only to be able to demonstrate with reasonable effort the calculation of the block error probability for&nbsp; "Bounded Distance Decoding"&nbsp; $\rm (BDD)$.
+{{ML-Fuß}}
-[[Category:Aufgaben zu  Kanalcodierung|^2.6 Fehlerwahrscheinlichkeit und Anwendungsgebiete^]]
+[[Category:Channel Coding: Exercises|^2.6 Block Error Probability of RS Codes^]]