Difference between revisions of "Aufgaben:Exercise 1.1: Music Signals"
From LNTwww
| (26 intermediate revisions by 4 users not shown) | |||
| Line 1: | Line 1: | ||
| − | {{quiz-Header|Buchseite= | + | {{quiz-Header|Buchseite=Signal_Representation/Principles_of_communication}} |
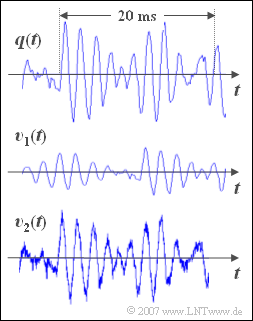
| − | [[File:P_ID339__Sig_A_1_1.png|right|frame| | + | [[File:P_ID339__Sig_A_1_1.png|right|frame|Music signals, <br>original, noisy and/or distorted?]] |
| − | + | On the right you see a $\text{30 ms}$ long section of a music signal <math>q(t)</math>. It is the piece »For Elise« by Ludwig van Beethoven. | |
| − | * | + | *Underneath are drawn two sink signals <math>v_1(t)</math> and <math>v_2(t)</math>, which were recorded after the transmission of the music signal <math>q(t)</math> over two different channels. |
| − | * | + | *The following operating elements allow you to listen to the first fourteen seconds of each of the three audio signals <math>q(t)</math>, <math>v_1(t)</math> and <math>v_2(t)</math>. |
| − | + | Original signal <math>q(t)</math>: | |
<lntmedia>file:A_ID9__Sig_A1_1Elise10sek22kb.mp3</lntmedia> | <lntmedia>file:A_ID9__Sig_A1_1Elise10sek22kb.mp3</lntmedia> | ||
| − | + | Sink signal <math>v_1(t)</math>: | |
<lntmedia>file:A_ID10__Sig_A1_1Elise10sek30Prozent22kb.mp3</lntmedia> | <lntmedia>file:A_ID10__Sig_A1_1Elise10sek30Prozent22kb.mp3</lntmedia> | ||
| − | + | Sink signal <math>v_2(t)</math>: | |
<lntmedia>file:A_ID12__Sig_A1_1elise10sek30dB22kb.mp3</lntmedia> | <lntmedia>file:A_ID12__Sig_A1_1elise10sek30dB22kb.mp3</lntmedia> | ||
| Line 24: | Line 24: | ||
| − | + | <u>Notes:</u> The exercise belongs to the chapter [[Signal_Representation/Principles_of_Communication|»Principles of Communication«]]. | |
| − | |||
| − | === | + | ===Questions=== |
<quiz display=simple> | <quiz display=simple> | ||
| − | { | + | {Estimate the signal frequency of <math>q(t)</math> in the displayed section. |
|type="()"} | |type="()"} | ||
| − | - | + | - The signal frequency is approximately <math>f = 250\,\text{Hz}</math>. |
| − | + | + | + The signal frequency is approximately <math>f = 500\,\text{Hz}</math>. |
| − | - | + | - The signal frequency is approximately <math>f = 1\,\text{kHz}</math>. |
| − | { | + | {Which statements are true for the signal <math>v_1(t)</math> ? |
|type="[]"} | |type="[]"} | ||
| − | + | + | + The signal <math>v_1(t)</math> is undistorted compared to <math>q(t)</math>. |
| − | - | + | - The signal <math>v_1(t)</math> shows distortions compared to <math>q(t)</math> . |
| − | - | + | - The signal <math>v_1(t)</math> is noisy compared to <math>q(t)</math> . |
| − | { | + | {Which statements are true for the signal <math>v_2(t)</math> ? |
|type="[]"} | |type="[]"} | ||
| − | + | + | + The signal <math>v_2(t)</math> is undistorted compared to <math>q(t)</math> . |
| − | - | + | - The signal <math>v_2(t)</math> shows distortions compared to <math>q(t)</math> . |
| − | + | + | + The signal <math>v_2(t)</math> is noisy compared to <math>q(t)</math> . |
| − | { | + | {One of the signals is undistorted and not noisy compared to the original <math>q(t)</math> . <br> Estimate the attenuation factor and the delay time for this. |
|type="{}"} | |type="{}"} | ||
<math> \alpha \ = \ </math> { 0.2-0.4 } | <math> \alpha \ = \ </math> { 0.2-0.4 } | ||
| Line 59: | Line 58: | ||
</quiz> | </quiz> | ||
| − | === | + | ===Solution=== |
{{ML-Kopf}} | {{ML-Kopf}} | ||
| − | '''(1)''' | + | '''(1)''' Correct is <u>solution 2</u>: |
| − | * | + | *In the marked range of $20$ milliseconds ⇒ approx. $10$ oscillations can be detected. |
| − | * | + | |
| + | *From this the result follows approximately for the signal frequency: $f = {10}/(20 \,\text{ms}) = 500 \,\text{Hz}$. | ||
| + | |||
| + | |||
| + | '''(2)''' Correct is <u>solution 1</u>: | ||
| + | *The signal <math>v_1(t)</math> is undistorted compared to the original signal <math>q(t)</math>. The following applies: $v_1(t)=\alpha \cdot q(t-\tau)$. | ||
| − | + | *An attenuation <math>\alpha</math> and a delay time <math>\tau</math> do not cause distortion, but the signal is then only quieter and delayed in time, compared to the original. | |
| − | |||
| − | |||
| − | '''(3)''' | + | '''(3)''' Correct are the <u>solutions 1 and 3</u>: |
| − | * | + | *One can recognize additive noise both in the displayed signal <math>v_2(t)</math> and in the audio signal ⇒ <u>solution 3</u>. |
| − | * | + | |
| − | * | + | *The signal-to-noise ratio is approx. $\text{30 dB}$ $($but this cannot be seen from the mentioned data$)$. |
| + | |||
| + | *Correct is also <u>solution 1</u>: Without this noise component <math>v_2(t)</math> would be identical with <math>q(t)</math>. | ||
| − | '''(4)''' | + | '''(4)''' The signal <math>v_1(t)</math> is identical in shape to the original signal <math>q(t)</math> and differs from it only |
| − | * | + | *by the attenuation factor $\alpha = \underline{\text{0.3}}$ $($this corresponds to about $\text{–10 dB)}$, |
| − | * | + | |
| + | *and the delay time $\tau = \underline{10\,\text{ms}}$. | ||
{{ML-Fuß}} | {{ML-Fuß}} | ||
__NOEDITSECTION__ | __NOEDITSECTION__ | ||
| − | [[Category: | + | [[Category:Signal Representation: Exercises|^1.1 Principles of Communication^]] |
Latest revision as of 15:29, 12 January 2024
On the right you see a $\text{30 ms}$ long section of a music signal \(q(t)\). It is the piece »For Elise« by Ludwig van Beethoven.
- Underneath are drawn two sink signals \(v_1(t)\) and \(v_2(t)\), which were recorded after the transmission of the music signal \(q(t)\) over two different channels.
- The following operating elements allow you to listen to the first fourteen seconds of each of the three audio signals \(q(t)\), \(v_1(t)\) and \(v_2(t)\).
Original signal \(q(t)\):
Sink signal \(v_1(t)\):
Sink signal \(v_2(t)\):
Notes: The exercise belongs to the chapter »Principles of Communication«.
Questions
Solution
(1) Correct is solution 2:
- In the marked range of $20$ milliseconds ⇒ approx. $10$ oscillations can be detected.
- From this the result follows approximately for the signal frequency: $f = {10}/(20 \,\text{ms}) = 500 \,\text{Hz}$.
(2) Correct is solution 1:
- The signal \(v_1(t)\) is undistorted compared to the original signal \(q(t)\). The following applies: $v_1(t)=\alpha \cdot q(t-\tau)$.
- An attenuation \(\alpha\) and a delay time \(\tau\) do not cause distortion, but the signal is then only quieter and delayed in time, compared to the original.
(3) Correct are the solutions 1 and 3:
- One can recognize additive noise both in the displayed signal \(v_2(t)\) and in the audio signal ⇒ solution 3.
- The signal-to-noise ratio is approx. $\text{30 dB}$ $($but this cannot be seen from the mentioned data$)$.
- Correct is also solution 1: Without this noise component \(v_2(t)\) would be identical with \(q(t)\).
(4) The signal \(v_1(t)\) is identical in shape to the original signal \(q(t)\) and differs from it only
- by the attenuation factor $\alpha = \underline{\text{0.3}}$ $($this corresponds to about $\text{–10 dB)}$,
- and the delay time $\tau = \underline{10\,\text{ms}}$.