Difference between revisions of "Aufgaben:Exercise 1.4: Nyquist Criteria"
| (20 intermediate revisions by 5 users not shown) | |||
| Line 1: | Line 1: | ||
| − | {{quiz-Header|Buchseite= | + | {{quiz-Header|Buchseite=Digital_Signal_Transmission/Properties_of_Nyquist_Systems |
}} | }} | ||
| − | [[File:P_ID1278__Dig_A_1_4.png|right|frame| | + | [[File:P_ID1278__Dig_A_1_4.png|right|frame|Rectangular Nyquist spectrum]] |
| − | + | Given by the sketch is the spectrum G(f) of the basic detection pulse, where the parameter A is still to be determined. Among other things it is to be checked whether this basic detection pulse fulfills one of the two Nyquist criteria. These are: | |
| − | * | + | *The '''first Nyquist criterion''' is fulfilled if for the spectral function holds: |
:$$\sum_{k = -\infty}^{+\infty} G(f - | :$$\sum_{k = -\infty}^{+\infty} G(f - | ||
| − | + | {k}/{T} ) = {\rm const.}$$ | |
| − | In | + | :In this case, the pulse $g(t)$ has zero crossings at $t = ν \cdot T$ for all integer values of ν except $ν = 0$. For the entire exercise, $T = 0.1 \, \rm ms$ is assumed. |
| − | * | + | *If the '''second Nyquist criterion''' is satisfied, $g(t)$ has zero crossings at ±1.5T, ±2.5T, etc. |
| − | |||
| − | + | ||
| + | |||
| + | Notes: | ||
| + | *The exercise belongs to the chapter [[Digital_Signal_Transmission/Properties_of_Nyquist_Systems|"Properties of Nyquist Systems"]]. | ||
| + | |||
| + | *The two equations are assumed to be known: | ||
:$$X(f) = \left\{ A0 \right.\quad | :$$X(f) = \left\{ A0 \right.\quad | ||
| − | \begin{array}{*{1}c} {\rm{ | + | \begin{array}{*{1}c} {\rm{for}}\hspace{0.15cm}|f| < f_0 \hspace{0.05cm}, |
| − | \\ {\rm{ | + | \\ {\rm{for}}\hspace{0.15cm}|f| > f_0 \hspace{0.08cm} \\ |
\end{array} \hspace{0.4cm}\bullet\!\!-\!\!\!-\!\!\!-\!\!\circ \hspace{0.4cm} x(t) | \end{array} \hspace{0.4cm}\bullet\!\!-\!\!\!-\!\!\!-\!\!\circ \hspace{0.4cm} x(t) | ||
| − | =2 \cdot A \cdot f_0 \cdot {\rm si}(2 \pi | + | =2 \cdot A \cdot f_0 \cdot {\rm si}(2 \pi f_0 T) \hspace{0.05cm},\hspace{0.4cm} {\rm si} (x) = \sin(x)/x\hspace{0.05cm},$$ |
| − | :$$\sin(\alpha) \cdot \cos (\beta) = \frac{1}{2} \cdot \ | + | :$$\sin(\alpha) \cdot \cos (\beta) = \frac{1}{2} \cdot \big[ \sin(\alpha - \beta) + \sin(\alpha + \beta)\big] |
\hspace{0.05cm}.$$ | \hspace{0.05cm}.$$ | ||
| − | === | + | |
| + | |||
| + | ===Questions=== | ||
<quiz display=simple> | <quiz display=simple> | ||
| − | { | + | {Does the given pulse g(t) satisfy the first Nyquist criterion? |
| − | |type=" | + | |type="()"} |
| − | + | + | +The first Nyquist criterion is fulfilled. |
| − | - | + | -The first Nyquist criterion is not fulfilled. |
| − | { | + | {Determine the parameter A such that $g(t = 0) = 2\, \rm V$ holds. |
|type="{}"} | |type="{}"} | ||
| − | A | + | $A \ = \ $ { 0.2 3% } $ \ \rm mV/Hz$ |
| + | |||
| + | {Calculate g(t) from G(f) by applying the inverse Fourier transform. What (normalized) function value is obtained at t=T? | ||
| + | |type="{}"} | ||
| + | $ g(t = T)/g(t = 0) \ = \ $ { 0. } | ||
| + | |||
| + | {Which (normalized) value results for t=2.5T? | ||
| + | |type="{}"} | ||
| + | $g(t = 2.5 T)/g(t = 0)\ = \ $ { -0.39346--0.37054 } | ||
| + | |||
| + | {Does the pulse $g(t)$ satisfy the second Nyquist criterion? | ||
| + | |type="()"} | ||
| + | -The second Nyquist criterion is fulfilled. | ||
| + | +The second Nyquist criterion is not fulfilled. | ||
| Line 41: | Line 60: | ||
</quiz> | </quiz> | ||
| − | === | + | ===Solution=== |
{{ML-Kopf}} | {{ML-Kopf}} | ||
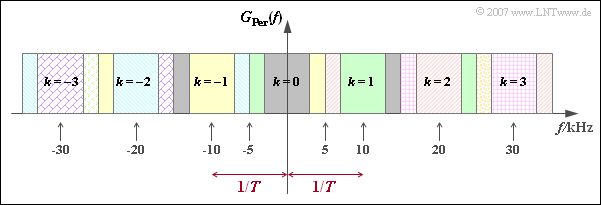
| − | '''(1)''' | + | '''(1)''' The following diagram shows the spectrum (the index "Per" here stands for "Periodic Continuation"): |
| − | '''(2)''' | + | [[File:P_ID1280__Dig_A_1_4a.png|right|frame|Illustration of the first Nyquist criterion]] |
| − | '''(3)''' | + | |
| − | '''(4)''' | + | :$$G_{\rm Per}(f) = \sum_{k = -\infty}^{+\infty} G(f - |
| − | '''(5)''' | + | \frac{k}{T} ) \hspace{0.05cm}.$$ |
| − | '''( | + | *The indexing variable k=0 indicates the original spectral function G(f). This is filled in gray. |
| + | *The spectrum shifted to the right by the value 1/T=10kHz belongs to k=1 and is marked in green, while k=−1 leads to the function highlighted in yellow. | ||
| + | *The red and blue areas mark the contributions of the indexing variables k=2 and k=−2. | ||
| + | |||
| + | |||
| + | It can be seen that GPer(f) is constant. From this it follows that the first Nyquist criterion is fulfilled ⇒ <u>solution 1</u> is correct. | ||
| + | |||
| + | |||
| + | '''(2)''' Due to the Fourier integrals the following relation holds: | ||
| + | :$$g(t=0) = \int_{-\infty}^{\infty}G(f) \,{\rm d} f | ||
| + | = A \cdot ( 2\,{\rm kHz}+6\,{\rm kHz}+2\,{\rm kHz})= A \cdot 10\,{\rm kHz}$$ | ||
| + | :⇒A=g(t=0)10kHz=2V10kHz=0.2mV/Hz_. | ||
| + | |||
| + | |||
| + | '''(3)''' Let g(t)=g1(t)+g2(t), where | ||
| + | *g1(t) contains the spectral components in the interval ±3kHz and | ||
| + | *g2(t) those between 13kHz and 15kHz (and between −13kHz and −15kHz). | ||
| + | |||
| + | |||
| + | With the Fourier correspondence given, the two components are: | ||
| + | :$$g_1(t) \ = \ A \cdot 6\,{\rm kHz} \cdot {\rm si}(\pi \cdot 6\,{\rm kHz} \cdot t) | ||
| + | \hspace{0.05cm},$$ | ||
| + | :$$g_2(t) \ = \ A \cdot 2\,{\rm kHz} | ||
| + | \cdot{\rm si}(\pi \cdot 2\,{\rm kHz} \cdot t) | ||
| + | \cdot 2 \cdot {\rm cos}(2 \pi \cdot 14\,{\rm kHz} \cdot t) | ||
| + | \hspace{0.05cm}.$$ | ||
| + | The second equation follows from the relation: | ||
| + | :$$G_2(f) = \left[ \delta(f + 14\,{\rm kHz}) + \delta(f - 14\,{\rm kHz})\right] \star \left\{ A0 \right.\quad | ||
| + | \begin{array}{*{1}c} {\rm{for}}\hspace{0.15cm}|f| < 1\,{\rm kHz} \hspace{0.05cm}, | ||
| + | \\ {\rm{for}}\hspace{0.15cm}|f| > 1\,{\rm kHz} \hspace{0.05cm}. \\ | ||
| + | \end{array}$$ | ||
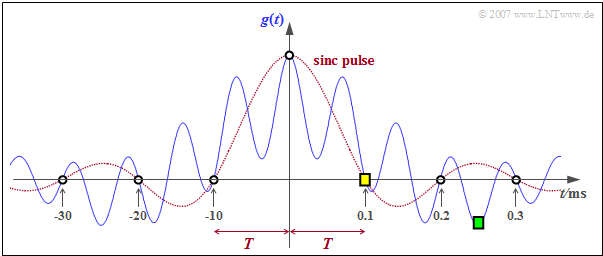
| + | The bottom diagram shows the numerically determined time history g(t). For the time t=T=0.1ms (yellow square) we get: | ||
| + | :$$g_2(t = T ) = 2A \cdot 2\,{\rm kHz} \cdot {\rm si}(0.2 \cdot \pi)\cdot \cos (2.8 \cdot \pi) | ||
| + | = \frac{ A \cdot 4\,{\rm kHz}}{0.2 \cdot \pi}\cdot {\rm sin}(0.2 \cdot \pi | ||
| + | )\cdot\cos (0.8 \cdot \pi) = \frac{ A \cdot 10\,{\rm kHz}}{ \pi}\cdot [{\rm sin}(-0.6 \cdot | ||
| + | \pi)+ {\rm sin}(\pi)] $$ | ||
| + | [[File:EN_Dig_A_1_4.png|right|frame|Higher frequency Nyquist pulse]] | ||
| + | :$$\Rightarrow \hspace{0.3cm} g_2(t = T ) = -\frac{ A \cdot 10\,{\rm kHz}}{ \pi}\cdot {\rm sin}(0.6 \cdot | ||
| + | \pi).$$ | ||
| + | |||
| + | For the first component g1(t), at time t=T: | ||
| + | :$$g_1(t = T ) = A \cdot 6\,{\rm kHz} \cdot {\rm sinc}(0.6 | ||
| + | )$$ | ||
| + | :$$\Rightarrow \hspace{0.3cm}g_1(t = T ) = \frac{ A \cdot 6\,{\rm kHz}}{0.6 \cdot \pi}\cdot {\rm sin}(0.6 \cdot \pi | ||
| + | )$$ | ||
| + | :⇒g1(t=T)=−g2(t=T) | ||
| + | :⇒g(t=T)=g1(t=T)+g2(t=T)=0_. | ||
| + | This result is not surprising because of the Nyquist property. | ||
| + | <br clear=all> | ||
| + | '''(4)''' For t=2.5T (green square), the following partial results are obtained: | ||
| + | :$$g_1(t = 2.5 T ) = A \cdot 6\,{\rm kHz} \cdot {\rm si}(1.5 \cdot \pi | ||
| + | )= \frac{ A \cdot 6\,{\rm kHz}}{1.5 \cdot \pi}\cdot {\rm sin}(1.5 \cdot \pi | ||
| + | )= - \frac{ A \cdot 4\,{\rm kHz}}{ \pi}\hspace{0.05cm},$$ | ||
| + | :$$g_2(t = 2.5 T ) = 2A \cdot 2\,{\rm kHz} \cdot {\rm si}(0.5 \cdot \pi | ||
| + | )\cdot \cos (7 \cdot \pi)=- \frac{ A \cdot 8\,{\rm kHz}}{ \pi} $$ | ||
| + | :⇒g(t=2.5T)=g1(t=2.5T)+g2(t=2.5T)=−A⋅12kHzπ. | ||
| + | Considering g(t=0)=A⋅10 kHz, we get: | ||
| + | :g(t=2.5T)g(t=0)=−1.2π=−0.382_. | ||
| + | |||
| + | |||
| + | '''(5)''' The second Nyquist criterion states that the Nyquist pulse g(t) has zero crossings at ±1.5T,±2.5T,±3.5T,... | ||
| + | *According to the result from '''(4)''' this condition is not fulfilled here. Therefore <u>solution 2</u> is correct. | ||
| + | |||
{{ML-Fuß}} | {{ML-Fuß}} | ||
| Line 54: | Line 135: | ||
| − | [[Category: | + | [[Category:Digital Signal Transmission: Exercises|^1.3 Nyquist System Properties^]] |
Latest revision as of 09:16, 20 May 2022
Given by the sketch is the spectrum G(f) of the basic detection pulse, where the parameter A is still to be determined. Among other things it is to be checked whether this basic detection pulse fulfills one of the two Nyquist criteria. These are:
- The first Nyquist criterion is fulfilled if for the spectral function holds:
- +∞∑k=−∞G(f−k/T)=const.
- In this case, the pulse g(t) has zero crossings at t = ν \cdot T for all integer values of ν except ν = 0. For the entire exercise, T = 0.1 \, \rm ms is assumed.
- If the second Nyquist criterion is satisfied, g(t) has zero crossings at \pm 1.5 T, \pm 2.5 T, etc.
Notes:
- The exercise belongs to the chapter "Properties of Nyquist Systems".
- The two equations are assumed to be known:
- X(f) = \left\{ \begin{array}{c} A \\ 0 \\\end{array} \right.\quad \begin{array}{*{1}c} {\rm{for}}\hspace{0.15cm}|f| < f_0 \hspace{0.05cm}, \\ {\rm{for}}\hspace{0.15cm}|f| > f_0 \hspace{0.08cm} \\ \end{array} \hspace{0.4cm}\bullet\!\!-\!\!\!-\!\!\!-\!\!\circ \hspace{0.4cm} x(t) =2 \cdot A \cdot f_0 \cdot {\rm si}(2 \pi f_0 T) \hspace{0.05cm},\hspace{0.4cm} {\rm si} (x) = \sin(x)/x\hspace{0.05cm},
- \sin(\alpha) \cdot \cos (\beta) = \frac{1}{2} \cdot \big[ \sin(\alpha - \beta) + \sin(\alpha + \beta)\big] \hspace{0.05cm}.
Questions
Solution
- G_{\rm Per}(f) = \sum_{k = -\infty}^{+\infty} G(f - \frac{k}{T} ) \hspace{0.05cm}.
- The indexing variable k = 0 indicates the original spectral function G(f). This is filled in gray.
- The spectrum shifted to the right by the value 1/T = 10\, \rm kHz belongs to k = 1 and is marked in green, while k = -1 leads to the function highlighted in yellow.
- The red and blue areas mark the contributions of the indexing variables k = 2 and k = - 2.
It can be seen that G_{\rm Per}(f) is constant. From this it follows that the first Nyquist criterion is fulfilled ⇒ solution 1 is correct.
(2) Due to the Fourier integrals the following relation holds:
- g(t=0) = \int_{-\infty}^{\infty}G(f) \,{\rm d} f = A \cdot ( 2\,{\rm kHz}+6\,{\rm kHz}+2\,{\rm kHz})= A \cdot 10\,{\rm kHz}
- \Rightarrow \hspace{0.3cm}A = \frac{g(t=0)}{10\,{\rm kHz}} = \frac{2\,{\rm V}}{10\,{\rm kHz}} \hspace{0.1cm}\underline {= 0.2 \, {\rm mV/Hz}} \hspace{0.05cm}.
(3) Let g(t) = g_{1}(t) +g_{2}(t), where
- g_{1}(t) contains the spectral components in the interval \pm 3 \, \rm kHz and
- g_{2}(t) those between 13 \, \rm kHz and 15 \, \rm kHz (and between -13 \, \rm kHz and -15 \, \rm kHz).
With the Fourier correspondence given, the two components are:
- g_1(t) \ = \ A \cdot 6\,{\rm kHz} \cdot {\rm si}(\pi \cdot 6\,{\rm kHz} \cdot t) \hspace{0.05cm},
- g_2(t) \ = \ A \cdot 2\,{\rm kHz} \cdot{\rm si}(\pi \cdot 2\,{\rm kHz} \cdot t) \cdot 2 \cdot {\rm cos}(2 \pi \cdot 14\,{\rm kHz} \cdot t) \hspace{0.05cm}.
The second equation follows from the relation:
- G_2(f) = \left[ \delta(f + 14\,{\rm kHz}) + \delta(f - 14\,{\rm kHz})\right] \star \left\{ \begin{array}{c} A \\ 0 \\\end{array} \right.\quad \begin{array}{*{1}c} {\rm{for}}\hspace{0.15cm}|f| < 1\,{\rm kHz} \hspace{0.05cm}, \\ {\rm{for}}\hspace{0.15cm}|f| > 1\,{\rm kHz} \hspace{0.05cm}. \\ \end{array}
The bottom diagram shows the numerically determined time history g(t). For the time t = T = 0.1\, \rm ms (yellow square) we get:
- g_2(t = T ) = 2A \cdot 2\,{\rm kHz} \cdot {\rm si}(0.2 \cdot \pi)\cdot \cos (2.8 \cdot \pi) = \frac{ A \cdot 4\,{\rm kHz}}{0.2 \cdot \pi}\cdot {\rm sin}(0.2 \cdot \pi )\cdot\cos (0.8 \cdot \pi) = \frac{ A \cdot 10\,{\rm kHz}}{ \pi}\cdot [{\rm sin}(-0.6 \cdot \pi)+ {\rm sin}(\pi)]
- \Rightarrow \hspace{0.3cm} g_2(t = T ) = -\frac{ A \cdot 10\,{\rm kHz}}{ \pi}\cdot {\rm sin}(0.6 \cdot \pi).
For the first component g_1(t), at time t = T:
- g_1(t = T ) = A \cdot 6\,{\rm kHz} \cdot {\rm sinc}(0.6 )
- \Rightarrow \hspace{0.3cm}g_1(t = T ) = \frac{ A \cdot 6\,{\rm kHz}}{0.6 \cdot \pi}\cdot {\rm sin}(0.6 \cdot \pi )
- \Rightarrow \hspace{0.3cm}g_1(t = T ) = - g_2(t = T )
- \Rightarrow \hspace{0.3cm} g(t = T ) = g_1(t = T ) + g_2(t = T )\hspace{0.1cm}\underline {= 0 } \hspace{0.05cm}.
This result is not surprising because of the Nyquist property.
(4) For t = 2.5 T (green square), the following partial results are obtained:
- g_1(t = 2.5 T ) = A \cdot 6\,{\rm kHz} \cdot {\rm si}(1.5 \cdot \pi )= \frac{ A \cdot 6\,{\rm kHz}}{1.5 \cdot \pi}\cdot {\rm sin}(1.5 \cdot \pi )= - \frac{ A \cdot 4\,{\rm kHz}}{ \pi}\hspace{0.05cm},
- g_2(t = 2.5 T ) = 2A \cdot 2\,{\rm kHz} \cdot {\rm si}(0.5 \cdot \pi )\cdot \cos (7 \cdot \pi)=- \frac{ A \cdot 8\,{\rm kHz}}{ \pi}
- \Rightarrow \hspace{0.3cm} g(t = 2.5 T ) = g_1(t = 2.5 T ) +g_2(t = 2.5 T ) = - \frac{ A \cdot 12\,{\rm kHz}}{ \pi} \hspace{0.05cm}.
Considering g(t = 0) = A \cdot 10 \ \rm kHz, we get:
- \frac{g(t = 2.5 T )}{g(t = 0)} = -\frac{ 1.2}{ \pi} \hspace{0.1cm}\underline {= -0.382 } \hspace{0.05cm}.
(5) The second Nyquist criterion states that the Nyquist pulse g(t) has zero crossings at \pm 1.5T, \pm 2.5T, \pm 3.5T, ...
- According to the result from (4) this condition is not fulfilled here. Therefore solution 2 is correct.