Difference between revisions of "Aufgaben:Exercise 1.10Z: Gaussian Band-Pass"
m (Guenter verschob die Seite 1.10Z Gauß-Bandpass nach Aufgabe 1.10Z: Gauß-Bandpass) |
|||
| (12 intermediate revisions by 5 users not shown) | |||
| Line 1: | Line 1: | ||
| − | {{quiz-Header|Buchseite= | + | {{quiz-Header|Buchseite=Digital_Signal_Transmission/Linear_Digital_Modulation_-_Coherent_Demodulation |
}} | }} | ||
| − | [[File:P_ID1697__Dig_Z_4_3.png|right|frame| | + | [[File:P_ID1697__Dig_Z_4_3.png|right|frame|Gaussian band-pass channel]] |
| − | + | For this exercise we assume: | |
| − | * | + | *Binary phase modulation $\rm (BPSK)$ is used for modulation. |
| − | * | + | *Demodulation is synchronous in frequency and phase. |
| − | + | For carrier frequency modulated transmission, the channel frequency response HK(f) must always be assumed to be a band-pass. The channel parameters are e.g. the center frequency fM and the bandwidth ΔfK, where the center frequency (German: "Mittenfrequenz" ⇒ subscipt: "M") fM often coincides with the carrier frequency (German: "Trägerfrequenz" ⇒ subscipt: "T") fT. | |
| − | In | + | In this exercise we will assume a Gaussian band-pass according to the diagram. For its frequency response holds: |
:$$H_{\rm K}(f) = {\rm exp} \left [ - \pi \cdot \left ( \frac {f - f_{\rm M} }{\Delta f_{\rm K}}\right )^2 \right ] | :$$H_{\rm K}(f) = {\rm exp} \left [ - \pi \cdot \left ( \frac {f - f_{\rm M} }{\Delta f_{\rm K}}\right )^2 \right ] | ||
+{\rm exp} \left [ - \pi \cdot \left ( \frac {f + f_{\rm M} }{\Delta f_{\rm K}}\right )^2 \right ]$$ | +{\rm exp} \left [ - \pi \cdot \left ( \frac {f + f_{\rm M} }{\Delta f_{\rm K}}\right )^2 \right ]$$ | ||
| − | + | For a simpler description, one often uses the equivalent low-pass ("TP") frequency response HK,TP(f). This results from HK(f) by | |
| − | * | + | *truncating the components at negative frequencies, |
| − | |||
| − | + | *shifting the spectrum by fT to the left. | |
| + | |||
| + | |||
| + | In the considered example with fT=fM for the equivalent low-pass frequency response results: | ||
:HK,TP(f)=e−π⋅(f/ΔfK)2. | :HK,TP(f)=e−π⋅(f/ΔfK)2. | ||
| − | + | The corresponding time function ("inverse Fourier transform") is: | |
:hK,TP(t)=ΔfK⋅e−π⋅(ΔfK⋅t)2. | :hK,TP(t)=ΔfK⋅e−π⋅(ΔfK⋅t)2. | ||
| − | + | However, the frequency response is also suitable for describing a phase-synchronous BPSK system in the low-pass range | |
:HMKD(f)=1/2⋅[HK(f−fT)+HK(f+fT)], | :HMKD(f)=1/2⋅[HK(f−fT)+HK(f+fT)], | ||
| − | + | where "MKD" stands for "modulator – channel (Kanal) – demodulator". Often - but not always - HMKD(f) and HK,TP(f) are identical. | |
| + | |||
| + | |||
| + | |||
| + | Notes: | ||
| + | *The exercise belongs to the chapter [[Digital_Signal_Transmission/Linear_Digital_Modulation_-_Coherent_Demodulation|"Linear Digital Modulation - Coherent Demodulation"]]. | ||
| − | + | *Reference is made in particular to the section [[Digital_Signal_Transmission/Linear_Digital_Modulation_-_Coherent_Demodulation#Baseband_model_for_ASK_and_BPSK|"Baseband model for ASK and BPSK"]]. | |
| − | * | + | |
| − | |||
| − | |||
| − | === | + | ===Questions=== |
<quiz display=simple> | <quiz display=simple> | ||
| − | { | + | {Give the impulse response hK(t) of the Gaussian band-pass channel. What is the (normalized) value for time t=0? |
|type="{}"} | |type="{}"} | ||
hK(t)/ΔfK = { 2 3% } | hK(t)/ΔfK = { 2 3% } | ||
| − | { | + | {Which statements are valid under the condition fT=fM? |
|type="[]"} | |type="[]"} | ||
| − | -HK,TP(f) | + | -HK,TP(f) and HMKD(f) coincide completely. |
| − | +HK,TP(f) | + | +HK,TP(f) and HMKD(f) are the same for low frequencies. |
| − | + | + | +The time function hK,TP(t) is real. |
| − | + | + | +The time function hMKD(t) is real. |
| − | { | + | {Which statements are true under the condition fT≠fM? |
|type="[]"} | |type="[]"} | ||
| − | -HK,TP(f) | + | -HK,TP(f) and HMKD(f) coincide completely. |
| − | -HK,TP(f) | + | -HK,TP(f) and HMKD(f) are the same for low frequencies. |
| − | - | + | -The time function hK,TP(t) is real. |
| − | + | + | +The time function hMKD(t) is real. |
| − | { | + | {What should be true with respect to a smaller bit error probability? |
| − | |type=" | + | |type="()"} |
+fM=fT, | +fM=fT, | ||
- fM≠fT. | - fM≠fT. | ||
| Line 65: | Line 70: | ||
</quiz> | </quiz> | ||
| − | === | + | ===Solution=== |
{{ML-Kopf}} | {{ML-Kopf}} | ||
| − | '''(1)''' | + | '''(1)''' For the band-pass frequency response HK(f) we can write: |
| − | :$$H_{\rm K}(f) = H_{\rm K,\hspace{0.04cm} TP}(f) \star \ | + | :$$H_{\rm K}(f) = H_{\rm K,\hspace{0.04cm} TP}(f) \star \big [ \delta (f - f_{\rm M}) + \delta (f + f_{\rm M}) \big ] .$$ |
| − | + | *The Fourier inverse transform of the bracket expression yields a cosine function of frequency fM with amplitude 2. | |
| + | |||
| + | *Thus, according to the convolution theorem: | ||
:hK(t)=2⋅ΔfK⋅exp[−π⋅(ΔfK⋅t)2]⋅cos(2πfMt)⇒hK(t=0)/ΔfK=2_. | :hK(t)=2⋅ΔfK⋅exp[−π⋅(ΔfK⋅t)2]⋅cos(2πfMt)⇒hK(t=0)/ΔfK=2_. | ||
| − | + | *This means: The low-pass impulse response hK,TP(t) is identical in shape to the envelope of the band-pass impulse response hK(t), but twice as large. | |
| + | |||
| + | |||
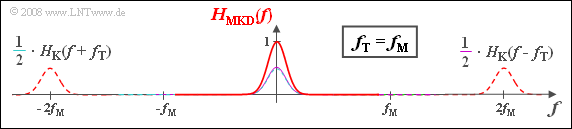
| + | [[File:P_ID1698__Dig_Z_4_3_b.png|right|frame|Resulting baseband frequency response for fT=fM]] | ||
| + | '''(2)''' <u>Statements 2, 3 and 4</u> are correct: | ||
| + | *The first statement is false because HMKD(f) also has components around ±2fT. | ||
| + | |||
| + | *The time function hK,TP(t) is real according to the given equation. | ||
| − | + | *The same is true for hMKD(t) also considering the ±2fT parts, since HMKD(f) is an even function with respect to f=0. | |
| − | + | ||
| − | + | *The diagram shows HMKD(f), which also has components around ±2fT. At low frequencies, HK,TP(f) is identical to HMKD(f). | |
| − | |||
| − | * | ||
| − | |||
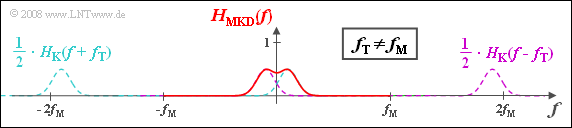
| − | '''(3)''' | + | [[File:P_ID1699__Dig_Z_4_3c.png|right|frame|Resulting baseband frequency response for fT≠fM]] |
| − | * | + | '''(3)''' Only <u>solution 4</u> is correct: |
| − | *HK,TP(f) | + | *Here HK,TP(f) and HMKD(f) differ even at the low frequencies. |
| − | * | + | *HK,TP(f) is a Gaussian function with maximum at $f_{ε} = f_{\rm M} - f_{\rm T}$. |
| − | * | + | *Because of this asymmetry, h_{\rm K,\hspace{0.04cm}TP}(t) is complex. |
| + | *In contrast, H_{\rm MKD}(f) is still an even function with respect to f = 0 with real impulse response h_{\rm MKD}(t). | ||
| + | *H_{\rm MKD}(f) is composed of two Gaussian functions at ± f_ε. | ||
| − | |||
| − | '''(4)''' | + | '''(4)''' Correct is of course the <u>first answer.</u> |
| Line 98: | Line 110: | ||
| − | [[Category: | + | [[Category:Digital Signal Transmission: Exercises|^1.5 Linear Digital Modulation^]] |
Latest revision as of 16:13, 7 May 2022
For this exercise we assume:
- Binary phase modulation \rm (BPSK) is used for modulation.
- Demodulation is synchronous in frequency and phase.
For carrier frequency modulated transmission, the channel frequency response H_{\rm K}(f) must always be assumed to be a band-pass. The channel parameters are e.g. the center frequency f_{\rm M} and the bandwidth \Delta f_{\rm K}, where the center frequency (German: "Mittenfrequenz" ⇒ subscipt: "M") f_{\rm M} often coincides with the carrier frequency (German: "Trägerfrequenz" ⇒ subscipt: "T") f_{\rm T}.
In this exercise we will assume a Gaussian band-pass according to the diagram. For its frequency response holds:
- H_{\rm K}(f) = {\rm exp} \left [ - \pi \cdot \left ( \frac {f - f_{\rm M} }{\Delta f_{\rm K}}\right )^2 \right ] +{\rm exp} \left [ - \pi \cdot \left ( \frac {f + f_{\rm M} }{\Delta f_{\rm K}}\right )^2 \right ]
For a simpler description, one often uses the equivalent low-pass ("TP") frequency response H_{\rm K,TP}(f). This results from H_{\rm K}(f) by
- truncating the components at negative frequencies,
- shifting the spectrum by f_{\rm T} to the left.
In the considered example with f_{\rm T} = f_{\rm M} for the equivalent low-pass frequency response results:
- H_{\rm K,\hspace{0.04cm} TP}(f) = {\rm e}^ { - \pi \hspace{0.04cm}\cdot \hspace{0.04cm}\left ( {f }/{\Delta f_{\rm K}}\right )^2 }.
The corresponding time function ("inverse Fourier transform") is:
- h_{\rm K,\hspace{0.04cm} TP}(t) = \Delta f_{\rm K} \cdot {\rm e}^ { - \pi \hspace{0.04cm}\cdot \hspace{0.04cm}\left ( {\Delta f_{\rm K}} \cdot t \right )^2 }.
However, the frequency response is also suitable for describing a phase-synchronous BPSK system in the low-pass range
- H_{\rm MKD}(f) = {1}/{2} \cdot \left [ H_{\rm K}(f-f_{\rm T}) + H_{\rm K}(f+f_{\rm T})\right ] ,
where "MKD" stands for "modulator – channel (Kanal) – demodulator". Often - but not always - H_{\rm MKD}(f) and H_{\rm K,TP}(f) are identical.
Notes:
- The exercise belongs to the chapter "Linear Digital Modulation - Coherent Demodulation".
- Reference is made in particular to the section "Baseband model for ASK and BPSK".
Questions
Solution
- H_{\rm K}(f) = H_{\rm K,\hspace{0.04cm} TP}(f) \star \big [ \delta (f - f_{\rm M}) + \delta (f + f_{\rm M}) \big ] .
- The Fourier inverse transform of the bracket expression yields a cosine function of frequency f_{\rm M} with amplitude 2.
- Thus, according to the convolution theorem:
- h_{\rm K}(t) = 2 \cdot \Delta f_{\rm K} \cdot {\rm exp} \left [ - \pi \cdot \left ( {\Delta f_{\rm K}} \cdot t \right )^2 \right ] \cdot \cos(2 \pi f_{\rm M} t ) \hspace{0.3cm} \Rightarrow \hspace{0.3cm}h_{\rm K}(t = 0)/\Delta f_{\rm K} \hspace{0.1cm}\underline {= 2}.
- This means: The low-pass impulse response h_{\rm K,\hspace{0.04cm}TP}(t) is identical in shape to the envelope of the band-pass impulse response h_{\rm K}(t), but twice as large.
(2) Statements 2, 3 and 4 are correct:
- The first statement is false because H_{\rm MKD}(f) also has components around \pm 2f_{\rm T}.
- The time function h_{\rm K,\hspace{0.04cm}TP}(t) is real according to the given equation.
- The same is true for h_{\rm MKD}(t) also considering the \pm 2f_{\rm T} parts, since H_{\rm MKD}(f) is an even function with respect to f = 0.
- The diagram shows H_{\rm MKD}(f), which also has components around \pm 2f_{\rm T}. At low frequencies, H_{\rm K,\hspace{0.04cm}TP}(f) is identical to H_{\rm MKD}(f).
(3) Only solution 4 is correct:
- Here H_{\rm K,\hspace{0.04cm}TP}(f) and H_{\rm MKD}(f) differ even at the low frequencies.
- H_{\rm K,\hspace{0.04cm}TP}(f) is a Gaussian function with maximum at f_{ε} = f_{\rm M} - f_{\rm T}.
- Because of this asymmetry, h_{\rm K,\hspace{0.04cm}TP}(t) is complex.
- In contrast, H_{\rm MKD}(f) is still an even function with respect to f = 0 with real impulse response h_{\rm MKD}(t).
- H_{\rm MKD}(f) is composed of two Gaussian functions at ± f_ε.
(4) Correct is of course the first answer.