Difference between revisions of "Aufgaben:Exercise 4.09: Decision Regions at Laplace"
m (Guenter verschob die Seite 4.09 Entscheidungsregionen bei Laplace nach Aufgabe 4.09: Entscheidungsregionen bei Laplace) |
|||
| (11 intermediate revisions by 4 users not shown) | |||
| Line 1: | Line 1: | ||
| − | {{quiz-Header|Buchseite= | + | {{quiz-Header|Buchseite=Digital_Signal_Transmission/Approximation_of_the_Error_Probability}} |
| − | [[File:P_ID2044__Dig_A_4_9.png|right|frame| | + | [[File:P_ID2044__Dig_A_4_9.png|right|frame|Three Laplace decision regions]] |
| − | + | We consider a transmission system based on the basis functions $\varphi_1(t)$ and $\varphi_2(t)$. The two equally probable transmitted signals are given by the signal points | |
:$$\boldsymbol{ s }_0 = (-\sqrt{E}, \hspace{0.1cm}-\sqrt{E})\hspace{0.05cm}, \hspace{0.2cm} | :$$\boldsymbol{ s }_0 = (-\sqrt{E}, \hspace{0.1cm}-\sqrt{E})\hspace{0.05cm}, \hspace{0.2cm} | ||
| − | \boldsymbol{ s }_1 = (+\sqrt{E}, \hspace{0.1cm}+\sqrt{E})\hspace{0.05cm}$$ | + | \boldsymbol{ s }_1 = (+\sqrt{E}, \hspace{0.1cm}+\sqrt{E})\hspace{0.05cm}.$$ |
| − | + | In the following we normalize the energy parameter to $E = 1$ for simplification and thus obtain | |
:$$\boldsymbol{ s }_0 \hspace{-0.1cm} \ = \ \hspace{-0.1cm} (-1, \hspace{0.1cm}-1) \hspace{0.2cm} \Leftrightarrow \hspace{0.2cm} m_0\hspace{0.05cm}, $$ | :$$\boldsymbol{ s }_0 \hspace{-0.1cm} \ = \ \hspace{-0.1cm} (-1, \hspace{0.1cm}-1) \hspace{0.2cm} \Leftrightarrow \hspace{0.2cm} m_0\hspace{0.05cm}, $$ | ||
:$$ \boldsymbol{ s }_1 \hspace{-0.1cm} \ = \ \hspace{-0.1cm} (+1, \hspace{0.1cm}+1)\hspace{0.2cm} \Leftrightarrow \hspace{0.2cm} m_1\hspace{0.05cm}.$$ | :$$ \boldsymbol{ s }_1 \hspace{-0.1cm} \ = \ \hspace{-0.1cm} (+1, \hspace{0.1cm}+1)\hspace{0.2cm} \Leftrightarrow \hspace{0.2cm} m_1\hspace{0.05cm}.$$ | ||
| − | + | The messages $m_0$ and $m_1$ are uniquely assigned to the signals $\boldsymbol{s}_0$ and $\boldsymbol{s}_1$ defined in this way. | |
| − | + | Let the noise components $n_1(t)$ and $n_2(t)$ be independent of each other and each be Laplace distributed with parameter $a = 1$: | |
:$$p_{n_1} (\eta_1) = {1}/{2} \cdot {\rm e}^{- | \eta_1|} \hspace{0.05cm}, \hspace{0.2cm} | :$$p_{n_1} (\eta_1) = {1}/{2} \cdot {\rm e}^{- | \eta_1|} \hspace{0.05cm}, \hspace{0.2cm} | ||
p_{n_2} (\eta_2) = {1}/{2} \cdot {\rm e}^{- | \eta_2|} \hspace{0.05cm} \hspace{0.3cm} | p_{n_2} (\eta_2) = {1}/{2} \cdot {\rm e}^{- | \eta_2|} \hspace{0.05cm} \hspace{0.3cm} | ||
\Rightarrow \hspace{0.3cm} \boldsymbol{ p }_{\boldsymbol{ n }} (\eta_1, \eta_2) = {1}/{4} \cdot {\rm e}^{- | \eta_1|- | \eta_2|} \hspace{0.05cm}. $$ | \Rightarrow \hspace{0.3cm} \boldsymbol{ p }_{\boldsymbol{ n }} (\eta_1, \eta_2) = {1}/{4} \cdot {\rm e}^{- | \eta_1|- | \eta_2|} \hspace{0.05cm}. $$ | ||
| − | + | The properties of such a Laplace noise will be discussed in detail in [[Aufgaben:Exercise_4.09Z:_Laplace_Distributed_Noise|"Exercise 4.9Z"]]. | |
| − | + | The received signal $\boldsymbol{r}$ is composed additively of the transmitted signal $\boldsymbol{s}$ and the noise component $\boldsymbol{n}$: | |
:$$\boldsymbol{ r } \hspace{-0.1cm} \ = \ \hspace{-0.1cm} \boldsymbol{ s } + \boldsymbol{ n } | :$$\boldsymbol{ r } \hspace{-0.1cm} \ = \ \hspace{-0.1cm} \boldsymbol{ s } + \boldsymbol{ n } | ||
\hspace{0.05cm}, \hspace{0.45cm}\boldsymbol{ r } = ( r_1, r_2) | \hspace{0.05cm}, \hspace{0.45cm}\boldsymbol{ r } = ( r_1, r_2) | ||
| − | \hspace{0.05cm}, | + | \hspace{0.05cm},\hspace{0.45cm} |
| − | + | \boldsymbol{ s } \hspace{-0.1cm} \ = \ \hspace{-0.1cm} ( s_1, s_2) | |
| − | \hspace{0.05cm}, \hspace{0. | + | \hspace{0.05cm}, \hspace{0.8cm}\boldsymbol{ n } = ( n_1, n_2) |
\hspace{0.05cm}. $$ | \hspace{0.05cm}. $$ | ||
| − | + | The corresponding realizations are denoted as follows: | |
| − | :$$\boldsymbol{ s }\text{:} \hspace{0.4cm} (s_{01},s_{02}){\hspace{0.15cm}\rm | + | :$$\boldsymbol{ s }\text{:} \hspace{0.4cm} (s_{01},s_{02}){\hspace{0.15cm}\rm and \hspace{0.15cm}} (s_{11},s_{12}) |
| − | \hspace{0.05cm}, | + | \hspace{0.05cm},\hspace{0.8cm} \boldsymbol{ r }\text{:} \hspace{0.4cm} (\rho_{1},\rho_{2}) |
| − | + | \hspace{0.05cm}, \hspace{0.8cm}\boldsymbol{ n }\text{:} \hspace{0.4cm} (\eta_{1},\eta_{2}) | |
| − | \hspace{0.05cm}, \hspace{0. | ||
\hspace{0.05cm}.$$ | \hspace{0.05cm}.$$ | ||
| − | + | The decision rule of the MAP and ML receivers $($both are identical due to the same symbol probabilities$)$ are: | |
| − | + | ⇒ Decide for the symbol $m_0$, if $p_{\boldsymbol{ r} \hspace{0.05cm}|\hspace{0.05cm}m } ( \rho_{1},\rho_{2} |m_0 ) > | |
p_{\boldsymbol{ r} \hspace{0.05cm}|\hspace{0.05cm}m } (\rho_{1},\rho_{2} |m_1 ) \hspace{0.05cm}.$ | p_{\boldsymbol{ r} \hspace{0.05cm}|\hspace{0.05cm}m } (\rho_{1},\rho_{2} |m_1 ) \hspace{0.05cm}.$ | ||
| − | + | ⇒ With the further conditions for the "decision for $m_0$" can also be written: | |
:$${1}/{4} \cdot {\rm exp}\left [- | \rho_1 +1|- | \rho_2 +1| \hspace{0.1cm} \right ] > | :$${1}/{4} \cdot {\rm exp}\left [- | \rho_1 +1|- | \rho_2 +1| \hspace{0.1cm} \right ] > | ||
{1}/{4} \cdot {\rm exp}\left [- | \rho_1 -1|- | \rho_2 -1| \hspace{0.1cm} \right ] $$ | {1}/{4} \cdot {\rm exp}\left [- | \rho_1 -1|- | \rho_2 -1| \hspace{0.1cm} \right ] $$ | ||
| Line 48: | Line 47: | ||
| \rho_1 -1|- | \rho_2 -1| < 0 \hspace{0.05cm}.$$ | | \rho_1 -1|- | \rho_2 -1| < 0 \hspace{0.05cm}.$$ | ||
| − | + | ⇒ This function $L(\rho_1, \rho_2)$ is frequently referred to in the questions. | |
| − | |||
| − | |||
| − | |||
| − | |||
| − | + | The graph shows three different decision regions $(I_0, \ I_1)$. | |
| + | :*For AWGN noise, only the upper variant $\rm A$ would be optimal. | ||
| + | :*Also for the considered Laplace noise, variant $\rm A$ leads to the smallest possible error probability, see [[Aufgaben:Exercise_4.09Z:_Laplace_Distributed_Noise|"Exercise 4.9Z"]]: | ||
| + | ::$$p_{\rm min} = {\rm Pr}({\cal{E}} \hspace{0.05cm}|\hspace{0.05cm} {\rm optimal\hspace{0.15cm} receiver}) = {\rm e}^{-2} \approx 13.5\,\%\hspace{0.05cm}.$$ | ||
| + | :*It is to be examined whether variant $\rm B$ or variant $\rm C$ is also optimal, i.e. whether their error probabilities are also as small as possible equal to $p_{\rm min}$. | ||
| − | |||
| − | |||
| − | |||
| + | Note: The exercise belongs to the chapter [[Digital_Signal_Transmission/Approximation_of_the_Error_Probability|"Approximation of the Error Probability"]]. | ||
| + | |||
| − | === | + | |
| + | ===Questions=== | ||
<quiz display=simple> | <quiz display=simple> | ||
| − | { | + | {Which of the decision rules are correct? Decide for $m_0$, if |
|type="[]"} | |type="[]"} | ||
+ $p_{\it r\hspace{0.03cm}|\hspace{0.03cm}m}(\rho_1, \ \rho_2\hspace{0.03cm}|\hspace{0.03cm}m_0) > p_{\it r\hspace{0.03cm}|\hspace{0.03cm}m}(\rho_1, \ \rho_2\hspace{0.03cm}|\hspace{0.03cm}m_1)$, | + $p_{\it r\hspace{0.03cm}|\hspace{0.03cm}m}(\rho_1, \ \rho_2\hspace{0.03cm}|\hspace{0.03cm}m_0) > p_{\it r\hspace{0.03cm}|\hspace{0.03cm}m}(\rho_1, \ \rho_2\hspace{0.03cm}|\hspace{0.03cm}m_1)$, | ||
| Line 74: | Line 73: | ||
- $L(\rho_1, \ \rho_2) = \rho_1 + \rho_2 ≥ 0$. | - $L(\rho_1, \ \rho_2) = \rho_1 + \rho_2 ≥ 0$. | ||
| − | { | + | {How can the expression $|x+1| \ -|x \ -1|$ be transformed? |
|type="[]"} | |type="[]"} | ||
| − | + | + | + For $x ≥ +1$: $|x + 1| \, -|x -1| = 2$. |
| − | + | + | + For $x ≤ \, -1$: $|x+1| \,-|x \, -1| = \, -2$. |
| − | + | + | + For $-1 ≤ x ≤ +1$: $|x+1| \, -|x \, -1| = 2x$. |
| − | { | + | {What is the decision rule in the range $-1 ≤ \rho_1 ≤ +1$, $-1 ≤ \rho_2 ≤ +1$? |
| − | |type=" | + | |type="()"} |
| − | + | + | + Decision for $m_0$, if $\rho_1 + \rho_2 < 0$. |
| − | - | + | - Decision for $m_1$, if $\rho_1 + \rho_2 < 0$. |
| − | { | + | {What is the decision rule in the range $\rho_1 > +1$? |
| − | |type=" | + | |type="()"} |
| − | - | + | - Decision for $m_0$ in the whole range. |
| − | + | + | + Decision for $m_1$ in the whole range. |
| − | - | + | - Decision for $m_0$ only if $\rho_1 + \rho_2 < 0$. |
| − | { | + | {What is the decision rule in the range $\rho_1 < \, -1$? |
| − | |type=" | + | |type="()"} |
| − | + | + | + Decision for $m_0$ in the whole range. |
| − | - | + | - Decision for $m_1$ in the whole range. |
| − | - | + | - Decision for $m_0$ only if $\rho_1 + \rho_2 < 0$. |
| − | { | + | {What is the decision rule in the range $\rho_2 > +1$? |
| − | |type=" | + | |type="()"} |
| − | - | + | - Decision for $m_0$ in the whole range. |
| − | + | + | + Decision for $m_1$ in the whole range. |
| − | - | + | - Decision for $m_0$ only if $\rho_1 + \rho_2 < 0$. |
| − | { | + | {What is the decision rule in the range $\rho_2 < -1$? |
| − | |type=" | + | |type="()"} |
| − | + | + | + Decision for $m_0$ in the whole range. |
| − | - | + | - Decision for $m_1$ in the whole range. |
| − | - | + | - Decision for $m_0$ only if $\rho_1 + \rho_2 < 0$. |
| − | { | + | {Which of the following statements are true? |
|type="[]"} | |type="[]"} | ||
| − | + | + | + Variant $\rm A$ leads to the minimum error probability. |
| − | + | + | + Variant $\rm B$ leads to the minimum error probability. |
| − | - | + | - Variant $\rm C$ leads to the minimum error probability. |
</quiz> | </quiz> | ||
| − | === | + | ===Solution=== |
{{ML-Kopf}} | {{ML-Kopf}} | ||
| − | '''(1)''' | + | '''(1)''' <u>Solutions 1 and 2</u> are correct: |
| − | * | + | *The joint probability densities under conditions $m_0$ and $m_1$, respectively, are: |
:$$p_{\boldsymbol{ r} \hspace{0.05cm}|\hspace{0.05cm}m } ( \rho_{1},\rho_{2} |m_0 ) | :$$p_{\boldsymbol{ r} \hspace{0.05cm}|\hspace{0.05cm}m } ( \rho_{1},\rho_{2} |m_0 ) | ||
\hspace{-0.1cm} \ = \ \hspace{-0.1cm} {1}/{4} \cdot {\rm exp}\left [- | \rho_1 +1|- | \rho_2 +1| \hspace{0.05cm} \right ]\hspace{0.05cm},$$ | \hspace{-0.1cm} \ = \ \hspace{-0.1cm} {1}/{4} \cdot {\rm exp}\left [- | \rho_1 +1|- | \rho_2 +1| \hspace{0.05cm} \right ]\hspace{0.05cm},$$ | ||
:$$p_{\boldsymbol{ r} \hspace{0.05cm}|\hspace{0.05cm}m } ( \rho_{1},\rho_{2} |m_1 ) | :$$p_{\boldsymbol{ r} \hspace{0.05cm}|\hspace{0.05cm}m } ( \rho_{1},\rho_{2} |m_1 ) | ||
\hspace{-0.1cm} \ = \ \hspace{-0.1cm} {1}/{4} \cdot {\rm exp}\left [- | \rho_1 -1|- | \rho_2 -1| \hspace{0.05cm} \right ]\hspace{0.05cm}.$$ | \hspace{-0.1cm} \ = \ \hspace{-0.1cm} {1}/{4} \cdot {\rm exp}\left [- | \rho_1 -1|- | \rho_2 -1| \hspace{0.05cm} \right ]\hspace{0.05cm}.$$ | ||
| − | * | + | *For equally probable symbols ⇒ ${\rm Pr}(m_0) = {\rm Pr}(m_1) = 0.5$, the MAP decision rule is: Decide for symbol $m_0$ ⇔ signal $s_0$, if |
:$$p_{\boldsymbol{ r} \hspace{0.05cm}|\hspace{0.05cm}m } ( \rho_{1},\rho_{2} |m_0 ) > | :$$p_{\boldsymbol{ r} \hspace{0.05cm}|\hspace{0.05cm}m } ( \rho_{1},\rho_{2} |m_0 ) > | ||
p_{\boldsymbol{ r} \hspace{0.05cm}|\hspace{0.05cm}m } (\rho_{1},\rho_{2} |m_1 ) \hspace{0.05cm}\hspace{0.3cm} | p_{\boldsymbol{ r} \hspace{0.05cm}|\hspace{0.05cm}m } (\rho_{1},\rho_{2} |m_1 ) \hspace{0.05cm}\hspace{0.3cm} | ||
| Line 135: | Line 134: | ||
| − | '''(2)''' <u> | + | '''(2)''' <u>All statements are true</u>: |
| + | *For $x ≥ 1$: | ||
:$$| x +1|- | x -1| = x +1 -x +1 =2 \hspace{0.05cm}.$$ | :$$| x +1|- | x -1| = x +1 -x +1 =2 \hspace{0.05cm}.$$ | ||
| − | + | *Similarly, for $x ≤ \, –1$, e.g. $x = \, –3$: | |
:$$| x +1|- | x -1| = | -3 +1|- | -3 -1| = 2-4 = -2 \hspace{0.05cm}.$$ | :$$| x +1|- | x -1| = | -3 +1|- | -3 -1| = 2-4 = -2 \hspace{0.05cm}.$$ | ||
| − | + | *On the other hand, in the middle range $–1 ≤ x ≤ +1$: | |
:$$| x+1|- | x -1| = x +1 -1 +x =2x \hspace{0.05cm}.$$ | :$$| x+1|- | x -1| = x +1 -1 +x =2x \hspace{0.05cm}.$$ | ||
| − | '''(3)''' | + | '''(3)''' <u>Solution 1</u> is correct: |
| − | * | + | *The result of subtask '''(1)''' was: Decide for the symbol $m_0$, if |
:$$L (\rho_1, \rho_2) = | \rho_1 +1| - | :$$L (\rho_1, \rho_2) = | \rho_1 +1| - | ||
| \rho_1 -1|+ | \rho_2 +1| - | \rho_2 -1| < 0 \hspace{0.05cm}.$$ | | \rho_1 -1|+ | \rho_2 +1| - | \rho_2 -1| < 0 \hspace{0.05cm}.$$ | ||
| − | * | + | *In the considered (inner) range $-1 ≤ \rho_1 ≤ +1$, $-1 ≤ \rho_2 ≤ +1$ holds with the result of subtask '''(2)''': |
:$$| \rho_1+1| - | \rho_1 -1| = 2\rho_1 \hspace{0.05cm}, \hspace{0.2cm} | \rho_2+1| - | \rho_2 -1| = 2\rho_2 \hspace{0.05cm}.$$ | :$$| \rho_1+1| - | \rho_1 -1| = 2\rho_1 \hspace{0.05cm}, \hspace{0.2cm} | \rho_2+1| - | \rho_2 -1| = 2\rho_2 \hspace{0.05cm}.$$ | ||
| − | * | + | *If we insert this result above, we have to decide for $m_0$ exactly if |
:$$L (\rho_1, \rho_2) = 2 \cdot ( \rho_1+\rho_2) < 0 | :$$L (\rho_1, \rho_2) = 2 \cdot ( \rho_1+\rho_2) < 0 | ||
\hspace{0.3cm} \Rightarrow \hspace{0.3cm} \rho_1+\rho_2 < 0\hspace{0.05cm}.$$ | \hspace{0.3cm} \Rightarrow \hspace{0.3cm} \rho_1+\rho_2 < 0\hspace{0.05cm}.$$ | ||
| − | '''(4)''' | + | '''(4)''' Correct is here <u>solution 2</u>: |
| − | * | + | *For $\rho_1 > 1$ ⇒ $|\rho_1+1| \, -|\rho_1 \, -1| = 2$, while for $D_2 = |\rho_2+1| \,-|\rho_2 \, -1|$ ⇒ all values between $-2$ and $+2$ are possible. |
| − | * | + | |
| + | *Thus, the decision variable is $L(\rho_1, \rho_2) = 2 + D_2 ≥ 0$. In this case, the rule leads to an $m_1$ decision. | ||
| − | '''(5)''' | + | '''(5)''' <u>Solution 1</u> is correct: |
| − | * | + | *After similar calculation as in subtask '''(3)''', one arrives at the result: |
:$$L (\rho_1, \rho_2) = -2 + D_2 \le 0 \hspace{0.3cm} \Rightarrow \hspace{0.3cm} | :$$L (\rho_1, \rho_2) = -2 + D_2 \le 0 \hspace{0.3cm} \Rightarrow \hspace{0.3cm} | ||
| − | {\rm | + | {\rm decision\hspace{0.15cm} on\hspace{0.15cm}} m_0\hspace{0.05cm}.$$ |
| − | '''(6)''' | + | '''(6)''' <u>Solution 2</u> is correct: Decision on $m_1$. |
| − | * | + | *Similar to subtask '''(4)''', the following holds here: |
:$$D_1 = | \rho_1 +1| - | \rho_1 -1| \in \{-2, ... \hspace{0.05cm} , +2 \} | :$$D_1 = | \rho_1 +1| - | \rho_1 -1| \in \{-2, ... \hspace{0.05cm} , +2 \} | ||
\hspace{0.3cm} \Rightarrow \hspace{0.3cm}L (\rho_1, \rho_2) = 2 + D_1 \ge 0 | \hspace{0.3cm} \Rightarrow \hspace{0.3cm}L (\rho_1, \rho_2) = 2 + D_1 \ge 0 | ||
| Line 176: | Line 177: | ||
| − | '''(7)''' | + | '''(7)''' <u>Solution 1</u> is correct: Decision on $m_0$. |
| − | * | + | *After similar reasoning as in the last subtask, we arrive at the result: |
:$$L (\rho_1, \rho_2) = -2 + D_1 \le 0 \hspace{0.3cm} \Rightarrow \hspace{0.3cm} | :$$L (\rho_1, \rho_2) = -2 + D_1 \le 0 \hspace{0.3cm} \Rightarrow \hspace{0.3cm} | ||
| − | {\rm | + | {\rm decision\hspace{0.15cm} on\hspace{0.15cm}} m_0\hspace{0.05cm}.$$ |
| + | |||
| + | [[File:P_ID2050__Dig_A_4_9h.png|right|frame|Summary of the results]] | ||
| + | '''(8)''' The results of subtasks '''(3)''' to '''(7)''' are summarized in the graph: | ||
| − | + | * Subarea $T_0$: Decision on $m_0$ or $m_1$ according to task '''(3)'''. | |
| − | + | * Subarea $T_1$: Decision on $m_1$ according to task '''(4)'''. | |
| − | + | * Subarea $T_2$: Decision on $m_0$ according to task '''(5)'''. | |
| − | * | + | * Subarea $T_3$: Decision on $m_1$ according to task '''(6)'''. |
| − | * | + | * Subarea $T_4$: Decision on $m_0$ according to task '''(7)'''. |
| − | * | + | * Subarea $T_5$: Decision on $m_0$ according to task '''(5)''', and on $m_1$ according to task '''(6)''' <br>⇒ For Laplace noise, it does not matter whether one assigns $T_5$ to region $I_0$ or $I_1$. |
| − | * | + | * Subarea $T_6$: Again, based on the results of task '''(6)''' and '''(7)''', one can assign this region to region $I_0$ or region $I_1$. |
| − | * | ||
| − | * | ||
| − | + | It can be seen: | |
| − | + | #For subarea $T_0$, ... , $T_4$ there is a fixed assignment to the decision regions $I_0$ (red) and $I_1$ (blue). | |
| − | + | #In contrast, the two yellow regions $T_5$ and $T_6$ can be assigned to both, $I_0$ and $I_1$ without loss of optimality. | |
| − | + | Comparing this graph with variants $\rm A$, $\rm B$ and $\rm C$ on the specification page, we see that <u>suggestions 1 and 2</u> are correct: | |
| − | + | # Variants $\rm A$ and $\rm B$ are equally good. Both are optimal. The error probability in both cases is $p_{\rm min} = {\rm e}^{\rm -2}$. | |
| − | + | # Variant $\rm C$ is not optimal; with respect to the subareas $T_1$ and $T_2$ there are mismatches. The error probability is therefore greater than $p_{\rm min}$. | |
{{ML-Fuß}} | {{ML-Fuß}} | ||
| − | [[Category: | + | [[Category:Digital Signal Transmission: Exercises|^4.3 BER Approximation^]] |
Latest revision as of 16:49, 29 July 2022
We consider a transmission system based on the basis functions $\varphi_1(t)$ and $\varphi_2(t)$. The two equally probable transmitted signals are given by the signal points
- $$\boldsymbol{ s }_0 = (-\sqrt{E}, \hspace{0.1cm}-\sqrt{E})\hspace{0.05cm}, \hspace{0.2cm} \boldsymbol{ s }_1 = (+\sqrt{E}, \hspace{0.1cm}+\sqrt{E})\hspace{0.05cm}.$$
In the following we normalize the energy parameter to $E = 1$ for simplification and thus obtain
- $$\boldsymbol{ s }_0 \hspace{-0.1cm} \ = \ \hspace{-0.1cm} (-1, \hspace{0.1cm}-1) \hspace{0.2cm} \Leftrightarrow \hspace{0.2cm} m_0\hspace{0.05cm}, $$
- $$ \boldsymbol{ s }_1 \hspace{-0.1cm} \ = \ \hspace{-0.1cm} (+1, \hspace{0.1cm}+1)\hspace{0.2cm} \Leftrightarrow \hspace{0.2cm} m_1\hspace{0.05cm}.$$
The messages $m_0$ and $m_1$ are uniquely assigned to the signals $\boldsymbol{s}_0$ and $\boldsymbol{s}_1$ defined in this way.
Let the noise components $n_1(t)$ and $n_2(t)$ be independent of each other and each be Laplace distributed with parameter $a = 1$:
- $$p_{n_1} (\eta_1) = {1}/{2} \cdot {\rm e}^{- | \eta_1|} \hspace{0.05cm}, \hspace{0.2cm} p_{n_2} (\eta_2) = {1}/{2} \cdot {\rm e}^{- | \eta_2|} \hspace{0.05cm} \hspace{0.3cm} \Rightarrow \hspace{0.3cm} \boldsymbol{ p }_{\boldsymbol{ n }} (\eta_1, \eta_2) = {1}/{4} \cdot {\rm e}^{- | \eta_1|- | \eta_2|} \hspace{0.05cm}. $$
The properties of such a Laplace noise will be discussed in detail in "Exercise 4.9Z".
The received signal $\boldsymbol{r}$ is composed additively of the transmitted signal $\boldsymbol{s}$ and the noise component $\boldsymbol{n}$:
- $$\boldsymbol{ r } \hspace{-0.1cm} \ = \ \hspace{-0.1cm} \boldsymbol{ s } + \boldsymbol{ n } \hspace{0.05cm}, \hspace{0.45cm}\boldsymbol{ r } = ( r_1, r_2) \hspace{0.05cm},\hspace{0.45cm} \boldsymbol{ s } \hspace{-0.1cm} \ = \ \hspace{-0.1cm} ( s_1, s_2) \hspace{0.05cm}, \hspace{0.8cm}\boldsymbol{ n } = ( n_1, n_2) \hspace{0.05cm}. $$
The corresponding realizations are denoted as follows:
- $$\boldsymbol{ s }\text{:} \hspace{0.4cm} (s_{01},s_{02}){\hspace{0.15cm}\rm and \hspace{0.15cm}} (s_{11},s_{12}) \hspace{0.05cm},\hspace{0.8cm} \boldsymbol{ r }\text{:} \hspace{0.4cm} (\rho_{1},\rho_{2}) \hspace{0.05cm}, \hspace{0.8cm}\boldsymbol{ n }\text{:} \hspace{0.4cm} (\eta_{1},\eta_{2}) \hspace{0.05cm}.$$
The decision rule of the MAP and ML receivers $($both are identical due to the same symbol probabilities$)$ are:
⇒ Decide for the symbol $m_0$, if $p_{\boldsymbol{ r} \hspace{0.05cm}|\hspace{0.05cm}m } ( \rho_{1},\rho_{2} |m_0 ) > p_{\boldsymbol{ r} \hspace{0.05cm}|\hspace{0.05cm}m } (\rho_{1},\rho_{2} |m_1 ) \hspace{0.05cm}.$
⇒ With the further conditions for the "decision for $m_0$" can also be written:
- $${1}/{4} \cdot {\rm exp}\left [- | \rho_1 +1|- | \rho_2 +1| \hspace{0.1cm} \right ] > {1}/{4} \cdot {\rm exp}\left [- | \rho_1 -1|- | \rho_2 -1| \hspace{0.1cm} \right ] $$
- $$\Rightarrow \hspace{0.3cm} | \rho_1 +1|+ | \rho_2 +1| < | \rho_1 -1|+ | \rho_2 -1|$$
- $$\Rightarrow \hspace{0.3cm} L (\rho_1, \rho_2) = | \rho_1 +1|+ | \rho_2 +1| - | \rho_1 -1|- | \rho_2 -1| < 0 \hspace{0.05cm}.$$
⇒ This function $L(\rho_1, \rho_2)$ is frequently referred to in the questions.
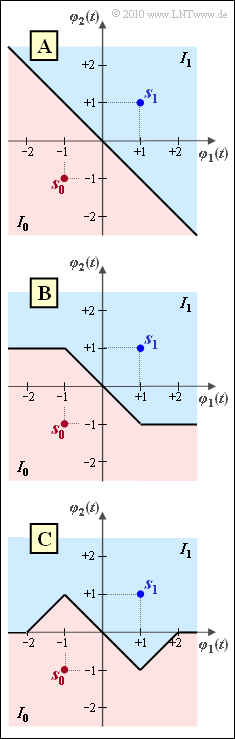
The graph shows three different decision regions $(I_0, \ I_1)$.
- For AWGN noise, only the upper variant $\rm A$ would be optimal.
- Also for the considered Laplace noise, variant $\rm A$ leads to the smallest possible error probability, see "Exercise 4.9Z":
- $$p_{\rm min} = {\rm Pr}({\cal{E}} \hspace{0.05cm}|\hspace{0.05cm} {\rm optimal\hspace{0.15cm} receiver}) = {\rm e}^{-2} \approx 13.5\,\%\hspace{0.05cm}.$$
- It is to be examined whether variant $\rm B$ or variant $\rm C$ is also optimal, i.e. whether their error probabilities are also as small as possible equal to $p_{\rm min}$.
Note: The exercise belongs to the chapter "Approximation of the Error Probability".
Questions
Solution
- The joint probability densities under conditions $m_0$ and $m_1$, respectively, are:
- $$p_{\boldsymbol{ r} \hspace{0.05cm}|\hspace{0.05cm}m } ( \rho_{1},\rho_{2} |m_0 ) \hspace{-0.1cm} \ = \ \hspace{-0.1cm} {1}/{4} \cdot {\rm exp}\left [- | \rho_1 +1|- | \rho_2 +1| \hspace{0.05cm} \right ]\hspace{0.05cm},$$
- $$p_{\boldsymbol{ r} \hspace{0.05cm}|\hspace{0.05cm}m } ( \rho_{1},\rho_{2} |m_1 ) \hspace{-0.1cm} \ = \ \hspace{-0.1cm} {1}/{4} \cdot {\rm exp}\left [- | \rho_1 -1|- | \rho_2 -1| \hspace{0.05cm} \right ]\hspace{0.05cm}.$$
- For equally probable symbols ⇒ ${\rm Pr}(m_0) = {\rm Pr}(m_1) = 0.5$, the MAP decision rule is: Decide for symbol $m_0$ ⇔ signal $s_0$, if
- $$p_{\boldsymbol{ r} \hspace{0.05cm}|\hspace{0.05cm}m } ( \rho_{1},\rho_{2} |m_0 ) > p_{\boldsymbol{ r} \hspace{0.05cm}|\hspace{0.05cm}m } (\rho_{1},\rho_{2} |m_1 ) \hspace{0.05cm}\hspace{0.3cm} \Rightarrow \hspace{0.3cm} {1}/{4} \cdot {\rm exp}\left [- | \rho_1 +1|- | \rho_2 +1| \hspace{0.05cm} \right ] > {1}/{4} \cdot {\rm exp}\left [- | \rho_1 -1|- | \rho_2 -1|\hspace{0.05cm} \right ] $$
- $$\Rightarrow \hspace{0.3cm} | \rho_1 +1|+ | \rho_2 +1| < | \rho_1 -1|+ | \rho_2 -1|\hspace{0.3cm} \Rightarrow \hspace{0.3cm} L (\rho_1, \rho_2) = | \rho_1 +1|- | \rho_1 -1|+ | \rho_2 +1| - | \rho_2 -1| < 0 \hspace{0.05cm}.$$
(2) All statements are true:
- For $x ≥ 1$:
- $$| x +1|- | x -1| = x +1 -x +1 =2 \hspace{0.05cm}.$$
- Similarly, for $x ≤ \, –1$, e.g. $x = \, –3$:
- $$| x +1|- | x -1| = | -3 +1|- | -3 -1| = 2-4 = -2 \hspace{0.05cm}.$$
- On the other hand, in the middle range $–1 ≤ x ≤ +1$:
- $$| x+1|- | x -1| = x +1 -1 +x =2x \hspace{0.05cm}.$$
(3) Solution 1 is correct:
- The result of subtask (1) was: Decide for the symbol $m_0$, if
- $$L (\rho_1, \rho_2) = | \rho_1 +1| - | \rho_1 -1|+ | \rho_2 +1| - | \rho_2 -1| < 0 \hspace{0.05cm}.$$
- In the considered (inner) range $-1 ≤ \rho_1 ≤ +1$, $-1 ≤ \rho_2 ≤ +1$ holds with the result of subtask (2):
- $$| \rho_1+1| - | \rho_1 -1| = 2\rho_1 \hspace{0.05cm}, \hspace{0.2cm} | \rho_2+1| - | \rho_2 -1| = 2\rho_2 \hspace{0.05cm}.$$
- If we insert this result above, we have to decide for $m_0$ exactly if
- $$L (\rho_1, \rho_2) = 2 \cdot ( \rho_1+\rho_2) < 0 \hspace{0.3cm} \Rightarrow \hspace{0.3cm} \rho_1+\rho_2 < 0\hspace{0.05cm}.$$
(4) Correct is here solution 2:
- For $\rho_1 > 1$ ⇒ $|\rho_1+1| \, -|\rho_1 \, -1| = 2$, while for $D_2 = |\rho_2+1| \,-|\rho_2 \, -1|$ ⇒ all values between $-2$ and $+2$ are possible.
- Thus, the decision variable is $L(\rho_1, \rho_2) = 2 + D_2 ≥ 0$. In this case, the rule leads to an $m_1$ decision.
(5) Solution 1 is correct:
- After similar calculation as in subtask (3), one arrives at the result:
- $$L (\rho_1, \rho_2) = -2 + D_2 \le 0 \hspace{0.3cm} \Rightarrow \hspace{0.3cm} {\rm decision\hspace{0.15cm} on\hspace{0.15cm}} m_0\hspace{0.05cm}.$$
(6) Solution 2 is correct: Decision on $m_1$.
- Similar to subtask (4), the following holds here:
- $$D_1 = | \rho_1 +1| - | \rho_1 -1| \in \{-2, ... \hspace{0.05cm} , +2 \} \hspace{0.3cm} \Rightarrow \hspace{0.3cm}L (\rho_1, \rho_2) = 2 + D_1 \ge 0 \hspace{0.05cm}.$$
(7) Solution 1 is correct: Decision on $m_0$.
- After similar reasoning as in the last subtask, we arrive at the result:
- $$L (\rho_1, \rho_2) = -2 + D_1 \le 0 \hspace{0.3cm} \Rightarrow \hspace{0.3cm} {\rm decision\hspace{0.15cm} on\hspace{0.15cm}} m_0\hspace{0.05cm}.$$
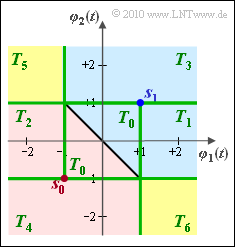
(8) The results of subtasks (3) to (7) are summarized in the graph:
- Subarea $T_0$: Decision on $m_0$ or $m_1$ according to task (3).
- Subarea $T_1$: Decision on $m_1$ according to task (4).
- Subarea $T_2$: Decision on $m_0$ according to task (5).
- Subarea $T_3$: Decision on $m_1$ according to task (6).
- Subarea $T_4$: Decision on $m_0$ according to task (7).
- Subarea $T_5$: Decision on $m_0$ according to task (5), and on $m_1$ according to task (6)
⇒ For Laplace noise, it does not matter whether one assigns $T_5$ to region $I_0$ or $I_1$. - Subarea $T_6$: Again, based on the results of task (6) and (7), one can assign this region to region $I_0$ or region $I_1$.
It can be seen:
- For subarea $T_0$, ... , $T_4$ there is a fixed assignment to the decision regions $I_0$ (red) and $I_1$ (blue).
- In contrast, the two yellow regions $T_5$ and $T_6$ can be assigned to both, $I_0$ and $I_1$ without loss of optimality.
Comparing this graph with variants $\rm A$, $\rm B$ and $\rm C$ on the specification page, we see that suggestions 1 and 2 are correct:
- Variants $\rm A$ and $\rm B$ are equally good. Both are optimal. The error probability in both cases is $p_{\rm min} = {\rm e}^{\rm -2}$.
- Variant $\rm C$ is not optimal; with respect to the subareas $T_1$ and $T_2$ there are mismatches. The error probability is therefore greater than $p_{\rm min}$.