Difference between revisions of "Exercise 2.5Z: Range and Bit Rate with ADSL"
m (Guenter moved page Exercise 2.5Z: Reach and Bit Rate with ADSL to Exercise 2.5Z: Range and Bit Rate with ADSL) |
|||
| (14 intermediate revisions by 5 users not shown) | |||
| Line 1: | Line 1: | ||
| − | {{quiz-Header|Buchseite= | + | {{quiz-Header|Buchseite=Examples_of_Communication_Systems/Methods_to_Reduce_the_Bit_Error_Rate_in_DSL |
}} | }} | ||
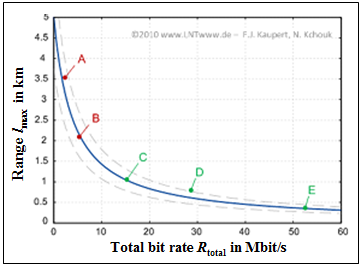
| − | [[File: | + | [[File:EN_Bei_Z_2_5.png|right|frame|"Range vs. bit rate" diagram]] |
| − | + | The development of xDSL technology began in 1995 with the first standard for $\rm ADSL$ (''Asymmetric Digital Subscriber Line''). From 2006, the faster $\rm VDSL$ (''Very High Data Rate Digital Subscriber Line'') also came into use in Germany. | |
| − | + | The graph shows five system variants in a diagram in which the achievable cable length lmax is plotted as a function of the total bit rate Rges : | |
| − | * $\boldsymbol{\rm A}\text{:} \ \text{ Bitraten} \ 0.2 \ {\rm Mbit/s} + 2 \ {\rm Mbit/s}; \ \text{ | + | * $\boldsymbol{\rm A}\text{:} \hspace{0.3cm} \text{ Bitraten} \ 0.2 \ {\rm Mbit/s} + 2 \ {\rm Mbit/s}; \hspace{0.2cm} \text{ cable length } l_{\rm max} \approx 3.5 \ {\rm km},$ |
| − | * $\boldsymbol{\rm B}\text{:} \ \text{ Bitraten} \ 0.2 \ {\rm Mbit/s} + 6 \ {\rm Mbit/s}; \ \text{ | + | * $\boldsymbol{\rm B}\text{:} \hspace{0.3cm} \text{ Bitraten} \ 0.2 \ {\rm Mbit/s} + 6 \ {\rm Mbit/s}; \hspace{0.2cm} \text{ cable length } l_{\rm max} \approx 2 \ {\rm km},$ |
| − | * $\boldsymbol{\rm C}\text{:} \ \text{ Bitraten} \ 2 \ {\rm Mbit/s} + 13 \ {\rm Mbit/s}; \ \text{ | + | * $\boldsymbol{\rm C}\text{:} \hspace{0.3cm} \text{ Bitraten} \ 2 \ {\rm Mbit/s} + 13 \ {\rm Mbit/s}; \hspace{0.3cm} \text{ cable length } l_{\rm max} \approx 1 \ {\rm km},$ |
| − | * $\boldsymbol{\rm D}\text{:} \ \text{ Bitraten} \ 2 \ {\rm Mbit/s} + 26 \ {\rm Mbit/s}; \ \text{ | + | * $\boldsymbol{\rm D}\text{:} \hspace{0.3cm} \text{ Bitraten} \ 2 \ {\rm Mbit/s} + 26 \ {\rm Mbit/s}; \hspace{0.3cm} \text{ cable length } \ l_{\rm max} \approx 0.8 \ {\rm km},$ |
| − | * $\boldsymbol{\rm E}\text{:} \ \text{ Bitraten} \ 2 \ {\rm Mbit/s} + 51 \ {\rm Mbit/s}; \ \text{ | + | * $\boldsymbol{\rm E}\text{:} \hspace{0.3cm}\text{ Bitraten} \ 2 \ {\rm Mbit/s} + 51 \ {\rm Mbit/s}; \hspace{0.35cm} \text{ cable length } l_{\rm max} \approx 0.4 \ {\rm km}.$ |
| + | To this graphic is to be noted further: | ||
| + | *All data applies to a balanced copper pair with 0.4 mm diameter. | ||
| + | *One of the bit rates given here is for upstream, the other is for downstream. | ||
| + | *The total bitrate is the sum of the two portions. | ||
| + | *Which bit rate refers to the upstream and which to the downstream is asked in the subtask '''(1)'''. | ||
| + | *The colored differentiation of the drawn points refers to the subdivision into ADSL and VDSL. This is referred to in the subtask '''(2)'''. | ||
| + | *The curve drawn in blue shows a rule of thumb that approximates the relationship between range and total bit rate: | ||
| + | :lmax[inkm]=204+Rges[inMbit/s]. | ||
| + | *Dashed are deviations from this by ±25%. | ||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | + | One often characterizes a wireline transmission system by the cable attenuation at half the bit rate (note the "a" in the attenuation): | |
| − | |||
:a⋆=aK(f=RB/2)=αK(f=RB/2)⋅l. | :a⋆=aK(f=RB/2)=αK(f=RB/2)⋅l. | ||
| − | + | The attenuation coefficient (noted as "alpha") is given for a $\text{0.4 mm}$ balanced copper pair as follows: | |
:αK(f)=[5.1+14.3⋅(f/(1MHz))0.59]dB/km. | :αK(f)=[5.1+14.3⋅(f/(1MHz))0.59]dB/km. | ||
| − | + | For the downlink of variant \boldsymbol{\rm A} with R_{\rm B} = 2 \ \rm Mbit/s thus results with l = l_{\rm max} = 3.5 \ \rm km: | |
:$$\alpha_{\rm K}(f = {\rm 1\,MHz}) = \left [ 5.1 + 14.3 \right ] {\rm dB}/{\rm km} = 19.4\,{\rm dB}/{\rm km}\hspace{0.3cm} | :$$\alpha_{\rm K}(f = {\rm 1\,MHz}) = \left [ 5.1 + 14.3 \right ] {\rm dB}/{\rm km} = 19.4\,{\rm dB}/{\rm km}\hspace{0.3cm} | ||
\Rightarrow \hspace{0.3cm} {\rm a}_{\rm \star} = 19.4\,{\rm dB}/{\rm km} \cdot 3.5\,{\rm km} = 67.9\,{\rm dB}\hspace{0.05cm}.$$ | \Rightarrow \hspace{0.3cm} {\rm a}_{\rm \star} = 19.4\,{\rm dB}/{\rm km} \cdot 3.5\,{\rm km} = 67.9\,{\rm dB}\hspace{0.05cm}.$$ | ||
| − | + | The values for the other system variants are to be determined in subtask '''(4)'''. | |
| + | |||
| + | |||
| Line 43: | Line 45: | ||
| − | |||
| − | + | Hint: | |
| − | |||
| + | *This exercise belongs to the chapter [[Examples_of_Communication_Systems/Methods_to_Reduce_the_Bit_Error_Rate_in_DSL|"Methods to Reduce the Bit Error Rate in DSL"]]. | ||
| + | |||
| − | === | + | |
| + | ===Questions=== | ||
<quiz display=simple> | <quiz display=simple> | ||
| − | { | + | {For example, consider the system variant \boldsymbol{\rm C}. Which applies? |
| − | |type=" | + | |type="()"} |
| − | - | + | - The upstream bit rate is 13 \ \rm Mbit/s. |
| − | + | + | + The downstream bit rate is 13 \rm Mbit/s. |
| − | { | + | {Which of the drawn variants are ADSL or VDSL systems? |
| − | |type=" | + | |type="()"} |
| − | - | + | - The red dots indicate VDSL systems. |
| − | + | + | + The green dots indicate VDSL systems. |
| − | { | + | {What range would result from the given rule of thumb for R_{\rm ges} = 1 \ \rm Gbit/s. |
|type="{}"} | |type="{}"} | ||
| − | l_{\rm max} \ = \ { 20 3% } \ m | + | l_{\rm max} \ = \ { 20 3% } $ \ \rm m$. |
| − | { | + | {Calculate the characteristic cable attenuations for the variant |
|type="{}"} | |type="{}"} | ||
\boldsymbol{\rm B}\text{:} \hspace{0.4cm} {\rm a}_{\ast} \ = \ { 64.9 3% } \ \rm dB | \boldsymbol{\rm B}\text{:} \hspace{0.4cm} {\rm a}_{\ast} \ = \ { 64.9 3% } \ \rm dB | ||
| Line 78: | Line 81: | ||
</quiz> | </quiz> | ||
| − | === | + | ===Solution=== |
{{ML-Kopf}} | {{ML-Kopf}} | ||
| − | '''(1)''' | + | '''(1)''' Correct is <u>the second proposed solution</u>: |
| − | * | + | *In all xDSL variants, the downstream is operated at a higher bit rate than the upstream. |
| − | * | + | *This principle results from user behavior. One fetches much more data to the computer (downstream) than in the reverse direction. |
| + | |||
| + | |||
| + | |||
| + | '''(2)''' Correct is <u>the second proposed solution</u>: | ||
| + | *VDSL offers higher data rates. | ||
| + | *High data rate, however, is only possible with relatively short line lengths. | ||
| − | |||
| − | |||
| + | '''(3)''' The range of such a Gbit/s system over two-wire line would be about $20/1000 | ||
| + | \ {\rm km} \underline{= 20 \ \rm meters}$. | ||
| + | *Consider this subtask rather academic. | ||
| − | |||
| − | |||
| − | '''(4)''' | + | '''(4)''' Here the following characteristic cable attenuations result. For |
| − | * | + | *variant \boldsymbol{\rm B} \ (R_{\rm B}/2 = 3 {\rm \ Mbit/s}, \ l_{\rm max} = 2 {\rm \ km})\text{:} |
:{\rm a}_{\rm \star} = \left [ 5.1 + 14.3 \cdot 3^{0.59} \right ] \cdot 2\,{\rm dB}\hspace{0.15cm}\underline{ \approx 64.9\,{\rm dB}}\hspace{0.05cm}, | :{\rm a}_{\rm \star} = \left [ 5.1 + 14.3 \cdot 3^{0.59} \right ] \cdot 2\,{\rm dB}\hspace{0.15cm}\underline{ \approx 64.9\,{\rm dB}}\hspace{0.05cm}, | ||
| − | * | + | *variant \boldsymbol{\rm C} \ (R_{\rm B}/2 = 6.5 {\rm \ Mbit/s}, \ l_{\rm max} = 1 {\rm \ km})\text{:} |
:{\rm a}_{\rm \star} = \left [ 5.1 + 14.3 \cdot 6.5^{0.59} \right ] \cdot 1\,{\rm dB} \hspace{0.15cm}\underline{\approx 48.2\,{\rm dB}}\hspace{0.05cm}, | :{\rm a}_{\rm \star} = \left [ 5.1 + 14.3 \cdot 6.5^{0.59} \right ] \cdot 1\,{\rm dB} \hspace{0.15cm}\underline{\approx 48.2\,{\rm dB}}\hspace{0.05cm}, | ||
| − | * | + | *variant \boldsymbol{\rm D} \ (R_{\rm B}/2 = 13 {\rm \ Mbit/s}, \ l_{\rm max} = 0.8 {\rm \ km})\text{:} |
:{\rm a}_{\rm \star} = \left [ 5.1 + 14.3 \cdot 13^{0.59} \right ] \cdot 0.8\,{\rm dB}\hspace{0.15cm}\underline{ \approx 56\,{\rm dB}}\hspace{0.05cm}, | :{\rm a}_{\rm \star} = \left [ 5.1 + 14.3 \cdot 13^{0.59} \right ] \cdot 0.8\,{\rm dB}\hspace{0.15cm}\underline{ \approx 56\,{\rm dB}}\hspace{0.05cm}, | ||
| − | * | + | *variant \boldsymbol{\rm E} \ (R_{\rm B}/2 = 25.5 {\rm \ Mbit/s}, \ l_{\rm max} = 0.4 {\rm \ km})\text{:} |
:{\rm a}_{\rm \star} = \left [ 5.1 + 14.3 \cdot 25.5^{0.59} \right ] \cdot 0.4\,{\rm dB}\hspace{0.15cm}\underline{ \approx 40.7\,{\rm dB}}\hspace{0.05cm}. | :{\rm a}_{\rm \star} = \left [ 5.1 + 14.3 \cdot 25.5^{0.59} \right ] \cdot 0.4\,{\rm dB}\hspace{0.15cm}\underline{ \approx 40.7\,{\rm dB}}\hspace{0.05cm}. | ||
| − | + | Further, it should be noted: | |
| − | * | + | *The characteristic cable attenuation {\rm a}_{\ast} of ADSL systems is in the range 65 \ \rm dB ... 68 \ \rm dB. |
| − | * | + | *The VDSL variants provide characteristic cable attenuations between 40 \ \rm dB and 56 \ \rm dB. |
| − | * | + | *It should be noted, however, that this system parameter {\rm a}_{\ast}, which is important in conventional binary baseband transmission, does not reflect the conditions in ''OFDM'' or ''Discrete Multitone Transmission'' sufficiently well. |
{{ML-Fuß}} | {{ML-Fuß}} | ||
| − | [[Category: | + | [[Category:Examples of Communication Systems: Exercises|^2.4 BER Lowering at DSL |
^]] | ^]] | ||
Latest revision as of 19:34, 25 March 2023
The development of xDSL technology began in 1995 with the first standard for \rm ADSL (Asymmetric Digital Subscriber Line). From 2006, the faster \rm VDSL (Very High Data Rate Digital Subscriber Line) also came into use in Germany.
The graph shows five system variants in a diagram in which the achievable cable length l_{\rm max} is plotted as a function of the total bit rate R_{\rm ges} :
- \boldsymbol{\rm A}\text{:} \hspace{0.3cm} \text{ Bitraten} \ 0.2 \ {\rm Mbit/s} + 2 \ {\rm Mbit/s}; \hspace{0.2cm} \text{ cable length } l_{\rm max} \approx 3.5 \ {\rm km},
- \boldsymbol{\rm B}\text{:} \hspace{0.3cm} \text{ Bitraten} \ 0.2 \ {\rm Mbit/s} + 6 \ {\rm Mbit/s}; \hspace{0.2cm} \text{ cable length } l_{\rm max} \approx 2 \ {\rm km},
- \boldsymbol{\rm C}\text{:} \hspace{0.3cm} \text{ Bitraten} \ 2 \ {\rm Mbit/s} + 13 \ {\rm Mbit/s}; \hspace{0.3cm} \text{ cable length } l_{\rm max} \approx 1 \ {\rm km},
- \boldsymbol{\rm D}\text{:} \hspace{0.3cm} \text{ Bitraten} \ 2 \ {\rm Mbit/s} + 26 \ {\rm Mbit/s}; \hspace{0.3cm} \text{ cable length } \ l_{\rm max} \approx 0.8 \ {\rm km},
- \boldsymbol{\rm E}\text{:} \hspace{0.3cm}\text{ Bitraten} \ 2 \ {\rm Mbit/s} + 51 \ {\rm Mbit/s}; \hspace{0.35cm} \text{ cable length } l_{\rm max} \approx 0.4 \ {\rm km}.
To this graphic is to be noted further:
- All data applies to a balanced copper pair with \text{0.4 mm} diameter.
- One of the bit rates given here is for upstream, the other is for downstream.
- The total bitrate is the sum of the two portions.
- Which bit rate refers to the upstream and which to the downstream is asked in the subtask (1).
- The colored differentiation of the drawn points refers to the subdivision into ADSL and VDSL. This is referred to in the subtask (2).
- The curve drawn in blue shows a rule of thumb that approximates the relationship between range and total bit rate:
- l_{\rm max}\,{\rm \big [in}\,\,{\rm km \big]} = \frac {20}{4 + R_{\rm ges}\,{\rm \big[in}\,\,{\rm Mbit/s\big]}} \hspace{0.05cm}.
- Dashed are deviations from this by \pm 25\%.
One often characterizes a wireline transmission system by the cable attenuation at half the bit rate (note the "a" in the attenuation):
- {\rm a}_{\rm \star} = {\rm a}_{\rm K}(f = {R_{\rm B}}/{2}) = \alpha_{\rm K}(f = {R_{\rm B}}/{2}) \cdot l\hspace{0.05cm}.
The attenuation coefficient (noted as "alpha") is given for a \text{0.4 mm} balanced copper pair as follows:
- \alpha_{\rm K}(f ) = \left [ 5.1 + 14.3 \cdot \left ({f}/({\rm 1\,MHz})\right )^{0.59} \right ] {\rm dB}/{\rm km} \hspace{0.05cm}.
For the downlink of variant \boldsymbol{\rm A} with R_{\rm B} = 2 \ \rm Mbit/s thus results with l = l_{\rm max} = 3.5 \ \rm km:
- \alpha_{\rm K}(f = {\rm 1\,MHz}) = \left [ 5.1 + 14.3 \right ] {\rm dB}/{\rm km} = 19.4\,{\rm dB}/{\rm km}\hspace{0.3cm} \Rightarrow \hspace{0.3cm} {\rm a}_{\rm \star} = 19.4\,{\rm dB}/{\rm km} \cdot 3.5\,{\rm km} = 67.9\,{\rm dB}\hspace{0.05cm}.
The values for the other system variants are to be determined in subtask (4).
Hint:
- This exercise belongs to the chapter "Methods to Reduce the Bit Error Rate in DSL".
Questions
Solution
- In all xDSL variants, the downstream is operated at a higher bit rate than the upstream.
- This principle results from user behavior. One fetches much more data to the computer (downstream) than in the reverse direction.
(2) Correct is the second proposed solution:
- VDSL offers higher data rates.
- High data rate, however, is only possible with relatively short line lengths.
(3) The range of such a Gbit/s system over two-wire line would be about 20/1000 \ {\rm km} \underline{= 20 \ \rm meters}.
- Consider this subtask rather academic.
(4) Here the following characteristic cable attenuations result. For
- variant \boldsymbol{\rm B} \ (R_{\rm B}/2 = 3 {\rm \ Mbit/s}, \ l_{\rm max} = 2 {\rm \ km})\text{:}
- {\rm a}_{\rm \star} = \left [ 5.1 + 14.3 \cdot 3^{0.59} \right ] \cdot 2\,{\rm dB}\hspace{0.15cm}\underline{ \approx 64.9\,{\rm dB}}\hspace{0.05cm},
- variant \boldsymbol{\rm C} \ (R_{\rm B}/2 = 6.5 {\rm \ Mbit/s}, \ l_{\rm max} = 1 {\rm \ km})\text{:}
- {\rm a}_{\rm \star} = \left [ 5.1 + 14.3 \cdot 6.5^{0.59} \right ] \cdot 1\,{\rm dB} \hspace{0.15cm}\underline{\approx 48.2\,{\rm dB}}\hspace{0.05cm},
- variant \boldsymbol{\rm D} \ (R_{\rm B}/2 = 13 {\rm \ Mbit/s}, \ l_{\rm max} = 0.8 {\rm \ km})\text{:}
- {\rm a}_{\rm \star} = \left [ 5.1 + 14.3 \cdot 13^{0.59} \right ] \cdot 0.8\,{\rm dB}\hspace{0.15cm}\underline{ \approx 56\,{\rm dB}}\hspace{0.05cm},
- variant \boldsymbol{\rm E} \ (R_{\rm B}/2 = 25.5 {\rm \ Mbit/s}, \ l_{\rm max} = 0.4 {\rm \ km})\text{:}
- {\rm a}_{\rm \star} = \left [ 5.1 + 14.3 \cdot 25.5^{0.59} \right ] \cdot 0.4\,{\rm dB}\hspace{0.15cm}\underline{ \approx 40.7\,{\rm dB}}\hspace{0.05cm}.
Further, it should be noted:
- The characteristic cable attenuation {\rm a}_{\ast} of ADSL systems is in the range 65 \ \rm dB ... 68 \ \rm dB.
- The VDSL variants provide characteristic cable attenuations between 40 \ \rm dB and 56 \ \rm dB.
- It should be noted, however, that this system parameter {\rm a}_{\ast}, which is important in conventional binary baseband transmission, does not reflect the conditions in OFDM or Discrete Multitone Transmission sufficiently well.