Difference between revisions of "Aufgaben:Exercise 2.1Z: Sum Signal"
m (Oezdemir moved page Aufgabe 2.1Z: Summensignal to Exercise 2.1Z: Summing Signal) |
|||
| (13 intermediate revisions by 3 users not shown) | |||
| Line 3: | Line 3: | ||
}} | }} | ||
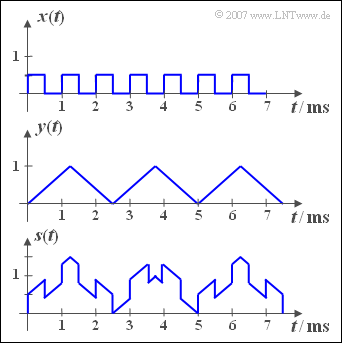
| − | [[File:P_ID319__Sig_Z_2_1.png|right|frame| | + | [[File:P_ID319__Sig_Z_2_1.png|right|frame|Rectangular signal, triangular signal, sum signal]] |
| − | + | The adjacent diagram shows the periodic signals x(t) and y(t), from which the sum s(t) – sketched in the lower diagram – and the difference d(t) are formed. | |
| − | + | Furthermore, in this task we consider the signal w(t), which results from the sum of two periodic signals u(t) and v(t) . The base frequencies of these signals are | |
:* fu=998Hz, | :* fu=998Hz, | ||
| Line 12: | Line 12: | ||
:* fv=1002Hz. | :* fv=1002Hz. | ||
| − | + | That is all we know about the signals u(t) and v(t). | |
| Line 20: | Line 20: | ||
| − | '' | + | ''Hints:'' |
| − | * | + | *The exercise belongs to the chapter [[Signal_Representation/General_Description|General description of periodic signals]]. |
| − | * | + | *With the interactive applet [[Applets:Period_Duration_of_Periodic_Signals|Period Duration of Periodic Signals]] the resulting period duration of two harmonic oscillations can be determined. |
| − | === | + | ===Questions=== |
<quiz display=simple> | <quiz display=simple> | ||
| − | { | + | {What is the period duration Tx and the basic frequency fx of the signal x(t)? |
|type="{}"} | |type="{}"} | ||
fx = { 1 3% } kHz | fx = { 1 3% } kHz | ||
| − | { | + | {What is the period duration Ty and the basic frequency fy of the signal y(t)? |
|type="{}"} | |type="{}"} | ||
fy = { 0.4 3% } kHz | fy = { 0.4 3% } kHz | ||
| − | { | + | {Determine the basic frequency fs and the period duration Ts of the sum signal s(t). <br>Verify your results with the help of the sketched signal. |
|type="{}"} | |type="{}"} | ||
Ts = { 5 3% } ms | Ts = { 5 3% } ms | ||
| − | { | + | {What is the period duration Td of the difference signal d(t) ? |
|type="{}"} | |type="{}"} | ||
Td = { 5 3% } ms | Td = { 5 3% } ms | ||
| − | { | + | {What is the period duration Tw of the signal w(t)=u(t)+v(t)? |
|type="{}"} | |type="{}"} | ||
Tw = { 500 3% } ms | Tw = { 500 3% } ms | ||
| Line 55: | Line 55: | ||
</quiz> | </quiz> | ||
| − | === | + | ===Solution=== |
{{ML-Kopf}} | {{ML-Kopf}} | ||
| − | '''(1)''' | + | '''(1)''' The following applies to the rectangular signal: Tx=1ms ⇒ |
| + | :$$f_x \hspace{0.15cm}\underline{= 1 \, \text{kHz}}.$$ | ||
| + | '''(2)''' The following applies to the triangular signal: Ty=2.5ms und | ||
| + | :fy=0.4kHz_. | ||
| − | + | '''(3)''' The basic frequency fs of the sum signal s(t) is the greatest common divisor fx=1kHz and fy=0.4kHz. | |
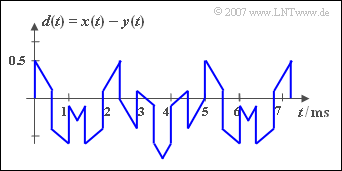
| − | + | [[File:P_ID320__Sig_Z_2_1_d_neu.png|right|frame|Difference d(t)=x(t)−y(t)]] | |
| − | + | ||
| − | + | *From this follows $f_s = 200 \,\text{Hz}$ and the period duration Ts=5ms_. | |
| − | + | * This is also evident from the graphic on the information page. | |
| − | '''(3)''' | ||
| − | |||
| − | |||
| − | |||
| − | |||
| − | [[File:P_ID320__Sig_Z_2_1_d_neu.png|right|frame| | ||
| − | |||
| + | '''(4)''' The period duration Td does not change compared to the period duration Ts, if the signal y(t) is not added but subtracted: | ||
| + | :Td=Ts=5ms_. | ||
| − | '''(5)''' | + | '''(5)''' The greatest common divisor of fu=998Hz and fv=1002Hz is fw=2Hz. The inverse of this gives the period duration Tw=500ms_. |
| − | |||
{{ML-Fuß}} | {{ML-Fuß}} | ||
| − | [[Category: | + | [[Category:Signal Representation: Exercises|^2.1 Description of Periodic Signals^]] |
Latest revision as of 14:33, 12 April 2021
The adjacent diagram shows the periodic signals x(t) and y(t), from which the sum s(t) – sketched in the lower diagram – and the difference d(t) are formed.
Furthermore, in this task we consider the signal w(t), which results from the sum of two periodic signals u(t) and v(t) . The base frequencies of these signals are
- fu=998Hz,
- fv=1002Hz.
That is all we know about the signals u(t) and v(t).
Hints:
- The exercise belongs to the chapter General description of periodic signals.
- With the interactive applet Period Duration of Periodic Signals the resulting period duration of two harmonic oscillations can be determined.
Questions
Solution
- fx=1kHz_.
(2) The following applies to the triangular signal: Ty=2.5ms und
- fy=0.4kHz_.
(3) The basic frequency fs of the sum signal s(t) is the greatest common divisor fx=1kHz and fy=0.4kHz.
- From this follows fs=200Hz and the period duration Ts=5ms_.
- This is also evident from the graphic on the information page.
(4) The period duration Td does not change compared to the period duration Ts, if the signal y(t) is not added but subtracted:
- Td=Ts=5ms_.
(5) The greatest common divisor of fu=998Hz and fv=1002Hz is fw=2Hz. The inverse of this gives the period duration Tw=500ms_.