Difference between revisions of "Aufgaben:Exercise 5.5: Fast Fourier Transform"
m (Text replacement - "Category:Exercises for Signal Representation" to "Category:Signal Representation: Exercises") |
|||
| (2 intermediate revisions by 2 users not shown) | |||
| Line 3: | Line 3: | ||
}} | }} | ||
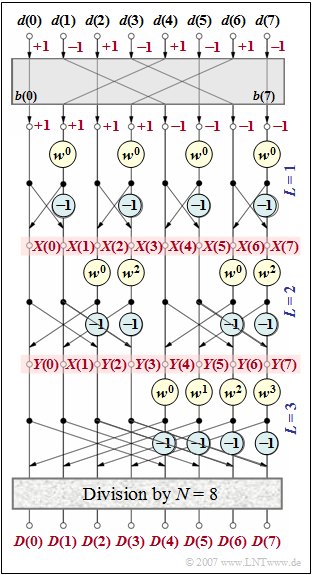
| − | [[File:EN_Sig_A_5_5.png|right|frame|FFT | + | [[File:EN_Sig_A_5_5.png|right|frame|FFT algorithm for N=8]] |
| − | + | The graph shows the signal flow diagram of the Fast Fourier Transform $\rm (FFT)$ for N = 8. | |
| + | |||
| + | The associated spectral coefficients $D(0), \hspace{0.03cm}\text{...} \hspace{0.1cm} , D(7)$ are determined from the time coefficients $d(0), \hspace{0.03cm}\text{...} \hspace{0.1cm}, d(7)$. The following applies to these with 0 ≤ μ ≤ 7: | ||
:$$D(\mu) = \frac{1}{N}\cdot \sum_{\nu = 0 }^{N-1} | :$$D(\mu) = \frac{1}{N}\cdot \sum_{\nu = 0 }^{N-1} | ||
| Line 11: | Line 13: | ||
\hspace{0.05cm}\mu}\hspace{0.05cm},$$ | \hspace{0.05cm}\mu}\hspace{0.05cm},$$ | ||
| − | + | where the complex rotation factor $w = \text{e}^{-\text{j}\hspace{0.05cm} \cdot | |
| − | \hspace{0.05cm}2\pi /N}$ | + | \hspace{0.05cm}2\pi /N}$ is to be used, i.e. $w = \text{e}^{-\text{j}\hspace{0.05cm} \cdot |
\hspace{0.05cm}\pi /4} für N = 8$. | \hspace{0.05cm}\pi /4} für N = 8$. | ||
| − | * | + | *The alternating ±1 sequence \langle\hspace{0.05cm} d(ν)\hspace{0.05cm}\rangle is applied to the input. |
| − | * | + | *After the bit reversal operation, this results in the sequence \langle \hspace{0.05cm}b(\kappa)\hspace{0.05cm}\rangle. |
| − | + | It holds that b(κ) = d(ν), if ν is represented as a dual number and the resulting three bits are written as κ in reverse order. For example | |
| − | * | + | * ν = 1 (binary 001) is followed by κ = 4 (binary 100), |
| − | * | + | * d(2) remains at the same position 2 (binary 010). |
| − | + | The actual FFT algorithm happens for the example N = 8 in \log_2 N = 3 stages, denoted L = 1, L =2 and L = 3. Further: | |
| − | * In | + | * In each stage, four basic operations - so-called '''butterflies''' - are to be performed. |
| − | * | + | * The values at the output of the first stage are designated in this task as X(0),\hspace{0.03cm}\text{...} \hspace{0.1cm} , X(7), <br>those of the second as Y(0), \hspace{0.03cm}\text{...} \hspace{0.1cm} , Y(7). |
| − | * | + | * After the third and last stage, all values must be divided by N. <br>The final result D(0), \hspace{0.03cm}\text{...} \hspace{0.1cm} , D(7) is available here. |
| Line 35: | Line 37: | ||
| − | '' | + | ''Hint:'' |
| − | * | + | *This task belongs to the chapter [[Signal_Representation/Fast_Fourier_Transform_(FFT)|Fast Fourier Transform (FFT)]]. |
| − | === | + | ===Questions=== |
<quiz display=simple> | <quiz display=simple> | ||
| − | { | + | {Calculate the DFT coefficient $D(\mu=3)$. |
|type="{}"} | |type="{}"} | ||
| − | D(3) \ = \ { 0. } | + | $D(\mu=3) \ = \ $ { 0. } |
| − | { | + | {Calculate the DFT coefficient $D(\mu=4)$. |
|type="{}"} | |type="{}"} | ||
| − | D(4) \ = \ { 1 3% } | + | $D(\mu=4) \ = \ $ { 1 3% } |
| − | { | + | {Determine the initial values X(0), ... , X(7) of the first stage. Which of the following statements are true? |
|type="[]"} | |type="[]"} | ||
| − | - | + | - All X values with even indices are equal to 2. |
| − | + | + | + All X values with odd indices are equal to 0. |
| + | |||
| + | {Determine the initial values Y(0), ... , Y(7) of the second stage. Enter the values $Y(0)$ and Y(4) as a check. | ||
| − | |||
|type="{}"} | |type="{}"} | ||
Y(0) \ = \ { 4 3% } | Y(0) \ = \ { 4 3% } | ||
Y(4) \ = \ { -4.12--3.88 } | Y(4) \ = \ { -4.12--3.88 } | ||
| − | { | + | {Calculate all N spectral values D(\mu), in particular |
|type="{}"} | |type="{}"} | ||
| + | D(\mu =3) \ = \ { 0. } | ||
D(\mu = 4) \ = \ { 1 3% } | D(\mu = 4) \ = \ { 1 3% } | ||
| − | |||
| − | { | + | {What would be the spectral coefficients for d(ν = 4) = 1 and d(ν \neq 4) = 0 ? <br>Enter the values $D(\mu =3)$ and $D(\mu =4)$ as a check. |
|type="{}"} | |type="{}"} | ||
D(\mu = 3) \ = \ { -1.03--0.97 } | D(\mu = 3) \ = \ { -1.03--0.97 } | ||
| Line 76: | Line 79: | ||
</quiz> | </quiz> | ||
| − | === | + | ===Solution=== |
{{ML-Kopf}} | {{ML-Kopf}} | ||
| − | '''(1)''' | + | '''(1)''' According to the general DFT equation given on the specification sheet, with $w = \text{e}^{-\text{j}\hspace{0.05cm} \cdot |
| − | \hspace{0.05cm}\pi /4}$ | + | \hspace{0.05cm}\pi /4}$ taking into account the alternating time coefficients: |
:$$8 \cdot D(3) = w^0 - w^3 + w^6- w^9+ w^{12}- w^{15}+ w^{18}- | :$$8 \cdot D(3) = w^0 - w^3 + w^6- w^9+ w^{12}- w^{15}+ w^{18}- | ||
| Line 86: | Line 89: | ||
w^{5}\hspace{0.05cm}.$$ | w^{5}\hspace{0.05cm}.$$ | ||
| − | * | + | *Here it is taken into account that due to the periodicity w_9 = w_1, w_{12} = w_4, w_{15} = w_7, w_{18} = w_2 und w_{21} = w_5 ist. |
| − | * | + | *After re-sorting, the same applies: |
:8 \cdot D(3) = (w^0 + w^4) - (w^1 + w^5)+ (w^2 + w^6) - (w^3 + w^7) = (1 + w + w^2+ w^3) \cdot (w^0 + w^4)\hspace{0.05cm}. | :8 \cdot D(3) = (w^0 + w^4) - (w^1 + w^5)+ (w^2 + w^6) - (w^3 + w^7) = (1 + w + w^2+ w^3) \cdot (w^0 + w^4)\hspace{0.05cm}. | ||
| − | * | + | *Thus, because w_0 = 1 and w_4 = \text{e}^{-\text{j}\pi } = \hspace{0.08cm} - \hspace{-0.08cm}1 , we obtain $\underline {D(\mu=3) = 0}$. |
| − | '''(2)''' In | + | '''(2)''' In analogy to sub-taske '''(1)''' , we now get: |
:$$ 8 \cdot D(4) = w^0 - w^4 + w^8- w^{12}+ w^{16}- w^{20}+ | :$$ 8 \cdot D(4) = w^0 - w^4 + w^8- w^{12}+ w^{16}- w^{20}+ | ||
w^{24}- w^{28}= 4 \cdot (w^0 - w^4)= 8 \hspace{0.3cm} | w^{24}- w^{28}= 4 \cdot (w^0 - w^4)= 8 \hspace{0.3cm} | ||
| − | \Rightarrow \hspace{0.3cm}\hspace{0.15 cm}\underline{D(4) = 1}\hspace{0.05cm}.$$ | + | \Rightarrow \hspace{0.3cm}\hspace{0.15 cm}\underline{D(\mu=4) = 1}\hspace{0.05cm}.$$ |
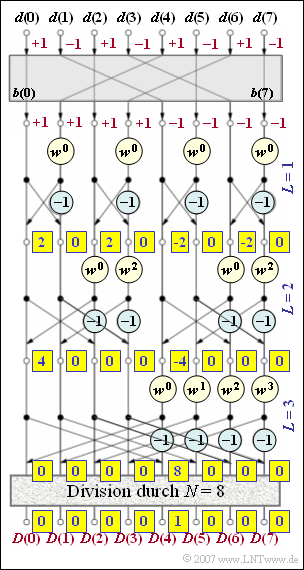
| − | [[File:P_ID1178__Sig_A_5_5c_neu.png|right|frame| | + | [[File:P_ID1178__Sig_A_5_5c_neu.png|right|frame|Example for the FFT algorithm]] |
| − | '''(3)''' | + | '''(3)''' <u>Proposed solution 2</u> is correct: |
| − | * | + | *The term w^0 = 1 does not have to be taken into account. |
| − | * | + | *All output values with odd indices are zero by subtracting two identical input values. |
| − | * | + | *The first statement is not true: It holds X(0) = X(2) = +2 and X(4) = X(6) = - 2. |
| − | '''(4)''' | + | '''(4)''' The multiplication with w^{2} = -{\rm j} can be dispensed with, since in the signal flow diagram the corresponding input values are zero. |
| − | * | + | *One thus obtains Y(0) \;\underline{= 4} and Y(4) \;\underline{= - \hspace{-0.03cm}4}. |
| − | * | + | *All other values are zero. |
| − | '''(5)''' | + | '''(5)''' Because of Y(5) = Y(6) =Y(7) = 0, the multiplications with w, w^2 and w^3 do not matter in the third stage either. All spectral coefficients D(\mu) therefore result in zero with the exception of |
| − | :$$\hspace{0.15 cm}\underline{D(4)} = {1}/{N}\cdot \left[Y(0) - Y(4) \right ] \hspace{0.15 cm}\underline{= 1} | + | :$$\hspace{0.15 cm}\underline{D(\mu=4)} = {1}/{N}\cdot \left[Y(0) - Y(4) \right ] \hspace{0.15 cm}\underline{= 1} |
| + | \hspace{0.05cm},$$ | ||
| + | :$$\hspace{0.15 cm}\underline{D(\mu=3)} = D(\mu\ne 4) \hspace{0.15 cm}\underline{= 0} | ||
\hspace{0.05cm}.$$ | \hspace{0.05cm}.$$ | ||
| − | + | This result agrees with the results from '''(1)''' und '''(2)'''. | |
| − | '''(6)''' | + | '''(6)''' Since both the time coefficients d(ν) and all spectral coefficients D(\mu) are purely real, there is no difference between the FFT and the IFFT. |
| − | * | + | *This means at the same time: The input and output values can be interchanged. |
| − | * | + | *Subtask '''(5)''' gave the following result: |
| − | :$$d({\rm | + | :$$d({\rm even}\hspace{0.15cm}\nu) = +1, \hspace{0.2cm}d({\rm |
| − | + | odd}\hspace{0.15cm}\nu)= -1$$ | |
:$$\Rightarrow | :$$\Rightarrow | ||
\hspace{0.3cm}D(\mu = 4)= 1,\hspace{0.2cm}D(\mu \ne 4)= 0.$$ | \hspace{0.3cm}D(\mu = 4)= 1,\hspace{0.2cm}D(\mu \ne 4)= 0.$$ | ||
| − | * | + | *By swapping the input and output values, we arrive at problem '''(6)''': |
:$$d(\nu = 4)= 1, \hspace{0.2cm}d(\nu \ne 4)= 0 \hspace{0.3cm} | :$$d(\nu = 4)= 1, \hspace{0.2cm}d(\nu \ne 4)= 0 \hspace{0.3cm} | ||
| Line 141: | Line 146: | ||
\hspace{0.05cm}.$$ | \hspace{0.05cm}.$$ | ||
| − | * | + | *In particular, this results in $D(\mu=3) \; \underline{= -1} und D(\mu=4) \; \underline{= +1}$. |
{{ML-Fuß}} | {{ML-Fuß}} | ||
__NOEDITSECTION__ | __NOEDITSECTION__ | ||
[[Category:Signal Representation: Exercises|^5.5 Fast Fourier Transform ^]] | [[Category:Signal Representation: Exercises|^5.5 Fast Fourier Transform ^]] | ||
Latest revision as of 18:08, 21 May 2021
The graph shows the signal flow diagram of the Fast Fourier Transform \rm (FFT) for N = 8.
The associated spectral coefficients D(0), \hspace{0.03cm}\text{...} \hspace{0.1cm} , D(7) are determined from the time coefficients d(0), \hspace{0.03cm}\text{...} \hspace{0.1cm}, d(7). The following applies to these with 0 ≤ μ ≤ 7:
- D(\mu) = \frac{1}{N}\cdot \sum_{\nu = 0 }^{N-1} d(\nu) \cdot {w}^{\hspace{0.03cm}\nu \hspace{0.05cm} \cdot \hspace{0.05cm}\mu}\hspace{0.05cm},
where the complex rotation factor w = \text{e}^{-\text{j}\hspace{0.05cm} \cdot \hspace{0.05cm}2\pi /N} is to be used, i.e. w = \text{e}^{-\text{j}\hspace{0.05cm} \cdot \hspace{0.05cm}\pi /4} für N = 8.
- The alternating ±1 sequence \langle\hspace{0.05cm} d(ν)\hspace{0.05cm}\rangle is applied to the input.
- After the bit reversal operation, this results in the sequence \langle \hspace{0.05cm}b(\kappa)\hspace{0.05cm}\rangle.
It holds that b(κ) = d(ν), if ν is represented as a dual number and the resulting three bits are written as κ in reverse order. For example
- ν = 1 (binary 001) is followed by κ = 4 (binary 100),
- d(2) remains at the same position 2 (binary 010).
The actual FFT algorithm happens for the example N = 8 in \log_2 N = 3 stages, denoted L = 1, L =2 and L = 3. Further:
- In each stage, four basic operations - so-called butterflies - are to be performed.
- The values at the output of the first stage are designated in this task as X(0),\hspace{0.03cm}\text{...} \hspace{0.1cm} , X(7),
those of the second as Y(0), \hspace{0.03cm}\text{...} \hspace{0.1cm} , Y(7). - After the third and last stage, all values must be divided by N.
The final result D(0), \hspace{0.03cm}\text{...} \hspace{0.1cm} , D(7) is available here.
Hint:
- This task belongs to the chapter Fast Fourier Transform (FFT).
Questions
Solution
- 8 \cdot D(3) = w^0 - w^3 + w^6- w^9+ w^{12}- w^{15}+ w^{18}- w^{21} = w^0 - w^3 + w^2- w^1+ w^{4}- w^{7}+ w^{6}- w^{5}\hspace{0.05cm}.
- Here it is taken into account that due to the periodicity w_9 = w_1, w_{12} = w_4, w_{15} = w_7, w_{18} = w_2 und w_{21} = w_5 ist.
- After re-sorting, the same applies:
- 8 \cdot D(3) = (w^0 + w^4) - (w^1 + w^5)+ (w^2 + w^6) - (w^3 + w^7) = (1 + w + w^2+ w^3) \cdot (w^0 + w^4)\hspace{0.05cm}.
- Thus, because w_0 = 1 and w_4 = \text{e}^{-\text{j}\pi } = \hspace{0.08cm} - \hspace{-0.08cm}1 , we obtain \underline {D(\mu=3) = 0}.
(2) In analogy to sub-taske (1) , we now get:
- 8 \cdot D(4) = w^0 - w^4 + w^8- w^{12}+ w^{16}- w^{20}+ w^{24}- w^{28}= 4 \cdot (w^0 - w^4)= 8 \hspace{0.3cm} \Rightarrow \hspace{0.3cm}\hspace{0.15 cm}\underline{D(\mu=4) = 1}\hspace{0.05cm}.
(3) Proposed solution 2 is correct:
- The term w^0 = 1 does not have to be taken into account.
- All output values with odd indices are zero by subtracting two identical input values.
- The first statement is not true: It holds X(0) = X(2) = +2 and X(4) = X(6) = - 2.
(4) The multiplication with w^{2} = -{\rm j} can be dispensed with, since in the signal flow diagram the corresponding input values are zero.
- One thus obtains Y(0) \;\underline{= 4} and Y(4) \;\underline{= - \hspace{-0.03cm}4}.
- All other values are zero.
(5) Because of Y(5) = Y(6) =Y(7) = 0, the multiplications with w, w^2 and w^3 do not matter in the third stage either. All spectral coefficients D(\mu) therefore result in zero with the exception of
- \hspace{0.15 cm}\underline{D(\mu=4)} = {1}/{N}\cdot \left[Y(0) - Y(4) \right ] \hspace{0.15 cm}\underline{= 1} \hspace{0.05cm},
- \hspace{0.15 cm}\underline{D(\mu=3)} = D(\mu\ne 4) \hspace{0.15 cm}\underline{= 0} \hspace{0.05cm}.
This result agrees with the results from (1) und (2).
(6) Since both the time coefficients d(ν) and all spectral coefficients D(\mu) are purely real, there is no difference between the FFT and the IFFT.
- This means at the same time: The input and output values can be interchanged.
- Subtask (5) gave the following result:
- d({\rm even}\hspace{0.15cm}\nu) = +1, \hspace{0.2cm}d({\rm odd}\hspace{0.15cm}\nu)= -1
- \Rightarrow \hspace{0.3cm}D(\mu = 4)= 1,\hspace{0.2cm}D(\mu \ne 4)= 0.
- By swapping the input and output values, we arrive at problem (6):
- d(\nu = 4)= 1, \hspace{0.2cm}d(\nu \ne 4)= 0 \hspace{0.3cm} \Rightarrow \hspace{0.3cm}D({\rm gerades}\hspace{0.15cm}\mu) = +1, \hspace{0.2cm}D({\rm ungerades}\hspace{0.15cm}\mu)= -1 \hspace{0.05cm}.
- In particular, this results in D(\mu=3) \; \underline{= -1} und D(\mu=4) \; \underline{= +1}.